Version française abrégée
Dans un livre historique, Galilée évoque la nature comme un grand livre, écrit en mots de mathématiques, dont les lettres sont le cube, la sphère, le cône, etc. Ces « patrons » de formes fondamentales sont en effet observés dans la nature. La bulle de savon, le cristal de chlorure de sodium, l'extrémité de certains fruits ou épines en sont des exemples. Il existe des explications physiques simples à l'existence de sphères dans la nature, et il existe des explications, certes plus sophistiquées, à l'existence de cubes (ou plus généralement de surfaces facettées comme les cristaux). À notre connaissance, il n'existe pas d'explication simple de l'existence de formes en fuseau, ou en cône. Or, on observe rarement des formes strictement sphériques, particulièrement en biologie. Souvent, les formes les plus simples se rapprochant de la sphère sont ovoı̈des, fusiformes ou pointues. À l'inverse, d'autres formes présentent une ré-entrance en un centre, comme par exemple les pommes ou les citrouilles. Bien entendu, il existe des exceptions, mais ces exceptions touchent surtout des structures à l'échelle cellulaire. Les arguments invoquant la polarité des structures, comme, par exemple, l'anisotropie des végétaux, courent le risque du raisonnement circulaire, si elles justifient l'anisotropie de croissance par l'existence d'une anisotropie du tissu, et réciproquement.
Nous montrons dans cet article qu'une des raisons de l'existence spontanée de cônes, ou de formes en fuseau, est le caractère fibré des tissus vivants. Des arguments issus de la physique, en particulier, la théorie de l'équilibre des formes cristallines, nous permettent de montrer, dans l'hypothèse la plus simple, qu'il doit exister des pointes au sommet des végétaux de formes sphéroı̈dales, s'ils sont faits d'un arrangement de cellules ou de fibres azimutal, ou méridien, ce qui est presque toujours le cas. De même, un petit domaine orienté circulairement (comme par exemple une condensation de fibroblastes) présentera naturellement une pointe en son centre, prémisse d'un poil ou d'une épine. Cette théorie simplifiée rapproche la formation d'une structure fibrée de la croissance cristalline, tout en montrant en quoi elle diffère. L'analogue physique le plus proche est le cristal liquide, présentant des domaines spontanément orientés. Ainsi, l'interaction entre microstuctures polaires induit des champs d'orientation macroscopiques présentant des singularités, et ces singularités définissent les axes privilégiés apparents sur la forme. Cet enchaı̂nement causal lève la circularité du raisonnement évoquée ci-dessus.
En s'inspirant des cristaux, on observera que, en croissance cristalline, la présence de coins dans ceux-ci est due à des anisotropies de tension de surface, en sorte que l'équilibre de l'interface dépend de l'orientation de la surface du cristal par rapport au réseau cristallin sous-jacent. Dans le cas d'une structure fibrée, il n'y a évidemment pas de réseau cristallin sous-jacent. Cependant, l'existence du champ d'orientation des fibres, auto-organisé, modifie l'énergie de la surface et crée des forces de tension de cette surface, qui dépendent du tracé des fibres dans la surface. Ainsi, il existera des forces de surface variables, dépendant non pas de l'orientation de la surface par rapport à un axe absolu (comme dans les cristaux), mais dépendant du tracé local du dessin de fibres. Mais, par ailleurs, le tracé local du dessin de fibres est conditionné par la forme globale de l'organe ou de l'organisme. En tout état de cause, si le champ de fibres n'est pas quelconque, aléatoire, homogène, il existera un tracé comportant un certain nombre de singularités, ou « pôles ». Dans ce cas, la physique montre qu'une pointe « sort » de la surface, dans la direction des « pôles » ou singularités de l'arrangement de fibres. Ceci explique naturellement la présence de pointes sur les citrons, les noyaux d'avocats ou les amandes, mais aussi à l'extrémité du corps des insectes, au sommet des spores de certains champignons, voire des proéminences observées à l'extrémité des doigts, du côté de la pulpe. La forme en fuseau, du fuseau mitotique au corps des poissons, apparaı̂t comme une forme très naturelle, à condition de prendre en compte un aspect matériel du tissu vivant : le caractère fibré.
Dans le détail, cette théorie ne peut viser à la généralité, mais elle explique un aspect universel des structures fibrées : leur polarité. Cette conséquence inverse le sens d'un certain nombre d'observations classiques. Ainsi, dans un végétal ou un organe donné d'une plante (citron, épine, floret, etc.) l'apparition d'une structure en pointe pourra classiquement être attribuée à l'existence d'une orientation, due par exemple à un comportement particulier de cellules apicales au sommet de la structure, provoquant une croissance anisotrope et l'émergence d'une pointe. Cette explication par l'existence d'un axe privilégié suppose par ailleurs une explication de l'existence de cet axe (pour échapper au cercle vicieux). En réalité, l'observation d'une structure fibrée dans ces organes ou organismes lève le cercle vicieux, en rendant inévitable l'existence de singularités, donc l'existence d'anisotropies, et donc l'existence de différentiels de croissance dans des directions choisies et stabilisées par l'anisotropie. C'est une observation courante que les directions de croissance sont congruentes avec les directions des fibres. L'anisotropie de croissance apparaı̂t comme une conséquence de la structure matérielle du tissu, elle-même conséquence du fait que la majorité des molécules du vivant sont des polymères. Ce rapprochement est sans doute ancien, mais il n'existait pas, à notre connaissance, de modèle simple, global, permettant de tracer la forme d'une structure fibrée autour d'un nombre restreint de pôles.
À première vue, un modèle simple n'invoquant qu'une énergie interfaciale de nature thermodynamique a une portée très limitée. Il faut noter que, du point de vue de la physique, la principale différence entre l'existence de pointes dans des structures microscopiques, comme des membranes cellulaires, et des structures macroscopiques, comme des citrons ou des amandes tient au type de variable dont la force (tension de surface) découle. Cependant, l'anisotropie a, quant à elle, la même origine orientationnelle dans les deux cas (orientation des molécules à la surface de la cellule dans un cas, des cellules à la surface du fruit ou de l'organisme dans l'autre). Dans le cas d'une structure microscopique, comme une membrane comportant en son sein des molécules orientées, on pourra faire dériver la force d'une énergie, éventuellement proportionnelle à la surface (énergie interfaciale), alors que, pour un objet macroscopique, il faudrait en principe traiter complètement les lois de l'élasticité, ce qui ne sera pas traité ici, car les lois de comportement d'un tissu vivant en cours de morphogenèse ne sont pas connues avec suffisamment de précision, et sont même peu intuitives. Nous nous contenterons de décrire le cas simple où l'énergie est proportionnelle à l'aire. (Les lois de comportement dont il est question sont celles de la morphogenèse, il ne s'agit pas de la loi de comportement instantanée d'un morceau de tissu vivant considéré comme un matériau. Par exemple, si l'on considère une tige de bois, elle fléchit et s'allonge comme un solide ordinaire au sens de la mécanique ; en revanche, en cours de morphogenèse, elle présente des comportements très inhabituels pour un solide, comme, par exemple, la faculté de s'allonger dans le sens opposé aux forces qui s'exercent sur elle, comme la gravité.)
Cependant, le cas particulier d'une énergie microscopique (thermodynamique) proportionnelle à l'aire est plus général, et convient, dans certains cas, à la description d'un objet macroscopique. Si l'on suppose, en effet, qu'il existe une homéostase mécanique des tissus, de telle sorte que, en régime de croissance lente, quasi-statique, les cellules maintiennent une tension de consigne fixée, identique pour chacune d'entre elles (hypothèse implicite pour les tissus type peau, épiderme, tuniques, etc.), alors l'équilibre d'une assemblée de cellules fixant ainsi leur tension individuelle s'exprime exactement comme l'équilibre d'une membrane ayant une énergie thermodynamique proportionnelle à l'aire. Dans le premier cas, il existe une énergie thermodynamique qui dérive d'une aire, et entraı̂ne l'existence d'une force linéique égale à l'énergie interfaciale ; dans le second cas, il existe une force linéique fixée par la cellule, indépendamment de tout concept d'énergie, mais l'équilibre vectoriel des forces est le même.
Notre modèle est également simpliste en ce qu'il ne traite qu'une surface simple, alors que la plupart des tissus vivants, et particulièrement les végétaux, sont composés de couches successives, présentant souvent des structures différentes (fibres orientées perpendiculairement, comme sur les peaux d'oignons, ou cellules de natures différentes d'une couche à l'autre, comme les cellules en palissade et les cellules en pièces de puzzle des feuilles végétales). L'existence de ces couches successives peut conduire à des phénomènes qui ne sont pas évoqués ici, comme des stress uni-axiaux (générateurs de plis, absents de notre modèle simple), invoqués depuis quelques années pour expliquer la morphogenèse des méristèmes, et, particulièrement, l'organisation phyllotactique.
Le modèle simple repose donc sur l'introduction d'une tension de surface (force tangentielle), qui dépend de l'orientation, mais à travers le dessin de fibres tracé sur l'organe ou l'organisme. Le modèle part d'une sphère homogène, mais fibrée. On sait qu'il est impossible de fibrer une sphère « parfaitement », c'est-à-dire sans laisser des points où l'orientation n'est pas définie. Ces points sont les « pôles », bien connus dans les cas les plus simples, où la sphère est fibrée suivant des méridiens et des parallèles. Au pôle, l'orientation n'est pas un vecteur bien défini, le champ de vecteur associé présente une singularité. Nous montrons que, en direction des pôles, l'aspect fibré induit un raidissement de la surface, soit que les fibres soient plus concentrées (effet de convergence des méridiens), soit que les fibres soient plus serrées (effet de rétrécissement des parallèles). Dans les deux cas, la tension de surface est modifiée. Dans le premier cas, la tension de surface augmente directement (concentration des fibres) ; dans le second cas, c'est le couple de rotation qui augmente ; or, on sait que le couple est égal à la dérivée le long de la surface de la tension de surface. Donc ces deux cas, méridien et parallèle, se comprennent simplement comme un cas où la tension augmente, et comme un cas où la dérivée de la tension augmente, ce qui relie simplement les deux modèles. Dans les deux cas, la tension présente une divergence aux pôles, qui se traduit par un angle parfait au sommet, où la structure est conique au-delà d'une certaine « latitude ». Ce cône est d'autant plus pointu que l'effet est important. On trouve ainsi un ensemble de formes, de la sphère à l'épine.
Ce modèle très simple, et qui ne prétend pas prendre en compte toute la réalité de la mécanique du tissu, explique qualitativement un ensemble de faits très intuitifs. Le premier est que les formes fibrées sont polaires ; la brisure de symétrie microscopique, moléculaire, induit une anisotropie de l'organisation, macroscopique. Une conséquence de « l'effet de pointe vers les pôles » est que les formes fibrées sont d'autant plus pointues quelles sont dures. Ainsi, le caractère pointu, et le caractère dur, viennent ensemble. Il n'est pas nécessaire, « pour la nature », d'évoluer indépendamment le caractère pointu et le caractère dur. On comprend l'intérêt de cette particularité des tissus orientés, pour la fabrication de défenses, telles que dards, épines, pointes, cornes, etc. La présence de ces organes devient une conséquence presque automatique de l'orientation des tissus et du changement d'une propriété matérielle du tissu, ce changement (par exemple, la présence d'une épine ou d'une feuille à l'aisselle d'une branche de citronnier) pouvant parfaitement avoir, quant à lui, une cause génétique. Une autre conséquence de ce phénomène est que les végétaux en couches successives doivent se présenter comme des fuseaux emboı̂tés, dont les fuseaux internes sont plus « tendus », et non comme des coques sphériques concentriques, ce qui est bien le cas.
Le second fait est que la taille des objets influe sur leur forme, à topologie ou bauplan constant. Ceci est très différent du cas des cristaux. En cristallographie, la forme d'un cristal est indépendante de sa taille, car la tension de surface ne dépend que du voisinage immédiat des atomes et de l'orientation dans le réseau cristallin. L'orientation dans le réseau est un concept indépendant de la taille. Ce fait a une importance historique très grande. En effet, l'abbé Haüy avait remarqué que tous les cristaux de calcites avaient la même forme, quelle que soit leur taille, et il en avait déduit, en extrapolant cette observation vers l'infiniment petit, que les solides cristallins étaient composés de l'addition côte à côte de « molécules intégrantes » identiques (ce qui marque la naissance de la physique du solide). Ainsi, le caractère homothétique de la forme d'équilibre d'un cristal renseigne sur la physique à la plus petite échelle. Dans le cas d'un arrangement de fibres, la tension de surface dépend de la courbure des fibres, de leur convergence ou divergence ; or, la courbure des fibres, ou leur convergence dans une surface, dépendent de la taille globale de cette surface, à géométrie constante. Ainsi, à topologie de lignes identique, un objet plus petit tend à être plus pointu qu'un objet plus grand, fait moins intuitif que pour les cristaux. Ceci peut être une des explications du changement de forme de certaines structures naturelles, lorsque leur taille augmente (bien des légumes, par exemple, deviennent plus ronds en grandissant). (L'autre explication est l'augmentation du volume relatif d'eau, par rapport au tissu sec, ce qui induit un changement de la physique de l'interface : la tension est plus grande, mais plus isotrope, le rôle des fibres étant moindre.) Cette explication, évidemment, est réduite à des structures telles que des épines ou des sphéroı̈des.
Il existe des cas où des croissances présentent un degré élevé de self-similarité, comme la croissance itérative de nombre d'arbres, ou, comme observé plus récemment, les solutions auto-translatées obtenues en tip-growth. Dans ce dernier cas, on peut obtenir la solution mathématique d'un tube, modélisant, par exemple, une radicelle présentant un bout arrondi se perpétuant éternellement, parce qu'il existe un état stationnaire. Le tube au bout arrondi « se pousse lui-même » vers l'avant perpétuellement de façon élasto-plastique, son profil se translate au cours du temps. Pour une forme de type oignon, navet, citron, etc., notre modèle prédit qu'il n'existe pas de solution self-similaire, homothétique, mais une famille de solutions, dont les profils changent avec la taille. Par conséquent, la distinction usuelle entre morphogenèse et croissance n'est pas complètement fondée : une simple croissance peut induire un changement de forme. A contrario, la physique prédit que la forme influe sur la croissance, l'anisotropie des propriétés physiques induisant des vitesses de croissance différentes suivant des directions différentes.
Où se situe ce modèle par rapport aux modèles de réaction–diffusion ? Il faut insister sur le fait que les modèles de morphogenèse qui ne prennent pas en compte cet aspect matériel du tissu vivant sont probablement incomplets. Depuis les travaux fameux de Turing, on traite le tissu vivant à l'aide de champs de concentration censés représenter l'activité mitotique ou des gradients de molécules d'adhésion. Cette vue des choses néglige la structure même du matériau vivant. On ne saurait limiter l'information de position à un scalaire (une concentration) lorsque le vecteur orientation, et la courbure, jouent un si grand rôle dans la description la plus simple d'une surface. Un exemple très frappant est celui des condensations chondrogéniques des os, des cartilages, des poils ou plumes, etc. Dans les modèles existant, des couples de réaction–diffusion « à la Turing » sont censés représenter l'apparition de foyers où se concentrent les cellules (fibroblastes). Le caractère orienté du tissu est complètement absent de ces modèles. Or les images mêmes ayant servi à produire ces modèles montrent que les fibroblastes sont orientés, y compris aux stades où ces modèles sont utilisés, et que les condensations chondrogéniques sont déjà des structures présentant un ordre « méridien–parallèles » jouant un rôle dans leur morphogenèse. Il est clair que si les fibroblastes forment des domaines analogues à des cristaux liquides, l'existence de singularités des domaines peut induire naturellement des pointes. Or, il est bien connu que la formation de domaines crée naturellement des réseaux carrés, hexagonaux, en zigzag, etc. ; par conséquent, il est tentant de suggérer que les réseaux de plumes ou de poils s'auto-organisent à partir de domaines de type cristaux liquides.
Plus récemment, un gène a été découvert, Spike1, réputé être le gène de la morphogenèse des feuilles. Qiu et al. montrent que les feuilles normales et les feuilles mutées ont des formes différentes (ronde ou lenticulaires), ce qu'ils expliquent par le fait que les cellules individuelles de ces feuilles ont des formes différentes (en pièce de puzzle ou en boı̂tes rectangulaires). La transition de morphologie s'explique donc simplement par un changement de conformation des cellules : isotrope–anisotrope, mais un maillon manque pour relier ce changement à la forme globale de la feuille, carence que cet article peut contribuer à combler.
1 Introduction
Is the book of nature really written with spheres? It is a common observation that spheres are not quite frequent in the biocenose. Unicellular organisms often depart from sphericity, except for the simplest cells, and, among the variety of simple organs or fruits, one often finds ovals or spindle-like structures, which depart from the sphere in the direction of apparent ‘poles’. We will present here a simple explanation for these facts. The polarity of the form may be simply due to the fact that the biological tissue is most generally fibred. Simple theoretical arguments from theory of crystal growth let us predict that spindle-like shapes are much more general, the sphere being only one limiting case of a family of shapes between sharp pins and spindles down to featureless spheres. In order to do so, we will propose a simple model of tissue equilibrium, in which the tension of the ‘living material’ will be the principle component. We will show that, if the fibred nature of the tissue is taken into account, then a picture very different from what is generally found in physics or mechanics of solid-state material is found. Especially, biological shapes will appear as structures whose outer form is controlled by an orientational field instead of a crystal lattice, with sharp features in the direction of orientational singularities instead of specific crystalline axis.
In crystals, the outer form is controlled by the absolute, non-local, crystalline planes that determine specific directions globally. Some of these directions are preferred thermodynamically, so that crystal surfaces exhibit facets that join at ‘corners’. These corners exclude the less favorable directions (in thermodynamical terms) given by the angles absent between the two planes that join at the corner.
In biological shapes, the outer form is controlled by the local orientational field, which determines the global shape through the self-organization of the orientational dynamics. Specific directions (‘polarity’) are determined by orientation singularities that are associated to stiffness anisotropies that exclude certain angles (hence the cone or spindle-like structure).
Our approach will be over-simplified, in that we shall not treat the complete mechanical problem. In addition to be of formidable complexity, the constitutive equations of a growing self-adapting living tissue (even the simplest vegetal or animal tissue) is simply not established [1–4]. Also, most vegetal or animal tissues, even the simplest of them, are composite, and generally made of several sheets superimposed or self-surrounding in a Russian-doll fashion [[2] and references therein]. The scope of this article is limited to a single spheroidal shell, whose shape will depart from sphericity because of its fibered nature.
Considering the difference in nature between a membrane at a molecular level, and a round shell at the level of an organ or organism, one may think that no general morphogenetic features can be established, and that each system should be addressed with different formalisms. While this is largely true, still, there exist at least two reasons to expect a similarity between membrane structures (at the molecular level) and the shape of organisms (made of individual cells). First of all, the origin of polarity in these systems as assumed here, is the same: the orientational order. In the case of membranes, the orientational order is due to the presence of elongated biopolymers, at the molecular level. In the case of macroscopic tissue, the order is due either to the anisotropy of individual cells, or to bundles of macromolecules forming elongated strands. The second reason is that there exists something common to the physics of a membrane, with a thermodynamic description, and the physics of a tissue undergoing morphogenesis in the simplest biomechanical description. Indeed, the form of interfaces can be derived, in the thermodynamical framework, from interfacial energy, and free-energy arguments [5,6]. A consequence of the existence of an interfacial energy is that there exists a surface force called simply surface tension. In the simplest case, the interfacial energy is a constant, and so is the surface tension. The equilibrium shape then stems from thermodynamic equilibrium of a given interface whose energy is proportional to the area. There is of course nothing like that for complete organisms or organs. Their tissue does not undergo thermal fluctuations, and there is no simple free energy from which the forces can be derived. One has to invoke elasto-plastic models of growth, which include viscous terms, and creep terms, which have only recently been studied at a quantitative level [3]. However, if we consider a living tissue as composed of cells that behave in such a way that they reach a homeostatic set-point of tension, then, although there is no free energy, there exists a local tension that, in the simplest case, is constant at the level of an individual cell (if we suppose a homogeneous tissue, with a quasi-static growth). Then, the mechanical equilibrium of an organ surface becomes identical to the equilibrium of a membrane in the classical molecular meaning. Of course, the homeostatic set point of the cell is reached by completely different means than the interaction between molecules, however there is a formal correspondence: tensile mechanostasis (macroscopic) ⇔ interaction energy (microscopic). This being said, the morphogenesis of a simple crystal, with a simple surface tension, becomes a possible start for the general study of the origin of spindle-like shapes.
Growth and form of crystals is a very important question in several fields of science. It has received a considerable attention in materials science [5]. It is well known that the surface tension determines the equilibrium shape of a crystal [6]. The out-of-equilibrium shape is that of a growing tree-like structure [7]. This tree is organized into a regular dendrite that branches along crystallographic directions, when the surface tension is anisotropic [7]. There exists already evidence that concepts from crystallogenesis may apply to biology, including to growth of plants [8] and of organs [9]. However, the origin of anisotropy, in materials science, lies in the long range ordering of atoms in a lattice, with straight alignments. There exist specific directions, namely the crystallographic axis, which imposes orientations, and polarities, which are defined absolutely. This is absent in biology. In biology the order of the tissue is that of a nematoid order of cells and fibers [10–14], which tend to form curvilinear alignments like epidermal ridges. The alignments are defined locally, and there is no absolute reference for the direction of the features (lines, fibers, folds, etc.). In the case of fibers, these are often bundled into rings, as seen for example in plants, in muscles, and in the abdomen of insects. The origin of this structure is often in centrifugal mitosis, which gives for example the annual rings of trees. The impact of this order on function of biological structures is already a matter of scientific interest (especially in the case of the heart [14]). Please note that there is a risk of a circular reasoning, which would ascribe the positioning of the orientational order to the existence of a polarity, and vice-versa. A way to avoid such a circular reasoning is to suppose the existence of specialized groups of cells (e.g., apical cells) that impose an anisotropy, which then lays down the fiber orientation. However, since field singularities induce strong variations of physical properties (especially, stresses) it is tempting to suggest, as the following formal derivation suggests, that the existence of an orientational order suffices to generate topological singularities, which in turn induce morphological consequences and lift the vicious-circle problem.
As a first step towards this demonstration, we propose a simple equilibrium description of a fibred shell or ‘pouch’, with features arranged in concentric circles around a singularity (called the ‘pole’ in the sequel). Our first example addresses a stack of rings, in azimuthal direction. The second example addresses the case of fibers running in a meridian fashion towards two opposite poles. In order to derive the mathematical construction of such a fibred surface, we need to explain the construction of crystal shapes.
2 The surface stiffness
Biologists and physicists are used to introducing a surface tension, say γ, in their problems. However, the surface tension, especially in biology, is generally considered as a constant, and the relation between the geometry and the surface tension is classically written (Eq. (1)):
| (1) |
In fact, the surface tension is generally not a constant. This is especially true in problems where an anisotropy exists. In this case, for example if we consider a 2D surface, in radial coordinates, the surface tension may vary with a polar angle, with a surface tension given by some γ(θ) function. In that case, Eq. (1) is modified and becomes, for certain class of problems to which we restrict ourselves:
| (2) |
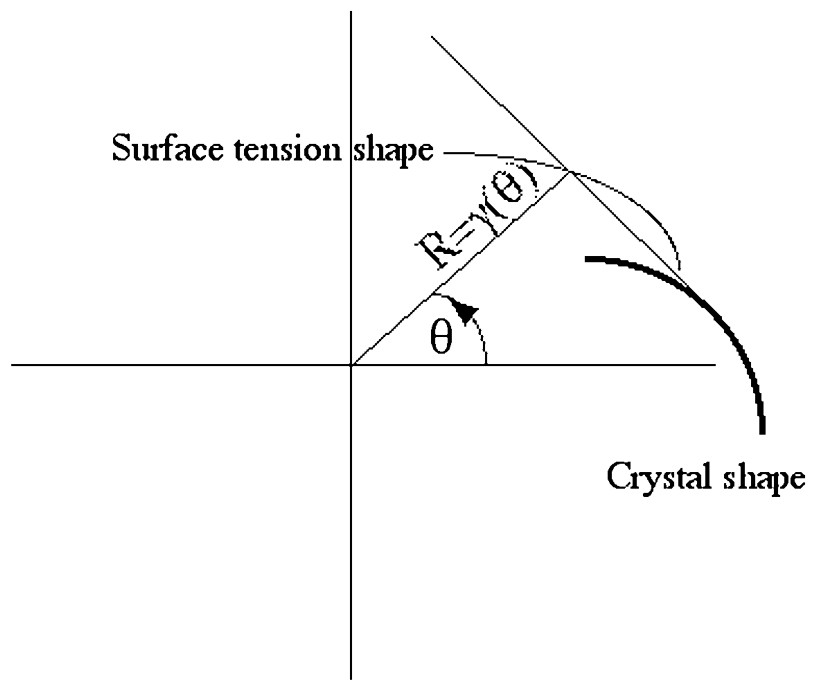
Construction of a crystal (bold line), from the shape of the surface tension in polar coordinates. The contour of the crystal is the podal of the surface tension, i.e., the envelope of the normal to the rays of length γ(θ).
One would dearly desire an equivalent construction for a ‘crystal’ made with biological tissue, in order to model, for example, the growth of a finger, a plant meristem, a radish, an avocado stone or an almond, or to use it as ingredient for a model of growth of kidney ducts or lung airways.
3 The equivalent form for a fibred bag
In the equilibrium condition of a crystal surface, the ∂2γ(θ)/∂2θ term comes from the torque exerted on a surface which would not be aligned with the crystallographic axes. The equilibrium condition of an element of surface, for a non-constant γ(θ) function, is obtained by writing the variation in free energy of an element of surface that would be rotated at the contour, by an amount dθ. This work is (Eq. (3)):
| (3) |
| (4) |
| (5) |
Then, Eq. (2) is derived by writing the static equilibrium of a little surface element under the tension force, and this additional force, which comes from resistance to rotation.
Let us now address the case of a fibred bag or shell (Fig. 2). In this first case, the fibers run azimuthally. Our question is: can the corresponding equilibrium shape be reconstructed from simple mathematics, such as the one coming from the Wulff construction? Fibers embedded in a surface may have an intrinsic curvature κ completely different from the curvature of the surface. For the azimuthal distribution of rings (Fig. 2), κ=1/r(θ)=1/Rcosθ. Suppose, now, that we look locally at a small element of the interface, close to a ring, and suppose we wish to rotate this element of interface (Fig. 3). We see that the ring exerts a torque on the surface, which is all the greater as the ring is more curved. This curvature should not be confused with the curvature of the surface itself. In the Hookean limit (linear elasticity), and neglecting the other deformations, we may simply consider the elementary torque dC as proportional to the curvature κ (Eq. (6) and Fig. 3):
| (6) |
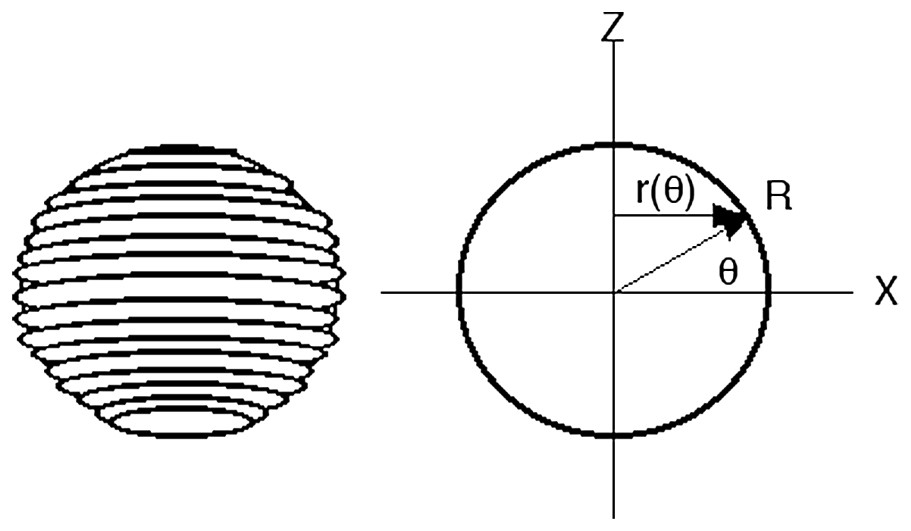
We wish to investigate the equilibrium shape of a biological vesicle, surface or membrane. The hypothesis is that a uniform material contains ring structures that run azimuthally along the surface and around a pole, or fibers that converge radially towards a pole. They are uniformly attached to the membrane (or they are the surface), and they resist against torsion of the interface. r(θ) is the radius of the ring; R(θ) is the spherical coordinate. The Laplace relationship links the surface tension to the drop of pressure, for the mechanical problem of a thin membrane with an excess of pressure inside. A similar relationship relates the surface tension and the chemical potential, whenever these quantities are actually defined, for a bulk material problem.
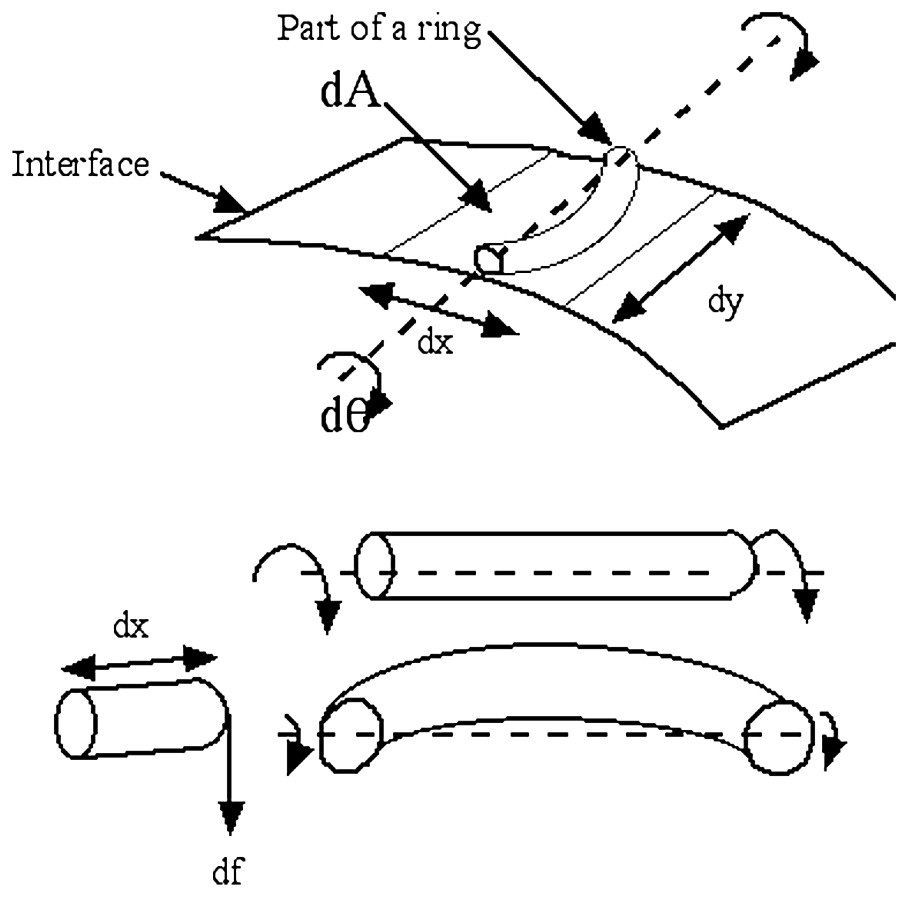
If we consider a little element of the surface, where a ring passes, we see that the torque exerted by the ring for a given rotation dθ of the membrane is higher when the ring is more curved. This induces a behavior of the surface tension such that the surface is stiffer wherever the pattern of fibers or cells forms a smaller ring, or a drawing with a smaller radius of curvature. In this image, we have represented the bundle as a rope of circular cross-section; it could as well be a ring of cuboidal cells, or a ring of fibers.
We see that this torque is bigger closer to the poles. This is very familiar: straw disks, hats or baskets are stiffer in the center than in the periphery. So, when rotating an element of surface away from the reference configuration, the fiber resists locally by , and this should overcome the other mechanical contributions in the pole region. The same term arises for bundles of meridian fibers that converge towards a pole and are arranged in rings. This torque corresponds to a variation in ‘free energy’ due to an equivalent surface tension γ such that (Eq. (7)):
| (7) |
We then get a contribution to the surface tension that satisfies:
| (8) |

The Wulff construction for our biological structure. (A) The γ(θ) curve obtained numerically γ(θ)=1+Integral(1/cosθ) (a constant of integration equal to 1 and a prefactor of 1 for the torque contribution). (B) The corresponding Wulff construction for one fourth of the plane. It can be considered as an approximation of a fingertip (see text), considering that the nail cuts the tip in equatorial plane along the horizontal axis. (C) The complete contour. There exist nail hypoplasias in which the fingerprint goes all around the tip. In such malformations, the pattern of epidermal ridges may be concentric, with a pole at the top of the finger. In these malformations [10, especially Fig. 46D] the tip of the fingers may be sharper than in normal fingers.
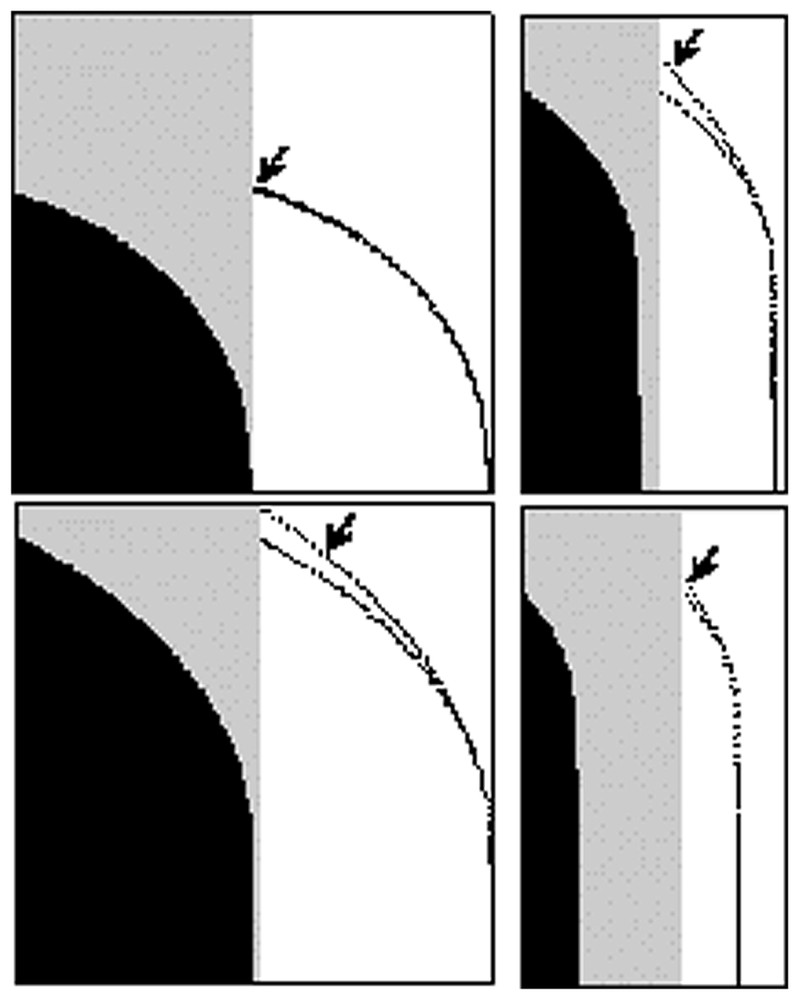
In this figure, the various contours A, B, C are obtained by a Wulff construction based on the β+Integral(α/Rcosθ) function (A: α=1, β=100; B: α=5, β=100; C: α=1, β=25). The initial Wulff construction (arrows) gives contours that actually depart from the sphere. The self-consistent construction is then made by iterating the Wulff construction (black and gray). Technically, the new contour is used as input for the calculation of γ(θ), which is used as input for the next Wulff construction. The final contour satisfies both the relation between surface tension and curvature of the fibers, and the fact that it is the equilibrium shape. The convergence to the self-consistent shape is very rapid.
Now, in the azimuthal configuration, we needed to calculate γ(θ) such that dγ/dθ=α/Rcosθ. If we consider a meridian distribution of fibers, and limit the contribution of the fibers to an increase in surface tension because of increased density of fibers, we simply have γ(θ)=β+α/Rcosθ. In this configuration, the surface tension description is exact formally, even for a macroscopic object, if we suppose that individual cells in the strands (for example, along the main fiber bundles) strive to reach the same mechanical homeostasis, as discussed above. Again, we can plot as a first approximation the Wulff construction of γ(θ) and then construct the self-consistent function r(θ) (Fig. 6). This iteration gives a profile whose radius r(θ) satisfies both the surface tension condition γ(θ)=β+α/Rcosθ and the condition ‘equilibrium profile of’ γ(θ)=β+Int(r(θ)).
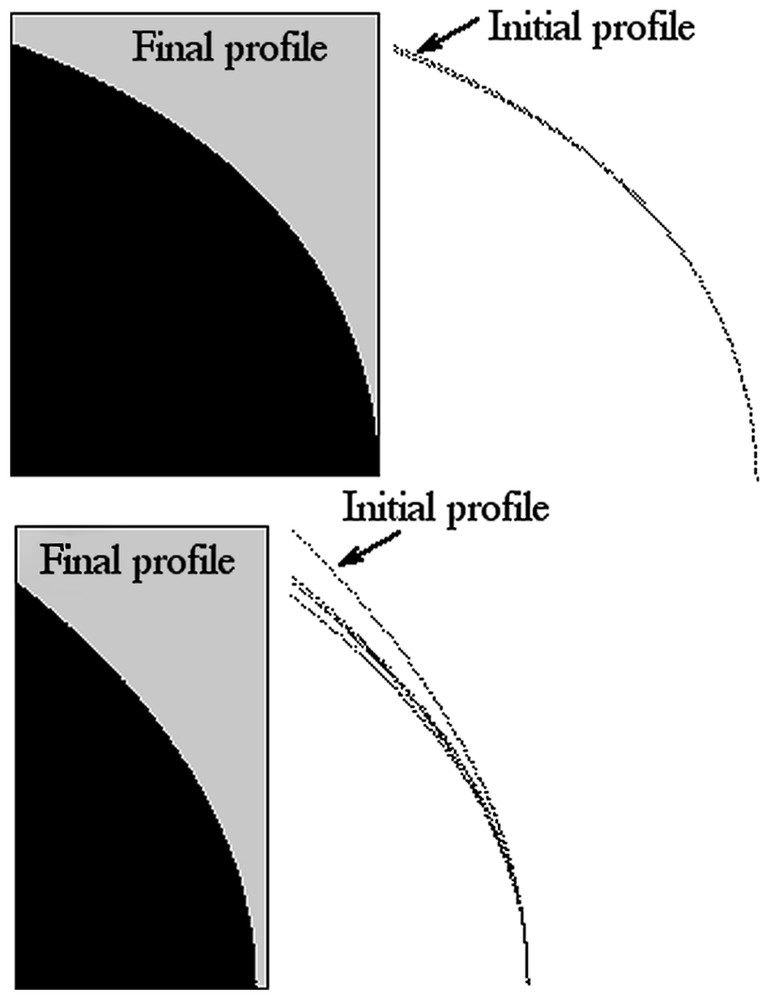
Set of shapes based on meridian fibers instead of azimuthal ones. In this case, the surface tension varies as the distance to the axes of rotation. A first construction, based on the β+α/Rcosθ function given by a circle, gives contours that, as in Fig. 5, depart from the circle (arrows). (A: α=1, β=150; B: α=90, β=15.)
4 Discussion
We acknowledge that the very simple description given above does not treat entirely the complete mechanical problem of a regular distribution of fibers on a dynamically expanding shell. There is increasing work dedicated at understanding the ‘flow’ of biological tissue during morphogenesis, in response to self-imposed stresses (especially, turgor pressure, and epidermal tension), and these studies will eventually incorporate the fiber behavior. There has been attempts, such as Paul Green's concept of reinforcement field [2], but these seem unable to treat the impact on a global shape, as addressed here; especially, they do not lift the chicken-in-the-egg problem of what drives the appearance of a polarity, oriented in the direction of the orientational singularities. In another hand, there exists interesting work describing such an orientational order in living organisms, but which does not link the orientational order to morphological features [10]. One possible alternative to the surface tension approach given here would consist in forming by iterative growth the elasto-plastic expansion of a shell, with fibers included in the surface, we are currently working on such a description. This would complement the work of Goriely and Tabor on tip growth [16], which finds a self-perpetuating profile. Please note that these authors find a self-similar profile, while in our case, the growth of an onion-like shell is not self-similar (see below).
The general problem of the dynamic evolution of a fibered material is a problem of considerable difficulty, in which the exact material properties will play an important role (more terms than just the torque or the tension in the constitutive equation). How the fibers (collagen, chitine, keratin, etc.) get their arrangement, whether the arrangement is stable, the possibility of several shells interacting in a Russian-doll fashion, all these points are absent from our simple model. However, this model enables one to derive a few very didactic and intuitive consequences. A considerable amount of biological structures are constructed with cells, fibers or linear folds arranged in rings or curves. When forming a spheroidal structure, these shapes are very rarely actually spherical, if not never. They most generally have a bump or a cusp towards the center of a pole where the fibers converge, or the rings concentrate like a target. This is quite obvious of a large class of vegetables and fruits, such as turnips, carrots, radishes, egg-plant, avocado stones, almonds, chestnut, lemon, prunes, etc., it is also apparent on root caps, meristems, buds and pins. At first glance, it appears obvious that the sphere is not a generic shape; it is also observed that the sharper forms are generally stiffer. There exist also many examples in animals, such as the abdomen of insects, muscles or teeth roots, the chondrogenic condensations and possibly horns and many similar defenses. Apart from pin-like structures, there exist more subtle configurations that can, astonishingly, be related to this problem. In humans, it is the case of the fingertip, and its epidermal ridges. This example is even more interesting, because the pattern of lines is neither concentric, nor radial. It is well known that fingerprints are not genetically coded [17–19]. The keratinocytes and other cell types form spontaneously a liquid-crystal nematoid order of crevasses and folds. But mathematics tells that covering a tubular surface with such a nematoid order leaves unavoidable orientational defects. The fingerprints are the solutions found by nature to cover a ‘tube end’ with ridges: they are more complex than the azimuthal solution with one defect at the pole. There are one or several defects left in the center of the finger, known as the core in forensic science [18, (chap. 1)]. This general structure is observed in more than 95% of the human fingers and also at fingers and tails of monkeys and koalas [20]. It has long been known that there is a correlation between the pattern of fingerprints and the finger shape [17–19]. Especially, there exists a bump, sometimes almost a cusp, in the finger, in the core region. The profile in Fig. 4B is close to the profile of fingers, and it would approximate the equilibrium shape of epidermal ridges having quite concentric whorls. However, loops (60% of fingers [18,19]) are more frequent than whorls (30%). For the sake of simplicity, we simplify the loop, symmetrize completely the pattern of streamlines observed at such fingertips, and get the sphere shown in Fig. 7A, which we call the fingerprint crystal. It is obviously not like the concentric rings of Fig. 2. Still, we can draw intuitively the equilibrium shape that would be generated by such a distribution of ridges, by considering two halves of the fibred vesicle rotated by 90°, and we get the image in Fig. 7B, which gives qualitatively the shape of the fingerprint crystal: a round tip that departs from a sphere in the region of the core, where there is a cusp. A more accurate treatment of this example will be presented in a forthcoming publication [15].
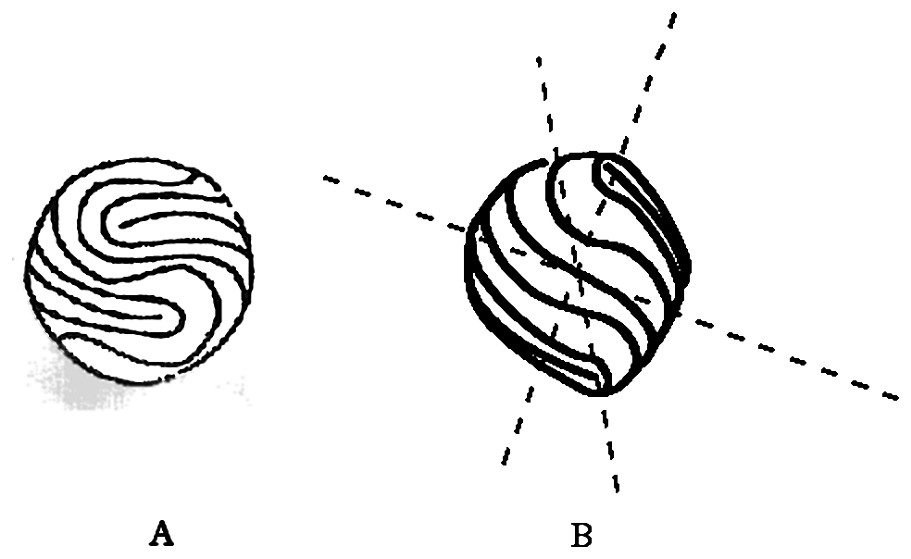
Epidermal ridges form a pattern of fibers that is not made of concentric rings. 60% of fingers have a loop, and only 30% have concentric rings in the so-called ‘core’ of the epidermal ridges. The loop pattern can be symmetrized across the plane of the nail, and across the center of the core, to give the equivalent fibred vesicle. Pattern (A) is reminiscent of the way tennis balls are sewed. The theoretical calculation of this shape is much more complex than that of the simple concentric pattern of Fig. 2. However, a simple intuitive approximation consists in forming the profile that accommodates two halves of the contour drawn in Fig. 4C, but rotated at 90° in the perpendicular plane of the drawing. This gives the shape in (B), which is the closest 3D approximation of a fingertip that our model can produce. To make the construction more intuitive, one should observe that the cusp amounts to a displacement of the pole in the direction perpendicular to the surface of the sphere. In order to get the image in (B), one should concentrate on the position of the four poles in (A), and then consider that they are gently displaced perpendicularly to the surface of the sphere. One on the top (the symmetrical pole in the bottom) is displaced vertically, another one on the side is displaced towards the reader (the symmetrical one is displaced in the background). The model then predicts the existence of a bump or cusp in the core of fingers, as actually observed. As soon as there is a difference between the transverse and longitudinal directions, in such a way that the fibers are stiffer in the longitudinal direction, the equilibrium shape departs from a sphere. Then, this behavior constitutes an effective anisotropy, but of orientational origin, and not of crystalline origin. A tube covered with such a distribution of fibers will branch in a regular order, akin to genuine dendrites.
We then conclude that the fibrous nature of the tissue is one important ingredient in the morphogenesis of most biological structures. The spindle-like shape of most spheroidal biological structures is easily accounted for by the presence of fibers. The other ingredient should naturally be the actual distribution of internal and external forces (r.h.s. in Eq. (2)), gravity, etc. Especially, if the kinetics of cell division is modified by the stiffness of the tissue (the individual tension is not constant) then differential growth may occur between shells. The logic is that if the kinetics of the shells is the same, then there should exist a cusp at poles, but if the kinetics of shell growth is different, then the most rapidly growing shells will be deformed by the other ones. This may be a natural explanation for inverted cusps at poles: internal spindles being stiffer may sometimes grow more slowly than outer shells, which are softer.
Our simple hypothesis (Eq. (7)) lets us predict qualitatively the presence of a bump or cusp at poles of spheroidal, but fibred, biological structures, including the approximate shape of the last phalanx of fingers (in this case, the ‘pole’ is not the apex, but the center of the phalanx, on the fingerprint side). As the fibers get stiffer, the arrangement of rings gets sharper, as well known in plants (for example in pins, or in fruit stones, which have generally a sharper cusp than the fruit around, e.g., lemon, almonds), and also in insects that have a dart that is both sharper and stiffer than the abdomen. This applies also to the structure of fingers, whose bones (especially the last phalanx) are both much sharper at the tip and stiffer than the tissue around. The link between stiffness and sharpness gives a straightforward construction toolbox for organs that need to be both hard and sharp, in the context of ‘survival of the fittest’ (teeth, horns, pins, and all sort of natural defenses). It also gives a construction rule for soft tissue that needs to be held inside by a stronger skeletal structure (a mere change in fiber density induces the transition from a sharp and strong inside to a soft and round envelope, therefore, the same genetic program, in which only a material switch is modified leads to tissues with different properties and form).
In terms of morphogenesis models, the examples treated here show that the orientational order of the tissue, which is a vector, should be incorporated into the growth models. So far, most morphogenesis models, such as Turing models [21], neglect this material aspect of the tissue. For example, all models of chondrogenic condensations neglect the orientational order of fibroblasts, although the in vivo images clearly show that such an order exists [22]. Segmentation of condensations respects the orientation of the segments, as also observed in plants. This is of upmost importance, since chondroblasts will eventually form the bones, and since the anisotropy of the tissue on each bone is necessary to properly position muscle ligaments and tendons around the joints. In plants, the transition between lenticular and round leaves of arabidopsis has been ascribed to the gene spike1, this gene induces a change in shape of individual cells, from jigsaw puzzle shape to shoebox like [22]. Our models may provide the missing link between such a change at the individual cell level, and the global change of the leaf shape.
One important prediction is that the exact shape depends on the size, even if the topology is identical. This point deserves an explanation. It is a fact in materials science that the equilibrium shape of crystals is independent of their size. This is because the total size appears in Eq. (2) as a prefactor (absorbed in R, in the equation). The Wulff construction gives a homothetic shape, depending on the total amount of matter contained in the crystal. It can even be argued that this fact is at the origin of the entire science of crystals itself. Indeed, the Abbé Haüy discovered the arrangement of molécules intégrantes inside a crystal, by observing that all calcite crystals had the same shape, independently of their size. He then extrapolated the observation towards the microscopic structure of the calcite, and concluded that it had to be made of a repetition of very small identical elements [23]. In brief, if one keeps adding the same atoms to a crystal surface, it expands homothetically. This is not the case with a fibered structure. This comes from the fact that the curvature of the fibred arrangement comes into play in the energetics of the surface. As a consequence, an azimuthal or meridian arrangement of fibers is stiffer when it is smaller. So, the very same distribution (same topology) of fibers becomes softer, as the structure becomes bigger. This may be the reason why many vegetables become rounder as their size increases. The very same bauplan does not give exactly the same shape at given scales, because of the change in materials' properties.
Not all fibred structures have a simple profile, like turnips or fingertips, though. Branched structures also are fibred. This is true of plants, of course, but also of branched organs. Let us observe that the stream of lines (rings or epidermal ridges) generate a symmetry breaking. In the oriented shell shape (Figs. 2, 4–6) there is one preferred direction: the pole. In the fingerprint shape, as described in Fig. 7, there are several axis: the direction in which the crystal points (equivalent to the finger direction), the direction of the stream of lines at the top (would be the direction of the edge of the nail), and the axis of the cusps. These three axes, as observed on 100% of actual fingers, except in an extremely rare autosomal trait (absence of fingerprints [18,19], only two families known), are a simple consequence of the materials properties and of the nematic order of the ridges. So, the existence of a symmetry breaking, forming three axes pointing out at right angles is latent in the shape of the lines (Fig. 7A), and it is revealed by the mechanical properties. This constitutes an anisotropy of the surface tension. Hence, on physical grounds, we expect out-of-equilibrium growth of fibrous interfaces to generate stable tips, as in dendritic growth, and regularly branching trees akin to the genuine dendrites, except that the anisotropy is not a crystalline anisotropy, but an orientationally induced one. For the situation in Fig. 4, the growth shape will be a sharp tip or dome as generally observed in roots, vegetables, pins, darts and similar organs, because there are no secondary directions of growth (two-fold anisotropy). This is also the mode of growth of abnormal fingers that have no nails and a concentric pattern of ridges; Cummins [17] has described several of such malformations, which give rather abnormally sharp extra-digits. For the situation depicted in Fig. 7, we expect a branching structure for out-of-equilibrium growth, because the surface exhibits several directions of growth. Let us recall that, in the context of crystal growth, an anisotropy of 1% is enough to shift the pattern from randomly dichotomous, to regular side branching. Such a regular order of branching is commonplace, especially in the kidney [24] and the lung [25,26], and it exhibits typical “zebra-shoulder” fibred structures at branching points in the form of cartilage rings [27]. Branching fingers with identical distributions of epidermal ridges also exist in not infrequent hand malformations [17].
Another important issue is linked to the fact that cells, like, for example, fibroblasts, tend to form layered tissues in which the cells are arranged at right angles, from one layer to the next. This implies, for our simple case, that both meridian and azimuthal fibers should be considered, in such a way that two terms of the kind shown above should be incorporated.
5 Conclusion
In conclusion we should say that, following the work of Alan Turing [21], a lot of effort has been devoted to finding reaction–diffusion models of growth in biology. Truly, there is very little, if any, evidence of Turing instabilities in the pattern formation of biological tissue. To our knowledge, the only convincing biological model studied with some precision concerns the dasycladean algae [28]. The animal coating patterns are beautiful but still speculative [29], and the reaction–diffusion models of feather condensations rely heavily on mechanical properties, they boil down to reaction–diffusion only through the similarity of the mathematical formalism. There exist a number of molecular pathways consisting of inhibitors and activators, but it is hard to tell whether these molecules actually diffuse, or are created by loops containing contact inhibition, and mechanical intermediaries.
Whatsoever, following the work presented here, the material properties are an essential part of the morphogenesis. The morphogenesis of fibred interfaces can be treated as a moving boundary problem, with a contribution from the curvature of the fibers' pattern. The stiffness increases towards the singularities (poles), either because the curvature of the rings increases, or because the density increases in proportion to the curvature. A cascade of simple predictions seems to spring immediately. The main predictions are the existence of cusps at poles of the contour, as indeed observed quite generally, and a dependence of shape with size. Let us insist that such cusps are mathematical singularities that are straightforward with the Wulff construction (when it applies), while they are impossible to generate by reaction–diffusion models playing with diffusion or deformation fields, because of the underlying formalism of Partial Differential Equations. The two parameters α and β defined above set the dimension and sharpness of the contour. The genetic control of shifts from round to sharp features is easy to imagine, by adjustment of the physical parameters of the fibers.
As a critical end, we should observe that there exist a number of spheroid structures that are almost spherical (eyes or a number of plants, also microorganisms), structures that exhibit meridian furrows (like garlic), or almost facetted sides (starfruit). Also, most natural ‘spindles’, like fruit stones, are not symmetrical: they exhibit a difference between a top and a bottom, which our theory does not find. It is clear that, in addressing only the equilibrium shape, our model is too simple. Differential forces (a non-constant term in the r.h.s. of Eq. (1)), the presence of gravity, of an epidermis, of attachment to a trunk, the segmentation into quarters, etc., should also play a role in the final shape. The gradient of pressure inside the organ might be the natural explanation of the bottom/top asymmetry. However, we clearly identify, in all these shapes, the presence of fibers or cells in specific arrangements that should be incorporated into the models prior to or at least together with any distribution of ‘morphogens’.


