1 Introduction
Iron(II) complexes displaying spin-crossover (SCO) between the 1A1 low-spin (LS) and the 5T2 high-spin (HS) states have been the subject of much work during the last decades [1–4]. Beside fundamental research, there have been several proposals for the design of information processing devices (e.g., memories, displays, etc.) based on SCO compounds. For example, the discovery of the LIESST (light induced excited state spin trapping) effect suggested that the SCO complexes could be used as optical switches [5, 6]. However, the LIESST phenomenon has been observed only at very low temperatures, typically below 80 K.
Another interesting approach to build memory devices from SCO materials is based on the fact that the thermal spin transition may occur with hysteresis loop, conferring a memory effect to these systems [7, 8]. Indeed, inside the temperature range of the hysteresis loop, the spin state of the system depends on its history, hence on the information that has been stored. Since the optical and magnetic properties of the material are markedly different in the two spin states, this information can be read easily by optical or magnetic means. Of course, the ideal situation is when the hysteresis cycle is centred on room temperature (RT). The first spin-crossover complex with large and abrupt thermal hysteresis loop around RT was reported in 1993 in conjunction with optical reading and thermal addressing of the stored information [9]. This report has been followed by an intense research for compounds with RT hysteresis, and today several examples are known [10]. Besides thermal addressing, switching of spin-states by short magnetic [11–15] and light pulses [16, 17] in the thermal hysteresis interval has also been investigated recently. However, reports on different possibilities for addressing these systems in the hysteresis loop are still rather scarce and the kinetic aspects (transition rates, macroscopic barrier heights, etc.), which have a foremost importance in the writing and erasing processes, are yet little characterized.
Concerning the magnetic field effects on the compound Fe(phen)2(NCS)2, the main findings can be summarized as follows [11]. Following a 1-s pulsed magnetic field of 32 T, sizeable changes are observed in the HS fraction. In the temperature range of the hysteresis loop, an increase in nHS is obtained, with an irreversible (reversible) character in the ascending (descending) branch of the loop (Fig. 1). A quasi-static magnetic field of 32 T theoretically corresponds to a variation of the spin-transition temperature (T1/2) – defined as the temperature for which nHS =
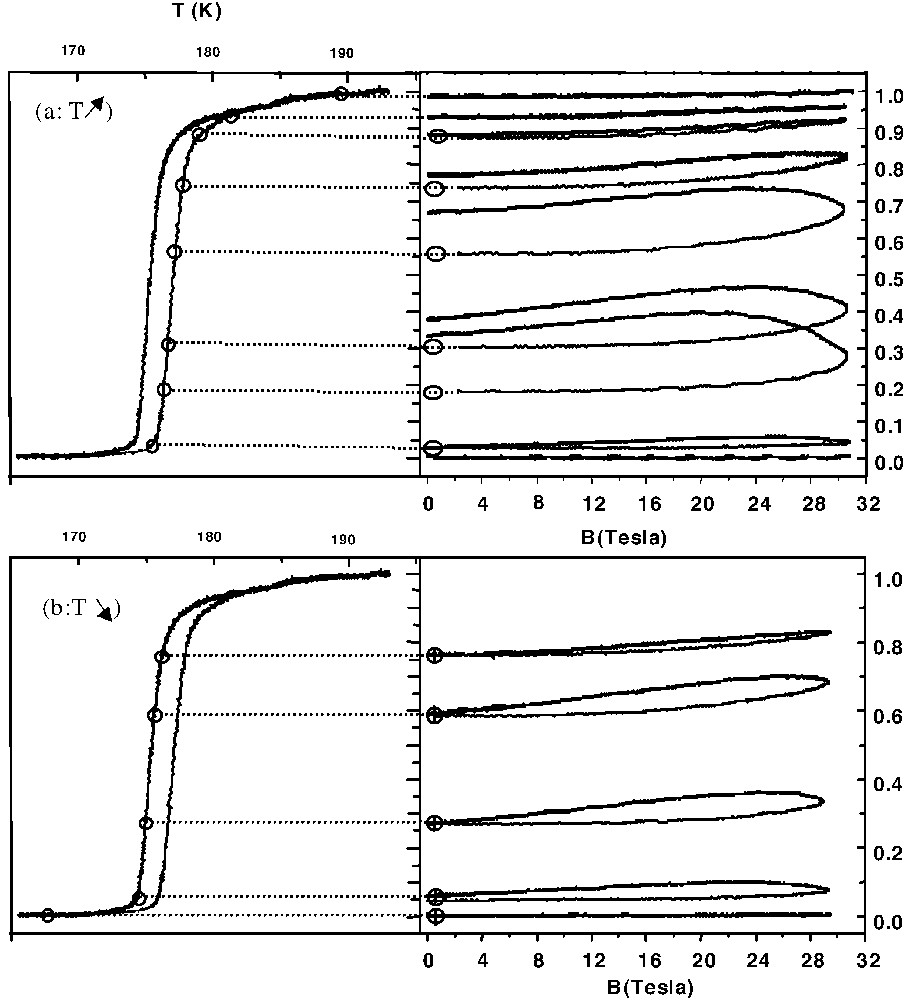
Effect of a pulsed magnetic field on the high-spin fraction of Fe(phen)2(NCS)2 in the ascending (a) and descending (b) branches of the thermal hysteresis loop (after [11]).
In this paper, we report on the effects of a pressure pulse in the thermal hysteresis loop of Fe(phen)2(NCS)2. It is well known that an external pressure stabilizes the state with smaller volume (LS state) in contrast to magnetic field, which stabilizes the state with higher spin (HS state). For relatively small applied pressures (up to 1000 bar) the work term, p ΔV, to the Gibbs’ free energy is already significant because of the large difference in volume (ΔVHL = VHS – VLS) between the HS and LS forms [2,4]. Indeed, the spin equilibrium as well as the HS to LS relaxation in SCO complexes was generally found to be very sensitive to external pressure. For example, T1/2 was found to shift by as much as 20–30 K/Kbar in several SCO compounds [18–20].
The compound Fe(phen)2(NCS)2, which we have selected for this study, has been extensively investigated under quasi-static pressure [21–27]. Under atmospheric pressure, it undergoes an abrupt spin-crossover around 176 K, with a hysteresis of ~1.3 K [11]. At room temperature, under hydrostatic conditions, the HS form can be converted into the LS form by application of high pressure. No change in space group has been observed during this transformation [26]. Concerning the transition pressure (p1/2), there are some discrepancies in the literature due to the polymorphism of this compound and also because of possible non-hydrostatic conditions. According to the thorough analysis of Roux et al. [27], polymorphs I and II exhibit SCO around 8 and 6.5 kbar, respectively. There are also different observations concerning the completeness of the transition under pressure, but under truly hydrostatic conditions it appears to be quasi-complete (< 10 % HS residue). It should also be noted that subsequent pressure cycles up to 15 kbar led to the irreversible alteration of the transition curve [27], while pressurization up to 10 kbar had no apparent effect on the spin transition curves [25]. The only pressure experiments conducted under cryogenic temperatures on this compound were reported by Usha et al. [25]. From the analysis of their magnetic susceptibility isobars they have obtained a quasi-linear shift of T1/2 as a function of the applied pressure, the shift being 22 (± 1) K per kilobar.
2 Experimental
The sample of Fe(phen)2(NCS)2 (polymorph I) has been prepared as described in [28]. The variable temperature, hydrostatic pressure system (He gas) and the optical reflectivity (550 ± 50 nm bandpass filter) detection device have been described in [29]. The ratio IR/I0 (reflected light intensity/incident light intensity) was monitored continuously, while pressure and temperature were governed independently. The stability of temperature was better than ± 0.1 K, while the pressure could be stabilized within ± 5 bar. According to previous studies [11], the IR/I0 ratio follows quite linearly the variation of nHS. Corrections were, however, necessary because small pressure effects on IR/I0 are present even in the absence of any spin state change. These effects are mainly due to change of optical properties of the pressure cell, and, to a lesser extent, to the shift (in intensity as well as in wavelength) of the optical absorption bands under pressure. Correction factors were obtained by recording LS and HS absorption spectra (300–1000 nm) at different pressures in the two spin states (Fig. 2).
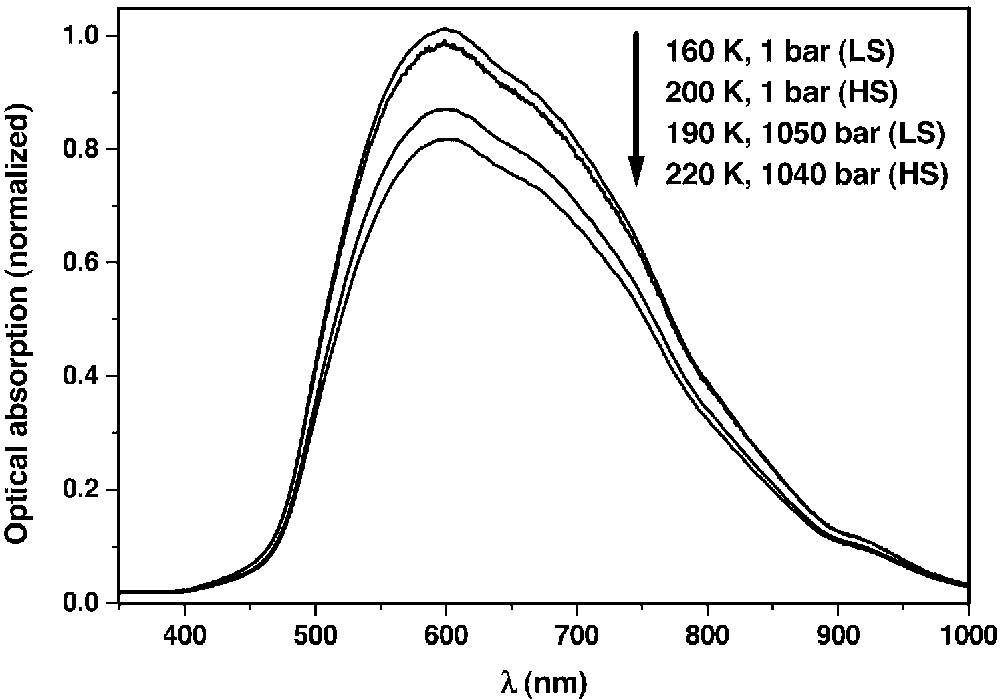
Optical absorption spectra of Fe(phen)2(NCS)2 at some selected temperatures and pressures illustrating the importance of correction factors applied on reflectivity data.
It is worth noting that reflectivity enables the investigation of the sample ‘surface’, hence the reflectivity data do not necessarily match those obtained by ‘bulk methods’ such as magnetic measurements [30]. Finally, one should also be aware that the thermocouple is placed relatively far from the sample (outside the pressure cell), leading to a small difference between the real and the displayed temperature (~3 K). This feature does not affect, however, the precision (i.e. the resettability) of temperature.
3 Results and discussion
Spin transition curves of Fe(phen)2(NCS)2 have been recorded at 1, 200 and 1030 bar as a function of T. As an example, Fig. 3 shows two successive temperature loops at 200 kbar, revealing a good reproducibility of the measurements. Alternatively, a pressure loop has also been recorded in isothermal conditions at 195 K (Fig. 4). Based on these measurements, the p–T phase diagram of the spin states could be established (Fig. 5). A linear fit leads to the variation dT/dp = 23 (± 0.4) K kbar–1, in excellent accord with the results of Usha et al. [25]. The slope is somewhat steeper for the line T1/2↓ compared to T1/2↑, in agreement with the mean-field theory [19], but the difference (0.8 K kbar–1) remains close to the experimental precision. Fig. 5 suggests also a tight relationship between pressure and temperature loops. Indeed, following the simple two-level analysis of [20], we could derive the shape of the isothermal loop from that of the isobaric loops; the computed shape is in good agreement with the experimental one.
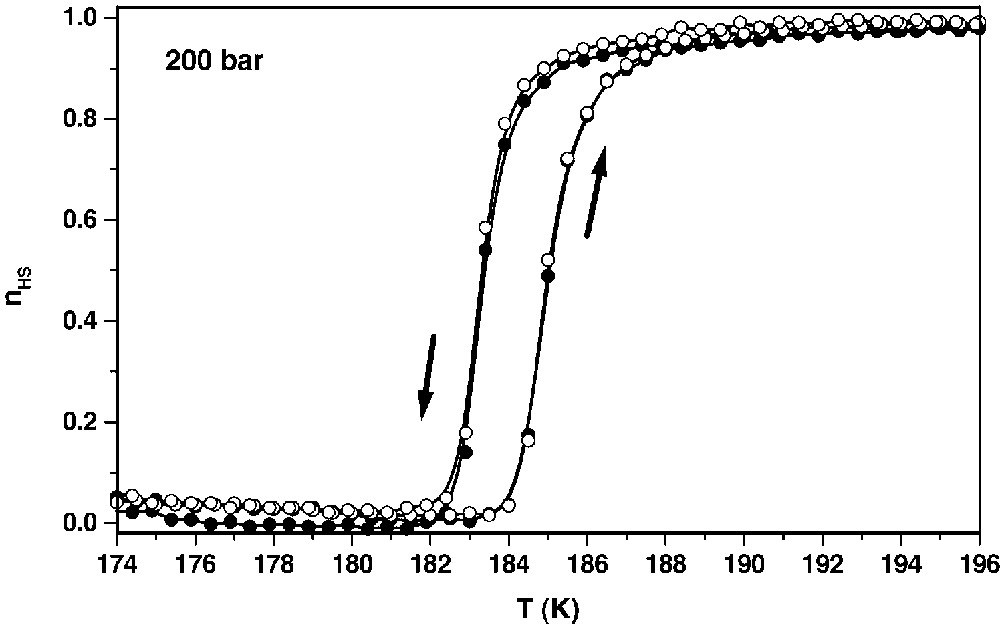
Successive thermal hysteresis loops of Fe(phen)2(NCS)2 at 200 bar (lines are inserted to guide the eye).
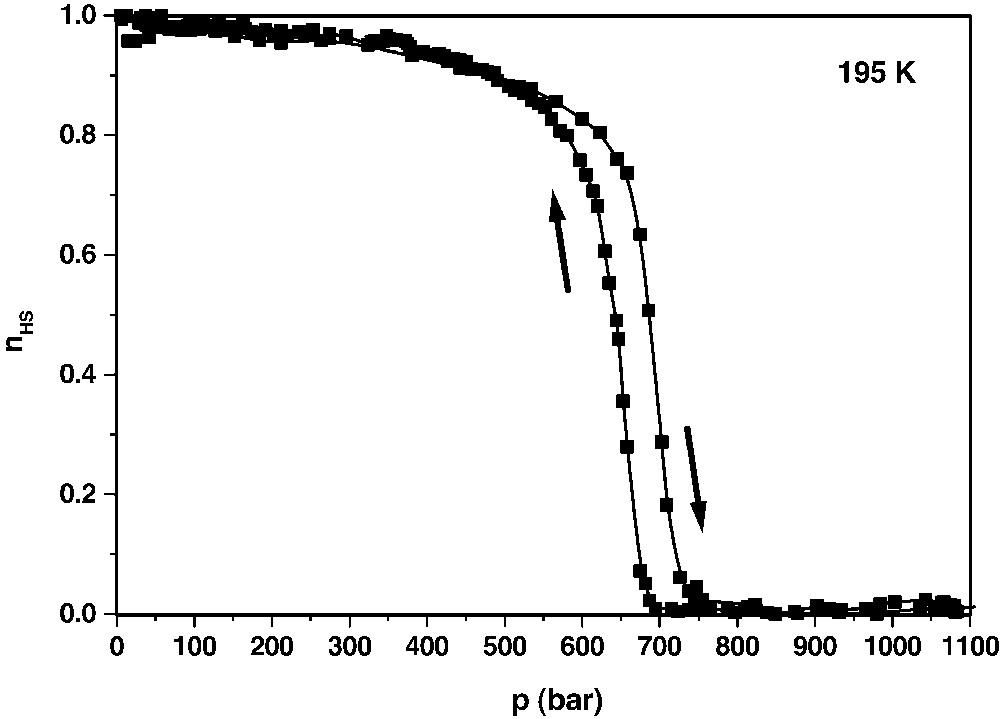
Pressure hysteresis loop of Fe(phen)2(NCS)2 at 195 K (lines are inserted to guide the eye).
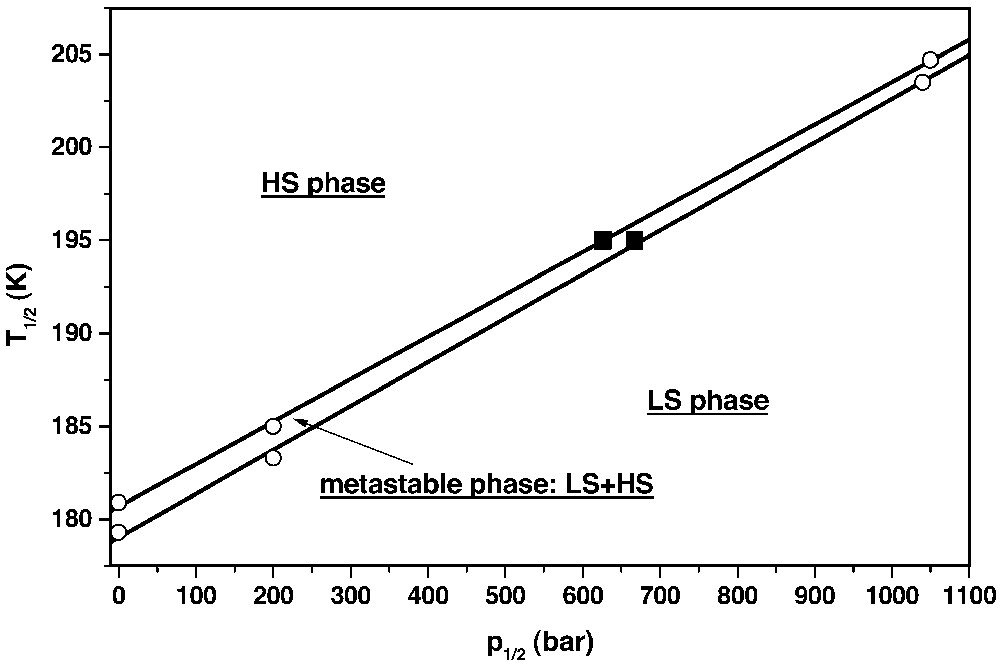
The (p,T) phase diagram of Fe(phen)2(NCS)2 from isobaric thermal hysteresis loops (○) and from isothermal piezo hysteresis loops (■). The straight lines represent linear fit on the data.
As explained in [20], the pressure dependence of the equilibrium temperature is expected to be linear:
| 1 |
Then, from the experimental ΔSHL value of 49 J K–1 mol–1 [31] – neglecting the small pressure dependence of the ratio ΔVHL/ΔSHL –, one can obtain a volume variation (ΔVHL) of ~19 Å3 per molecule, in excellent agreement with the value of 18 Å3 per molecule calculated from variable-temperature X-ray diffraction data (at ambient pressure) [26].
We also carried out a series of pulsed pressure measurements. The pressure pulses (up to 300 bar) were applied at different temperatures in the hysteresis loop. Before each measurement in the ascending (descending) branch of the hysteresis loop, the sample was reset at 150 K (200 K). After setting the desired temperature, 10 min were allowed for the sample to reach complete thermal stability, and then the pressure pulse was applied. The maximum pressure is reached in ~5 min and then decreased to 1 bar in ~5 min, but it should be noted that, in the present state of the device, the pressure is controlled manually, thus the shape of the pulse is not completely reproducible. Fig. 6 shows a typical pulsed pressure experiment (50 bar, 180.3 K) in the descending branch of the thermal spin-transition curve. It can be seen that the nHS fraction decreases with increasing pressure, but when releasing the pressure the system does not return completely to its initial state. The complicated variation of the nHS fraction upon decompression is of course related to the shape of the pressure pulse. We shall see later that the experiment contains a kinetic aspect, which also should be reflected in the shape of the response of the system.
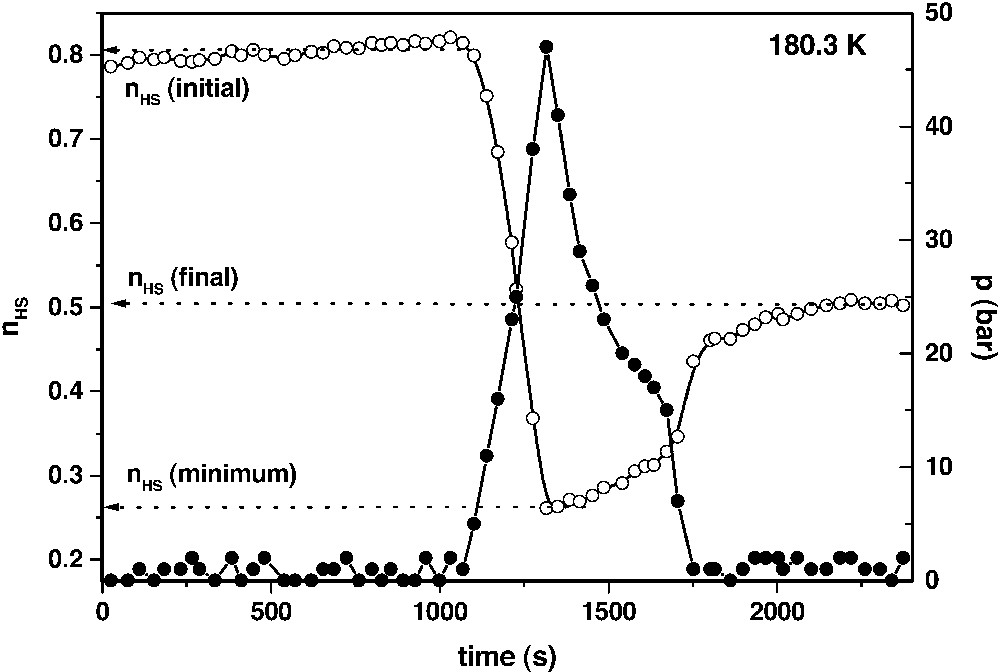
A typical pulsed pressure experiment in the descending branch of the thermal hysteresis loop showing the time dependence of the pressure pulse (•) and the nHS fraction (○) (lines are inserted to guide the eye).
In Fig. 7, the effect of a 50 bar pressure pulse is compared for the ascending and descending branches of the hysteresis loop. These figures show that a sizeable triggering of the spin transition is obtained for both branches. However, in the descending branch, the irreversible decrease of nHS is evidenced by the observation over a rather long time (10–15 min), while the drop of nHS appeared to be completely reversible in the ascending branch.
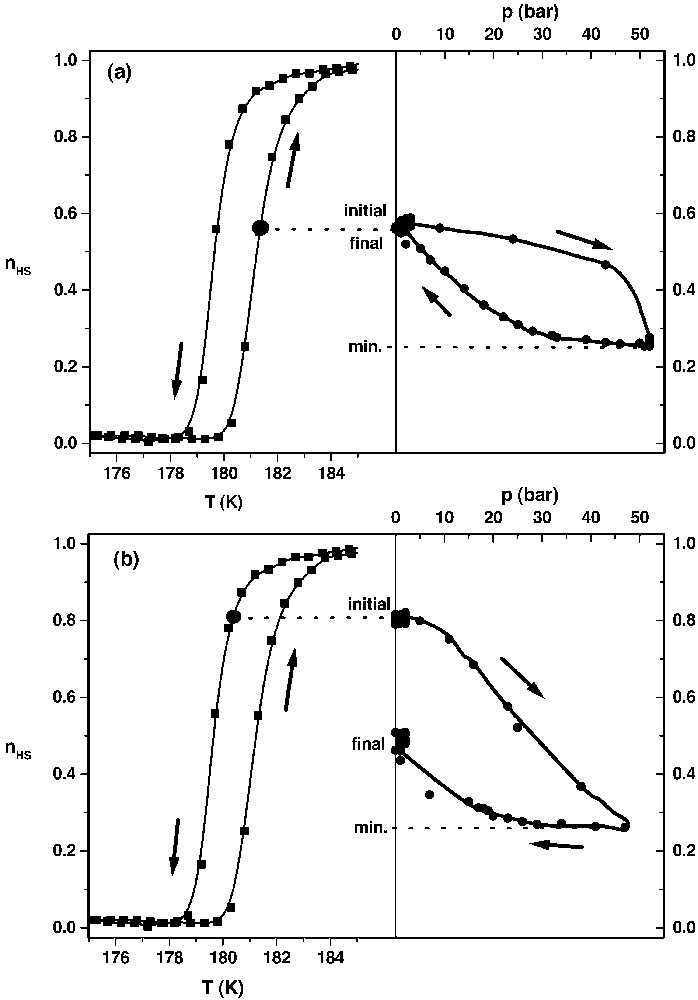
Pressure effect on the high spin fraction in Fe(phen)2(NCS)2 in the ascending (a) and descending (b) branches of the thermal hysteresis loop (lines are inserted to guide the eye).
We now discuss the amplitudes of spin conversion. The data obtained for different pressures and initial nHS values in the ascending and descending branch of the hysteresis loop are listed in Table 1. Due to the somewhat arbitrary form of the pressure pulses, only a qualitative comparison can be made. It appears, however, clearly that the variation of nHS is always reversible (irreversible) in the ascending (descending) branch and these variations (both the reversible and irreversible ones) are proportional to pressure.
Experimental values of the amplitudes of spin conversion for different pressures and initial nHS values in the ascending and descending branches of the hysteresis loop.
| nHS initial | nHSminimum | nHS final | Δp (bar) | |
| T↑ | 0.4 | 0 | 0.4 | 50 |
| 0.55 | 0.1 | 0.55 | 50 | |
| 0.6 | 0.25 | 0.6 | 50 | |
| 0.7 | 0.4 | 0.7 | 50 | |
| 1 | 0.6 | 1 | 50 | |
| 0.75 | 0.15 | 0.75 | 100 | |
| 0.75 | 0.10 | 0.75 | 220 | |
| 0.75 | 0 | 0.75 | 300 | |
| T↓ | 0.2 | 0.1 | 0.15 | 50 |
| 0.80 | 0.25 | 0.5 | 50 | |
| 0.75 | 0.1 | 0.4 | 110 | |
| 0.75 | 0.05 | 0.05 | 220 |
In a simplified approach [20], one can consider the effect of the pressure pulse to be equivalent to a temperature-induced effect [11]. Actually, this equivalence merely correlates the partial derivatives ∂/∂p, ∂/∂T of the function nHS(T,p), as suggested by the present experimental data. It is interesting thus to note that – compared to the temperature shifts that can be expected from the dT(p)/dp slope of the phase diagram – the experimentally observed response of the system is attenuated. For example, 50 bar correspond (theoretically) to a shift of 1.15 K in the transition temperature, thus it should trigger a quasi-complete and irreversible spin conversion for any initial nHS value in the descending branch of the hysteresis loop. Contrary to this expectation, only a partial triggering was achieved even with a 110-bar pulse (Table 1). Such a discrepancy between (quasi-)static theory and dynamic experiments has already been evidenced in pulsed magnetic field measurements and was related to the kinetic aspects of the process [11,14]. As explained in [15], a relatively slow relaxation may account for the attenuation of the response and also its time delay.
4 Conclusions
The present pulsed pressure experiments revealed properties symmetrical of our earlier pulsed magnetic field experiments: The pressure and magnetic field induce ‘mirror’ effects. While an applied magnetic field stabilizes the HS state via the Zeeman effect, external pressure stabilizes the LS state due to the work term. Therefore, a pressure (magnetic) pulse results in an irreversible decrease (increase) of nHS in the descending (ascending) branch of the thermal hysteresis cycle, whereas these changes are always reversible in the ascending (descending) branch (Figs. 1 and 7). If one compares the theoretical shift of the transition temperature under quasi-static external pressure and magnetic field in the case of Fe(phen)2(NCS)2, it appears that the effect of 1 T is roughly the opposite of the shift caused by 2.5 bar!
The fact that such small pressure as 200 bar triggered a quasi-complete crossover between the two spin-states suggests that piezo effects might be implemented in devices for addressing SCO materials. Further experiments are in progress to better control and understand the dynamical aspects of the pressure induced spin-state switch. A possible issue will be the comparison to the pressure minor hysteresis loops [32], which can be obtained in quasi-static regime.
Acknowledgements
The authors are indebted to Dr M.-L. Boillot (University Paris sud, Orsay, France) for preparation of the sample and to A. Wack (LMOV) for technical support. This work was financially supported by the CNRS and the European Community (TMR–TOSS ERB–FMRX–CT98–0199). GM acknowledges the French CNRS for a ‘poste-rouge’ fellowship and NM the ‘Ministerio de Education y Cultura’ (Spain) for a postdoctoral fellowship (FIP98).



Vous devez vous connecter pour continuer.
S'authentifier