1 Introduction
Photoinduced electron-transfer reactions in donor (D)-C60 linked systems have been extensively investigated [1]. Though there is a number of papers for photoinduced electron-transfer reactions in D-C60 linked compounds, most of them focused on intramolecular electron-transfer reactions of singlet excited state of C60 (1C60*) [2] in spite of the very high quantum yield of the triplet excited state of C60 (3C60*).
Magnetic-field effects (MFEs) on the reaction kinetics or yields of photochemical reactions in the condensed phase have been studied [3–6]. Previously, we have found that the decay rate of photogenerated biradicals in D-acceptor linked systems was remarkably reduced in the presence of magnetic fields up to 1 T [7–13]. In addition, the reverse phenomena in the MFEs, i.e. that the decay rate of photogenerated biradical steeply decreases in the lower region of magnetic fields and then increases in the higher region of magnetic fields, have been reported. The reverse phenomena have been observed only in high magnetic fields above 1 T [14–20].
Recently, we have reported the photoinduced electron-transfer reactions and the MFEs on the decay rates of biradical in D-C60 linked compounds with long methylene groups using zinc-tetraphenylporphyrin (ZnP) or phenothiazine (Ph) as D (ZnP(8)C60 or Ph(n)C60) [9–12]. In both systems, the reverse phenomena in the MFEs have been observed for the first time below 1 T. In the ZnP–C60 system, the decay rate of the biradical dramatically decreased in lower magnetic fields (0–0.1 T) and gradually increased and finally became almost constant in higher magnetic fields (0.1–1.2 T) [12]. In the Ph–C60 systems with methylene groups, similar phenomena in the MFEs at 288 K were observed, but smaller than that of the ZnP–C60 system [10,11]. These unique phenomena may be explained by the contribution of the anisotropic Zeeman interaction to the spin–lattice relaxation (SLR) of the biradical.
Temperature dependences on the lifetimes of triplet biradicals in the presence of 0.6 T have been examined for xanthone–xanthene and benzophenone–diphenylamine bifuntional chain molecules by Tanimoto et al. [21]. These results revealed that the lifetimes of the triplet biradicals were controlled by the spin-relaxation process. In the meantime, the temperature dependences for the triplet biradicals derived from 2-phenylcycloalkanones have been reported by Turro et al. [22]. In that case, the results indicated that the rate-determining step for biradical decay changed from intersystem crossing (ISC) process to chain dynamics process on going from room temperature to lower temperature. The temperature dependence on the lifetime of triplet biradical in xanthone–xanthene bifuntional chain molecules has also been reported by Tanimoto et al. [23]. The results indicated that the unusual temperature dependence on the lifetime of triplet biradical was ascribed to the difference in the temperature dependence on the chain motion and the recombination reaction. Therefore, the measurement of temperature dependence on the MFEs and of the biradical decay is very useful for verifying the mechanism of the MFEs and the decay dynamics of the biradicals. However, temperature dependence on the MFEs in Ph(n)C60 or ZnP(8)C60 has not been reported yet.
In the present paper, we have examined the temperature dependence on the dynamics of the biradical of a Ph–C60 linked compound with six methylene group, Ph(6)C60 in benzonitrile, in the absence and presence of magnetic field (up to 1 T) and we found the novel MFEs on the decay profiles. The results revealed that the novel MFEs were attributed to the contribution of the anisotropic Zeeman interaction to the SLR of the biradical.
2 Experimental
A phenothiazine-C60 linked compound with flexible six methylene group (Ph(6)C60) and a reference C60 compound (C60ref) as shown in Scheme 1 were synthesised according to the previous papers [9,10]. Their structure and purity were confirmed by 1H-NMR and MALDI-TOF MS spectra, and elemental analysis. 10-Methyl phenothiazine (MePh) (Wako, extra Pure) as another reference compound was purchased commercially. Benzonitrile (Wako, guaranteed reagent) was used as received. Steady-state absorption, transient absorption spectra, cyclic voltammetry (CV), differential pulse voltammetry (DPV) and MFEs were measured according to the previous papers [9–12]. The temperature of the sample solutions was controlled by circulating the temperature-controlled water through the water jacket equipped with the quartz cell.

3 Results and discussion
3.1 Absorption spectra and electrochemical measurements
Absorption spectra of Ph(6)C60 and C60ref were measured in benzene. The Ph moiety has no absorption band above 400 nm. Two spectra were essentially same in the 400–800 nm region. Similar results were also observed in benzonitrile. These results are in good agreement with those in Ph(n)C60 (n = 8–12) as reported previously in [9–11]. Thus, it is suggested that there are no appreciable electronic interactions between the C60 and the Ph moieties in Ph(6)C60 in the two solvents.
Redox potentials of Ph(6)C60, C60ref, and MePh by CV and/or DPV in benzonitrile were measured. Two redox couples were observed for Ph(6)C60 (E1/2(Ph•+/Ph) = +0.26 V and E1/2(C60/C60•–) = –1.00 V versus Fc+/Fc). These values are roughly the sum of MePh (E1/2(Ph•+/Ph) = +0.30 V versus Fc+/Fc) and C60ref (E1/2(C60/C60•–) = –0.98 V versus Fc+/Fc). These results are in good agreement with the absorption spectra, showing no appreciable electronic interaction between the C60 and the Ph moieties in the ground state.
Thermodynamic data for the present intramolecular electron-transfer reactions in Ph(6)C60 were evaluated as reported previously [9–12]. In the case of benzonitrile, accurate driving forces ΔG (ΔGCR, ΔGCS(S) and ΔGCS(T)) for the intramolecular charge recombination (CR) process from C60– to Ph•+ and for the intramolecular charge separation (CS) processes from Ph to 1C60* (S) and 3C60* (T) were calculated using the redox potentials of C60 and Ph moieties as described above. A centre-to-centre distance (Rc) between Ph and C60 moieties for Ph(6)C60 was estimated to be 14.0 Å by assuming the conformation based on MM2 analysis. The Coulombic term in Ph(6)C60 can be negligible because of the relatively large Rc and the high dielectric constant (ε = 25.2) of benzonitrile. The evaluated Gibbs free energy changes are summarised in Table 1.
Gibbs free energy changes (ΔGCS(S) and ΔGCS(T)) for CS from Ph to 1C60*(S) and 3C60*(T) and Gibbs free energy change (ΔGCR) for CR in Ph(6)C60
| ΔGCS(S) | ΔGCS(T) | ΔGCR | |
| Benzonitrile | –0.50 | –0.24 | –1.26 |
| Benzene | 0.28 | 0.54 | –2.04 |
In the case of benzene, the free energy changes, ΔG (ΔGCR, ΔGCS(S) and ΔGCS(T)), were calculated from the Rehm-Weller and Born equations [9–11] by using the redox potentials in benzonitrile for convenience (Table 1). However, it should be noted that the Born equation was tentatively used due to the overestimation of the non-polarity of benzene.
3.2 Transient absorption spectra
Transient absorption spectra in benzene and benzonitrile were measured to elucidate the photoinduced electron-transfer reactions in Ph(6)C60 by nanosecond laser-flash photolysis. The transient absorption spectra of Ph(6)C60 by laser excitation at 532 nm in both solvents are shown in Fig. 1. In benzene, the broad absorption band around 700 nm due to T1–Tn absorption of the C60 moiety was observed (Fig. 1a). No appreciable absorption bands due to Ph•+ were observed. Therefore, the results indicate that photoinduced electron-transfer reaction dose not occur in benzene. On the other hand, the T1–Tn absorption band of the C60 moiety disappeared and the absorption band due to the Ph•+ (λmax, 520 nm) [7–11] was clearly observed in benzonitrile, as shown in Fig. 1b. The results indicate that intramolecular electron transfer from Ph to 1C60* or 3C60* occurs, and then the biradical is generated in benzonitrile. Table 1 strongly indicates that the intramolecular electron transfer from Ph to 1C60* or 3C60* in Ph(6)C60 is thermodynamically favourable in benzonitrile, but unfavourable in benzene. This is in fair consistency with the solvent effect in the transient absorption spectra (Fig. 1). These results in Ph(6)C60 were in good agreement with those in Ph(n)C60 (n = 8–12) [9–11].
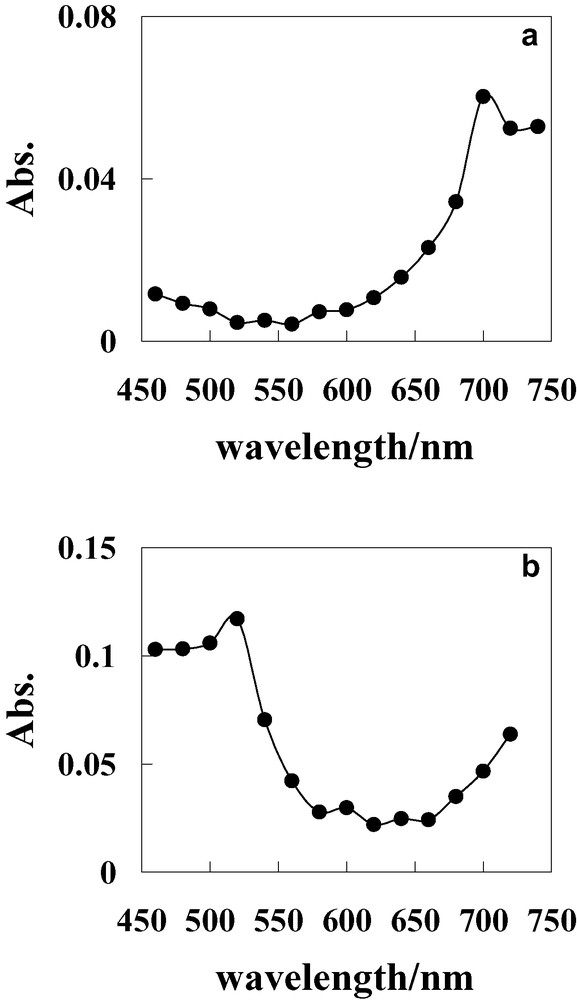
Transient absorption spectra of Ph(6)C60 (1.0 × 10−4 mol dm−3) (a) at 1.0 μs in benzene and (b) at 0.06 μs in benzonitrile after laser excitation at 288 K.
3.3 Temperature dependence of photoinduced electron-transfer reactions and the decay of photogenerated biradical
Transient absorption spectra of Ph(6)C60 were measured in benzonitrile at 283, 303, 323, and 343 K, respectively. The absorption band due to the Ph+ at 520 nm was observed at all temperatures as in Fig. 1b. The decay profiles of transient absorption at 520 nm were shown at 283 K (a) and 343 K (b) in Fig. 2. The decay curves can be expressed by the following equation:
| (1) |
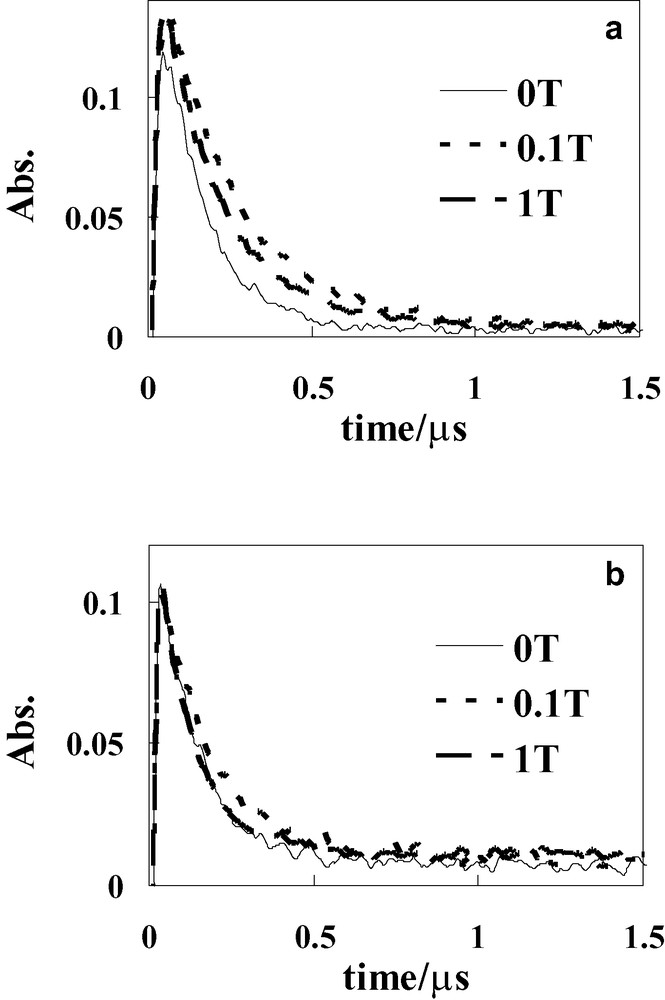
Temperature dependence on decay profiles of transient absorption at 520 nm of Ph(6)C60 in benzonitrile at 0, 0.1, and 1.0 T: (a) 283, (b) 343 K.
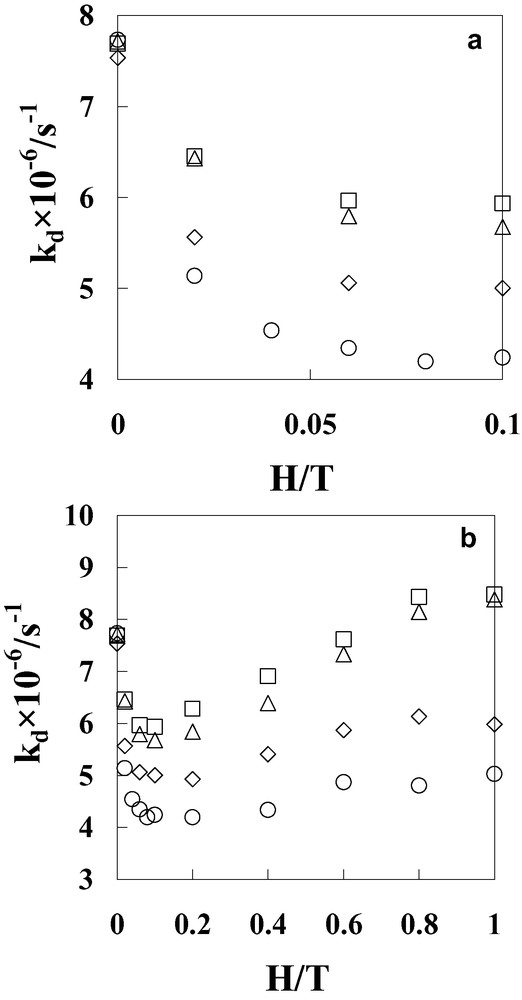
Temperature dependence of the MFEs on the decay rate constants (kd) for the biradical as evaluated from the transient absorption at 520 nm upon laser excitation of Ph(6)C60 in benzonitrile 283 (○), 303 (◊), 323 (∆), and 343 (□) K; (a) in 0 ≤ H ≤ 0.1 T and (b) in 0 < ≤ H < ≤ 1 T.

3.4 MFEs on the decay of biradical at various temperatures
The MFEs on the photoinduced electron-transfer in Ph(6)C60 were examined in benzonitrile at various temperatures (283–343 K) in Figs. 2 and 3. At lower temperature (283 K), the decay of transient absorption at 520 nm was suppressed in the presence of a low magnetic field (0.1 T), but became slightly faster at 1.0 T as compared with that at 0.1 T (Fig. 2a). With the increase of the magnetic field, the kd-value decreased steeply at lower magnetic fields (< 0.2 T), and then recovered slightly in the 0.2 T ≤ H < 0.6 T region and finally became almost constant in the higher magnetic fields (0.6 T ≤ H < 1.0 T), as shown in Fig. 3 at 283 K. As a result, the reverse phenomenon in the MFEs occurring at 0.2 T was clearly observed. Similar reverse phenomena were also observed in Ph(8)C60 and Ph(12)C60 at 288 K in a previous paper [11]. However, these reverse phenomena had not been discussed in detail, because the magnitudes of the reverse phenomena were very small.
In contrast, the reverse phenomena in the MFEs appeared more clearly with increasing temperature in Fig. 3. At 303 K, the magnetic-field dependence of the kd-value was similar as that at 283 K and the minimum of kd-value (at 0.2 T) was larger than that at 283 K. On the other hand, at 323 and 343 K, the minima of kd-values were observed at 0.1 T and showed a lower field shift as compared with those at 283 and 303 K. In addition, the kd-value decreased steeply at lower magnetic fields (< 0.1 T), and then recovered gradually (0.1 T ≤ H < 1 T). At 323 and 343 K, the kd-values above 0.8 T were larger than that in the absence of magnetic field (0 T) as shown in Figs. 2b and 3, in a similar manner as in the case of ZnP(8)C60 at 288 K [12].
The magnitudes of the MFEs were evaluated by the ratios (Δlow = kd(0 T)/kd(0.2 T) and Δhigh = kd(1.0 T)/kd(0.2 T)) in the case of the lower temperatures (283 and 303 K) or (Δlow = kd(0 T)/kd(0.1 T) and Δhigh = kd(1.0 T)/kd(0.1 T)) in the case of the higher temperatures (323 and 343 K), respectively. The Δlow-values at 283, 303, 323 and 343 K were estimated to be 1.84, 1.53, 1.36 and 1.30, respectively. Thus, the Δlow-value was reduced by 1.4 times on going from 283 to 343 K. On the other hand, the Δhigh-values at 283, 303, 323 and 343 K were estimated to be 1.20, 1.21, 1.48 and 1.43, respectively. Thus, the Δhigh-value was enhanced by 1.2 times on going from 283 to 343 K. On the basis of these observations, it is concluded that with increasing temperature the magnitudes of MFEs in lower magnetic fields were reduced, while those in higher magnetic fields were enhanced. As the result of the temperature dependence, the reverse phenomena in the MFEs were more clearly observed at high temperatures. In the Ph–viologen systems, similar reverse phenomena of the MFEs have been reported in the higher magnetic fields (> 1 T), but not in the lower magnetic fields (< 1 T) [16]. From the comparison with Ph–C60 and Ph–viologen linked compounds, the C60 moiety in Ph(6)C60 is most likely responsible for the present unusual phenomenon on the MFE [9–12].
The temperature dependences in the MFEs in the lower magnetic fields were clearly observed. Thus the MFEs (< 0.2 T) cannot be explained only by the isotropic hyperfine coupling (hfc) mechanism, since the hf interactions are independent on temperature. In the region of lower magnetic fields (< 0.2 T), MFEs on the kd for the triplet biradical can be explained by hfc mechanism and SLR mechanism due to the anisotropic hf and the dipole–dipole (dd) interactions [5,6]. The consideration is in fair agreement with that from a semitheoretical value (B1/2) [3,9,11,12].
In general, the MFEs for the biradical in higher magnetic fields can be explained by SLR mechanism due to anisotropic Zeeman interaction or Δg mechanism [3–6]. Δg mechanism is dominated by the difference of g-values between the donor and acceptor, and is independent of temperature. On the other hand, SLR mechanism due to anisotropic Zeeman interaction is dominated by the g anisotropy, and is dependent on temperature. Thus, the temperature dependence revealed that the MFEs in the higher magnetic fields are ascribed by SLR mechanism due to anisotropic Zeeman interaction.
The MFEs on the decay of the radical pair between a C60 cluster anion and a pyrene cation have been observed in a micellar system [20]. In addition, MFEs, at 77 K on the decay of biradical have been also reported in a carotenoid–porphyrin–C60 triad system, but no MFEs were observed at 298 K [26]. Those MFEs are different from the novel MFEs, as described in the donor-C60 linked compounds including the present case (Ph(6)C60). The temperature effect strongly supports that the novel MFEs in higher magnetic fields are ascribed to properties of C60 moiety and probably interpreted by the SLR mechanism due to anisotropic Zeeman interaction in donor-C60 linked systems. The present study provides useful informations for regulating the biradical lifetime in the donor-C60 systems in novel molecular spin systems. Further investigations on the Ph–C60 systems with different chain length and quantitative analysis of temperature dependence on the MFEs are in progress.
Acknowledgements
The authors are grateful to Mr. H. Horiuchi for the preparation of quartz cells for transient absorption spectral experiments. The authors also thank The Centre of Advanced Instrumental Analysis, Kyushu University, for 1H-NMR measurements. The present study was financially supported by the grants of the Inamori Foundation and the society for the support of Kyushu University, and by the Grant-in-Aids for Scientific Research: Priority Area of ‘Innovative Utilisation of Strong Magnetic Fields’ (Area 767, No. 15085203) and ‘Molecular Nano Dynamics’ (Area 432, No. 17034051), Scientific Research (C) (No. 17550131), and 21st Century COE Program ‘Function Innovation of Molecular Informatics’ from MEXT of the Japan.


