The inner core and the geodynamo

Stéphane Labrosse; Mélina Macouin

doi:10.1016/S1631-0713(03)00013-0

Internal Geophysics / Géophysique interne

The inner core and the geodynamo
[La graine et la géodynamo]

Stéphane Labrosse ¹ ; Mélina Macouin ¹

¹ Département de géomagnétisme et paléomagnétisme, Institut de physique du Globe, 4, place Jussieu, 75252 Paris cedex 05, France

Comptes Rendus. Géoscience, Volume 335 (2003) no. 1, pp. 37-50.

Résumés

Anglais
Français

Using energy and entropy constraints applicable to the Earth's core, the heat flow at the core–mantle boundary (CMB) needed to sustain a given total dissipation in the core can be computed. Reasonable estimates for the present Joule dissipation in the core gives a present heat flow of 6 to 10 TW at the CMB. Palaeointensity data acquired from rocks younger than 3.5 Ga provide support that the Joule dissipation in the core before inner core crystallization was between today's value and four times lower than today. Prior to inner core crystallization (around 1 Ga), the magnetic field was maintained by thermal convection driven by core cooling, and our calculations of the two extreme cases predict that the heat flow at the CMB at that time was either 14 to 24 TW in the case of constant dissipation, or essentially the same as today in the lower field intensity case.

Les contraintes thermodynamiques intégrales portant sur l'énergie et l'entropie dans le noyau de la Terre sont utilisées pour relier la dissipation dans le noyau au flux de chaleur à la frontière noyau–manteau (FNM). Des estimations raisonnables de la dissipation par effet Joule dans le noyau actuel nécessitent un flux de chaleur total entre 6 et 10 TW. Les données de paléointensités disponibles pour des âges inférieurs à 3,5 Ga peuvent être utilisées pour contraindre l'évolution de la dissipation par effet Joule au cours du temps, et en particulier pour la période avant l'existence de la graine, la dynamo fonctionnant alors uniquement par convection thermique. Les données étant très partielles, deux interprétations sont possibles : la dissipation est constante, indépendamment de la croissance de la graine, ou la dissipation par effet Joule était environ quatre fois plus faible avant l'apparition de la graine. Dans le premier cas, la convection thermique étant moins efficace que la convection compositionnelle, un flux de chaleur à la FNM entre 14 et 24 TW a été nécessaire il y a environ 1 Ga, alors que dans le second cas, un flux de chaleur proche du flux actuel était suffisant.

Métadonnées

Reçu le : 2002-10-22
Accepté le : 2002-10-28
Publié le : 2003-01-01

DOI : 10.1016/S1631-0713(03)00013-0

Keywords: Core thermodynamics, Thermal evolution, Geomagnetism, Palaeomagnetism, Palaeointensity, Earth's magnetic field
Mots-clés : Thermodynamique du noyau, Évolution thermique, Géomagnétisme, Paléomagnétisme, Paléointensité, Champ magnétique de la Terre

Affiliations des auteurs :

Stéphane Labrosse ¹ ; Mélina Macouin ¹

¹ Département de géomagnétisme et paléomagnétisme, Institut de physique du Globe, 4, place Jussieu, 75252 Paris cedex 05, France

@article{CRGEOS_2003__335_1_37_0,
     author = {St\'ephane Labrosse and M\'elina Macouin},
     title = {The inner core and the geodynamo},
     journal = {Comptes Rendus. G\'eoscience},
     pages = {37--50},
     publisher = {Elsevier},
     volume = {335},
     number = {1},
     year = {2003},
     doi = {10.1016/S1631-0713(03)00013-0},
     language = {en},
}

TY  - JOUR
AU  - Stéphane Labrosse
AU  - Mélina Macouin
TI  - The inner core and the geodynamo
JO  - Comptes Rendus. Géoscience
PY  - 2003
SP  - 37
EP  - 50
VL  - 335
IS  - 1
PB  - Elsevier
DO  - 10.1016/S1631-0713(03)00013-0
LA  - en
ID  - CRGEOS_2003__335_1_37_0
ER  -

%0 Journal Article
%A Stéphane Labrosse
%A Mélina Macouin
%T The inner core and the geodynamo
%J Comptes Rendus. Géoscience
%D 2003
%P 37-50
%V 335
%N 1
%I Elsevier
%R 10.1016/S1631-0713(03)00013-0
%G en
%F CRGEOS_2003__335_1_37_0

Stéphane Labrosse; Mélina Macouin. The inner core and the geodynamo. Comptes Rendus. Géoscience, Volume 335 (2003) no. 1, pp. 37-50. doi : 10.1016/S1631-0713(03)00013-0. https://comptes-rendus.academie-sciences.fr/geoscience/articles/10.1016/S1631-0713(03)00013-0/

Version originale du texte intégral

1 Introduction

The inner core of the Earth influences the dynamo in several ways. Since the proposition by Jacobs [22] of a mechanism for its formation by gradual crystallization accompanying core cooling, it has been recognized that this process may provide an important contribution to the power driving the dynamo. Verhoogen [52] pointed out that the inner core crystallization produces latent heat that could drive thermal convection in the outer core, whereas Braginsky [3] proposed that this process also produced a chemical differentiation that could drive compositional convection. Both processes have been accepted as part of the driving power for the dynamo and show an obvious connection between the inner core and the Earth's magnetic field.

The inner core also has a more dynamical effect on the generation of the magnetic field. Because of the fast rotation of the Earth, the fluid flow tends to be independent of z, the position along the axis of rotation (Proudman–Taylor theorem). For this reason, the tangent cylinder of the Earth's core, the imaginary cylinder that is parallel to the axis of rotation and tangent to the inner core, is thought to have an important role on the dynamics of the core. In some dynamo models (see [7] for a critical review) the magnetic field is primarily generated outside of that tangent cylinder, so that the volume available to that end decreases with the inner core growth much faster than the volume of the outer core. For this reason, Jault [23] suggested that if the radius of the inner core becomes larger than a critical size, r_c say, the dynamo would stop. Although the findings of Jault [23] rely on a particular choice of model, it is physically based on the model-independent Proudman–Taylor theorem and it has been found in other models since then [1,34]. The exact value of the critical radius r_c seems however model dependent. Glatzmaier and Roberts [11] do not find any sign of a critical size of the inner core, possibly because, in their model, the magnetic field is mainly produced in the tangent cylinder.

The inner core is also likely to affect the dynamo through its finite conductivity. As pointed out by Hollerbach and Jones [20], the diffusive timescale associated with the inner core exceeds 10³ yr, and imposes some stability to the magnetic field at that time scale. In particular, it was found in some numerical models of the geodynamo [10] that after the magnetic field reverses in the outer core, to be stabilized in the reverse direction, it needs to reverse in the inner core, necessarily by diffusion. Gubbins [13] argued that this is what makes the difference between an excursion and a reversal of the geomagnetic field: an excursion is a reversal in the outer core that did not succeed in propagating all the way in the inner core. However, these ideas were recently challenged by Wicht [53], essentially because of the small size of the inner core (4% of the volume of the core) and of the large periods (of order 10⁴ years) that dominates the dynamics of the outer core.

All these effects of the inner core on the dynamo have been studied by several authors, mainly in the context of the present time Earth. However, the inner core is a small fraction of the total volume of the core and its crystallization up to its present size can have been quite fast. Some recent studies [28,29] have found that the inner core is less than 2.5 Ga old, an age of 1±0.5 Ga being preferred. It is then tempting to try and predict the expected signature of the apparition of the inner core on the palaeomagnetic record and search for it in the data. The uncertainties on physical processes involved as well as on the palaeomagnetic record render the task formidable and all the conclusion that are given are rather speculative. We will then try to emphasize, in the course of the present paper, where improvements are the most needed.

In this paper, we will concentrate on the effect of the inner core on the available power to drive the geodynamo and the lack of the corresponding power before the inner core started to crystallize. Sakuraba and Kono [43] have demonstrated the feasibility of a dynamo in the absence of inner core, the driving power coming from internal heating or secular cooling. However, their model uses a Boussinesq approximation and, as will be developed below, the major difficulty in driving a dynamo before the inner core comes from maintaining the adiabatic gradient with a limited heat flux at the core mantle boundary (CMB), a problem that cannot arise in such a model.

2 Thermodynamics of the core

2.1 Average profiles

Several time scales are involved in the dynamics of the core, but they are not all relevant to the problem considered in the present paper, and we need to separate them. This matter is only briefly presented here, but is discussed in details in [4]. Since we are interested in the thermal evolution of the core, the time scale is imposed by the total heat loss of the core, the heat flow at the core mantle boundary (CMB) that is controlled by mantle convection. All processes occurring with time scales shorter than, say, the million year can therefore be averaged. In particular, the convection processes, at the origin of the magnetic field, only enter in the problem in maintaining the outer core in a state close to hydrostatic, well mixed and adiabatic, which respectively reads:

\frac{\partial P}{\partial r} = - ρ g

(2.1)

\frac{\partial ξ}{\partial r} = 0

(2.2)

\frac{\partial T}{\partial r} = - \frac{α g T}{C_{P}}

(2.3)

with P, ξ and T the pressure, composition in light elements and temperature, ρ and g the radius-dependent density and acceleration due to gravity and α and C_P the coefficients of thermal expansion and the heat capacity by unit mass. The temperature being adiabatic means that any given fluid parcel that is moved up- or downward fast enough that it does not exchange heat, stays in dynamic equilibrium with its surrounding. Its temperature changes only due to its compressibility following Eq. (2.3). Eqs. (2.2), (2.3) also imply that the outer core is isentropic (e.g., [4,28]).

The light element is kept unspecified here, and it is likely to actually be a mixture of several elements [40]. This question might be of importance, since the composition influences the physical characteristics of the outer core and, in particular, the way the mixture is affected by crystallization at the ICB has a major effect on the energy available to drive the dynamo. Gubbins et al. [15] argue that only oxygen is likely to be significantly released upon freezing the outer core mixture and is then the only light element useful for driving the dynamo. These questions are still debated and we will keep the light element unspecified, the fraction of which that fractionate at the inner core boundary (ICB) being a variable parameter in the problem.

To supplement these equations, one needs an equation of state relating the density ρ to the pressure and temperature and the equation for the acceleration due to gravity, $g (r) = \frac{4 π G}{r^{2}} \int_{0}^{r} u^{2} ρ (u) d u$ with G the gravitational constant. This system of coupled equations can be solved iteratively to any required precision [29], providing the boundary condition that the temperature at the ICB (r=c(t)) is equal to the liquidus at this depth. The adiabatic temperature profile is then conveniently written as

T_{ad} (r, t) = T_{s} [c (t)] \exp [\frac{c^{2} (t) - r^{2}}{D^{2}}] with D = \sqrt{\frac{3 C_{P}}{2 π α ρ_{c} G}}

(2.4)

The solidification temperature T_s can be expressed in a similar form by use of the Lindemann law of melting [29].

The fluctuation around this profile average to zero in most terms relevant to the thermal evolution, that is everywhere except when their correlations with the velocity field are involved.

2.2 Energy balance

The energy balance of the core reads [4–6,12,16,28–30]

Q_{CMB} = Q_{ICB} + Q_{C} + Q_{L} + Q_{G} + Q_{R}

(2.5)

and states that the total heat loss of the core, the heat flux at the core mantle boundary, Q_CMB, is balanced by the sum of the heat sources: the heat flow coming from the inner core, Q_ICB, the secular cooling, Q_C, the latent heat, Q_L, the gravitational energy, Q_G and the radiogenic heat, Q_R. In this equation, the pressure heating has been neglected [14,29] as well as the heat of reaction, and the pressure effect on freezing can be included in the latent heat [14]. All these source terms, save Q_R, can be expressed as

Q_{X} = P_{X} (c) \frac{d c}{d t}

(2.6)

The detailed expressions for all the P_X have been computed elsewhere [29] and will not be replicated here. The fact that all these terms can be expressed in terms of the inner core radius and growth rate is not obvious for all these terms and need some explanations.

The largest source is the secular cooling of the core, Q_C, and can be written as

Q_{C} = - \int_{V} ρ C_{P} \frac{\partial T_{ad}}{\partial t} d V

(2.7)

with V the volume of the outer core. To a good approximation [29], the inner core can be assumed adiabatic so that Q_C can include the cooling of the inner core (Q_ICB). The above expression (Eq. (2.7)) is kept the same except that V is then the total volume of the core. The expression of the adiabatic temperature profile (Eq. (2.4)) shows that the only time variation for this profile is linked to the radius of the inner core, justifying the expression (2.6) for this source term.

The latent heat of crystallization is obviously related to the growth rate of the inner core, but the case of gravitational energy is more complex. When the temperature of the outer core decreases, some outer core material close to the ICB crystallizes and becomes part of the inner core. Assuming that the outer core is on the iron-rich side of the eutectic, the concentration of light elements in the solid formed is smaller than the concentration in the liquid. Conservation of matter implies that the remaining light elements are released to the outer core where they drive compositional convection. This process, responsible in part for the generation of the magnetic field, enters as a source in the energy balance due to the rearrangement of light elements in a non-uniform chemical potential field [4]. This term is not equal to the total change in gravitational energy of the core, as claimed in [47], but only to the part due to chemical differentiation of the inner core (see Appendix B of [4]). The gravitational energy of the core also changes, due to freezing of the inner core and thermal contraction of the core and mantle, but this does not enter in the global energy balance and is merely balanced by the work of the pressure forces on the CMB during contraction [16]. The part that enters the energy balance can be computed rigorously (e.g., [4,15,29]) but it suffices here to say that it is proportional to the rate of release of light elements at the ICB, and by conservation of matter to the rate of growth of the inner core. This justifies Eq. (2.6) for Q_G.

This discussion about the gravitational energy change of the core and the part of which that enters the energy balance shows that it is improperly named and should rather be called compositional energy. This energy term would still be present in the balance in an imaginary steady-state situation where the light elements enter at the bottom of the system through a membrane and goes out at the same rate through an upper surface membrane. In this situation, the gravitational energy is kept constant, but a compositional energy term is present in the global energy balance. In the case of the core, the CMB is usually assumed to be impermeable to the light elements and the release of these elements at the ICB and their mixing in the outer core make the gravitational energy of the core decrease and this part of the gravitational energy decrease is equal to the compositional energy term [4].

Finally, the heat equation can be written as:

Q_{CMB} = [P_{C} (c) + P_{L} (c) + P_{G} (c)] \frac{d c}{d t} + h (t) M_{N}

(2.8)

If the heat flow at the CMB is known as a function of time, the growth history of the inner core can be computed from this equation for any given radiogenic heat generation per unit mass h, M_N being the mass of the core.

2.3 The age of the inner core

If one is not interested in the exact growth history of the inner core, but rather in the onset of its crystallization, Eq. (2.8) can be integrated between this time t=−a and the present, t=0, where the present radius of the inner core c=c_f is well known, to give:

\underset{- a}{\int^{0}} Q_{CMB} (t) d t = \underset{0}{\int^{c_{f}}} [P_{C} (c) + P_{L} (c) + P_{G} (c)] d c + \underset{- a}{\int^{0}} M_{N} h (t) d t

If the concentration in radioactive elements in the core and Q_CMB(t) are known as a function of time, this equation can be solved for the age of the inner core a [29].

The heat flux at the base of an internally heated convective system being dominated by the arrival of cold down-welling structures (plates in the Earth's mantle) [27], it can be argued [29] that this heat flux should roughly follow the exponential decrease of the radiogenic heat in the mantle. Exploring such time evolution laws within reasonable bounds, Labrosse et al. [29] obtained a maximum age of 2.5 Ga for the inner core and a value of 1±0.5 Ga strongly favoured. The magnetic field of the Earth is known to have existed for at least 3.5 Ga (e.g., [18,32]), which means that the mode of its generation may have changed when the inner core started to grow.

2.4 The entropy balance

The energy balance presented in Section 2 is not sufficient to derive the efficiency factors that are needed to relate the strength of the magnetic field to the energy sources. The reason for this is well known: the dissipation of the system does not enter in the global energy balance, because it corresponds to an exchange of energy inside the core. Efficiency calculations applied to convective flows differ from their Carnot engine counterpart in that the useful work is done inside the system and can, in some cases, exceed the heat flows at the boundaries [2,19].

This section follows the arguments first developed by Backus [2] and Hewitt et al. [19] and subsequently developed by several authors [4,14–16,42] and applies them to the present core as well as for the period prior to the inner core formation. The details of the derivation are not given here but can be found in the cited literature. The general line is easy to understand: the local entropy equation is integrated over the outer core and this equation is then combined with the energy equation (2.5) to relate the dissipation to the energy sources.

The entropy equation integrated on the volume V of the outer core is:

\frac{Q_{CMB}}{T_{CMB}} = \frac{Q_{ICB} + Q_{L}}{T_{ICB}} + \int_{V} k {(\frac{\nabla T}{T})}^{2} d V + \int_{V} \frac{ϕ}{T} d V + \int_{V} \frac{ρ}{T} (h - C_{P} \frac{\partial T_{ad}}{\partial t}) d V

where each heat source appears divided by the temperature at which it is supplied. The local dissipative shear and Joule heating:

ϕ = \frac{J^{2}}{σ} + τ : (\nabla u)

with

J

the electric current density, σ the electrical conductivity,

u

the fluid velocity and

τ

the viscous stress tensor, is usually assumed to be largely dominated by the Joule heating part. The gravitational energy can then be reintroduced in the problem by the use of the energy equation (2.5):

\int_{V} \frac{ϕ}{T} d V + \int_{V} k {(\frac{\nabla T}{T})}^{2} d V = \frac{Q_{G}}{T_{CMB}} + (Q_{ICB} + Q_{L}) (\frac{1}{T_{CMB}} - \frac{1}{T_{ICB}}) + \int_{V} ρ (h - C_{P} \frac{\partial T_{ad}}{\partial t}) (\frac{1}{T_{CMB}} - \frac{1}{T}) d V

(2.9)

Several implications of this equation need to be pointed out. First, each thermal source for the dynamo, X say, that is provided to the core at the temperature T_X, is multiplied by a factor of the form (1/T_CMB−1/T_X) which is the classical Carnot efficiency of heat engines. On the other hand, the gravitational energy due to the inner core chemical differentiation enters in the entropy equation (2.9) with a much larger factor of 1/T_CMB, as was originally proposed in [3]. The secular cooling and radioactive heating, that are mathematically equivalent [25], enter with a small efficiency because these sources are distributed over the whole core. Of course, these efficiency arguments are only indicative and the exact part of each heat source to the process of the geodynamo depends on the total energy that each source provide as well. The respective amplitude of these sources can be related to the growth rate of the inner core, as was done for the energy balance in Section 2.2.

Another point to make is that all the energy sources on the right-hand side of Eq. (2.9) are not only used to produce the magnetic field that appear as Joule heating in the dissipation term, but also to maintain the conduction along the adiabatic temperature gradient. This second term on the left-hand side can be computed by use of Eq. (2.4) to give

\int_{V} k {(\frac{\nabla T}{T})}^{2} d V = 4 π \underset{c}{\int^{b}} k {(\frac{α g}{C_{P}})}^{2} r^{2} d r ≃ 150 MW K^{- 1}

with b the radius of the CMB.

The question that we want to answer now is: what are the requirement for the core to be able to sustain a magnetic field of a given strength? By reasonably assuming the viscous part of the dissipation negligible, one could estimate the total ohmic dissipation for this given magnetic field and use the entropy equation (2.9) to constrain the energy sources that are necessary to sustain it. All the source terms of Eq. (2.9), except the one arising from the radioactive heating, can be expressed as a function S_c of the inner core radius times the inner core growth rate:

\int_{V} \frac{ϕ}{T} d V + \int_{V} k {(\frac{\nabla T}{T})}^{2} d V = S_{c} (c) \frac{d c}{d t} + \int_{V} ρ h (\frac{1}{T_{CMB}} - \frac{1}{T}) d V

(2.10)

This growth rate can then be inferred from the entropy requirements for any given radioactive heating rate. This can then be injected in the energy equation (2.5) to get the heat flux at the CMB necessary to produce this magnetic field. One must realize that the solution is not unique as the radioactive heat in the core and the age of the inner are not known, so that only a relation between these two parameters is obtained.

This procedure, although simple, is in practice not easy, since only part of the magnetic field is measured at the surface, the large-scale poloidal field. Using this part of the field can only provide a lower bound for the total dissipation and the contribution of intermediate to small scale currents, possibly via turbulent dissipation [4,42], as well as the total contribution from the toroidal field that is confined to the core may be much larger. Roberts et al. [42] estimate the total ohmic dissipation to be between 1 and 2 TW, about 20 times larger than the large scale dissipation that could possibly be estimated from the surface of the Earth. To go from this estimate to the first term on the left-hand side of Eq. (2.9) requires the knowledge of the effective temperature at which this dissipation occur, which they call T_D. This temperature is not known, but T_ICB>T_D>T_CMB, which converts to $200 MW K^{- 1} ⩽ \int_{V} (ϕ / T) d V ⩽ 600 MW K^{- 1}$ . On the other hand, Gubbins et al. [14] estimate that the entropy production due to ohmic heating is between 500 and $800 MW K^{- 1}$ . Using these estimates, Roberts et al. [42] find that a heat flux at the CMB between 6 and 10 TW is required to sustain their estimate for the present dissipation without radioactivity, whereas Gubbins et al. [15] find 16 TW in the same situation. Both proposition give an inner core around 1 Ga old or less, in agreement with the results of [29]. It is however well known that the geodynamo operated for much its history (Section 3) and the question of dynamo efficiency before the existence of the inner core needs to be addressed.

2.5 Efficiency of the dynamo before the inner core

Before the onset of the inner core crystallization, the dynamo must have operated by thermal convection alone. This section is devoted to the dynamo requirements in that case. These come from a simplification of the previous derivation, after suppressing the energy sources coming from the inner core growth, the latent heat of freezing and the gravitational energy due to chemical differentiation. The energy equation simply expresses a balance between the heat flux at the CMB and the sum of secular cooling and radiogenic heating:

Q_{CMB} = \int_{V} ρ [h (t) - C_{P} \frac{\partial T_{ad}}{\partial t}] d V

(2.11)

where now the adiabatic temperature profile has to be parameterized by the temperature either at the centre or at the CMB, and the secular cooling term can be computed by the use of the average profiles of density and temperature (Section 2.1) and is proportional to the time-derivative of the temperature at the centre T_c:

- \int_{V} ρ C_{P} \frac{\partial T_{ad}}{\partial t} d V = - 4 π ρ_{c} C_{P} ℐ \frac{d T_{c}}{d t}

ℐ

is the integral of the exponentials entering the expression for the average temperature and density profiles and can be easily computed analytically [29].

The entropy equation is also obtained from a simplification of Eq. (2.9) where only the last term on the right-hand side is kept. This term contains the contribution from the radiogenic heat and the secular cooling, the latter being expressed as a function S_T of T_c times dT_c/dt:

\int_{V} \frac{ϕ}{T} d V + \int_{V} k {(\frac{\nabla T}{T})}^{2} d V = - S_{T} (T_{c}) \frac{d T_{c}}{d t} + \int_{V} h (\frac{1}{T_{CMB}} - \frac{1}{T}) d V

(2.12)

Gubbins et al. [14] find that a heat flux of 30 TW across the CMB in necessary to maintain the present dissipation without the inner core whereas Roberts et al. [42] find that the dynamo efficiency is around 3% in the absence of the inner core and radioactivity.

2.6 Buoyancy fluxes

Following [19], the total dissipation can also be estimated by making use of a well-known equilibrium between the work of buoyancy forces on one side and the total dissipation on the other (e.g., [2–4,6,12,19,28,30]):

\int_{V} ϕ d V = - \int_{V} u \cdot (\nabla P - ρ g) d V

(2.13)

This equation is strictly valid only if one neglects the rate of change of kinetic and magnetic energies [12] or averages over a convective cycle [4].

This equation implies clearly that dissipative heating, hence dynamo action, can only arise from deviations from the hydrostatic balance (Eq. (2.1)). These deviations, although small, cannot be neglected in Eq. (2.13) since they are multiplied by the large velocity, as discussed in Section 2.1. Hewitt et al. [19] proposed to use this equation to estimate the total dissipation from the buoyancy flux in thermal convection. Lister and Buffett [30] extended that approach to the core case where both thermal and compositional buoyancy fluxes contribute to Eq. (2.13). This equation can be developed around the hydrostatic balance to give:

\int_{V} ϕ d V = - \int_{V} ρ_{0} u \cdot (ξ' \nabla μ + s' \nabla T) d V

(2.14)

with ρ₀ the averaged (hydrostatic) density, ξ′ and s′ the perturbation of composition and entropy around the average profiles and μ the chemical potential. Lister and Buffett [30] argue that this equation makes no distinction between chemical and thermal buoyancy, so that both processes power the dynamo equally well. This statement seems to contradict the arguments based on the entropy equation (2.9), but one must realize that the distinction between thermal and compositional convection lies in the link between the buoyancy fluxes and the total energies available. In particular, the compositional buoyancy fluxes can be computed from the reference state and the buoyancy provided at boundaries, whereas the thermal buoyancy fluxes are affected by the distribution of the dissipation through the local equation of energy conservation and cannot be computed a priori without solving the full dynamo solution. In particular, depending on the distribution of the dissipation in the core, the thermal buoyancy will be enhanced (if it is released close to the ICB) or decreased (if it is closed to the CMB). This uncertainty is the same as the one involved in transforming the total dissipation into its contribution to the entropy equation, that is in the correct value of the temperature T_D (Section 2.4). Lister and Buffett [30] show that this uncertainty is of the order of 15%, which may be considered as negligible if the total dissipation is small compared with the other heat sources. On the other hand, if the estimate of the total dissipation of Roberts et al. [42] holds, 1 TW⩽Φ⩽2 TW, it is of the same order as the other heat sources and this uncertainty needs to be considered.

A further issue deserves a careful examination: what happens to the buoyancy provided on the right-hand side of Eq. (2.14) in the case of non-dynamo solution? One is forced to accept that the viscous dissipation has then to be large enough to equilibrate the work of buoyancy forces. In the present core, the molecular viscosity of liquid iron at core condition is too low [39] to dissipate the work of buoyancy fluxes, and Braginsky and Roberts [4] showed that in the core even the turbulent viscosity gives a negligible contribution to the total dissipation. However, viscous dissipation strongly depends on the scale of movements, and it has to be much different when no dynamo is operating. It is well known that the magnetic field tends to increase the spatial scales of the flow hence reducing viscous dissipation. A simple scenario for the transition from the weak field to the strong field branches of the geodynamo can then be proposed: when a weak field of finite amplitude develops for a Rayleigh number larger than the critical Rayleigh number for dynamo action, the magnetic field makes the viscous dissipation decrease by enlarging the convective structures of the flow, leaving more room on the left-hand side of Eq. (2.14) for Joule dissipation, leading to a further decrease of viscous dissipation. This process may then amplify until viscous dissipation becomes negligible and all the work of buoyancy forces is balanced by Joule heating. This scenario is an alternative view to the more classical view based on force balances (e.g., [46]). Conversely, when the importance of buoyancy sources is decreased in a strong field dynamo situation, there will be a point where the decrease of the magnetic field and the associated Lorenz force will tend to increase the viscous dissipation which will slow further down dynamo action. Since the level of Joule dissipation at which this transition occurs is not known, it is not possible to infer the minimum energetic requirements for strong dynamo action. It would be tempting to get that value by setting a zero dissipation in the entropy equation but the previous discussion shows that it is incorrect: the dynamo process would undergo a transition from strong field to weak field before and the dissipation would then be mostly viscous. On the other hand, for all the situations where a strong magnetic field exists, as is the case for most of the Earth's history, the viscous dissipation plays no role and the requirement to maintain a given Joule dissipation can be derived from the entropy equation.

3 Constraints from palaeointensity data

It is tempting to search the palaeomagnetic records for an evidence of the onset of the inner core crystallization. As was presented above (Section 2) the dynamo process is more efficient when an inner core is growing and driving compositional convection than before. For this reason, Stevenson et al. [48] predicted a sharp increase in the intensity of the magnetic field on the appearance of the inner core. Although the inner core takes some time to reach an appreciable size, precise calculations [28,48] indeed predict a rather fast increase of the energy sources related to that growth. Hale [17] claimed that such an increase could be seen in his palaeointensity curve, at 2.7 Ga. However, this intensity increase is only constrained by one data at 2.5 Ga and needs to be reconsidered.

Before turning to the data, one must realize that the link between the power available for the dynamo and the intensity is far from obvious. As discussed in Section 2.4, the available power relates to the ohmic dissipation but the dipole field is a small contribution to the total dissipation. The assumption underlying the prediction of Stevenson et al. [48] is that the available power affects the magnetic field at all scales in the same way, keeping constant the shape of its spectrum during its time evolution. This assumption is quite strong and could well be found erroneous with future developments of the dynamo theory. In particular, it has been found recently [26] that the ratio of the dipole to the rest of the magnetic field may be very sensitive to the value of the Rayleigh number. However, all present dynamo models are still operating in a regime that is quite remote from the Earth's one [7] and the assumption made by Stevenson et al. [48] will be used in the rest of the paper for the sake of discussion. It means that the total ohmic dissipation will be assumed to scale as the square of the intensity of the dipole.

We select, from the Montpellier intensity database [36,37,50], data obtained from Thellier–Thellier-type experiments [51] using pTRM checks, the only technique that provides robust palaeointensity data. During this procedure, consistency checks are regularly performed in order to detect alteration or irreversible changes in magnetic mineralogy. The use of other techniques, such as alternating field method, systematically overestimate the average Virtual Dipole Moment (VDM) [24]. We added to the database selection Precambrian palaeointensity measurements performed in the last years [33,44,49,54,55]. Recently, Macouin et al. [31] obtained 22 new intensity estimates from Canadian dykes well-dated between 1 and 2.4 Ga, increasing significantly the Precambrian intensity database. The data that are marked as transitional are also removed from the analysis, because they would bias the result to low intensity without being representative of the long term evolution of the field. Finally, 87 data for the 1–300 Ma and 45 data for the Precambrian are selected.

Selected VDMs are plotted in Fig. 1, where each point represents one cooling unit (lava flow, dyke), so that one study can provide more than one point. The clustering of the data points then represent the short-term variability of the field as well the quality of the sampling.

Fig. 1
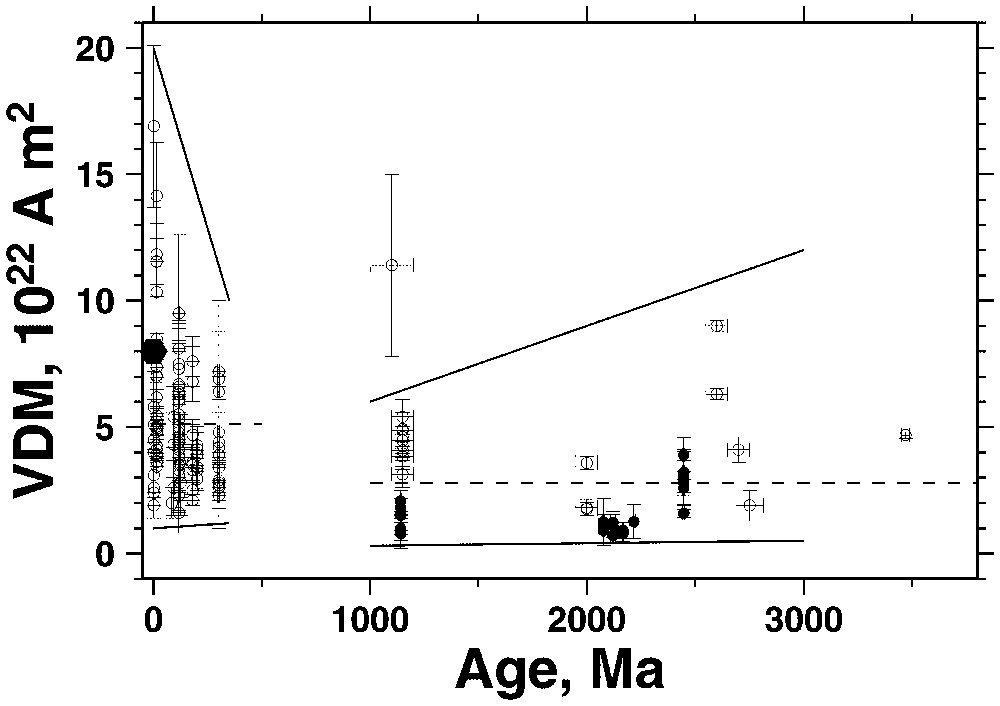
Virtual dipole moment (VDM) as a function of age. Open circles represent data from the database that are selected and solid circles represent new data from [31]. The large hexagone is the present value. The dashed lines are the average values for the recent and ancient times, whereas the solid lines are tentative envelop curves for the same periods.
Moment du dipôle virtuel en fonction de l'âge. Les cercles vides représentent les données de la base qui sont sélectionnées (voir texte) et les cercles pleins sont les nouvelles données de la référence [31]. L'hexagone noir est la valeur du dipôle actuel. Les lignes tiretées sont les valeurs moyennes pour les périodes anciennes et récentes, alors que les lignes pleines sont des enveloppes approximatives du signal pour les mêmes périodes.

At first sight, most intensity data for the period before 1 Ga ago seem of lower intensity than in the more recent period. However, two studies reported rather high intensity data that are plotted in Fig. 1. The 1.1-Ga-old $11.4 \pm 3.6 \times 10^{22} A m^{2}$ value obtained by [38] has been kept in the figure, despite the fact that it is not supported by pTRM checks, because it is often cited. However, it has not been used in the computation of the averaged VDM. Two other rather high values are the $9 \pm 0.2 \times 10^{22} A m^{2}$ and $6.3 \pm 0.2 \times 10^{22} A m^{2}$ intensities obtained from two 2.6-Ga-old dykes of the Slave Province (Canada) [54]. The largest of these values is consistent with the 2.5-Ga-old one used by [17] to claim the appearance of the inner core (which is not kept here because it does not satisfy the selection criteria) and is indeed larger than the few older data, but it is also larger than most younger values, as well as the other value from the same study. Furthermore, when looking at the intensity variations in the better sampled recent period (age ⩽301 Ma) one can see important variations on short time scales, not related to the growth of the inner core. The mantle driven variations of the heat flux at the CMB can produce variations of the magnetic field on 100 Ma time scales and the turbulent dynamics of the core, even under perfectly constant boundary condition, can produce variations on shorter time scales. In particular, the dipole field is responsible for a very small portion of the total dissipation in the core [42] and the variations of the dipole moment do not imply variations of the total dissipation on the same timescales: it might just correspond to redistribution of energy between the dipole (represented in Fig. 1) and higher-order components of the field. These variations of the spectrum of the magnetic field may very well occur at constant total dissipation. An uneven sampling of such variations can easily lead to incorrect conclusions and one must use the envelope of the signal to obtain any robust result.

It can be seen from Fig. 1 that the present dipole ( $8 \times 10^{22} A m^{2}$ ) is rather high compared to the recent period (1–301 Ma) average of $(5.1 \pm 2.6) \times 10^{22} A m^{2}$ (note that this value is not significantly different from the value of $4.6 \pm 3.2 \times 10^{22} A m^{2}$ obtained by [45] for the 0.3–300 Ma using the database and new data from submarine basaltic glass). Moreover, the 301 Ma of the recent period represent approximatively twice the age of the oldest ocean and is then a timescale in which the heat flux at the CMB can vary, but likely not by orders of magnitude. The spatial configuration of the heat flux at the CMB can change on that timescale and affect the spectrum of the magnetic field, even if keeping the net heat flow constant. For all these reasons, we will represent the whole period by a unique value for the dissipation, with a large error bar. The values discussed before (Section 2.4) refer to the present magnetic field, which has a moment of $8 \times 10^{22} A m^{2}$ , but smaller values could be defended for the recent period based in Fig. 1.

The VDM averaged over the period before 1 Ga old is $(2.8 \pm 1.8) \times 10^{22} A m^{2}$ , that is more than twice lower than the present value and 55% of the average for the period 1–301 Ma. These differences are small compared to the short-term variation within each period, but they may be significant. In the following, two alternative interpretations of Fig. 1 will be considered. The intensity of the field is essentially constant on the time scale of the thermal evolution of the Earth, so that the same Joule dissipation has to be maintained by core cooling before the existence of the inner core or the intensity of the field before the inner core is half the recent one, giving a Joule dissipation that is 25% of the present one. The former interpretation is essentially the one proposed by Prévot and Perrin [41], who stressed the limited number of data, in particular for the Precambrian, but the larger present number of available data can be used to argue for the latter interpretation.

4 Dynamo requirements through time

We will try in this section to construct a thermal history of the core that is able to produce the magnetic field before the onset of the inner core crystallization (a period we will term ancient), as well as the present field, although by different processes. The ancient magnetic field must be sustained by thermal convection only, powered by secular cooling of the core and possibly some radiogenic heat, whereas the present magnetic field is sustained by both thermal and compositional buoyancy sources.

We have seen in the previous section that there is a suggestion of a magnetic field having a somewhat smaller intensity during the Precambrian than in the last few hundreds of Ma. Considering the very sparse sampling of the Precambrian period, such a suggestion must be taken with great caution until more measurements are made. We will then use the intensity of the ancient magnetic field relative to the present one as a control parameter for the model. The total dissipation for the ancient core will be assumed to scale as a square of this parameter time the present dissipation.

Another control parameters of the model is the concentration in radioactive elements. It was usually assumed, in the recent literature, that the Earth's core does not contain a significant amount of any radioactive elements (although the possibility was included in [29]), but Roberts et al. [42] used data on partitioning coefficient between silicates and a iron–nickel alloy [35] to infer the other extreme possibility, that is the largest concentration of potassium that could have been incoporated in the core during its formation. This maximum value they obtained, 1420 ppm, gives a present-day heat generation in the core of more than 9 TW. If confirmed, such an important heat generation would change considerably the current views on the thermal evolution of the Earth and its magnetic field generation. However, Gessmann and Wood [9] criticized the results of [35] and predicted an upper bound of 2 TW for the present heat production by potassium in the core, and strongly preferred a much smaller value. Consequently, the concentration of radioactive elements will be assumed negligible in the following of the paper.

The general strategy is simple: given a total dissipation in the present core, the growth rate of the inner core needed to sustain it is obtained from the entropy equation (2.10) and is then injected in energy equation (2.8) to obtain the corresponding heat flow at the CMB. For a time before the inner core crystallization, the total dissipation is first obtained from the present one and the ratio between ancient- and present-field intensities. This dissipation is then converted into cooling of the core, using the relevant entropy equation (2.12), which can then be used to infer the heat flux at this time (Eq. (2.11)). Although apparently simple, this procedure hides another parameter: the onset time of inner core crystallization. This should be a result of the whole procedure, since it can be computed from the heat flux at the core–mantle boundary (Section 2.3). However, to apply the thermodynamic procedure for the time before the inner core, we need to know at which time it has to be applied. This means that a consistency check has to be performed after the heat flow has been obtained. In practice, the thermodynamic balances are applied for the present and the time t=−1 Ga that can be argued to be before the inner core crystallization (Section 3). This provides two points for the time–heat flux curve which is then interpolated to compute the onset time of the inner core crystallization. There is no strong support for any special form of interpolation, but two options are used: either exponential in the case of a heat flux decreasing with time, arguing that this heat flux is primarily controlled by down-welling currents in the mantle [27,29] or linear in the case of a heat flux constant or slightly increasing with time. If the age of the inner core is found to be larger than the time at which the ancient dissipation is used, this time is adjusted and the computation is performed again, until convergence of the process. In practice, this situation was encountered only once (Table 1).

Table 1

Results of the efficiency calculation concerning the heat flux at the core mantle boundary (CMB), the age of the inner core and the temperature at the CMB just after core formation (t=−4.5 Ga). Two different values of the total dissipation have been considered and for each of these: the dissipation before the inner-core crystallization can either be the same as the present one (constant dissipation) or four times smaller (variable dissipation), corresponding to a magnetic field twice smaller. The term ‘ancient’ stands for an era before an age that is the maximum between the age of the inner core and 1 Ga

Résultats des calculs de rendement concernant le flux de chaleur à la frontière noyau–manteau (FNM), l'âge de la graine et la température à la FNM juste après la formation du noyau, il y a 4,5 Ga. Deux valeurs de la dissipation totale ont été employées et, pour chacune, la dissipation totale avant l'existence de la graine est supposée être, soit égale à la dissipation actuelle (constant dissipation), soit quatre fois plus faible (variable dissipation), correspondant alors à un champ magnétique deux fois moins intense. Le terme « ancient » se rapporte à la période avant un âge qui est le maximum entre l'âge de la graine et 1 Ga

	$\int (ϕ / T) d V (MW K^{- 1})$	350	700
	Q_CMB (TW) present	5.7	9.7
Constant	Q_CMB (TW) ancient	13	23
dissipation	age of the IC	923 Ma	620 Ma
	$T_{CMB} (t = - 4.5 Ga)$	8783 K	12940 K
Variable	Q_CMB (TW) ancient	6.4	8.7
dissipation	age of the IC	1.3 Ga	860 Ma
	$T_{CMB} (t = - 4.5 Ga)$	4241 K	4283 K

Two values of the present entropy contribution $\int (ϕ / T) d V$ from Joule dissipation, $350 MW K^{- 1}$ and $700 MW K^{- 1}$ have been studied, spanning a large range of proposed values and, for each one of these values, two scenarios: the total dissipation is either supposed to be the same before the onset of the inner-core crystallisation or to be 25% of the present value, corresponding to a magnetic field of half the present strength.

The results of the calculation given in Table 1 have been obtained with the same numerical values as used in [29], except the thermal expansion coefficient which is derived from the identity $α = γ ρ C_{P} / K_{S}$ , the Grüneisen parameter γ and C_P being kept constant and ρ/K_S being derived from our equation of state fitted to PREM [8]. This gives a thermal expansion coefficient that is variable with radius, but an averaged value of 1.3×10⁻⁵ K⁻¹ is precise enough for the present purpose [14].

The temperature at the CMB at t=−4.5 Ga is computed by assuming that the heat flux variation between the onset of the inner-core crystallization and the present can be extrapolated backward to that time. The equation of conservation of energy (2.11) then allows an analytic computation of the temperature at the beginning of the Earth's history.

As can be seen in Table 1, a present heat flow at the CMB of 6 to 10 TW is enough to sustain the present magnetic field, with the help of compositional convection and latent heat associated with the inner-core growth. If the same dissipation has to be maintained by cooling of the core only before the inner-core existence, a heat flow of 13 to 23 TW is necessary 1 Ga ago, the age of the inner core being then between 600 and 900 Ma. The largest dissipation considered requires a large increase of the heat flow at the CMB with age which in turn implies a very large initial temperature at the CMB. On the other hand, if the ohmic dissipation before the inner core is about a fourth of the present value, an hypothesis marginally supported by paleointensity data, a heat flow fairly constant with age is sufficient, giving an age of the inner-core of 1±0.3 Ga. This second scenario is preferred, since an increase of the available buoyancy should produce an increase in Joule dissipation, the viscous dissipation being negligible at all times. This shows, if necessary, that more paleointensity data, particularly for ages between 500 Ma and 1 Ga, would be highly valuable in understanding the thermal evolution and the dynamics of the Earth.

5 Conclusion

Modeling the thermal evolution of the core and the growth of the inner core requires the knowledge of the heat flux at the core–mantle boundary and of its variation with time [28,29], both of which being poorly constrained and depending on mantle convection [27]. Following [16] and [42], an entropy equation for the core can be used to relate the Joule dissipation in the core to energy sources available to drive the geodynamo and this theoretically offers the possibility of a mantle-independent model of cooling of the core as well as the use of palaeomagnetic data as constraints.

However, this approach suffers from many uncertainties, particularly concerning two points: the relation between the large-scale magnetic field and the total dissipation in the core and the Precambrian paleomagnetic record. The increasing amount of available satellite data gives a better knowledge of the present magnetic field [21] and, hopefully, the crustal magnetic field will soon be known with enough precision to allow a derivation of the poloidal magnetic field in the core to a smaller scale than presently. The toroidal magnetic field will however stay out of reach of this type of approach and its knowledge will have to rely on dynamo models. The present models are still too remote from the dynamical regime of the Earth [7] to be of much use in that respect, and the use of hyper-diffusivity render their prediction in terms of small scale dissipation unreliable.

Concerning the paleomagnetic record, difficulties come from the scarcity of the available data as well as their interpretation. The amount of data is being currently increased and the global record should then improve in the forthcoming years. It will however stay limited by the existing well-conserved magmatic outcrops.

Concerning the interpretation of the record, several challenging questions remain and are linked to the problem of the dissipation in the core. The very large amplitude variation on short time scales can be argued to come from processes internal to the core rather than variations in the energy sources available. The rather small variations on long time scales on the other hand has to be related to thermal evolution processes and can be interpreted either as a constant dissipation in the core (considering the large error bars) or as a factor-two increase between the pre-inner-core period (age greater than about 1 Ga) and the recent period. The latter model has the advantage of giving rather small variations in the heat flow across the core mantle boundary as well as a reasonable initial temperature in the deep Earth.

Acknowledgements

This paper was written when S.L. was hosted and partially supported by the Mars exploration group at the Jet Propulsion Laboratory. S.L. would like to express his gratitude, in particular to D. McCleese, B. Banerdt, S. Smrekar, and C. Raymond. Reviews by D. Stevenson and J.-P. Poirier, careful reading by J.-L. Le Mouël, S. Gilder, J. Lister and P. Roberts, and discussions with E. Dormy and G. Hulot lead to many improvements of the paper. This is IPGP contribution No. 1858.

Bibliographie

[1] J. Aurnou, F. Al-Shamali, M. Heimpel, Dynamo processes in a thin shell geometry, EOS Trans. AGU 82, fall Meet. Suppl., Abstract GP31A-0176

[2] G.E. Backus Gross thermodynamics of heat engines in deep interior of earth, Proc. Natl. Acad. Sci. USA, Volume 72 (1975), pp. 1555-1558

[3] S.I. Braginsky Magnetohydrodynamics of the Earth's core, Geomagn. Aeron., Volume 4 (1964), pp. 898-916

[4] S.I. Braginsky; P.H. Roberts Equations governing convection in Earth's core and the geodynamo, Geophys. Astrophys. Fluid Dynam., Volume 79 (1995), pp. 1-97

[5] B.A. Buffett; H.E. Huppert; J.R. Lister; A.W. Woods Analytical model for solidification of the Earth's core, Nature, Volume 356 (1992), pp. 329-331

[6] B.A. Buffett; H.E. Huppert; J.R. Lister; A.W. Woods On the thermal evolution of the Earth's core, J. Geophys. Res., Volume 101 (1996), pp. 7989-8006

[7] E. Dormy; J.-P. Valet; V. Courtillot Numerical models of the geodynamo and observational constraints, Geochem. Geophys. Geosyst., Volume 1 (2000) (paper 2000GC000 062)

[8] A.M. Dziewonski; D.L. Anderson Preliminary reference Earth model, Phys. Earth Planet. Inter., Volume 25 (1981), pp. 297-356

[9] C.K. Gessmann; B.J. Wood Potassium in the Earth's core?, Earth Planet. Sci. Lett., Volume 200 (2002), pp. 63-78

[10] G.A. Glatzmaier; P.H. Roberts A three-dimensional self-consistent computer simulation of a geomagnetic field reversal, Nature, Volume 377 (1995), pp. 203-209

[11] G.A. Glatzmaier; P.H. Roberts The geodynamo, past, present and future, Geophys. Astrophys. Fluid Dynam., Volume 94 (2001), pp. 47-84

[12] D. Gubbins Energetics of the Earth's core, J. Geophys., Volume 43 (1977), pp. 453-464

[13] D. Gubbins The distinction between geomagnetic excursions and reversals, Geophys. J. Inter., Volume 137 (1999), p. F1-F3

[14] D. Gubbins, D. Alfè, G. Masters, D. Price, M.J. Gillan, Can the Earth's dynamo run on heat alone?, Geophys. J. Int. (submitted)

[15] D. Gubbins, D. Alfè, G. Masters, D. Price, M.J. Gillan, Gross thermodynamics of 2-component core convection, Geophys. J. Int. (submitted)

[16] D. Gubbins; T.G. Masters; J.A. Jacobs Thermal evolution of the Earth's core, Geophys. J. R. Astron. Soc., Volume 59 (1979), pp. 57-99

[17] C.J. Hale Palaeomagnetic data suggest link between the Archean–Proterozoic boundary and inner-core nucleation, Nature, Volume 329 (1987), pp. 233-237

[18] C.J. Hale; D.J. Dunlop Evidence for an Early Archean geomagnetic-field: a paleomagnetic study of the Komati formation, Barberton greenstone-belt, South-Africa, Geophys. Res. Lett., Volume 11 (1984), pp. 97-100

[19] J.M. Hewitt; D.P. McKenzie; N.O. Weiss Dissipative heating in convective flows, J. Fluid Mech., Volume 68 (1975), pp. 721-738

[20] R. Hollerbach; C.A. Jones Influence of the Earth's inner-core on geomagnetic fluctuations and reversals, Nature, Volume 365 (1993), pp. 541-543

[21] G. Hulot; C. Eymin; B. Langlais; M. Mandea; N. Olsen Small-scale structure of the geodynamo inferred from Oersted and Magsat satellite data, Nature, Volume 416 (2002), pp. 620-623

[22] J.A. Jacobs The Earth's inner core, Nature, Volume 172 (1953), pp. 297-298

[23] D. Jault Sur l'inhibition de la régénération du champ magnétique dans certains modèles de dynamo planétaire en présence d'une graine solide, C. R. Acad. Sci. Paris, Ser. IIa, Volume 323 (1996), pp. 451-458

[24] M.T. Juarez; L. Tauxe The intensity of the time-averaged geomagnetic field: the last 5 Myr, Earth Planet. Sci. Lett., Volume 175 (2000), pp. 169-180

[25] R. Krishnamurti Finite-amplitude convection with changing mean temperature. Part 1. Theory, J. Fluid Mech., Volume 33 (1968), pp. 445-455

[26] C. Kutzner; U.R. Christensen From stable dipolar towards reversing numerical dynamos, Phys. Earth Planet. Inter., Volume 131 (2002), pp. 29-45

[27] S. Labrosse Hot spots, mantle plumes and core heat loss, Earth Planet. Sci. Lett., Volume 199 (2002), pp. 147-156

[28] S. Labrosse; J.-P. Poirier; J.-L. Le Mouël On cooling of the Earth's core, Phys. Earth Planet. Inter., Volume 99 (1997), pp. 1-17

[29] S. Labrosse; J.-P. Poirier; J.-L. Le Mouël The age of the inner core, Earth Planet. Sci. Lett., Volume 190 (2001), pp. 111-123

[30] J.R. Lister; B.A. Buffett The strength and efficiency of the thermal and compositional convection in the geodynamo, Phys. Earth Planet. Inter., Volume 91 (1995), pp. 17-30

[31] M. Macouin, J.-P. Valet, J. Besse, K. Buchan, R. Ersnt, M. LeGoff, U. Scharer, Palaeointensity determination from Proterozoic dykes swarms, Superior province, Canada, Earth Planet. Sci. Lett. (submitted)

[32] M.W. McElhinny; W.E. Senanayake Paleomagnetic evidence for the existence of the geomagnetic field 3.5 Ga ago, J. Geophys. Res., Volume 85 (1980), pp. 3523-3528

[33] C. Morimoto; Y. Otofuji; M. Miki; H. Tanaka; T. Itaya Preliminary palaeomagnetic results of an Archaean dolerite dyke of west Greenland: Geomagnetic field intensity at 2.8 Ga, Geophys. J. Int., Volume 128 (1997), pp. 585-593

[34] G. Morrison; D.R. Fearn The influence of Rayleigh number, azimuthal wavenumber and inner core radius on 2–1/2D hydromagnetic dynamos, Phys. Earth Planet. Inter., Volume 117 (2000), pp. 237-258

[35] E. Ohtani; H. Yurimoto; S. Seto Element partitioning between metallic liquid, silicate liquid, and lower mantl'e minerals: implications for core formation of the Earth, Phys. Earth Planet. Inter., Volume 100 (1997), pp. 97-114

[36] M. Perrin; E. Schnepp; V. Shcherbakov Paleointensity database updated, EOS, Volume 79 (1998), p. 198

[37] M. Perrin; V. Shcherbakov Paleointensity of the Earth's magnetic field for the past 400 Ma: evidence for a dipole structure during the Mesozoic low, J. Geomagn. Geoelectr., Volume 49 (1997), pp. 601-614

[38] L.J. Pesonen; H.C. Halls Geomagnetic-field intensity and reversal asymmetry in Late Precambrian Keweenawan rocks, Geophys. J. R. Astron. Soc., Volume 73 (1983), pp. 241-270

[39] J.-P. Poirier Transport properties of liquid metals and viscosity of the Earth's core, Phys. Earth Planet. Inter., Volume 92 (1988), pp. 99-105

[40] J.-P. Poirier Light elements in the Earth's core: a critical review, Phys. Earth Planet. Inter., Volume 85 (1994), pp. 319-337

[41] M. Prévot; M. Perrin Intensity of the Earth's magnetic field since Precambrian from Thellier-type paleointensity data and inferences on the thermal history of the core, Geophys. J. Int., Volume 108 (1992), pp. 613-620

[42] P.H. Roberts; C.A. Jones; A.R. Calderwood Energy fluxes and ohmic dissipation in the Earth's core (C.A. Jones; A.M. Soward; K. Zhang, eds.), Earth's Core and Lower Mantle, Taylor & Francis, London, 2003

[43] A. Sakuraba; M. Kono Effect of the inner core on the numerical solution of the magnetohydrodynamic dynamo, Phys. Earth Planet. Inter., Volume 111 (1999), pp. 105-121

[44] P.A. Selkin; J.S. Gee; L. Tauxe; W.P. Meurer; A.J. Newell The effect of remanence anisotropy on paleointensity estimates: a case study from the Archean Stillwater complex, Earth Planet. Sci. Lett., Volume 183 (2000), pp. 403-416

[45] P.A. Selkin; L. Tauxe Long-term variations in palaeointensity, Phil. Trans. R. Soc. Lond. A, Volume 358 (2000), pp. 1065-1088

[46] M.St. Pierre The strong field branch of the Childress–Soward dynamo (M.R.E. Proctor; P.C. Matthews; A.M. Rucklidge, eds.), Solar and Planetary Dynamos, Vol. 1, NATO Advanced Study Intitute, Cambridge University Press, Cambridge, UK, 1993, pp. 295-302

[47] F.D. Stacey; C.H.B. Stacey Gravitational energy of core evolution: implications for thermal history and geodynamo power, Phys. Earth Planet. Inter., Volume 110 (1999), pp. 83-93

[48] D. Stevenson; T. Spohn; G. Schubert Magnetism and thermal evolution of the terrestrial planets, Icarus, Volume 54 (1983), pp. 466-489

[49] I. Sumita; T. Hatakeyama; A. Yoshihara; Y. Hamano Palaeomagnetism of the Late Archean rocks of Hamersley basin, Western Australia and the paleointensity at Early Proterozoic, Phys. Earth Planet. Inter., Volume 128 (2001), pp. 223-241

[50] H. Tanaka; M. Kono; H. Uchimura Some global features of paleointensity in geological time, Geophys. J. Int., Volume 120 (1995), pp. 97-102

[51] E. Thellier; O. Thellier Sur l'intensité du champ magnétique terrestre dans le passé historique et géologique, Ann. Geophys., Volume 15 (1959), pp. 285-378

[52] J. Verhoogen Heat balance of the Earth's core, Geophys. J. R. Astron. Soc., Volume 4 (1960), pp. 276-281

[53] J. Wicht Inner-core conductivity in numerical dynamo simulations, Phys. Earth Planet. Inter., Volume 132 (2002), pp. 281-302

[54] A. Yoshihara; Y. Hamano Intensity of the Earth's magnetic field in Late Archean obtained from diabase dikes of the Slave Province, Canada, Phys. Earth Planet. Inter., Volume 117 (2000), pp. 295-307

[55] Y.J. Yu; D.J. Dunlop Paleointensity determination on the Late Precambrian Tudor gabbro, Ontario, J. Geophys. Res., Volume 106 (2001), pp. 26331-26343

Commentaires - Politique