1 Introduction
The solar system formed 4.566 billion years ago [2] from the collapse of a dense molecular cloud. Its history is full of water, from the initial collapse of the parent molecular cloud to the sweeping out of the last remains of the solar nebula by the solar wind of the young Sun, after . A molecular cloud of one solar mass would contain about a million times the water mass of the oceans, but the D/H ratio of that water is much greater than that of the terrestrial oceans (e.g. [16,57,65]). During the collapse, heating and evolution of the solar nebula, a similar amount of water is likely to form from the reaction of the overwhelmingly abundant hydrogen and out of equilibrium carbon monoxide, but the D/H ratio of this hydrogen is very small [20] and the isotope fractionation factor , between hydrogen and the newly formed water, is probably too small at the reaction temperatures to arrive at the ratio of the oceans. Later on (about a hundred thousand years) (e.g., [7]), the young Sun burns its small proportion of deuterium into helium-3, clears the inner solar nebula of its last remains with a strong wind of that deuterium-free hydrogen, while that space is heated to temperatures where carbon monoxide becomes again dominant over water. There is no more water.
The remaining water has been stored before in the cores of giant planets [17,38] or removed to the outer spaces of the inner and outer Oort clouds, from where most of the comets come, or in the closer Kuiper belt beyond Neptune. The D/H ratio of the comets' water, about 50% in mass, is closer to the oceans' ratio than the original interstellar water molecules, but still two times larger (e.g., [19]).
Most of the clues about the origin of the Earth's water are isotopic. They come not only from the isotopes of hydrogen for the identification of water sources, but also, indirectly, from oxygen isotopes for the identification of the solid Earth's material, and, more generally from the so-called stable isotopes (more precisely stable and non radiogenic) of carbon, nitrogen and other light elements [28–31]. The abundance of some radiogenic isotopes (e.g., 53Cr, 182W, 187Os, etc.) provide constraints in identifying the origin of terrestrial materials, for example, the origin of that ‘late veneer’, present in virtually every Earth model, small in mass but probably full of water (e.g., [4,25,36]).
The formation of the Earth's core is very reducing and the presence of water during that period is not favourable for such conditions. Experiments seem to allow the presence of some water during the core formation [51], but the chondritic remains of the nebular story show no such presence of strongly reduced, yet water-bearing material (e.g., [31,62]). Another possibility is that the solid Earth at this time contained hydrogen-bearing compounds that were later oxidized into water, providing an internal source for part of the oceans and mantle water.
The Earth represents about three millionths of the solar system mass. Its water represents a similar proportion of the water that now and then was part of the solar nebula. How and when has the encounter of the two occurred in the solar system?
2 Brief chronology
From the initial collapse of the parent molecular cloud, 4.566 billion years ago [2] to the end of significant water uptake by young planet Earth, about 100 million years have elapsed. Here are the major events of that period.
2.1 The Sun
It takes a maximum of 20 million years [7] for the Sun to grow to its full size, start its first nuclear reaction (deuterium burning to give helium-3), blow the residual solar nebula by the so-called T-Tauri solar wind (possibly preceded by violent Fu Orionis outbursts), and finally settle on the so-called main sequence of the Hertzprung–Russell diagram. This corresponds to the present state of hydrogen burning, which was the Sun's fate for ∼4.5 billion years and will also be its fate for about 5 more billion years to go. However, its growth to full size, during which it probably accretes an important fraction of the original water, is much quicker, five to six million years at most, and the start of the deuterium burning occurs after only ∼0.1 million years when the size of the Sun is already 30 to 50% of its present mass. The really important period for our concern stops with the T-Tauri wind, after 6 to 10 million years. If the T-Tauri wind contributes to the Earth's water budget (as hydrogen first) as it does for the rare gases (solar noble gases of the deep Earth's mantle), then this part of the water budget was devoid of deuterium.
2.2 The nebula
It very rapidly (0.1 million years) evolves to the shape given in Fig. 1, that of a disk of about 30-AU diameter and 0.2-AU thickness, fed by the collapsing cloud [17]. Very rapidly also, significant matter ejection occurs. In the heated nebula, the water-to-carbon-monoxide ratio varies, strongly increasing in the medium temperature range (300–1000 K) and decreasing again to low values above 1000 K. These temperature conditions vary with time, increase, peak, then decrease again, and with the position at a given time: the regions distant from the Sun and, at a given distance, from the median plane are colder and richer in water. In the stirred nebula, the incoming deuterium-rich water is going to mix and exchange with the newly formed, deuterium-poor, water, and exchange also with the major molecular species, hydrogen, very poor in deuterium. Most of these exchanges occur during the first 5 million years. Although difficult to model, these exchanges have been described rather convincingly by Moussis et al. [46]. Their main success is to explain rather well the intermediate D/H ratios found in the few comets isotopically studied to date (Halley, Hale-Bopp) as the result of exchange between the original interstellar water and the nebular hydrogen. After about 10 million years (end of Jupiter's accretion, continuing accretion of the other giant planets and maximum of the T-Tauri wind), most of the water fate is settled in the nebula. A large portion has led, as water ice mixed with dust, to the formation of the cores of giant planets, followed by runaway accretion of gas around them. The rest has gone much further, to the inner and outer Oort clouds, and a little to the nearer reaches of the Kuiper belt [47]. In summary, at 10 million years, the cometary water source is, at least isotopically, individualized at . A large fraction is trapped forever in the cores of the giant planets, and the inner nebula is completely devoid of water and, probably, of any other gas. These are good conditions for forming the iron–nickel cores of the inner planets, because they need strongly reducing conditions, hence no water. However deuterium-devoid hydrogen may have been implanted in the inner planet-forming material.
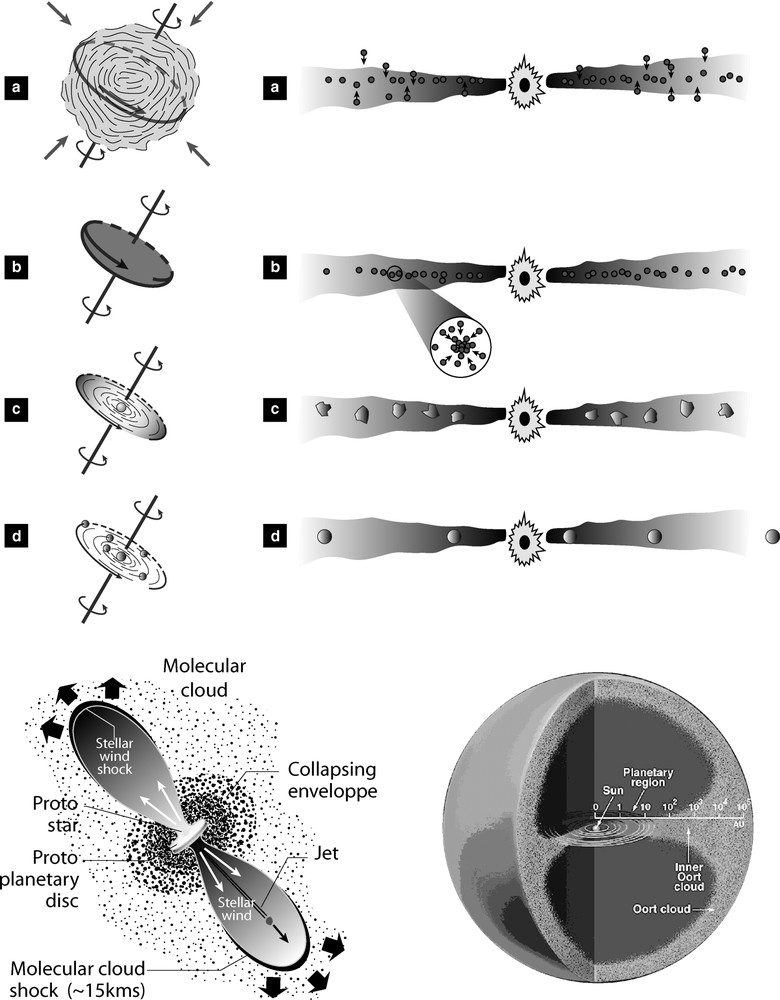
Artist's views of the solar nebula evolution. Top left: collapse of molecular cloud and angular momentum conservation. Top right: progressive accretion of planetesimals and planets. Bottom left: more elaborated model of in and out flows. Note the proposed directions of flows relative to the protoplanetary disk. Bottom right: sketch of the cometary material concentration. Inner and outer Oort clouds. The Kuiper belt, not indicated, lies at the outer edge of the planetary region. Note also that the distance scale is logarithmic.
Quelques aspects de l'évolution de la nébuleuse solaire. En haut à gauche : la conservation du moment angulaire au cours de l'effondrement du nuage moléculaire. En haut à droite : accrétion progressive des planétésimales et des planètes. En bas à gauche : une idée plus précise des flux entrants et sortants. Noter la direction des flux par rapport au disque protoplanétaire. En bas à droite : schéma des concentrations de matériel cométaire, nuages de Oort interne et externe. La ceinture de Kuiper, non indiquée, se situe à la lisière de la région planétaire. Noter également que l'échelle des distances est logarithmique.
2.3 The inner planets
Models of inner planets formation by collisions among planetesimal populations describe a growth corresponding to time constants of 10 to 12 million years, which means that all the inner planets would be completed up to more than 95% within a time span of 30 million years maximum.
This is in particular the case for the Earth [54,64].
However, for our planet, two more events have to be considered.
The first one is the so-called great impact. Most planetologists now consider it absolutely necessary to explain the very large angular momentum-to-mass ratio of the Earth–Moon system with respect to the other planets. This is explained by the late encounter of a completed Earth with a Mars-sized object that provides the remaining 15%. This impact puts the Earth to shambles, largely melts it, starts the last episode of the core differentiation, gives birth to the Moon, and, on top of that, provides the right angular momentum [5]! The second one, more dispersed in time, is the so-called late veneer, which most earth modellers invoke to explain the present mantle concentration of siderophile elements. This late veneer, as we shall see, plays also a very important role for the Earth's water. The great impact is likely to have occurred at million years, but more precise and objective estimates can be given by radiochronology (e.g., [36]). The majority of the late veneer was probably accreted during the following 100 million years.
3 Radiochronology and the meteorite record
The oldest and most primitive (not processed or differentiated in planetary objects) remains of the early solar system history available on Earth are the meteorites called chondrites: they contain chondrules (spherical grains resulting most probably from fusion phenomena), imbedded in a more or less fine-grained matrix formed at lower temperature. The strong disequilibrium between matrix and chondrules, or even inside matrix and chondrules, are the proof of their primitive character [18,41,62,63].
Another proof is the very restricted range (a few million years) of very old ages (circa 4.5 billion years) given by the long period radiochronometers (RbSr, KAr, UThPb, SmNd, etc.) [40,43,44,61].
The chondrites are classified in nine major groups (CI, CM, CV, CO, H, L, LL, EH, and EL) of very variable oxidation states (Fig. 2), from the very oxidized carbonaceous chondrites (CI, CM, CV, CO), to the intermediate state of ordinary chondrites, the most numerous groups (H, L, LL), and to the extremely reduced enstatite chondrites (EH and EL). The EH group matches almost exactly the Earth both in nickel–iron content and in oxidation state, and it is the only one [18,28,31,41,63].

Urey–Craig diagram [59]. This diagram classifies the main chondrite groups according to their total iron contents (isoconcentrations parallel to the second diagonal) and the reduced/oxidized ratio of this iron. Note that, for total iron content and oxidation state, only one group, the EH, fits with the Earth's characteristics.
Diagramme de Urey–Craig des chondrites [59]. Ce diagramme classe les principaux groupes de chondrites suivant leur concentration globale en fer (droites d'isoconcentrations parallèles à la seconde bissectrice) et le rapport entre fer réduit (métal plus sulfures) et oxydé (oxydes plus silicates). Un seul groupe (EH) correspond aux caractéristiques de la Terre.
The oldest object found in the solar system, a refractory inclusion in the carbonaceous chondrite Allende, has been dated very precisely at 4.566 billion years [2].
To date precisely the small age intervals between the chondrite groups with respect to the oldest Allende inclusion, cosmochemists rely more and more on short-period ‘extinct radioactivity’ radiochronometers (129I–129Xe, million years, 53Mn–53Cr, million years, 182Hf–182W, million years, and so on) able to date events of 4.5 billion years ago with a precision better than one million years. These are tricky techniques, but they become rapidly more and more reliable. They allow the following record of the nine main groups of chondrites classified in Fig. 2: seven have been formed in a time span of less than 7 million years since the beginning of the solar system formation at 4.566 billion years; thus they would have a minimum age of 4.559 million years. These are the most oxidized (CI, CM, CV, CO, H, L, LL), and some of them (CI) contain up to 10% water.
The remaining two groups have been formed significantly later (about million years, hence with an age of 4.557 billion years [56,60]) and their minerals record also minor events from a little before 9 million years up to 40 million years. They contain small amounts of hydrogen that could, if oxidized, form amounts of water corresponding to about half of the Earth's present water content.
In the asteroid belt, that region extending from 2 to 4 AU between Mars and Jupiter, the similarities in reflectance properties (albedo) between the asteroids and the various chondrite families are used to figure out that the most oxidized and water-rich chondrites, the carbonaceous group, come from the outer part of the belt, the EH and EL from the inner border of the belt, with the ordinary chondrites (H, L, LL) in-between. This simple, probably oversimplified, image relates the oxidation state and water abundance to the distance to the Sun, with the water-rich and oxidized meteorites as remains of the outer part of the nebula and the enstatite chondrites representing the conditions of the inner solar system, where the terrestrial planets now lie [6].
4 Stable isotope tracers
The chondrite classification is the occasion to introduce the stable isotope tracers. The isotope ratios of light elements (D/H, 13C/12C, 15N/14N, 18O/16O, 17O/16O, etc., up to sulphur and chlorine isotopes) vary in a given body according to simple rules, briefly recalled below [27,50].
Anyone isotope ratio hereafter called R (D/H, 13C/12C, etc.) fractionates between different molecules, minerals, physical states of a given compound, A and B, according to:
These fractionations are called mass dependent. When an element has more than two isotopes, such as oxygen or sulphur, the fractionation is proportional to the mass difference. For example: .
Hence, in a – diagram, the samples from a given body (for example, the Earth) will plot along a straight line of slope 0.5 (called the terrestrial line in the case of the Earth [8,10].
Finally the Rs or δs obeys mass balance equations such as , where the s are the molar fractions of the different parts of a system.
An interesting example of the mass balance equations regarding the water problem is the following: if water and minerals (or rocks, meteorites) exchange oxygen isotopes in a closed system, then, at the equilibrium, , and similarly with 17O. This means that the most abundant the water in the meteorite–water system, the most remote the will be from the initial composition of the system. Similarly, for water–hydrogen exchange of deuterium, we would have . In this latter case, since hydrogen is overabundant, is close to 1 and this reduces to .
Hence the oxygen or hydrogen isotope composition can give clues to the solid-to-water ratio at the time of formation of a given system.
5 The chondrite oxygen isotope classification
In Fig. 3a and b from Refs. [8–10,66,67] the groups of Fig. 2 have been plotted as a function of their oxygen isotope composition1 and one remarks immediately the same groupings as in Fig. 2.
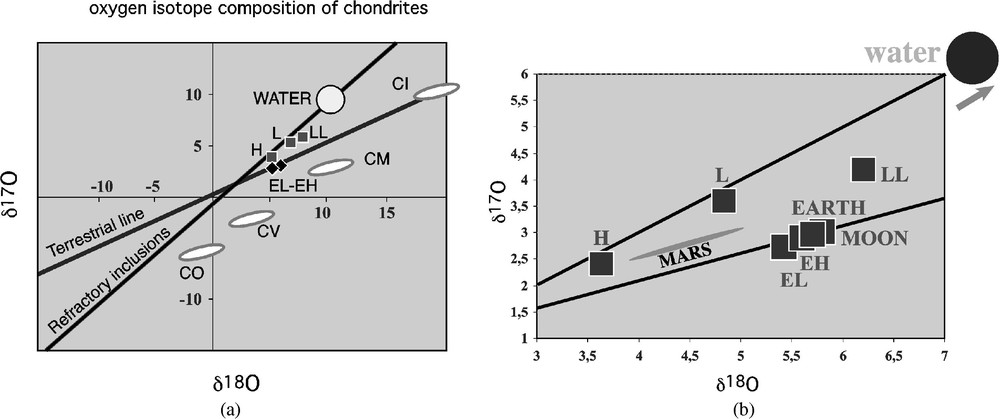
(a) 17O–18O diagram of chondrite groups. The slope 1 straight line (equation ) is defined by unaltered refractory inclusions of CV type chondrites (‘Allende inclusions’). These objects date the beginning of the solar system (t=0, 4.568 billion years ago). The original nebular water lies at the top of that line. The ∼0.5-slope line is the terrestrial line , where all terrestrial samples lie. The bulk composition of the main chondrite groups defines segments of slope ∼0.5, parallel to the terrestrial line. All chondrite groups, except EH–EL, correspond to ages t between 3 and 7 Myr after the Allende inclusions. Note also that the distance of the chondrite groups to the Allende inclusions' line is directly correlated to their water content. The 17O–18O diagram is the first image of the solids–water relationship in the solar nebula. (b) Detail of Fig. 3a: even if H, L, and LL groups lie close to the Allende line, they are not on it. Note the exact coincidence (the points have been separated only for the sake of clarity) of EH, EL, the Earth's mantle and the Moon. The Mars composition, deduced from SNC meteorites, appears as a possible mixture of EH–EL and H–L material [55].
(a) Diagramme 17O–18O des chondrites. La droite de pente 1 (d'équation ) est définie par les inclusions réfractaires des chondrites CV (inclusions d'Allende). Ces objets datent la naissance du système solaire (t=0, il y a 4,568 Ma). La composition de l'eau nébulaire primitive est au sommet de cette ligne. La droite de pente 1/2 (en fait ∼0,52) est la droite terrestre sur laquelle on trouve la composition de n'importe quel échantillon terrestre. La composition globale des principaux groupes de chondrites définit des segments de pente ∼0,5 parallèles à la droite terrestre. Tous les groupes de chondrites sauf EH–EL correspondent à des âges de 3 à 7 millions d'années après les inclusions d'Allende. Notez que la distance des groupes de chondrites à la droite d'Allende est directement liée à leur contenu en eau (voir § Stable isotope tracers). Le diagramme 17O–18O est la première image des relations solides–eau dans la nébuleuse primitive. (b) Détail de la Fig. 3a : même si les groupes H, L, et LL sont proches de la droite d'Allende, ils ne sont pas exactement dessus. Notez la coïncidence presque parfaite de la Terre, de la Lune et des chondrites EH et EL (les points ont été légèrement disjoints uniquement pour distinguer les différents objets). La composition de Mars, déduite des météorites SNC, apparaît comme un mélange possible entre EH, H et L [55].
The meteorite groups plot as straight line segments parallel to one another and to the terrestrial line, with a limit slope of ∼0.5. However, while the ordinate at the origin of the terrestrial line is zero, meaning that the Earth contains the reference standard, i.e. the oceans, the ordinates at the origin of the various chondrite lines are displaced relative to one another. This fundamental scheme, discovered by Clayton et al. in 1973 [9], means that the different bodies had not received the same initial share of 16O when they first formed. This is the trace of a major event responsible for the initial collapse of the nebula, the explosion of a nearby supernova**,2 whose effects show up in many other isotope compositions, particularly, but not only, in the extinct radioactive systems (MnCr, IXe, HfW), which now help dating these events.
The initial oxygen heterogeneity, generated by the injection of pure 16O by the supernova, appears unmodified by the later events in the straight line of slope 1 at the left of the diagram [66,67]. It corresponds to time . The initial oxygen isotope composition of the cloud lies on top of that line, including that of the original water, and the place of the samples on the line of slope 1 reflects the various proportions of pure 16O received at . Inclusions of refractory minerals in CV, CO and CM, and some chondrules of ordinary chondrites, have kept intact the memory of the initial event on the line. They plot down to to 45‰, reflecting a maximum proportion of pure 16O of up to 5%.
After that initial event, most of the chondrite-group material has exchanged oxygen isotopes with the surrounding water. If we apply the simple mass-balance equation given above, , we see that the most remote the chondrite groups are from the initial line, the richer their environment was in water (CO should also possibly be considered for these exchange equations, since the original repartition of oxygen in the cloud is approximately in three comparable parts, solids, water and CO; however, CO is much more reluctant to exchange than water). Indeed, the chondrite group by far the richest in water is the CI group, followed by the CM group, then the CV, and CO groups.
The closed-system equation is too crude to reflect exactly what happened during these first few million years of the evolution, but it shows that we have a first clue to estimate the water impact on the different chondrite groups.
What for the isotopic coincidences in this diagram? Given the complexity of the evolution described above, they have no chance to be accidental. For example, the coincidence between the Earth's mantle, the Moon, the EH and EL groups at and has about one chance in 30 millions to result from a random coincidence. Similarly, the small positive displacement of the Martian line (measured in SNC meteorites) is the trace of a slight deficit in the supernova component of Mars relative to the Earth.
Hence oxygen isotopes help find the identity of the primitive, undifferentiated, bulk Earth composition: it is given by EH meteorites (EL represent early differentiates of the EH composition, having already lost part of their iron).
This identity reflects in the isotope composition of all elements studied so far (oxygen, nitrogen, copper, zinc, molybdenum, chromium, etc.) [14,28,33,34,39,56]: all meteorite groups present significant, whether big or small, differences (called isotopic anomalies) relative to the Earth mantle, except the EH–EL group (as well as a related differentiated meteorites group, the aubrites or enstatite achondrites).
What about water in the EH chondrites? They are more distant from the slope-1 line than, for example, the ordinary chondrites. Hence they should have resided in a reasonably water-rich environment. Yet they contain no water, and water would have probably oxidized to some extent the metallic iron they contain, which is absolutely not the case. But they contain a significant proportion of deuterium-poor hydrogen [15,28,65], which points out to hydrogen interaction. We have also to remember that they are younger by about 5 million years than the other groups, and that their age coincides with the estimate of the T-Tauri period. Their mineral paragenesis indicates an equilibrium temperature around 800 °C.
To summarize, the EH chondrites represent the bulk Earth material. This material was cooked in the very reducing environment of the T-Tauri solar wind. A consequence of that treatment is that it contained low deuterium hydrogen. If that hydrogen survived at least partly the high temperature phenomena of the Earth's accretion (particularly the Great Impact), it may have provided up to half of the Earth's present share of water.
6 Bulk Earth material and its evolution
It is given by the average composition of EH chondrites [18,41,63]. The silicate fraction of that material has a composition rather different from that of the present Earth's upper mantle, richer in silicon, poorer in magnesium, calcium, and aluminium. This implies a marked chemical contrast between upper and lower mantle, acquired during the differentiation of the core by the uptake of silicon and after the Great Impact by the formation of a magma ocean roughly half in mass of the total mantle. This leads, from the beginning, to the building of a stable two-levels mantle convecting system. This agrees with all geochemical studies showing the clear-cut distinction between a depleted and outgassed upper mantle and a lower mantle providing only small fluxes to the surface and rich in primitive characteristics (e.g., solar noble gases) [11,45]. This means that the lower mantle has had, up to now, limited connexions to the surface. Hence the mass balance between the water input due to the bulk Earth (before the late veneer, see above and below) and the water contribution of the late veneer has to consider only the upper mantle. However that ‘dynamic’ upper mantle is not limited to the part above 650 km. It represents the whole upper half, above the depth of 1000–1100 km [32].
An essential character of the EH material is its strong reducing power. The total absence of oxidized iron and, even more, the presence of elemental silicon dissolved in the FeNi metal, correspond to a unique buffering power of oxygen fugacity. Only such a composition is able to achieve the dissolution of up to 7–8% more silicon into the metal to give the Earth's core its present density.
This occurred through the critical reaction:
This reaction, which takes place in the temperature–pressure range 1800–2200 °C, 10–25 GPa [21,23,28], is the only realistic way to make silicon enter the core. That dissolution has started, as we saw above [18] at the nebular stage but in a very different low-pressure mode, and continues during the Earth's accretion as the building blocks attain a Moon size (∼1.5% of the Earth's one). The completion of this process is performed during and after the Great Impact that gave birth to the Moon and put the final touch to the core formation ( million years).
In order to make the mantle as we see it now, with approximately 6% of oxidized iron (Fe II) in the upper mantle and probably up to 10% in the lower mantle, we have to oxidize part of the metallic iron. Finally, in order to explain the present density contrast between the liquid core and the solid core, we have to put about 3% oxygen in the core (silicon alone would not do the job). The above reaction will satisfy all these requirements by dissolving silicon into the sinking metal and transferring the dense iron oxide through the mantle. It will partly react with the surrounding mantle silicates and partly continue its track down to the lower mantle, then to the core. In the present state of the Earth, the amount of oxidized iron is distributed quasi evenly between the upper mantle, the lower mantle and the core. The dating of the Great Impact is possible through a fitted extinct radioactivity chronometer, the 182Hf–182W couple (period 9.7 million years). Tungsten is strongly siderophile and hafnium strongly lithophile. The differentiation of the core produces a mother–daughter separation of the two elements, which can be dated. The present estimate places the core differentiation at million years after the initial solar cloud collapse (e.g., [36]).
The formation of the core implies little or no water. This is precisely the case in the EH material. The few reliable analyses by classical chemistry or by ion probe point out to hydrogen not necessarily incorporated in water molecules. This hydrogen may be linked to solid carbon compounds (a sort of hydrogen-poor kerogen), which would make it more resistant to outgassing than water or hydrated minerals. It has a striking isotope signature (a D/H ratio lower than half the oceans D/H ratio and values possibly down to zero, a δD between −500 and ), coherent with the T-Tauri wind episode [15,65]. If oxidized, that hydrogen content would give about 450 ppm water. Because of its very low D/H ratio, this hydrogen is a key component if other tracers indicate a significant contribution of deuterium-rich comets: since their D/H ratio is about twice that of the oceans, we would then need another, deuterium-poor component to counterbalance their deuterium excess and get the right terrestrial composition. EH rare gases also tend to show solar rare gases characteristics [11].
The appearance of oxidized iron and the timing of that appearance are also critical to the problem of terrestrial water, because iron compounds are the only practical large-scale redox system in the present Earth able to oxidize or reduce important quantities of another element. So, the absence of water during the initial period of the Earth growth makes possible the retention of hydrogen under a reduced form and its subsequent appearance renders its oxidation into water and degassing possible as well.
7 The isotope signature of solar system waters
The Sun's parent cloud contained about of water and water creation during the solar nebula warming could have added an equivalent amount, a total of . Where did all that water go? The cores of the four giant planets are thought to have been formed with approximately 50:50 mixtures of rock and water ice [46]. This amounts to . The comets from the two Oort clouds and Kuiper belt can also be roughly described as 50:50 mixtures of rock and water. The estimates regarding the mass of these clouds are very uncertain. They range from 1029 to 1030 g, half of it being water. This amounts to . Terrestrial water and asteroidal water are negligible. So the reasonably identified remains of water from the nebular history represent no more than 3–4% of the maximum water content that once existed in the nebula. The rest probably made its way to the Sun itself.
On the other hand, cometary water represents about 150 000 times the mass of the Earth's oceans, distributed between 1012 and 1013 comets. If the mass of comet Halley (1017 g) is a good estimate of the average comet, the oceans could have been delivered to the Earth by about 40 million Halley-type objects. Do they have the right isotope composition? Certainly not at first sight, since their average D/H is almost exactly twice that of the Earth's oceans.
Let us now investigate more completely the D/H message (Fig. 4).
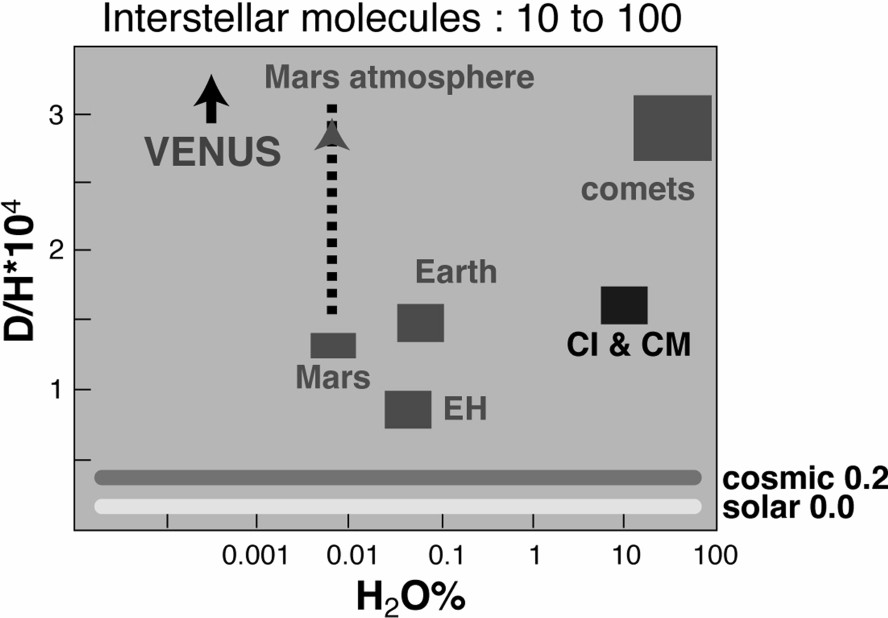
D/H ratios and water concentrations of some solar and cosmic water sources.
Rapport D/H et concentration en eau de quelques sources solaires et cosmiques.
Terrestrial water, with a D/H ratio of , is enriched by a factor 7.8 relative to the primordial hydrogen , and comets, twice as much (e.g., [52]). What about chondritic water? Very rare chondrite samples (2 or 3) display what could look like average primitive water D/H [16] from the molecular cloud. This represents an average enrichment factor of 36.5 relative to the nebular hydrogen. But even these rare samples contain another, much more ‘normal’ water component, with D/H ranging from 0.8 to [24,65].
All chondrite groups display more or less this kind of isotopic dichotomy, with two kinds of water (or hydrogen): one with low D/H (0.9 to 0.5 of the terrestrial value), the other with high D/H (generally close to cometary values). The first one is generally contained in terrestrial-like phyllosilicates (clay minerals, chlorite, etc.), whereas the high D/H ratios are contained in the so-called ‘aromatic polymers’, similar in some ways to terrestrial kerogens but specific to the chondrites [24,35,53,65].
These values represent an evolution from the initial extreme compositions, interstellar hydrogen and interstellar molecules enriched in deuterium through their synthesis by ion-molecule reactions . That evolution can be explained by the combination of two mechanisms:
- (1) an approach to chemical equilibrium during the warming of the nebula by the creation of water, according to the reaction . This new water will have a low D/H, a priori governed by the equilibrium fractionation between water and hydrogen. (D/H)hydrogen. These phenomena occur in a maximum temperature range of ∼160 to 700 K, where α can be conveniently expressed as:
- (2) an approach to isotopic equilibrium of the pre-solar, deuterium-rich, water, which exchanges with the surrounding hydrogen, with the same fractionation factors, but with lesser efficiency than in process 1, where water is created from the hydrogen with which it has to equilibrate.
This last process has been described by Moussis et al. [46]. They model the late evolution of a residual (∼0.01 to 0.1 solar masses), turbulent nebula, after the Sun has already acquired its total mass. The most interesting point of that model is that it convincingly represents the way the cometary material could have acquired its present, little variable D/H ratio.
So, apart from the EH material, which brought to the bulk Earth a share of mantle hydrogen between 0 and 50% of the present terrestrial water mass, with very low D/H (possibly down to zero), the possible solar sources of water are:
- – the comets, with a D/H roughly equal to 2(D/H)terrestrial;
- – the chondritic materials, which contain both low and high D/H ratios.
In fact, the only serious chondritic candidates are those that contain enough water to make the complement to EH hydrogen without altering the Earth's other isotope characteristics, for example, its oxygen isotope composition. Only two groups satisfy that condition, the CI and CM groups, which can bring respectively around 10 and 7% water equivalent in hydrogen. This hydrogen can be considered as a mixture of about 85% deuterium-poor hydrogen, corresponding to hydrosilicates, and 15% cometary-like hydrogen , contained in kerogen material. The isotopic balance is close to the present bulk terrestrial value .
This water complement, between 50 and 100%, is brought by the late veneer.
8 The late veneer
8.1 Its reality
That popular concept is more than an attractive hypothesis. It designs the last infall of material on the planets and especially the Earth, after its main differentiation. We can see presently the heavy traces of that infall on our satellite the Moon, on Mercury and Mars. The Moon surface is the most useful place to describe its chronology: the bulk of the infall occurred during the first 700 million years of our planet and of its satellite, and the end of that intensive phase corresponds also to the appearance of the oldest dated rocks on Earth. However, it is still continuing today and, to take an example, the meteorite that created the Chixculub crater ∼65 million years ago (and is one of the possible causes of the Dinosaurs' dismay) belongs to the ‘tail’ of that veneer. Was that veneer big? Yes and no. It constitutes about 0.2 to 0.3% of the Earth's mass, which is relatively small. However, these represent about 10 million objects of the size of the Chixculub object! So it is understandable that it has erased all traces of terrestrial events during its main period (about 700 million years), the inferno of the ‘Hadean’ period. But the period during which it was really heavy is probably much shorter. The infall repartition is strongly concentrated toward the beginning. It is estimated that 80 to 90% of the load occurred during the first 100 million years. By ‘really heavy’, I mean a bombardment which gave also the Moon craters and was able to melt large portions of the Earth's surface and to incorporate rapidly and deeply into the mantle its most conspicuous products, such as the strongly siderophile elements.
8.2 Its mean age
The early history of the Earth is marked by events that are impossible to date separately, because they have been erased immediately by new ones [2]. However, some of these events, taken together, can build an integrated signal whose mean age can be evaluated. Long-period chronometers (UTh Pb mainly) describe the incorporation of new products into the early mantle and the extraction of others: the evaluation they give is about 100 million years after the initial event, that is, until about 4.45 billion years before present. This matches with the deciphering of the Moon craters system. So the greatest part of the addition of new material covers the first 100 million years. This includes the addition of water, and/or water precursors.
8.3 Its composition
The first use of the veneer was to explain the presence in the mantle of elements so strongly siderophile (e.g., the platinum group elements Os, Ir, Pt or gold, palladium, rhenium, etc.), with partition coefficients of 103 to 105 in favour of the liquid metal. These elements should thus be completely absent of the mantle, but they are not. They exist at very small concentrations, in ‘chondritic’ proportions. The simplest explanation is that they were brought by the late veneer. Since they are added to a siderophile-free mantle, the present mantle concentrations represent simply a dilution of the chondritic concentrations, which gives a straightforward evaluation of the mass of veneer necessary: mass of veneer/mass of mantle = mantle concentration/chondrite concentration. For this, all chondrites have similar concentrations and the result is: mass of veneer=∼0.8 to 1% of the upper mantle [42].
The second use is related to the nitrogen isotope composition (Fig. 5). Sediments are 2–3‰ richer in 15N than the atmospheric nitrogen (which is the international standard). So the global surface nitrogen is richer in 15N than the atmosphere. Mantle nitrogen, as analysed in diamonds or basalts, is significantly poorer in 15N than the atmosphere ( in average and down to in some diamonds), and the isotopic fractionations occurring during mantle outgassing of nitrogen go in the wrong direction (they deplete further the outgassed nitrogen in 15N). So the terrestrial nitrogen accretion is necessarily heterogeneous: to the 15N-depleted nitrogen of the EH source, we must add a veneer of 15N-enriched nitrogen [31,33,34]. There are at least three candidates: the CI and CM meteorites, and the comets. All three contain large water concentrations.
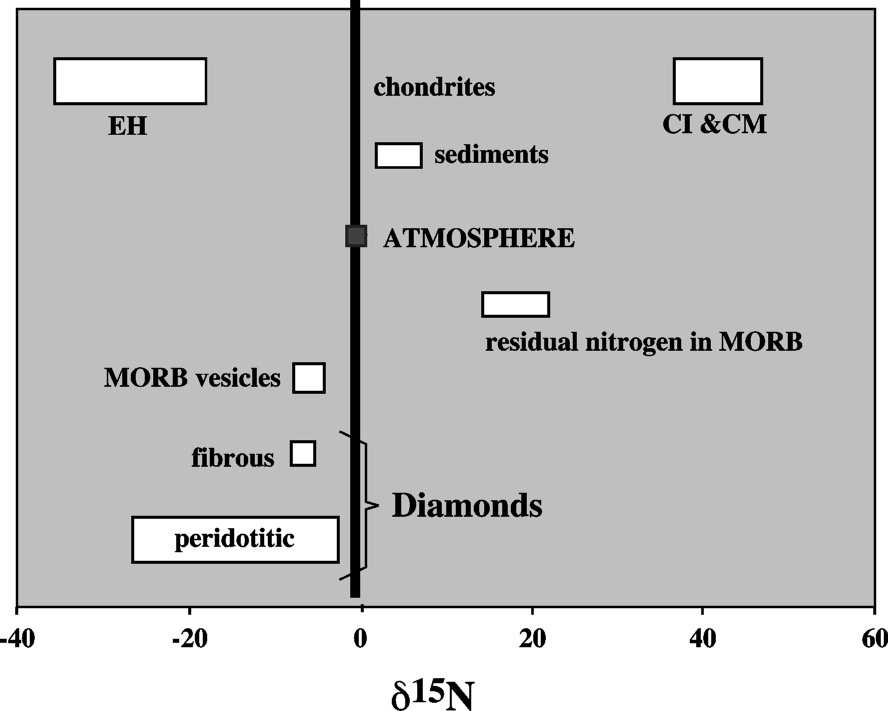
Nitrogen isotope composition of surface and mantle nitrogen compared to the Bulk Earth's (EH) and possible late veneer (CI and CM) sources. The fact that present surface nitrogen and residual nitrogen resulting from mantle outgassing are both richer in 15N than mantle nitrogen implies that nitrogen accretion has been heterogeneous, with the late surface addition richer in 15N.
Composition isotopique de l'azote terrestre superficiel et mantellique, comparée à celle de la Terre globale (EH) et de sources possibles du vernis tardif (CI et CM). Le fait que l'azote superficiel actuel et l'azote résiduel résultant du dégazage du manteau (dans les basaltes) soient tous deux plus riches en 15N que l'azote mantellique implique l'addition superficielle d'un vernis tardif plus riche en 15N (CI, CM, ou comètes ?) que celui du manteau primitif.
So we come to the third use of the late veneer, the most important for our present purpose. What is the real source of water?
Again the use of isotope indicators will be crucial.
9 The veneer isotope signature
Let us summarize: the EH material does not show any isotope anomaly relative to the Earth, whatever the element. We saw just above the nitrogen isotope condition for which we have three veneer candidates, CI, CM, and the comets. For hydrogen, we have two candidates that could each alone make 100% of the terrestrial water with bulk δDs close to zero: the CI (preferred) and CM chondrites. The third one, the comets, should be mixed with an equal amount of EH-like hydrogen coming from mantle outgassing.
But what for siderophile elements? Siderophile elements in the mantle are brought only by the veneer. So the veneer must have the right isotopic composition.
Recently, the attention has been brought to the isotopic composition of mantle osmium, more precisely the abundance of 187Os, whose concentration is expressed by the ratios 187Os/186Os or 187Os/188Os. 187Os is partly produced by 187Re, another strongly siderophile element. So the couple ReOs in the mantle is the (differentiated) image of the veneer. Meisel et al. [42] have reconstructed the primitive 187Os/188Os for the mantle. They have a very limited range, which agrees well with enstatite and ordinary chondrites but is far from the carbonaceous range. The confidence range they give for the primitive mantle ratio is maybe a little too optimistic, but the difference with the carbonaceous chondrite range is so large that we have to incorporate the osmium criterion in our discussion (Fig. 6).

Osmium isotope composition of chondrites and of the primitive mantle [42]. If this criterion is further validated, it rules out the CI and CM as a possible late veneer source. This veneer then is a combination of EH (bringing the necessary siderophile elements) and some cometary material (for the water).
Composition isotopique de l'osmium dans les chondrites et le manteau terrestre primitif [42]. Si ce critère est vérifié, il élimine les CI et CM comme source possible du vernis tardif. Celui-ci est alors un mélange de EH (pour les sidérophiles) et d'un peu de comètes (pour l'eau).
If that criterion is not confirmed by further studies, then the veneer can be made of CI (preferred) or CM-type material, which will then constitute close to 100% of terrestrial water.
But this criterion up to now looks rather serious: then the veneer should have consisted of two parts.
The major part (80%) is of EH type. This sounds rather logical: if the bulk of the terrestrial material was of a given type, then the last fractions should show some continuity with that type. This part would have brought most of the siderophile mantle load, with the right Re/Os ratio and initial osmium isotopic composition. It will bring negligible amounts of hydrogen (less than 0.5% of the total).
The rest (20%) is made of comets, with their water and their D/H equal to two times the present terrestrial ratio. Their water has to be mixed with an equal amount of mantle water and that mixing has to be quick and thorough, so that, after ∼700 million years at most, negligible traces remain from that initial isotope heterogeneity. Convection and corresponding spreading rates were at least one order of magnitude higher than the present ones at that time. This should render that condition applicable.
Comets have another advantage, which looked hindered by the D/H discrepancy, but which is made possible by the EH-comet double origin: this is to give a simple explanation to a long-time geochemical puzzle, that of the atmospheric distribution of the rare gases, particularly the overabundance of krypton and xenon. That overabundance would be made possible by the very-low-temperature (10–20 K) adsorption of noble gases on comets grains. For that explanation to be complete, it has to be checked experimentally (or maybe directly with a 10-year delay!) that this selective adsorption also brings the required isotope effect for these heavy noble gases [3].
Finally, I have made preliminary calculations that show that, if applied to Venus, this model of mixed late veneer (EH plus comets) could explain elegantly the main features of Venus' atmosphere regarding water and carbon dioxide: large carbon dioxide concentration and residual water with a very high D/H ratio . The main difference would be in the proportion of comets in the veneer (less than 3% instead of 15–20%), which makes sense for a planet closer to the Sun, and in the higher surface temperature. These preliminary calculations explain the D/H ratio of Venus water by reduction of water by carbon at around 400 °C.
10 Early evolution of terrestrial water. Its isotopic labelling
Terrestrial water presently displays two very remarkable isotope features, linked to two major phenomena: the first one is an incredibly strong δD– in meteoric waters, the second one is the buffering of the oxygen isotope composition of the oceans by hydrothermal circulation at oceanic ridges. These two phenomena may have been operative very early in the Earth's history.
10.1 The oceans' signature
I shall describe immediately two present-day characteristics of the ocean system, which probably applied very soon in the Earth's history. One is the building of the meteorological system and its very interesting isotopic translation. The other one is the interaction of the ocean system with the spreading centres of the shallow mantle, again with a very interesting isotope result.
Let us start with the second one. In Fig. 7a, we have a typical profile of in the oceanic crust: this profile results from the hydrothermal activity at Mid Ocean ridges, where the ocean water, infiltrated at low temperatures, alters the oceanic crust at progressively increasing temperatures. This results first in a increase of the altered material at the expense of ocean water, whose hence decreases progressively, while it goes deeper and deeper. Because it continues to alter the underlying crust and because the fractionation rock-water linked to the alteration process gives progressively decreasing in the rock, the rock eventually decreases below the initial mantle value, until finally the isotopic evolution of the rock reverses with increasing depth and gets back to the original mantle value when the maximum penetration of water is attained [1,22,26]. The surface balance between parts 1 and 2 is a measure of the total enrichment of the crust during the hydrothermal process, and of the corresponding depletion of the ocean water. One can show rather easily that, starting from any initial difference between the of the ocean and the of the mantle, the process will arrive to a steady state where the two surfaces are equal and no enrichment or depletion occurs: the of the small ocean is buffered by the of the large mantle and this is the reason of the puzzling invariance of the of the oceans observed during geological times. The time constant of that buffering is presently about 50 million years and is a decreasing function of the average spreading rate. This rate was probably several times higher at the beginning of the Earth's history. Hence the oceans must have acquired very rapidly their present signature.
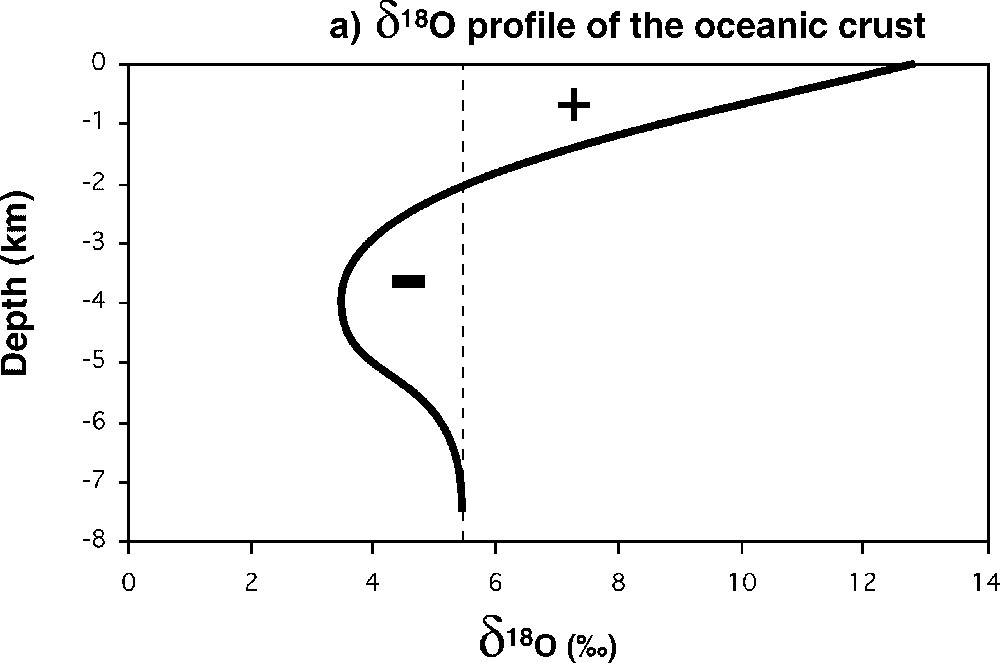
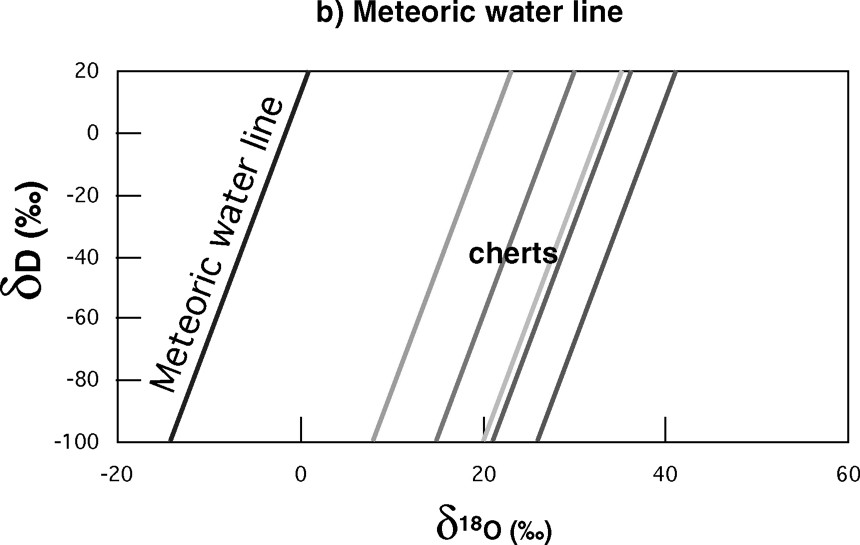
(a) Typical profile across oceanic crust. It results from crust-infiltrated seawater interaction. The upper crust is enriched in 18O by interaction at low temperature and the resulting 18O-depleted seawater depletes the lower crust at higher temperatures. The fact that the two surfaces marked + and − are equal is the expression of a steady state where the seawater composition is buffered by that interaction. The rate of buffering is directly related to the rate of mantle convection. It has certainly been very fast at the high early spreading rates of the mantle. (b) The meteoric water straight line, of equation , lies at the extreme left of this diagram. The striking simplicity of the δD– relationship among meteoric (rain and fresh waters) waters results from the little variability of the ΔD/ ratio during water vapour condensation. This line is thought to have existed since the beginning of the meteoric cycle. δD– lines defined by Archean and more recent cherts show up to the right of the meteoric water line, with ages from 3.5 billion years to 50 Myr.
Régulation de la composition isotopique de l'eau superficielle (océans et eaux météoriques). (a) Profil typique à travers la croûte océanique. Il résulte de l'interaction avec la croûte d'eau de mer infiltrée. La partie supérieure de la croûte est enrichie en 18O par l'interaction à haute température et l'eau, appauvrie, quant à elle, en 18O, appauvrit la croûte inférieure par interaction à haute température. Ce phénomène évolue vers un état stationnaire où les surfaces marquées + et − sont égales, et la composition de l'eau infiltrée est alors la même à l'entrée et à la sortie. La composition des océans finit par être « tamponnée » par ce phénomène. Actuellement, la constante de temps de ce phénomène (temps nécessaire pour que la totalité des océans soit isotopiquement recyclée) est d'environ 50 millions d'années. Ce temps, inversement proportionnel à la vitesse de convection du manteau, était certainement beaucoup plus faible au début de l'histoire terrestre. (b) La droite des eaux météoriques, d'équation , se trouve à l'extrême gauche du diagramme. La remarquable simplicité de cette relation résulte de la faible variabilité du rapport ΔD/ entre liquide et vapeur durant la condensation de l'eau. On pense que cette relation a existé très tôt dans le cycle météorologique. Les droites représentatives des cherts (silice précipitée en milieu marin) d'âge Archéen (3,5 milliards d'années) à Récent (∼50 millions d'années), se trouvent dans la partie droite du diagramme.
10.2 The meteoric water system
Fig. 7b shows the δD– relationship among the worldwide rainwater system [12,13].
The detailed explanation of this incredibly simple relationship for such a complex system needs some time but the main feature is rather simple: for the two elements linked in the water molecule, the liquid–vapour isotopic fractionation varies little in the temperature range of rain precipitation: it is approximately equal to the ratio of the relative mass variations when going from hydrogen to deuterium and oxygen-16 to oxygen-18, that is 8. The only out-of-isotopic equilibrium step in rainmaking is the first one, the evaporation over the tropical oceans, which leads to a small (10) offset of the line from the origin. So again one can expect that relationship to have been established rather soon, unless very high temperature conditions prevailed for a very long time.
This explains why many authors have used the present isotopic reference frame for oceans and meteoric waters as a good approximation for the discussion of Proterozoic and Archean results. In the present paper, I just want to evoke two of them, the Earth's surface temperature and the size of the early oceans. Fig. 7b gives a sketch of the δD– relationship found in Archean, Palaeozoic, and modern cherts.
10.3 Surface temperature and/or growth of the oceans
The oceans isotope composition and the meteoric water line are the basis for the isotopic interpretation of the Earth's surface record and, particularly, of the sedimentary record. If the sediments contain hydrogen and oxygen (clay minerals or cherts, very important in the Archean and Proterozoic record), these sediments are characterized by their isotopic fractionation coefficients with water for hydrogen and oxygen, and (Fig. 8a). When measuring the isotopic composition of Archean and Proterozoic cherts, for example, one can hope to deduce the Archean and Proterozoic temperatures by the distance between the meteoric water line and the parallel lines corresponding to the cherts of various epochs. This has been attempted [37], and the corresponding numbers are given in Fig. 8a. The results (surface temperatures up to 80 °C) are somewhat puzzling, but may be true after all, except that there are no independent checks of such a hypothesis.
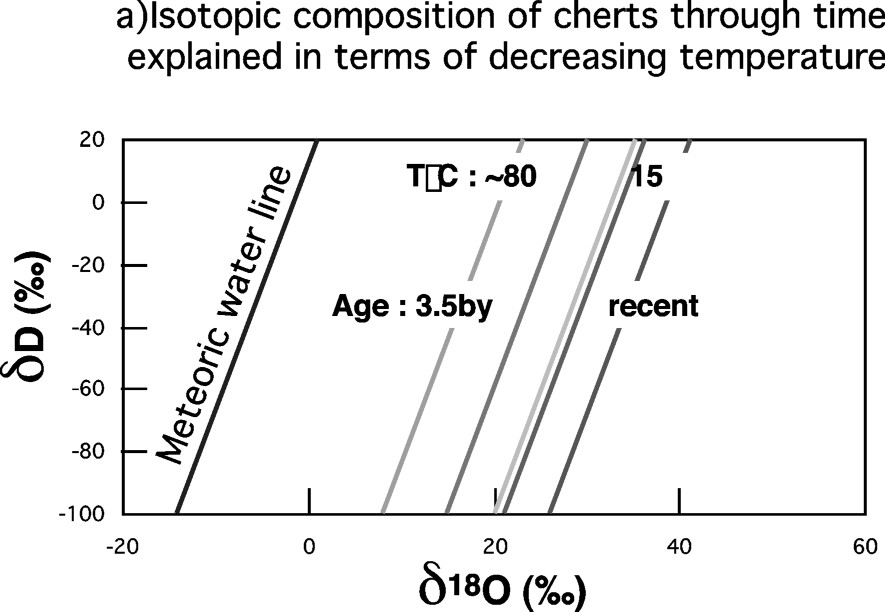
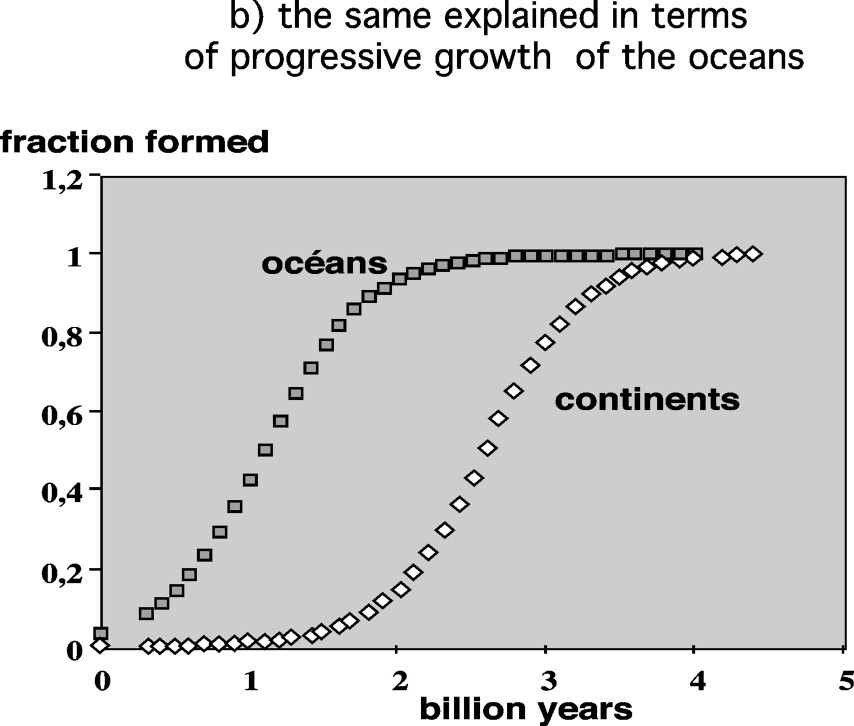
Temperatures or/and ocean masses at the beginning of Earth's History. (a) The cherts' lines, grossly parallel to the meteoric water line, result from equilibration with the water system at variable temperatures. The closer they are to this line, the higher their temperature of formation. This could indicate temperatures as high as 80 °C for the oldest cherts [37]. (b) Alternatively, however, these variable distances could simply reflect a mass balance effect between the cherts' mass and the growing ocean mass (see § Stable isotope tracers). In this case, one can deduce the chronology of the oceans' growth and compare it to that of the continents.
Températures et/ou masses des océans au début de l'histoire terrestre. (a) Les droites représentatives des cherts, grossièrement parallèles à celle des eaux météoriques, peuvent résulter de l'équilibration de ces cherts avec l'océan à des températures variables : plus elles sont proches de la droite des eaux météoriques, plus la température est élevée. Cela pourrait indiquer des températures aussi élevées que 80 °C à l'Archéen [37]. (b) Cependant, au contraire, ces distances variables pourraient indiquer, à température constante, un rapport de masse variable entre l'océan et les cherts au cours du temps, d'autant plus petit que la droite des cherts se situe plus près de la droite météorique (voir § Stable isotope tracers). Dans ce cas, on peut en déduire la chronologie de la croissance des océans et la comparer à celle des continents.
However, there is another way of explaining the apparently shorter distance of Archean cherts lines to the meteoric water line: if the size of the Archean oceans was much smaller at the beginning, then the large enrichment in 18O of large cherts deposits depleted significantly the oceans in 18O, making the Archean ocean's negative and the meteoric waterline translated to the left (the hydrogen balance is not significantly modified because of the low water concentration of the cherts). If we know the approximate mass of the cherts of a given period, we can calculate, approximately also, the corresponding size of the oceans. Fig. 8b gives an example of the results of such a calculation for the ocean growth, compared to the continental growth.
Of course, the correct interpretation of such results is probably a mixture of the two above possibilities, decreasing temperature plus ocean growth. It must also be pointed out that the results for Archean cherts do not concern formations older than 3.4 billion years, 1 billion years already after the major late veneer input. In this respect, it would be very interesting to have the same results on small-banded iron formations outcropping among 3.8-billion-year-old rocks in Greenland.
In any case, the Oceans growth modelling is linked to important questions regarding the mechanisms of the water input to the Earth's surface:
- – the schedule of the veneer deposition: it is largely concentrated on the first hundred million years, but this concerns the total mass of the veneer. If the veneer is CI, then the water (or water precursor) supply and the veneer supply are synchronous. If the water is brought by comets, then the comets may be delayed relative to the main veneer flux;
- – similarly, in the comet hypothesis, the fraction coming from the mantle outgassing becomes important (about 50%). So its timing relative to the comets input is important as well. This timing is related to that of the oxidation reactions in the mantle;
- – finally, one must point out that comets provide directly water. In the case of a CI veneer, it must be noted that only part of the hydrogen is under the form of water. Another form, trapped in the kerogen polymer, has to be oxidized first.
So this small incursion into the possible chronology of Oceans growth opens a range of questions, difficult to answer for most of them at the time being, but essential for the history of the first seven hundred millions of the Earth's surface, and even, of the mantle.
11 Surface–mantle relationships. The global recycling of water
11.1 The present picture
The present picture of the surface–mantle relationships can be summarized as follows.
During volcanism at ocean ridges, the MORB basaltic magmas carry with them an average 0.3% of dissolved water (range 0.05–0.7%). Due to the large solubility of water in such magmas and to the average pressure over ridges, this water is very little outgassed to the ocean. Approximately 95% of the water stays in the basalt and is entrained in the spreading of oceanic crust (about 1014 g, or 100 million tons per year) [30,48].
On the contrary, more water is fixed into the oceanic crust, first by the ridges hydrothermal system, then by ‘low-temperature’ hydrothermalism. Calculations of this water flux, whose ultimate fate will be subduction, give about ten times more (1015 g or 1 billion tons per year, which includes as well the water from subducted sediments), but ∼90% of that flux is returned to the atmosphere ocean or crust through the subduction zone volcanism (see below).
The role of water in subducted oceanic crust is primordial to constrain the type of volcanism prevalent in subduction zones (island-arc and continental-margin volcanism) [49]. The combination of high water concentrations and of the particular P–T relationships given by the deep subduction of previously cold material gives birth to fluids very rich in dissolved silicates, which induce, in the above mantle ‘wedge’, the formation of water-rich magmas (2 to 20%, with an average of 5–6%). These characteristics can be shown by petrologic studies (particularly on glass inclusions, small samples of magma trapped at high pressures by the developing crystals), and also by the general study of oxygen and hydrogen isotopes on rocks and gases. It is thus possible to show that the return flux of water by subduction volcanism to the surface and the crust is about 90% of the water-subduction flux. This volcanic flux is shared about evenly between the perioceanic subaerial volcanism and the plutonic part of the magmas, trapped in the crust.
The result is that the surface–mantle exchanges for water are balanced: the mantle water concentration, as well as the average hydrogen isotope composition of the mantle, do not vary globally with time, even if, locally, large D/H variations (40 to 60‰) can result from extreme outgassing effects. But one must keep in mind that this applies only to the upper mantle (above 1000–1100 km), that is, about half of the mantle. Very little, if anything, is known of water below that depth.
11.2 Historical aspect
What was the situation in the past? Studies of ancient mantle rocks (back to 2 billion years at least), isotopic in particular, give the impression that the situation of mantle water was very little different from the present one. However, it is very difficult to find old rocks absolutely free of any alteration, and one of the first ‘victims’ of that alteration, however small it may be, will be the hydrogen isotope composition. Because of a peculiar dependence to temperature, the alteration mechanisms for hydrogen isotopes frequently give fractionations very similar to those in the magmatic range, so that these indications are not completely reliable.
So it is better to reason from surface and mantle masses, and corresponding fluxes. The masses are of the order of 1024 g, and the present net fluxes of 1014 g yr−1. So the residence times in any reservoir are in the order of 5 to 10 billion years with the present-day fluxes.
What were these fluxes at the beginning?
For this, we have to see if the initial situation, particularly in terms of mantle concentrations, was very different from, or similar to the present. We have also to see the oldest state similar to the present. With the caveat about alteration given above, it seems that the present situation prevailed since at least 2 to 3 billion years.
Then the CI and comet hypothesis give contrasted situations: in the CI case, the equivalent of the present oceans mass was very soon present at the surface. The isotopic composition of hydrogen was globally close to the present one and the only evolution to follow to reach the present state was to gently incorporate a quarter of the total water into the mantle, that is . With the present-day fluxes, this corresponds to a few billion years. So, even if the very early fluxes were larger than the present ones (and they probably were), we do not need to imagine drastic changes in the rate of surface–mantle exchanges.
In the comet case, however, the situation is much more contrasted: half of the water mass (1024 g) is in the mantle, with a very low D/H, while the other half is at the surface, with a D/H double of what it is now. Hence the system has to be thoroughly mixed in order to explain that, about one billion years later, we can see no significant isotopic trace of that early heterogeneity. This implies fluxes one or two orders of magnitude higher for at least the first hundred million years of the Earth.
Again the fact that the temperatures, the convection rates, the outgassing rates, were larger in the first billion years looks simply reasonable. The problem is: to which extent?
To have a better evaluation, it is interesting to compare the water case to the needs imposed by the other two major volatile elements: carbon and nitrogen. This is because, for these elements, there is no real difference between the CI and the comet hypothesis: both hypotheses give comparable concentrations, and, to our knowledge, comparable isotope problems. For example, terrestrial nitrogen will have to be the mixture of a 15N-poor bulk Earth component and a 15N-rich veneer component. The isotopic study of old diamonds shows that, ∼3 billion years ago, the degree of mixing of both into the mantle was very similar to what it is today.
We can model that mixing with a ‘realistic’ first-order rate law, where the outgoing flux from a given reservoir is proportional to the mass it contains. The results are given in Fig. 9.
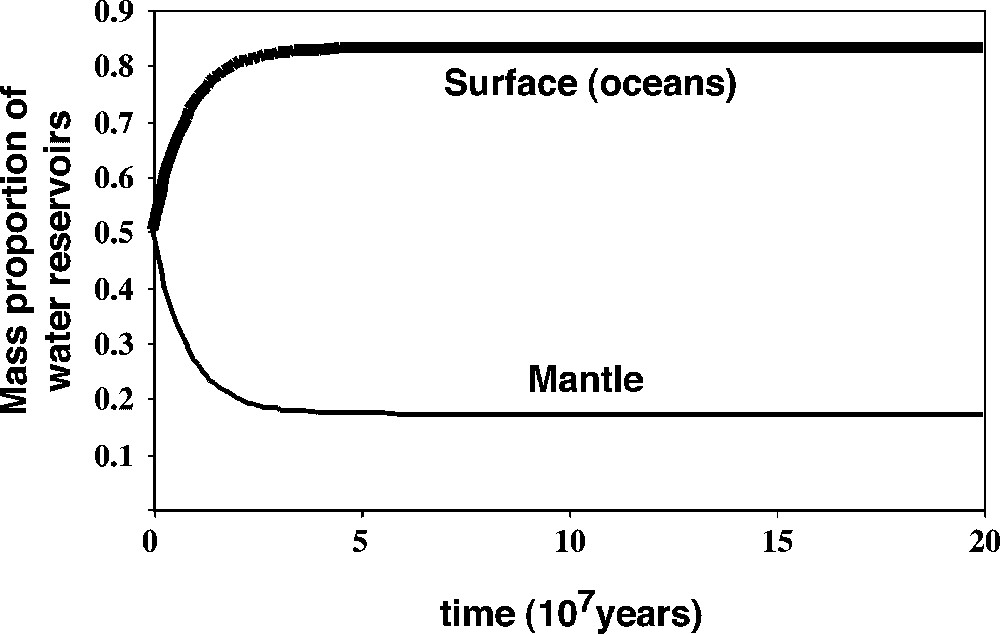
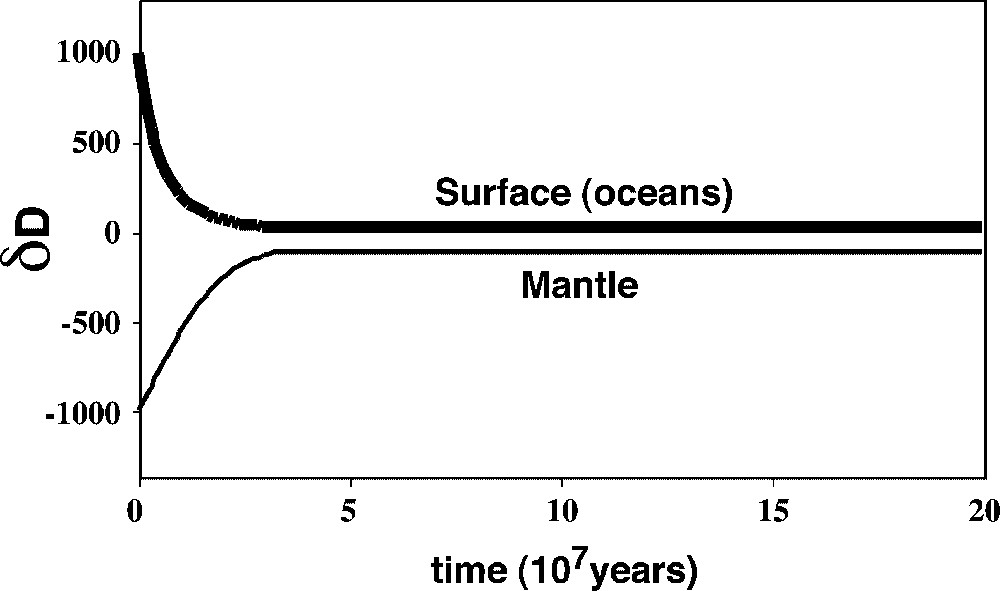
Time evolution of the relative equivalent water masses (as water or as hydrogen) in the upper mantle and at the surface. This evolution is particularly critical if the late veneer water if solely of cometary origin (δD of the veneer close to +1000), because the amount of water outgassing from the mantle must then be very significant and its δD very different (∼−1000). The masses are expressed as a fraction of the total mass (∼2×1024 kg). Due to the very high initial mantle convection rates, the present steady state can have been reached in time spans as low as 80 Myr.
Évolution temporelle des masses d'équivalent eau (eau ou hydrogène) relatives entre surface et manteau. Cette évolution est particulièrement critique si l'eau du vernis tardif est uniquement d'origine cométaire (δD du vernis proche de +1000), parce que la quantité d'équivalent eau dégazant du manteau doit alors être importante et son δD très différent (∼−1000). Les masses sont exprimées en fraction de la masse totale (∼2×1024 kg). À cause des très grandes vitesses initiales de convection mantellique, l'état stationnaire actuel peut avoir été obtenu dans une durée aussi courte que 80 millions d'années.
12 Conclusions
During the first hundred million years of the solar system, water enters the solar nebula and isotopically exchanges with hydrogen. Some more water is created, then partially destroyed with growing temperature. A large part enters the Sun, another the core of giant planets. Finally all remains are swept out and eventually stored in the outer edge of the asteroid belt and in the comet-forming clouds. From there, some of it is delivered to the inner solar system. All these different waters are deuterium-labelled. The original water is deuterium-rich , but later exchanges isotopically with hydrogen and its D/H is lowered towards the comet value . The newly created water is deuterium-poor ( or even lower). Its remains appear mainly in carbonaceous chondrites (CI and CM) together with 10–15% comet-like water (cometary D/H ratio). That combination of deuterium-rich and deuterium-poor water gives these materials a global D/H ratio close to ‘terrestrial’ . Finally, the T-Tauri wind is the source of deuterium-free hydrogen that can be implanted in terrestrial material together with solar rare gases, now seen to evolve from the Earth's mantle. If oxidized, this hydrogen can be the source of early-outgassed water.
What are the opportunities for the Earth to get a share of these different waters? With its material processed under the very reducing conditions of the T-Tauri wind in the 6–12-million-year period, its accretion completed after the great impact at million years, covered with a late veneer of chondrites and comets mainly before 100 million years, the Earth has got part of its water budget during the main accretion (under the form of hydrogen), and part of it from the late veneer.
If no other criterion is available, both carbonaceous chondrites and comets can be the source of the veneer water. If it is carbonaceous chondrites, they carry the right D/H signature and the contribution of mantle outgassing has been small.
If the 187Os/188Os criterion that rules out the carbonaceous source is confirmed, then the veneer was made of ∼90% EH material and ∼10% comets. In that case, mantle outgassing represents about 50% of the terrestrial water supply and a very efficient isotope mixing has occurred during the very fast convection system of the first 500 million years of the Earth's mantle history.
Another argument favouring the comets' hypothesis is the distribution of rare gases in the terrestrial atmosphere. The anomalous concentrations and isotopic compositions of atmospheric krypton and xenon have been explained in the last twenty years by several complicated, not satisfying, and difficult-to-test mechanisms. None is as simple and as convincing than the idea of very low temperature adsorption of these gases on comet grains and in comet water ice [47]. Successful elemental segregation experiments of rare gases under cometary conditions have been performed. If krypton and xenon isotopic fractionations during these experiments reproduce the Earth's atmosphere compositions, then the comets hypothesis will be inevitable and we shall have to admit that half of the terrestrial water is of cometary origin.
1 The stable isotope composition of an element is generally reported as relative deviations of an isotope ratio (e.g., R18 = 18O/16O or RD = D/H) named δ (e.g., ((R18)sample/(R18)std−1) or ((RD)sample/(RD)std−1). Oxygen and hydrogen have the same international standard, SMOW, which is essentially the bulk isotope composition of the terrestrial oceans.
2 The slope 1 line could be due alternatively to high-energy (e.g., governed by UV radiation) chemistry, which also produces this type of slope 1 isotope evolution. Discovered by Mark Thiemens in 1983 [58], these isotope evolutions are important in the present-day upper atmosphere and were possibly widespread in the Earth's early atmosphere.


