Analysis of the ‘Biarez–Favre’ and ‘Burland’ models for the compressibility of remoulded clays

Jean-Louis Favre; Mahdia Hattab

doi:10.1016/j.crte.2007.11.004

Geomaterials

Analysis of the ‘Biarez–Favre’ and ‘Burland’ models for the compressibility of remoulded clays
[Analyse des modèles « Biarez–Favre » et « Burland » pour la compressibilité des argiles reconstituées]

Présenté par : Georges Pédro

Jean-Louis Favre ¹ ; Mahdia Hattab ²

¹ Laboratoire de mécanique des sols, structures et matériaux, CNRS–UMR 8579, École centrale Paris, Grande voie des Vignes, 92295 Châtenay-Malabry cedex, France
² Laboratoire de physique et mécanique des matériaux, CNRS–UMR 7554, université Paul-Verlaine, île du Saulcy, 57045 Metz cedex 1, France

Comptes Rendus. Géoscience, Volume 340 (2008) no. 1, pp. 20-27.

Résumés

Anglais
Français

This study aims at comparing the prediction by the Biarez and Favre model as well as by the more recent Burland one, established for reconstituted normally consolidated clays submitted to oedometric loading. The former, proposed in the 1970s, uses the liquidity index I_L, and while the latter introduces a parameter, I_v, which is a normalised void index based on two characteristic void ratios ( $e_{100}^{*} and e_{1000}^{*}$ ) corresponding to the oedometric curve of ${σ^{'}}_{v} = 100 kPa$ and ${σ^{'}}_{v} = 1000 kPa$ . The aim of these models is to predict the compressibility parameters based on the identification of parameters represented by the Atterberg limits (w_L, w_P, I_p) as well as of other physical parameters such as the void ratio e or the natural water content w_nat, taking into account the effective overburden pressure ${σ^{'}}_{v}$ . These models, which represent the intrinsic properties of clays under compression, are compared with two experimental curves, the first one representing remoulded and reconstituted clay, and the other one a deepwater clay sediment taken from the Gulf of Guinea at a depth of 700 m.

On se propose, dans cette étude, de comparer le fonctionnement de deux modèles établis pour les argiles reconstituées, normalement consolidées, soumises à un changement œdométrique. Le modèle de Biarez et Favre, assez ancien, qui utilise l’indice de liquidité I_L, et le modèle de Burland, un peu plus récent, qui introduit I_v, un indice des vides normalisé, basé sur deux valeurs caractéristiques de l’indice des vides $e_{100}^{*} et e_{1000}^{*}$ , à ${σ^{'}}_{v} = 100 kPa$ et ${σ^{'}}_{v} = 1000 kPa$ de la courbe de chargement œdométrique. Le principe de ces modèles est de prédire les paramètres de compression à partir des paramètres d’identification, représentés par les limites d’Atterberg (w_L, w_P, I_p), et des paramètres physiques ou d’état, comme e ou w_nat, en tenant compte de la contrainte en place ${σ^{'}}_{v}$ . Ces modèles, qui représentent les propriétés intrinsèques à la compression, sont confrontés aux résultats expérimentaux de deux argiles, l’une remaniée et reconstituée au laboratoire et l’autre naturelle provenant de sédiments marins du golfe de Guinée, par 700 m de profondeur.

Métadonnées

Reçu le : 2006-09-11
Accepté le : 2007-11-19
Publié le : 2008-01-01

DOI : 10.1016/j.crte.2007.11.004

Keywords: Intrinsic compressibility, Oedometric tests, Remoulded reconstituted clays
Mot clés : Compressibilité intrinsèque, Essais œdométriques, Argile remaniée reconstituée

Affiliations des auteurs :

Jean-Louis Favre ¹ ; Mahdia Hattab ²

¹ Laboratoire de mécanique des sols, structures et matériaux, CNRS–UMR 8579, École centrale Paris, Grande voie des Vignes, 92295 Châtenay-Malabry cedex, France
² Laboratoire de physique et mécanique des matériaux, CNRS–UMR 7554, université Paul-Verlaine, île du Saulcy, 57045 Metz cedex 1, France

@article{CRGEOS_2008__340_1_20_0,
     author = {Jean-Louis Favre and Mahdia Hattab},
     title = {Analysis of the {{\textquoteleft}Biarez{\textendash}Favre{\textquoteright}} and {{\textquoteleft}Burland{\textquoteright}} models for the compressibility of remoulded clays},
     journal = {Comptes Rendus. G\'eoscience},
     pages = {20--27},
     publisher = {Elsevier},
     volume = {340},
     number = {1},
     year = {2008},
     doi = {10.1016/j.crte.2007.11.004},
     language = {en},
}

TY  - JOUR
AU  - Jean-Louis Favre
AU  - Mahdia Hattab
TI  - Analysis of the ‘Biarez–Favre’ and ‘Burland’ models for the compressibility of remoulded clays
JO  - Comptes Rendus. Géoscience
PY  - 2008
SP  - 20
EP  - 27
VL  - 340
IS  - 1
PB  - Elsevier
DO  - 10.1016/j.crte.2007.11.004
LA  - en
ID  - CRGEOS_2008__340_1_20_0
ER  -

%0 Journal Article
%A Jean-Louis Favre
%A Mahdia Hattab
%T Analysis of the ‘Biarez–Favre’ and ‘Burland’ models for the compressibility of remoulded clays
%J Comptes Rendus. Géoscience
%D 2008
%P 20-27
%V 340
%N 1
%I Elsevier
%R 10.1016/j.crte.2007.11.004
%G en
%F CRGEOS_2008__340_1_20_0

Jean-Louis Favre; Mahdia Hattab. Analysis of the ‘Biarez–Favre’ and ‘Burland’ models for the compressibility of remoulded clays. Comptes Rendus. Géoscience, Volume 340 (2008) no. 1, pp. 20-27. doi : 10.1016/j.crte.2007.11.004. https://comptes-rendus.academie-sciences.fr/geoscience/articles/10.1016/j.crte.2007.11.004/

Version originale du texte intégral

1 Introduction

Two parameter classes may be used to characterize clay sediment properties. The first one concerns the geotechnical index properties linked to the particles’ mineralogical nature and can be represented, for example, by the Atterberg limits, or by their equivalent void ratios, $e_{L} = (γ_{s} / γ_{w}) w_{L} and e_{P} = (γ_{s} / γ_{w}) w_{P}$ , where γ_s and γ_w are the unit weight of the solids and of the water, respectively. The second relates to the mechanical parameters (e or w_nat) that express the geometric arrangement of particles and their evolution. Particles may be bonded due to cementation during the sedimentation and consolidation processes [2,7]. In this case, the clay is non-remoulded and the link between the particles is an important parameter in the phenomenological behaviour [6], in particular during an oedometric loading in a compressibility study [8,9]. When there is no bond between the particles or when the bond has been destroyed (by a high loading for example), the soil is described as remoulded. It is important to study the behaviour of material that has been remoulded and reconstituted in the laboratory in normally consolidated and overconsolidated conditions [5,14] because the ‘intrinsic’ mechanical properties can then be deduced, making it possible to define a fixed reference framework in order to explain the behaviour of natural, non-remoulded clay. Biarez and Favre [3,4], and later Burland [8], introduced the concept of intrinsic compression properties in order to describe, in one normalized plane, the behaviour of one-dimensional, normally consolidated clay that had been remoulded and reconsolidated (at w_sat = 1.5 w_L). This paper proposes a comparative analysis of these two approaches. It considers two experimental curves, the first one pertaining to a remoulded reconstituted clay (the Kaolinite P300) which has been the subject of several laboratory studies [14], and the other one to a natural clay taken from deepwater sediments (at a depth of 700 m) in the Gulf of Guinea [13].

2 The Biarez & Favre (B&F) model

The soil – a granular and discontinuous medium (DM) – may be seen as a virtual continuous medium (CM), using, for statistical connections, the logical framework shown in Table 1. The ‘mechanical properties of soils’ thus appear as the integration of the grain properties (called ‘nature of grains’) in their spatial configuration (called ‘arrangement of grains’) and their mechanical boundary conditions (which can be represented by the consolidation stress tensor). In the case of remoulded clays, Favre [11,12] shows that the Atterberg limits (‘nature of grain’ parameters) allow us to explain the mechanical properties. In mineral clays, these properties are strongly linked by the following relation:

I_{p} = 0.73 (w_{L} - 13)

(1)

In this way, the model proposed in Fig. 1a, which, plotted with the Casagrande A line, defines the mineralogical nature of the clay.

Fig. 1
Biarez and Favre correlations for the normally consolidated remoulded clays.
Fig. 1. Corrélations de Biarez et Favre pour les argiles reconstituées, normalement consolidées.

On the other hand, a large number of results collected by Favre [11,12] show that the 6.5- and 1000-kPa loadings on the oedometric path correspond, on average, to w_L and w_P, respectively (or e_L and e_P, considering $γ_{s} / γ_{w} = 2.7$ ), see relation (2) and Fig. 1b. This leads first to equations (3) or (6) for the compressibility index C_c, then to relation (4) when using (1) in (3).

\{\begin{array}{c} w_{sat} = w_{L} for {σ^{'}}_{v} \sim 6.5 kPa \\ w_{sat} = w_{P} for {σ^{'}}_{v} \sim 1 MPa \end{array}

(2)

C_{c} = \frac{(w_{L} - w_{P}) 2.7 / 100}{\log (1000 / 6.5)}

(3)

C_{c} = 0.009 (w_{L} - 13)

(4)

C_{c} = 0.007 (w_{L} - 10)

(5)

C_{c} = \frac{I_{p}}{81}

(6)

By comparison with relation (5), proposed by Skempton [16] for remoulded clays, we underestimate by 10% the compression index C_c for w_L = 40% and by 5% for w_L = 70%. Nevertheless, the simple model represented by relations (2) and (4) was retained for the oedometric test, and hence, by relation (1), it uses only one Atterberg limit, w_L. It is a straight line in the $(e, \log {σ^{'}}_{v})$ or $(w_{sat}, \log {σ^{'}}_{v})$ plane, passing through the two points given by relation (2), with a slope defined by equation (4). A single abacus was then obtained in equation (7), ${σ^{'}}_{v}$ being expressed in kPa and $I_{L} = (w - w_{P}) / (w_{L} - w_{P})$ :

I_{L} = 0.46 (3 - log {s^{'}}_{v})

(7)

The ‘arrangement of grains’ therefore appears to be directly linked to the consolidation stress tensor, through the mineralogical properties. This connection corresponds in the $(I_{L} - {σ^{'}}_{v})$ plane to a straight line called NCRS (Normally Consolidated Remoulded Simplified, see Fig. 1c). Thus, a normally consolidated remoulded clay will be represented by points located on the NCRS, the overconsolidated remoulded clay by points below the NCRS. In this case, the overconsolidation of the material only depends on the clay loading history, expressed by the overconsolidation ratio $O C R = {σ^{'}}_{p} / {σ^{'}}_{v}$ . Here ${σ^{'}}_{p}$ is the maximum effective stress and ${σ^{'}}_{v}$ the overburden effective stress.

3 Burland's model

Burland [8] collected and analyzed the oedometric compressibility of several clay sediments remoulded and reconstituted in the laboratory and with liquid limit w_L varying from 25% to 159%. The curves obtained in the ( $e - \log {σ^{'}}_{v}$ ) plane are slightly concave when ${σ^{'}}_{v}$ is located between 10 and 1000 kPa (Fig. 2a). The intrinsic compressibility index $C_{c}^{*}$ is therefore introduced (equation (8)) as the difference between $e_{100}^{*} and e_{1000}^{*}$ , two characteristic quantities corresponding to the consolidation stresses at 100 and 1000 kPa, respectively. $C_{c}^{*}$ is also the slope of the oedometric compression curve (linear in this interval of stresses), the quantity {−[log(100)−log(1000)]} being equal to 1. With the transformation of the variable defined in (9), and by introducing the normalized void index I_v, the Burland model requires all experimental curves to pass through two fixed points in the ( $I_{v} - \log {σ^{'}}_{v}$ ) plane, corresponding to $e = e_{100}^{*}, I_{v} = 0$ and $e = e_{1000}^{*}, I_{v} = - 1$ for ${σ^{'}}_{v} = 100 kPa$ and ${σ^{'}}_{v} = 1000 kPa$ , respectively.

C_{c}^{*} = e_{100}^{*} - e_{1000}^{*}

(8)

I_{v} = \frac{e - e_{100}^{*}}{C_{c}^{*}}

(9)

Fig. 2
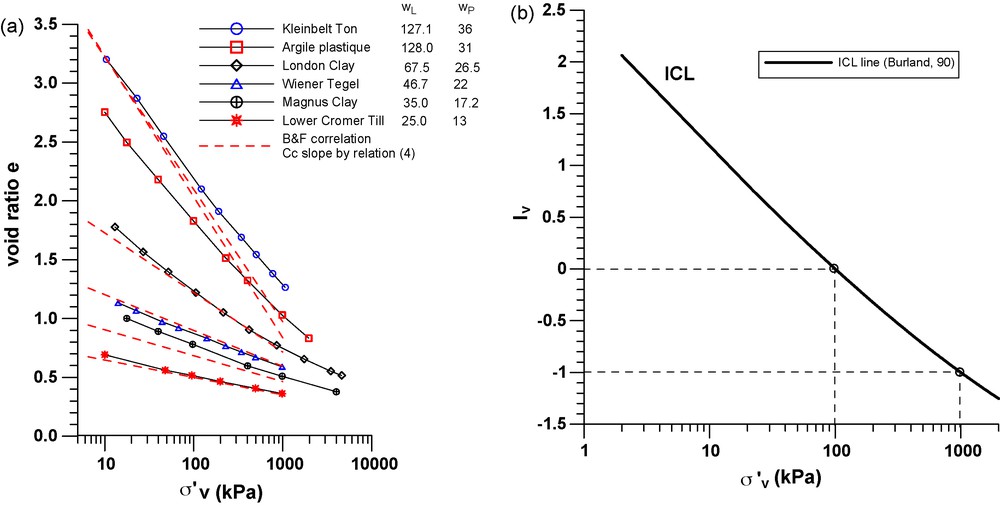
The intrinsic compression line by Burland [8].
Fig. 2. Ligne de compression intrinsèque de Burland [8].

The ICL (Intrinsic Compression Line) curve, corresponding to equation (10) and represented by Fig. 2b, can then be deduced from a statistical model based on the experimental curves passing through the two specific points ( ${σ^{'}}_{v} = 100 kPa$ , I_v = 0) and ( ${σ^{'}}_{v} = 1000 kPa$ , I_v = –1). ${σ^{'}}_{v}$ is expressed in kPa.

I_{v} = 2.45 - 1.285 \log {σ^{'}}_{v} + 0.015 {(\log {σ^{'}}_{v})}^{3}

(10)

The two particular parameters $e_{100}^{*} and e_{1000}^{*}$ for the ‘arrangement of grains’ are, on the other hand, connected by equation (11) to e_L, the void ratio corresponding to the liquid limit, and the only parameter for the ‘nature of grains’.

\{\begin{array}{l} e_{100}^{*} = 0.109 + 0.679 e_{L} - 0.089 e_{L}^{2} + 0.016 e_{L}^{3} \\ C_{c}^{*} = e_{100}^{*} - e_{1000}^{*} = 0.256 e_{L} - 0.04 \end{array}

(11)

3.1 Comment

Although both approaches appear to be based on a similar method, there is a fundamental difference in the manner in which the intrinsic characteristics of the ‘arrangement of grains’ were defined on the oedometric path. Burland does this using two experimental values ( $e_{100}^{*} and e_{1000}^{*}$ ) for the ‘arrangement of grains’. On the other hand, the B&F method, by introducing I_L, used two parameters of ‘nature of grains’ (e_L and e_P). However, both models link the ‘nature of grains’ parameters to the ‘arrangement of grains’ ones, using statistical correlations under oedometric loading. This corresponds to relation (2) in the B&F model and to relation (11) in Burland's model. An examination of Figs. 2a and 3a reveals a difference in the C_c (B&F approach) and $C_{c}^{*}$ (Burland approach) slopes, in particular between ${σ^{'}}_{v} = 100 kPa$ and ${σ^{'}}_{v} = 1000 kPa$ , when the liquid limit is high (w_L = 100% to 160%). On the other hand, for the lower w_L values, C_c and $C_{c}^{*}$ are similar, and fit well the experimental data given by Burland [8] for different values of w_L (see Fig. 3a).

Fig. 3
Variations of $C_{c}^{*}$ and $e_{100}^{*}$ parameters according to e_L.
Fig. 3. Variations des paramètres $C_{c}^{*}$ et $e_{100}^{*}$ en fonction de e_L.

4 Comparison of models and mixed model

4.1 B&F's model in Burland's space ( $I_{v} - \log {σ^{'}}_{v}$ )

According to correlation (7), we have:

I_{L} = \frac{e - e_{P}}{e_{L} - e_{P}} = 0.46 (3 - \log {σ^{'}}_{v})

(12)

Using Eqs. (9) and (12), to express B&F's model as a function of I_v, we get the NCRS equation (13) in Burland's space.

I_{v} = 1.38 \frac{e_{L} - e_{P}}{C_{c}^{*}} - \frac{e_{}^{*} - e_{P}}{C_{c}^{*}} - 0.46 \frac{e_{L} - e_{P}}{C_{c}^{*}} \log {σ^{'}}_{v}

(13)

According to B&F, hence (2)

e_{P} = e_{100}^{*}

and

e_{L} = e_{6,5}^{*}

, equation (13) becomes:

I_{v} = \frac{e_{}^{*} - e_{}^{*}}{C_{c}^{*}} [1.38 - 0.46 \log {σ^{'}}_{v}] - 1 .

(14)

Considering a linear variation between

Δ e and Δ log {σ^{'}}_{v}

, we obtain:

\frac{e_{100}^{*} - e_{1000}^{*}}{\log 100 - \log 1000} = \frac{e_{6.5}^{*} - e_{1000}^{*}}{\log 6.5 - \log 1000}

(15)

Then, B&F's model given by relation (14) becomes:

I_{v} = 2 - \log {σ^{'}}_{v}

(16)

Equation (16) is a straight line in the ( $I_{v} - log {σ^{'}}_{v}$ ) plane, passing through two specific points (I_v = 0, ${σ^{'}}_{v} = 100 kPa$ ) and (I_v = −1, ${σ^{'}}_{v} = 100 kPa$ ), represented by the NCRS line in Fig. 4a. A representation of both the NCRS and ICL lines on Burland's plane shows that the two curves converge in a vertical stress range between 40 and 1000 kPa. Normalization by means of the I_v parameter and $e_{P} = e_{1000}^{*}$ assumption are, of course, fundamental to this result, because they force the NCRS to have the (I_v = −1, ${s^{'}}_{v} = 1000 kPa$ ) point on the ICL line. However, in the stress range lower than 40 kPa, the two lines diverge slightly due to the concavity of the curves (Fig. 2a), which is taken into account in the ICL expression. Both approaches may thus be summarized in Table 2, with the $e_{100}^{*} and e_{1000}^{*}$ indices for ‘B&F in Burland space’ being deduced from both interpolation (15) and relation (1), considering $e_{P} = e_{1000}^{*}$ and $e_{L} = e_{6.5}^{*}$ .

Fig. 4
Comparison between the two statistical models for the one-dimensional loading in ( ${σ^{'}}_{v}$ , I_v) and ( ${σ^{'}}_{v}$ , I_L) planes.
Fig. 4. Comparaison entre les deux modèles statistiques pour le chargement unidimensionnel dans les plans ( ${σ^{'}}_{v}$ , I_v) et ( ${σ^{'}}_{v}$ , I_L).

	Burland's model	B&F's model	B&F's mixed model
Equation	$I_{v} = 2.45 - 1.285 \log {σ^{'}}_{v} + 0.015 {(\log {σ^{'}}_{v})}^{3}$	$I_{L} = 0.46 (3 - \log {σ^{'}}_{v})$	$I_{v} = 2 - \log {σ^{'}}_{v}$
Characteristic points	$\{\begin{array}{l} e_{100}^{} = 0.109 + 0.679 e_{L} - 0.089 e_{L}^{2} + 0.016 e_{L}^{3} \\ e_{1000}^{} = 0.149 + 0.423 e_{L} - 0.089 e_{L}^{2} + 0.016 e_{L}^{3} \end{array}$	$\begin{array}{l} w_{6.5} = w_{L} \\ w_{1000} = w_{P} \end{array}$	$\{\begin{array}{l} e_{6.5}^{} = e_{L} \\ e_{100}^{} = 0.60 e_{L} + 0.14 \\ e_{1000}^{*} = 0.27 e_{L} + 0.26 \end{array}$
Compression index	$C_{c}^{*} = 0,256 e_{L} - 0,04$	$C_{c} = 0,009 (w_{L} - 13)$	$C_{c}^{*} = C_{c} = 0,33 e_{L} - 0,12$

4.2 B&F's ‘mixed’ model represented in Burland's space ( $I_{v} - \log {σ^{'}}_{v}$ )

In the linear relation (13) resulting from B&F, $e_{100}^{*} and C_{c}^{*}$ are replaced by expressions (11) proposed by Burland for the ICL expression. This gives the ‘mixed’ model (17):

I_{v} = 0.46 ζ [3 - \log {σ^{'}}_{v}] - χ

(17)

Here ζ and χ are the parameters given by equation (18) as function of the e_L and e_P limits, defined by their experimental values:

\{\begin{array}{l} ζ = \frac{e_{L} - e_{P}}{0.256 e_{L} - 0.04} \\ χ = \frac{0.109 + 0.679 e_{L} - 0.089 e_{L}^{2} + 0.016 e_{L}^{3} - e_{P}}{0.256 e_{L} - 0.04} \end{array} .

(18)

The ‘mixed’ model described above is applied to the two kinds of clay and are represented in the ( $I_{v} - \log {σ^{'}}_{v}$ ) plane: the Kaolinite P300 (Fig. 4a, NCRS_a(k)) whose Atterberg's limits are w_L = 40% and w_P = 20%, and the deepwater sediments from the Gulf of Guinea (Fig. 4a, NCRS_a(m-s)), whose w_L and w_P limits vary according to depth (therefore according to ${σ^{'}}_{v}$ too) from 110% to 160% for w_L and from 30% to 90% for w_P [13]. Since the assumption of $e_{P} = e_{1000}^{*}$ is not considered, the NCRS_a line is not forced to pass through a point on the ICL line. The NCRS_a line, however, will depend on the e_L limit. When equation (17) is applied to the kaolinite (through the e_L value), we obtain a curve termed NCRS_a(k) located very close to the ICL line, whatever the stress domain is. Both correlations are thus very similar, and compatible with the experimental curve, on the oedometric path, of the kaolinite represented in Fig. 4a in the same plane (the kaolinite having first been consolidated under one-dimensional conditions under an axial stress of 140 kPa). On the other hand, the NCRS_a(m-s) curve calculated for the marine sediments, through their e_L values, diverges from the ICL and the NCRS. The principal difference between the ICL and the NCRS, on the one hand, and NCRS_a(m-s), on the other hand (whereas the calculation of the ‘mixed model’ NCRS_a was focused on the remoulded state condition) seems to be due mainly to the fact that the ‘mixed model’ does not take into account the hypothesis of Biarez's model, expressed by $e_{P} = e_{1000}^{*}$ . Two experimental curves (at z = 9.5 m and z = 16.5 m depths) of the natural non-remoulded marine sediments were plotted in Fig. 4a to compare the two models, in particular at the end of oedometric loading, when the initial structure was destroyed.

The experimental path evolves first from one point located above the ICL curve (sensitive clays) towards a domain that exhibits a ‘fictitious consolidation stress’ and a supplementary cohesion on the oedometric curve. The latter is due to the beginning of the degradation of the cementation between particles developed in situ during the sedimentation and the consolidation processes. Afterwards, the cementation is gradually destroyed (destructuring according to Leroueil et al. [15], and the experimental curves tend to converge at high stresses (up to 1000 kPa) towards the ICL curve of the remoulded state of the material. Clays structuring has been quantified by Cotecchia and Chandler [9,10] using the sensitivity parameter S_t (Skempton and Northey [17]) as a normalizing parameter for the structure. Thus, the evolution of S_t may be used to describe the destructuration phenomenon (destructuration law of Baudet and Ho [1]).

4.2.1 Remark

Burland's relation (10) expressed in the (I_L – log σ’_v) plane using equation (11) becomes equation (19) as a function of the e_L and e_P limits, ζ and χ being defined by relation (18):

I_{L} = \frac{1}{ζ} [2.45 - 1.285 log {σ^{'}}_{v} + 0.015 {(\log {σ^{'}}_{v})}^{3} + χ]

(19)

Since the ICL depends on e_L (Fig. 4b), it is transformed in the ( $I_{v} - \log {σ^{'}}_{v}$ ) plane by a bundle of parallel curves. These curves, for low w_L values, are located in the upper part of the bundle, whereas for high values of w_L, they are located in the lower part. In this plane, the similarity between the two models can be clearly seen, which is also in accordance with the experimental curve for the low w_L values (case of the kaolinite ICL(k)). As previously in Burland's plane, the ICL(m-s) of marine clay, located in the high w_L domain, diverges from the NCRS. The experimental oedometric loading curves (at z = 9.5 m and z = 16.5 m) converge towards the ICL(m-s) in the domain of high stresses, which shows that Burland's correlation is closer to the experimental results for these high values of w_L than B&F's one.

B&F's assumption that $e_{P} = e_{1000}^{*}$ cannot be introduced in Burland's model, because it would force the ICL(m-s) to converge to the NCRS at (I_L = 0, ${σ^{'}}_{v} = 1000 kPa$ ) point; in this case the curve no longer reflects the experimental observation.

5 Conclusion

Two intrinsic compressibility concepts proposed for the remoulded reconstituted clays are discussed in this paper. The NCRS, resulting from the Biarez and Favre's correlation [2,3], is based on the liquid index parameter I_L = (w–w_P)/(w_L/w_P). The ICL, resulting from the Burland's correlation [8], is based on a normalized void index parameter $I_{v} = (e - e_{100}^{*}) / (e_{100}^{*} - e_{1000}^{*})$ .

The ‘arrangement of grains’ in Biarez and Favre is defined through w_L and w_P (or e_L and e_P) – two ‘nature parameters’ – whereas in Burland, this is done by means of the two ‘arrangement parameters’ $e_{100}^{*} and e_{1000}^{*}$ . However, the two approaches have in common the fact that they propose statistical correlations between the ‘nature of grains’ parameters and the ‘arrangement of grains’ ones for the normalized oedometric tests.

When the NCRS is represented in Burland's plane (I_v, ${σ^{'}}_{v}$ ), the difference between the two statistical models is small and the two approaches appear to be similar. The ‘mixed’ model, however, shows that their behaviour is different and that the similarity observed with the NCRS is due to the assumption $e_{P} = e_{1000}^{*}$ , which imposes the (I_L = 0, ${σ^{'}}_{v} = 1000 kPa$ ) point as a common point to both curves. If the ICL and the NCRS are, indeed, close when w_L is low, they nevertheless diverge for the high values of w_L. This result is obtained by representing the two curves in B&F's plane (I_L, ${σ^{'}}_{v}$ ), which appears to be the more explicative plane. This study shows that Burland's ICL line reflects better the experimental results and represents the compressibility for a large range of w_L. On the other hand, the NCRS in the B&F model is less well adapted to the high values of w_L, but remains valid for representing compressibility in the case of medium and low values of w_L. The NCRS also has the advantage of being simpler to use because it is based on two current ‘nature parameters’: the Atterberg's limits w_L and w_P.

Acknowledgements

This work was conducted in the framework of the CLAROM Deepwater sediments project – on the site B Stacor core from the Gulf of Guinea. Project partners were: IFP, IFREMER, Fugro-France, Saipem-SA, Stolt Offshore, Technip, and Total.

Bibliographie

[1] B.A. Baudet; W.L. Ho On the behaviour of deep-ocean sediments, Geotechnique, Volume 54 (2004) no. 9, pp. 571-580

[2] K. Been; G.C. Sills Self-weight consolidation of soft soils: An experimental and theoretical study, Geotechnique, Volume 31 (1981) no. 4, pp. 519-535

[3] J. Biarez, J.-L. Favre, Parameters filing and statistical analysis of data in soils mechanics, in: Proc. 2nd Int. Conf. Appl. Stat. Prob., Vol. 2, Aix-la-Chapelle, 1975, p. 249.

[4] J. Biarez, J.-L. Favre, Statistical estimation and extrapolation from observations. Rapport à la session spécialisée n° 6, C. R. du 9^e congrès Int. Méc. Sols Trav. Fond, Tokyo, 1977, Vol. 3, p. 505.

[5] J. Biarez; P.-Y. Hicher Elementary mechanics of soils behaviour. Saturated remoulded soils, Rotterdam/Brookfield, Balkema, 1994

[6] J. Biarez, T. Fayad, S. Taillez, A. Gomes Correia, E. Flavigny, D. Branque, Argiles et craies du tunnel sous la Manche – grains sans et avec colle, in: 2^nd Int. Conf. on Hard Soil and Soft Rocks, Naples, Italy, Vol. 1, Balkema, Rotterdam, 1998, pp. 437–445.

[7] J. Blewett; W.J. Mccarter; T.M. Chrisp; G. Starrs Monitoring sedimentation of a clay slurry, Geotechnique, Volume 51 (2001) no. 8, pp. 723-728

[8] J.B. Burland On the compressibility and shear strength of natural clays, Geotechnique, Volume 40 (1990) no. 3, pp. 329-378

[9] F. Cotecchia; R.J. Chandler The influence of structure on the pre-failure behaviour of a natural clay, Geotechnique, Volume 47 (1997) no. 3, pp. 523-544

[10] F. Cotecchia; R.J. Chandler A general framework for the mechanical behaviour of clay, Geotechnique, Volume 50 (2000) no. 4, pp. 431-447

[11] J.-L. Favre, Table ronde sur les corrélations de paramètres en mécanique des sols, École centrale Paris, Vol. II, ed. Biarez, 1972, pp. 1–51.

[12] J.-L. Favre, Milieu continu et milieu discontinu – Mesure statistique indirecte des paramètres rhéologiques et approche probabiliste de la sécurité, thèse d’État ès sciences physiques, université Pierre-et-Marie-Curie–Paris-6, 1980.

[13] J.-L. Favre; M. Hattab Properties of deepwater marine clays, Rev. Fr. Geotech. (2006) no. 116, pp. 3-13

[14] M. Hattab; P.-Y. Hicher Dilating behaviour of overconsolidated clay, Soils and Foundations, Volume 44 (2004) no. 4, pp. 27-40

[15] S. Leroueil; F. Tavenas; F. Brucy; P. La Rochelle; M. Roy Behaviour of destructuted natural clays, Proc. Am. Soc. Civ. Eng., Volume 105 (1979) no. GT6, pp. 759-778

[16] A.W. Skempton Notes on compressibility of clays, Q. J. Geol. Soc., Volume 100 (1944), pp. 119-135

[17] A.W. Skempton; R.D. Northey The sensitivity of clays, Geotechnique, Volume 3 (1952) no. 1, pp. 30-53

Commentaires - Politique