1 Introduction
In eukaryotic cells, membranes of different organelles are functionally connected to each other via vesicular transport. Formation of transport vesicles from invaginated buds of the plasma membrane is called endocytosis [1]. In clathrin-mediated vesiculation, vesicle formation starts with the assembly on the donor membrane of a highly organized ‘coat’ of clathrins [2], which acts both to shape the membrane into a bud and to select cargo proteins [3–7]. The mechanism by which an invaginated clathrin-coated bud is converted to a vesicle (scission) involves the action of a cytoplasmic GTPase protein called dynamin [6,8]. Dynamins form oligomeric rings at the neck of deeply invaginated membrane buds and induce scission [9,10]. How exactly dynamin is recruited and how the scission actually occurs remains unclear [11,12]. In this paper we propose that dynamin recruitment by clathrin coats could be driven by long-ranged physical forces mediated by the membrane curvature elasticity.
Cryo-electron microscopy has recently revealed the detailed structure of the clathrin coats at 21-Å resolution [2]. Clathrin units, also called ‘triskelions’, have a star-like structure with three legs. Initially solubilized into the cytoplasmic fluid, they self-assemble onto the membrane surface into a curved, two-dimensional solid scaffold. The latter is a honeycomb made of hexagons and pentagons (geometrically providing the curvature) the sides of which are built by the overlapping legs of the clathrin triskelions. In the plasma membrane, clathrins usually interact with ‘adaptor’ transmembrane proteins, which also serve to select cargo proteins. However, it has been shown that clathrin coats can readily self-assemble onto protein-free liposomes [13,14].
Dynamin is known to be solubilized in the cytosol as tetramers, and to aggregate in low-salt buffers into rings and spirals [9]. Dynamin also self-assembles onto lipid bilayers, forming helically striated tubules that resemble the necks of invaginated buds (tube diameter ≃50 nm) [10]. Addition of GTP induces morphological changes: either the tubules constrict and break [15], or the dynamin spiral elongates [16]. These findings suggest that the scission of clathrin-coated buds is produced by a mechanochemical action [16,17].
At the earlier stages of the budding process, dynamins already strongly interact with bilayer membranes. Indeed, in vivo studies showed that dynamin binds acidic phospholipids in a way that is essential to its ability to form oligomeric rings on invaginated buds [18–22]. Using a model lipid monolayer spread at the air-water interface, it was shown that dynamins actually penetrate within the acyl region of the membrane lipids [23]. This finding was recently confirmed by the three-dimensional reconstruction of the dynamin structure by cryo-electron microscopy at 20 Å resolution [24]: dynamins form T-shaped dimers the ‘leg’ of which inserts partly into the outer lipid leaflet.
It was long ago suggested [25,26] that particles inserted within bilayers should feel long-range interactions mediated by the elasticity of the membrane. Indeed, a protein penetrating within a bilayer and binding its lipids – such as dynamin – may in general produce a local membrane curvature (see Fig. 1). Because of the very nature of the curvature elasticity of fluid membranes, this deformation relaxes quite slowly away from its source, and the presence of another inserted particle produces an interference implying an interaction energy [25]. This holds as long as the separation between the inclusions is smaller than the characteristic length
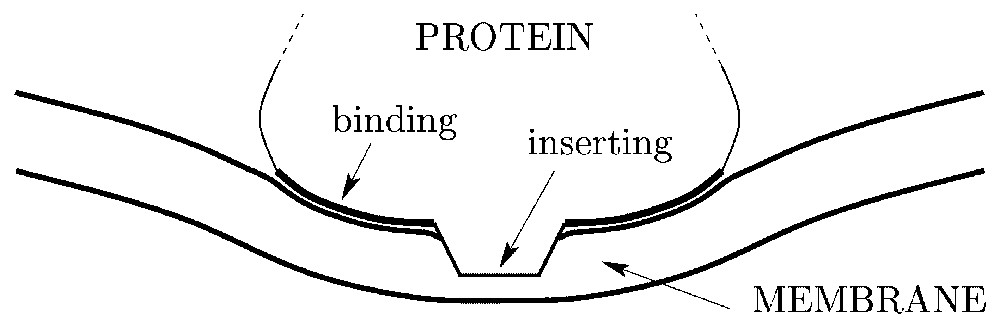
Schematic representation of a cytosol protein partly inserting within a lipid bilayer and inducing a local membrane curvature via a binding region.
The first detailed calculation of the membrane-mediated interaction was performed for two isotropic particles each locally inducing a spherical curvature [29,30]. The interaction was found to be repulsive, proportional to the rigidity κ of the membrane and to the sum of the squares of the imposed curvatures; it decays as R−4, where R is the distance between the particles. The case of anisotropic particles producing non-spherical membrane deformations is even more interesting, since their collective action on the membrane is expected to have nontrivial morphological consequences [31–33]. The local deformation of a membrane actually involves two distinct curvatures, associated with two orthogonal directions (as in a saddle or in a cylinder). Recent calculations showed that the interaction between two anisotropic inclusions is very long-ranged and decays as R−2 [34–36]. It is always attractive at large separations and favors the orientation of the axis of minor curvature along the line joining the particles [35]. Note that these elastic interactions prevail at large separations, since they are of much longer range than other forces, such as van der Waals or screened electrostatic interactions.
2 Model
Among the above informations, let us outline the three points that are essential for our model. (i) Clathrin coats are solid scaffolds that rigidly shape extended parts of the membrane into spherical caps. (ii) Dynamins are solubilized proteins that partly insert within the membrane bilayer. (iii) Inserted membrane hosts that imprint a local membrane curvature interact with long-range forces of elastic origin.
We therefore build the following model. We consider a clathrin-coated bud as being a membrane patch bearing a constant, fixed spherical curvature. Technically, we shall build the bud by placing a large number of point-like spherical curvature sources at the vertex of a hexagonal lattice (see Fig. 2). Within the present formalism, this is the simplest way to define a rigid, almost non-deformable, spherically curved zone. Since the dynamins will not penetrate the bud, we expect that our results will not depend on whether the bud is geometrically enforced (which would be conceptually simpler but technically harder here) or built by an inclusion array. Note that the shape of the neck around the clathrin bud will result from the minimization of the total energy, and will therefore not be enforced artificially.
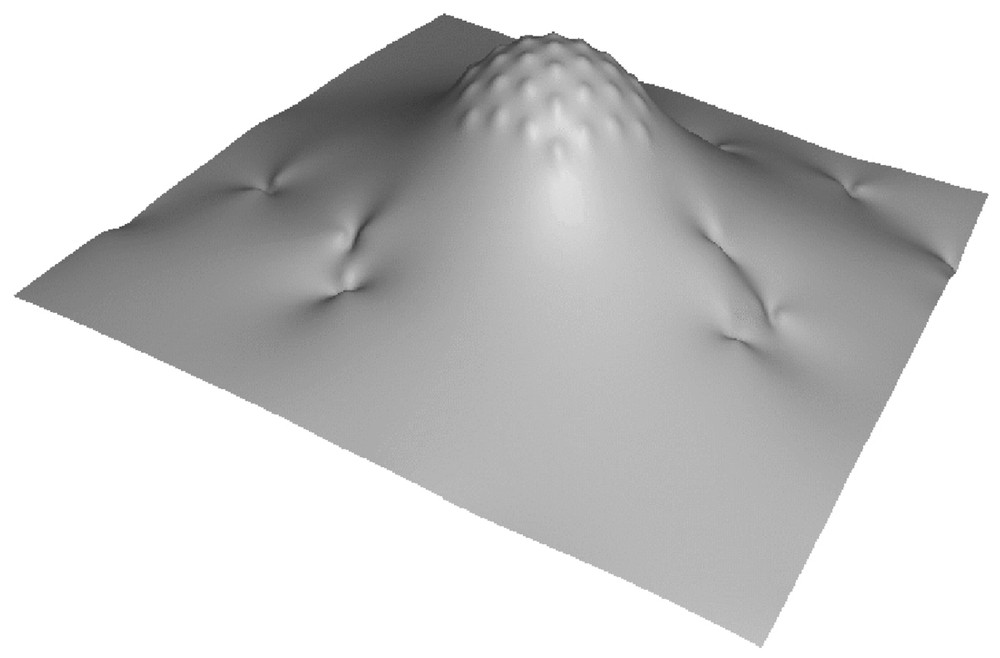
Piece of a model membrane showing a clathrin coated bud and the imprints of inserted dynamins.
Because dynamins partly insert within the membrane and seem to accommodate cylindrical curvature, we model them as sources locally imprinting a cylindrical curvature. We place a large number of such ‘dynamins’ on a membrane in the presence of an artificial bud as described above (Fig. 2), and we study whether the latter will recruit or not the dynamins through elastic long-range forces.
2.1 Long-range elastic interactions between many membrane inclusions
The elastic interaction between N isotropic or anisotropic membrane hosts can be calculated from first principles [35,37–39]. The membrane is described as a surface which is weakly deformed with respect to a reference plane. Without this assumption, analytical calculations are virtually impossible. An obvious consequence is that we can accurately describe only weakly invaginated buds; nevertheless, we expect that our results will hold qualitatively for strongly invaginated buds. The membrane hosts are described as point-like sources bearing two curvatures c1 and c2, associated with two orthogonal directions. These values represent the two principal curvatures that the hosts imprint on the membrane through their binding with the membrane lipids (assuming the binding region is itself curved). For instance, a spherical impression corresponds to c1/c2=1, a cylindrical impression corresponds to c1/c2=0, and a saddle-like impression corresponds to c1/c2=−1. This over-simplified model actually contains the essential ingredients responsible for the long-range elastic interactions between membrane inclusions: for protein hosts of a size comparable to the thickness of the membrane, it yields accurate interactions for separations as small as about three times the particles size. Note that the curvature actually impressed by a particle could be affected by the vicinity of another inclusion, we shall however neglect this effect for the sake of simplicity (strong binding hypothesis).
The point-like curvature sources describing the membrane hosts diffuse and rotate within the fluid membrane, because of the forces and torques exerted by the other membrane hosts and of the thermal agitation
2.2 Pairwise interactions
Before studying the collective interaction between model dynamins and clathrin coats, let us describe how point-like spherical and cylindrical sources interact pairwise (in the absence of membrane tension, as discussed in Section 1).
The membrane distortion produced by two inclusions modeled as point-like spherical curvature sources is shown in Fig. 3a. Each inclusion appears as a small spherical cap away from which the membrane relaxes to a flat shape. As evidenced by the plot of the interaction energy (see Fig. 3a), such spherical inclusions repel one another. Calling κ the bending rigidity of the membrane, a the thickness of the membrane (which is comparable to the size of the inclusions), c the curvature set by the inclusions and R their separation, our calculation gives (see Appendix A):
| (1) |
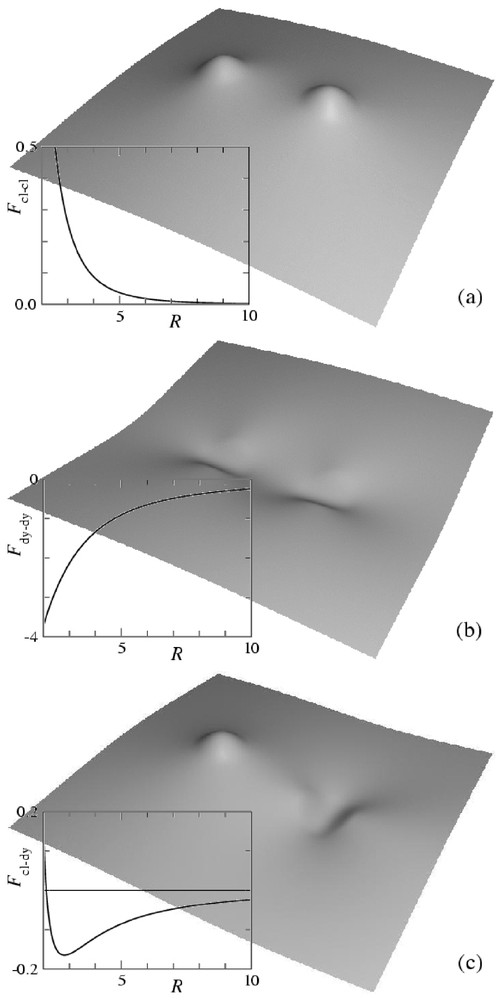
Shape of a membrane distorted by two inclusions imprinting local curvatures and interaction energy as a function of separation. Distances are rescaled by the membrane thickness a and energies by
Shape of a membrane distorted by two inclusions imprinting local curvatures and interaction energy as a function of separation. Distances are rescaled by the membrane thickness a and energies by
The membrane distortion produced by two inclusions modeled as point-like cylindrical curvature sources is shown in Fig. 3b. Each inclusion appears as a small cylindrical cap away from which the membrane relaxes to a flat shape. The interaction between two such hosts depends on their relative orientation. The minimum energy is found when the axes of the cylinders are parallel to the line joining the inclusions. As evidenced by the plot of the interaction energy (see Fig. 3b), the interaction is then attractive. It therefore turns out that two such hosts produce a weaker membrane deformation when they are close to one another than when they are far apart. As described in [35], when their curvature is strong enough, such inclusions tend to aggregate and to form linear oligomers. Their asymptotic interaction energy is given by
| (2) |
Finally, we show in Fig. 3c the membrane distortion produced by the interaction between a spherical source and a cylindrical one. The latter is oriented in the direction that minimizes the energy. As evidenced by the plot of Fig. 3c, the interaction is attractive at large separations and repulsive at short separations, with a stable minimum configuration at a finite distance. Calling c the curvature set by the cylindrical inclusion and c′ the one set by the spherical inclusion, our calculations give the asymptotic interaction
| (3) |
3 Collective interactions between model dynamins and clathrin buds
As described in Section 2, we build a model clathrin-coated bud by placing in a membrane Ncl point-like spherical inclusions of curvature ccl on a hexagonal array with lattice constant b. Here, we have chosen Ncl=37 and
To study the collective behavior of this system under the action of the multibody elastic interactions (see Section 2.1) and of thermal agitation, we perform a Monte Carlo simulation. The details of the simulations are given in Appendix B. To prevent unphysical divergences of the elastic interaction energy, it is necessary to introduce a hardcore steric repulsion preventing two inclusions to approach closer than a distance d. Since the size of the inclusions imprints is of the order of the membrane thickness a, we have chosen
The results of the Monte Carlo simulations are summarized in the phase diagram of Fig. 4, in terms of the curvatures ccl and cdy of the clathrins and dynamins imprints, respectively. Here, we have chosen to span ccl between 0 to
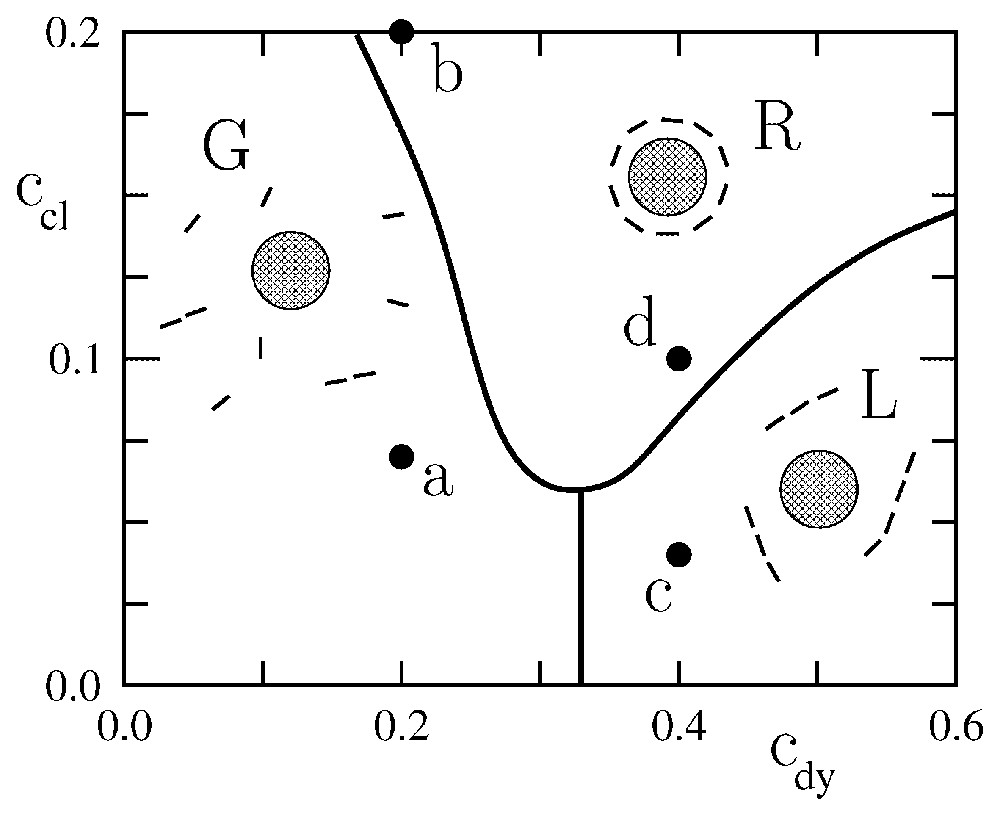
Phase diagram representing the typical equilibrium configurations of a system of model dynamins in the vicinity of a curved clathrin scaffold. cdy and ccl are the curvatures associated with the dynamins and clathrins, respectively, in units of the inverse membrane thickness a. In region G, the dynamins form a ‘gas’ non interacting with the clathrin scaffold. In region L, the dynamins form a system of linear oligomers non interacting with the clathrin scaffold. In region R, the dynamins form a ring around the clathrin scaffold, which is reminiscent of real endocytosis. Masquer
Phase diagram representing the typical equilibrium configurations of a system of model dynamins in the vicinity of a curved clathrin scaffold. cdy and ccl are the curvatures associated with the dynamins and clathrins, respectively, in units of the ... Lire la suite
The phase diagram displays three regimes (see Fig. 4): a state in which the dynamins are disordered in a gas-like fashion (G), a state in which the dynamins form linear oligomers that do not interact with the clathrin bud (L), and a state in which the dynamins form a ring around the clathrin bud (R). In region (R), due to the shallowness of the dynamin imprints, the system is disordered by thermal agitation. Increasing the curvature of the dynamin imprints increases the elastic attraction between the dynamins (see Fig. 3b) and leads to the formation of linear oligomers (L). These oligomers wrap around the clathrin bud when the latter is sufficiently developed (R). Typical snapshots corresponding to the four points (a), (b), (c), (d) in Fig. 4 are shown in Fig. 5. Note that in Fig. 5b the dynamin collar is rather ‘gaseous’ due to the weakness of the dynamins' imprints, while in Fig. 5d the ring is tight and well ordered. (See also Fig. 6.)
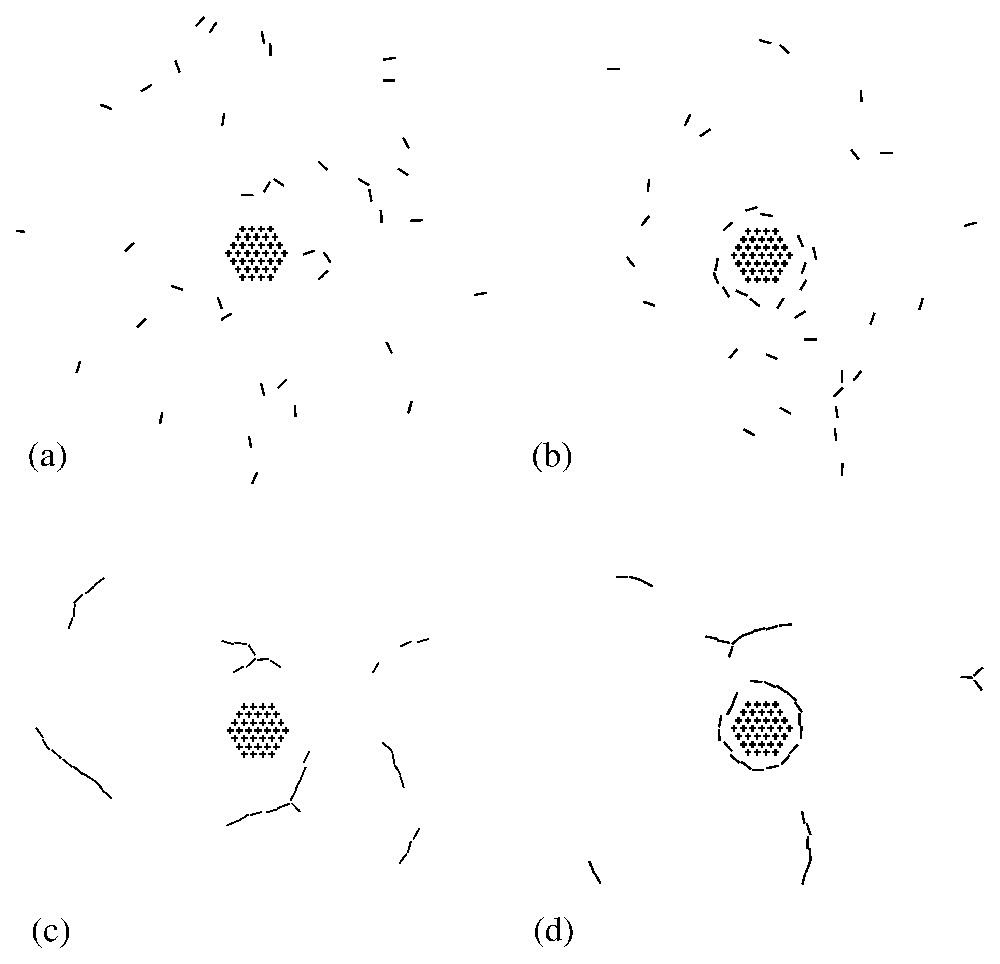
Typical snapshots showing the equilibrium arrangement of model dynamins (bars) in the vicinity of the clathrin scaffold (hexagonal array). The figures (a), (b), (c) and (d) refer to the corresponding points in the phase diagram of Fig. 4.
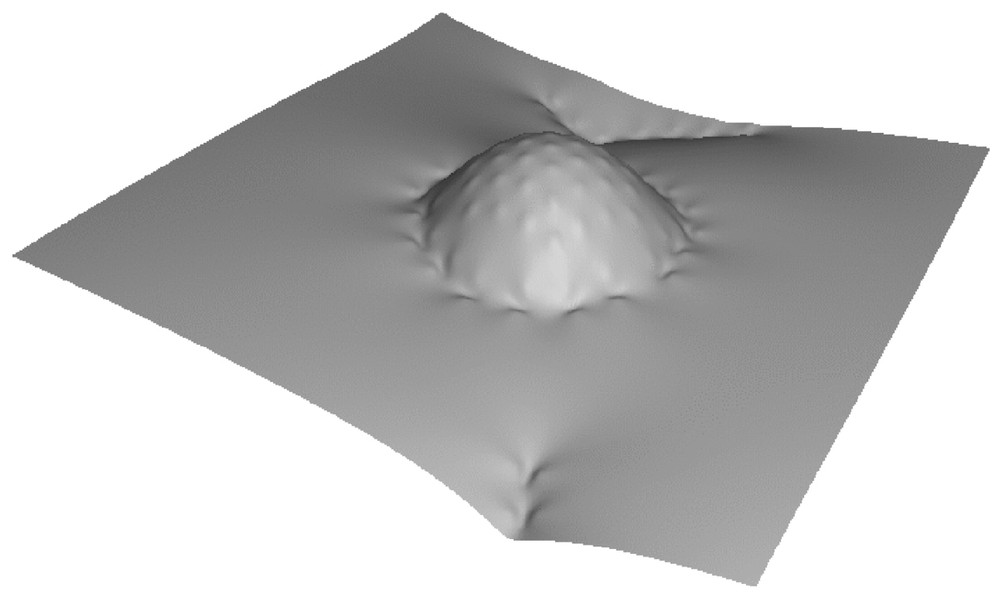
Membrane shape corresponding to point (d) in Figs. 4 and 5, showing the self-assembly of a ring of dynamins around a clathrin bud.
It is difficult within the present paper to discuss the nature of the boundaries between the different ‘phases’ displayed in Fig. 4. Because we are considering a rather small (thermodynamically speaking) number of dynamins, and because they are under the influence of a localized curvature field, the transition lines between the various regions of Fig. 4 are actually broad. Attempting to describe them as first- or second-order transition lines should not be meaningfull.
4 Conclusion
In this paper, we have shown that if membrane-inserted dynamins produce cylindrical imprints and if the latter are sufficiently curved, then the resulting long-range forces mediated by the membrane elasticity are strong enough to overcome Brownian motion and bring them into a collar around the neck of a clathrin bud. Of course, simple diffusion could also bring dynamins around clathrin buds, and their binding into a ring could be the result of specific biochemical interactions. However, if a cylindrical imprint can speed up this process, then evolution may have selected it.
To test this model, one might look experimentally for linear oligomers of dynamins (see Fig. 5c). However, since ‘gaseous’ rings are also possible (see Fig. 5b), the existence of such linear aggregates may not be necessary. It would be more interesting to directly check, e.g., by cryo-electron microscopy, the shape of the dynamin region that penetrates within the membrane.
Finally, note that our model is obviously over-simplified: (i) many other integral proteins float around dynamins, (ii) dynamins may interact with various lipidic domains within the bilayer, (iii) the membrane may have a spontaneous curvature due to its asymmetry, (iv) fluctuations are not only thermal but also active, and hence could be larger than we estimate, and (v) large values of the membrane tension could shorten the range at which the dynamins are recruited (see Section 1). Nonetheless, we believe that our model correctly captures the effects of the anisotropic elastic interactions.
Acknowledgements
We acknowledge fruitful discussions with R. Bruinsma, F. Jülicher, F. Képès, J.-M. Delosme, B. Goud, P. Chavrier, V. Norris, and J.-M. Valleton.
Appendix A Many-body interactions between point-like curvature sources
Let us outline the derivation of the interaction between N anisotropic point-like sources that locally imprint a curvature on the membrane. As explained in the text, such constraints modelize a wide class of membrane inclusions, including transmembrane and cytosol proteins partly inserted within the membrane.
For small deformations u(x,y) with respect to the (x,y) plane, the free energy associated with the curvature elasticity of a membrane is given by [42]:
| (A.1) |
Determining the shape of the membrane in the presence of inclusions at positions rα imprinting local curvatures requires minimizing the elastic energy (A.1) with local constraints on the membrane curvature tensor. In the small deformation limit, the elements of the latter are given by the second spatial derivatives of the membrane shape: u,xx(r), u,xy(r) and u,yy(r). Introducing
| (A.2) |
| (A.3) |
| (A.4) |
| (A.5) |
We introduce a column matrix K containing the values of the 3N constraints
| (A.6) |
| (A.7) |
| (A.8) |
| (A.9) |
| (A.10) |
| (A.11) |
| (A.12) |
| (A.13) |
When α=β,
| (A.14) |
| (A.15) |
| (A.16) |
As an illustration, let us consider the case of two identical isotropic inclusions, each prescribing the curvature c. Then
| (A.17) |
| (A.18) |
When many inclusions are present, the matrix M and its inverse, which determines the interaction energy through equation (A.12), can be easily calculated numerically once the positions of the inclusions are defined.
Appendix B Monte Carlo simulations
The Monte Carlo simulation that we perform employs the standard Metropolis algorithm [43]. For given positions and orientations of the particles representing the dynamins, the energy is numerically calculated from equation (A.12). At each Monte Carlo step, we perform a Metropolis move consisting in either a translation or a rotation of one arbitrarily chosen dynamin particle. The amplitude of the moves is adjusted in order to keep an average acceptance rate of 50%. We confine the dynamins inside a circular box (of radius


