Version française abrégée
Introduction
L'Antarctique, d'une surface de 14 millions de kilomètres carrés, est recouverte d'une couche de glace d'épaisseur moyenne de 2200 m, ce qui représente 90% des glaces terrestres ou une couche de 70 m du niveau des océans en équivalent eau. Chaque année, environ 2200 gigatonnes (approximativement 2200 km3) de neige tombe sur le continent : cette neige s'enfonce, se transforme en glace et est rejetée plusieurs milliers plus tard, voire plusieurs centaines de milliers d'années plus tard, vers l'océan [33]. Cet échange de masse annuel représente l'équivalent de 6 mm du niveau des océans, de telle sorte qu'un léger déséquilibre peut se sentir en termes de variations du niveau des océans. La partie ouest du continent (Fig. 1) est d'un volume dix fois moindre que la partie est, mais repose sur un socle très enfoncé sous le niveau de la mer, dont le flux géothermique est beaucoup plus fort que pour la partie est [26,45]. Certains auteurs soupçonnent que l'Antarctique de l'Ouest est potentiellement instable. Il a d'ailleurs connu dans le passé des fluctuations de masse beaucoup plus forte que la partie est.
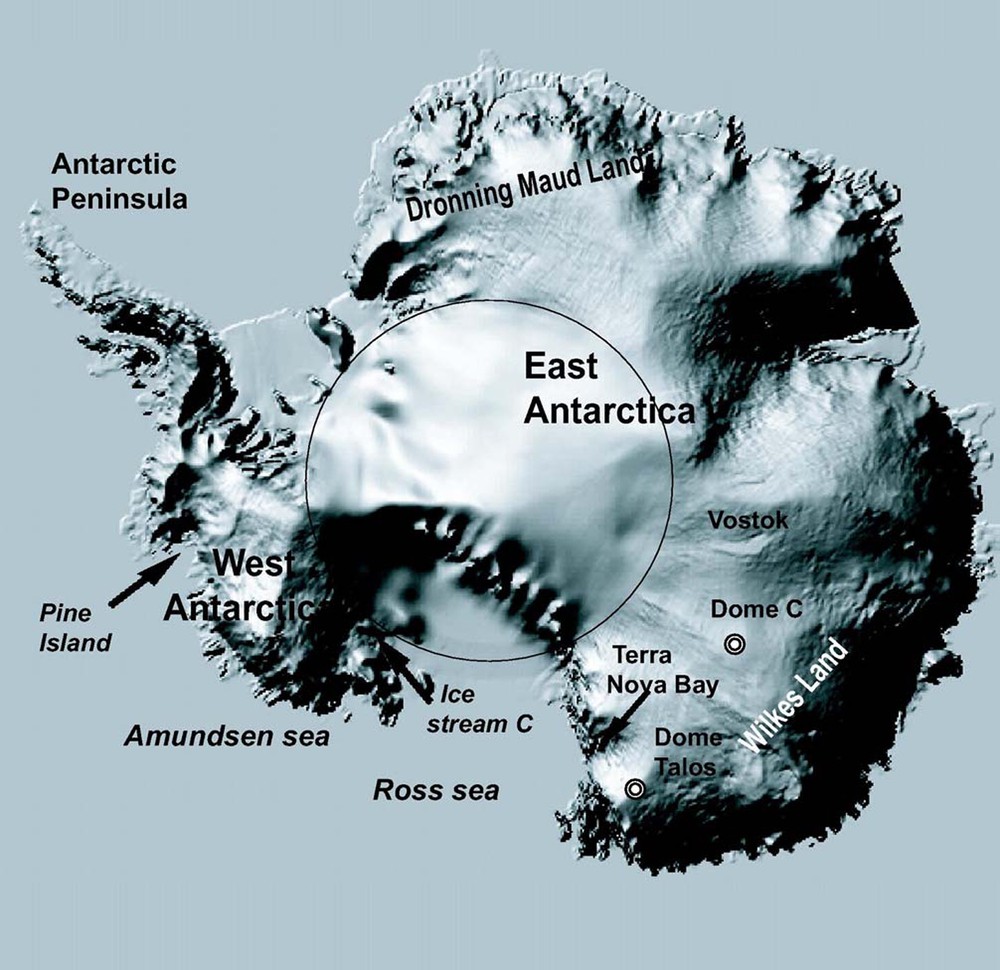
Topography of the Antarctic ice sheet derived from the ERS-1 geodetic mission [38]. The circle shows the ERS inclination and the main locations mentioned in the text are shown.
Topographie de la calotte Antarctique déduite de la mission géodésique d'ERS 1 [38]. L'inclinaison d'ERS ainsi que les principaux lieux mentionnés dans le texte sont indiqués.
Estimer le bilan de masse peut consister à comparer la quantité de neige qui tombe et la quantité de glace rejetée dans l'océan (voir Section 2). On peut aussi directement estimer l'effet intégré sur la topographie, soit en mesurant les variations de volume par altimétrie, soit en comparant les taux d'accumulation de neige avec les vitesses d'enfouissement (voir Section 3). On peut aussi modéliser les précipitations neigeuses et l'écoulement de la glace, afin de prédire l'évolution future (voir Section 4).
De nombreuses raisons rendent ce travail délicat : le continent est grand et difficile d'accès, les mesures in situ y sont rares, les mesures satellites sont peu validées et peu étalonnées. Les processus physiques, climatologiques et dynamiques sont complexes et encore peu connus. Les conditions limites ne sont pas suffisamment connues. La variabilité spatio-temporelle est extrêmement forte. Enfin, les temps de réaction face à une perturbation climatique sont très variés (voir Fig. 2), très faibles en ce qui concerne les précipitations, extrêmement longs en ce qui concerne l'écoulement de la glace. Ainsi, l'Antarctique n'est toujours pas stabilisé depuis le réchauffement climatique du début de l'Holocène.
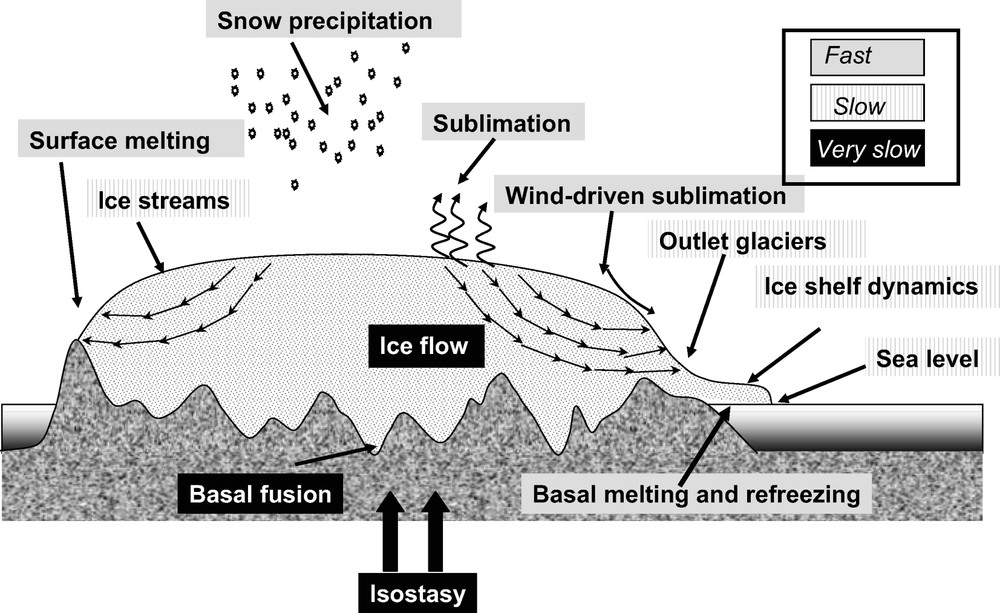
Ice-sheet mechanism and time-scale outline adapted from [33]. Surface melting, snow precipitation, sublimation, wind-driven sublimation, and basal melting or refreezing are assumed to instantaneously react to climate change. Ice streams, outlet glaciers and ice-shelf dynamics are assumed to react slowly to climate change, meaning between 100 and 1000 yr. On the contrary, ice flow, basal temperature and fusion isostasy take a very long time to react, meaning that the time lag is between 10 000 to 100 000 yr.
Les principaux mécanismes agissant sur la géométrie de l'Antarctique et les différents temps de réaction associés, adapté de [33]. La fonte à la surface, les précipitations de neige, la sublimation ou les phénomènes de fonte–regel sous les plates-formes de glace réagissent pratiquement instantanément à une variation climatique. En revanche, les fleuves de glace, les glaciers émissaires ou la dynamique des plates-formes réagissent un peu plus lentement, environ 100 ou 1000 ans après la perturbation. Quant à l'écoulement de la glace, la température à la base de la calotte ou l'isostasie, le temps de réaction est fort long, de l'ordre de 10 à 100 000 ans.
Analyse des flux
Le flux de neige entrant est estimé sur la totalité d'un bassin et les flux de glace sortant sont estimés aux limites côtières de ce bassin par des mesures simultanées d'épaisseur de glace et de vitesse d'écoulement. La difficulté majeure de cette technique réside dans l'estimation des taux d'accumulation de neige. Des processus atmosphériques complexes, joliment illustrés par la présence de mégadunes récemment découvertes (Fig. 3) [9], redistribuent, sur de grandes distances, la neige tombée. Néanmoins, les résultats de cette méthode [43] suggèrent un certain équilibre de la partie est et un bilan négatif pour la partie ouest .
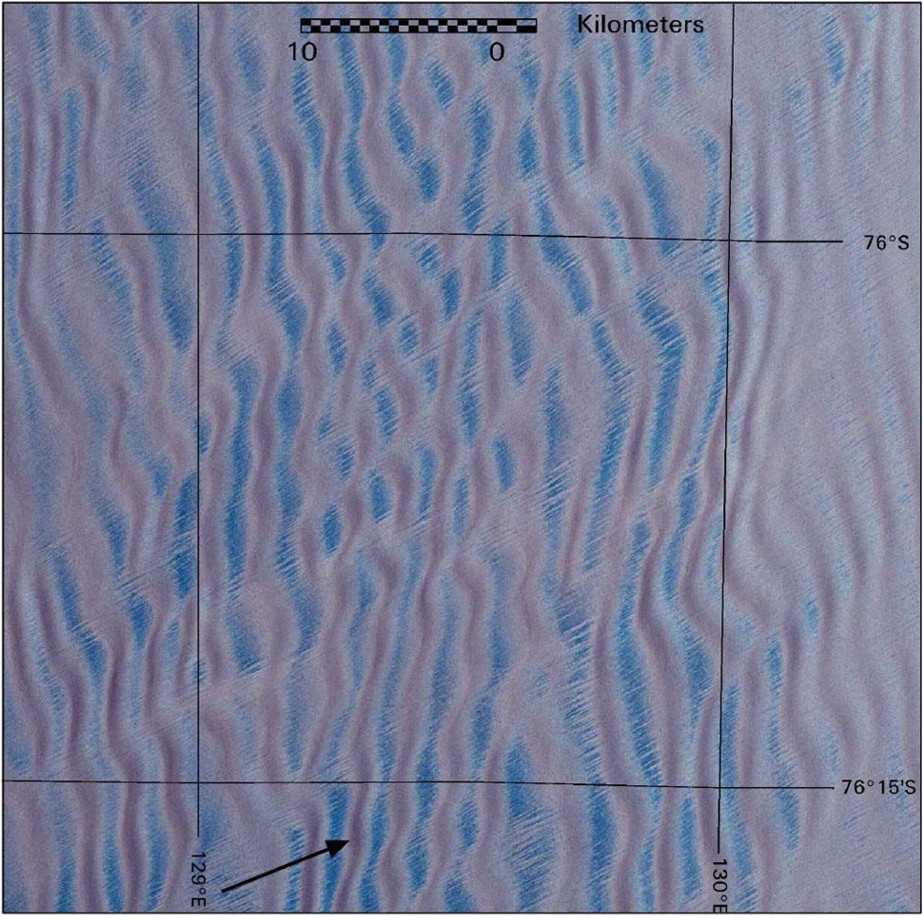
Subscene of Landsat ETM in false colour showing a plateau area with megadunes. The arrow shows the prevailing wind direction. These unexpected structures were recently discovered in a satellite image. They are due to a feedback system between the cryosphere and the atmosphere [6,9].
Scène du satellite Landsat en fausse couleur montrant un champ de « mégadunes » sur le plateau Antarctique. La flèche indique la direction prédominante du vent. Ces structures inattendues ont été découvertes récemment, grâce à des observations par satellite. Elles sont dues à une interaction entre la cryosphère et la neige [6,9].
Suivi intégré
Les altimètres européens ERS1 et 2 survolent depuis 1991 plus de 80% de l'Antarctique. Aux erreurs inhérentes de l'altimétrie (orbite, propagation de l'onde à travers l'atmosphère ou la ionosphère...) il faut rajouter des erreurs spécifiques, à savoir l'erreur causée par la pente de la surface qui décale le point d'impact et celle causée par la pénétration de l'onde radar dans la neige [39]. La Fig. 4, qui montre la différence d'estimation de la topographie entre les deux fréquences différentes de l'altimètre d'Envisat, illustre la pénétration de l'onde dans la neige. L'analyse de six années de données ERS [57] suggère aussi une relative stabilité de la partie ouest et un bilan négatif pour la partie est . Cependant, la comparaison, purement relative, avec des données plus anciennes de Seasat (1978) montre que la partie ouest se déforme légèrement, certaines zones en amont des grands fleuves émissaires semblant encore subir l'effet de la hausse des océans ayant eu lieu au début de l'Holocène [34].
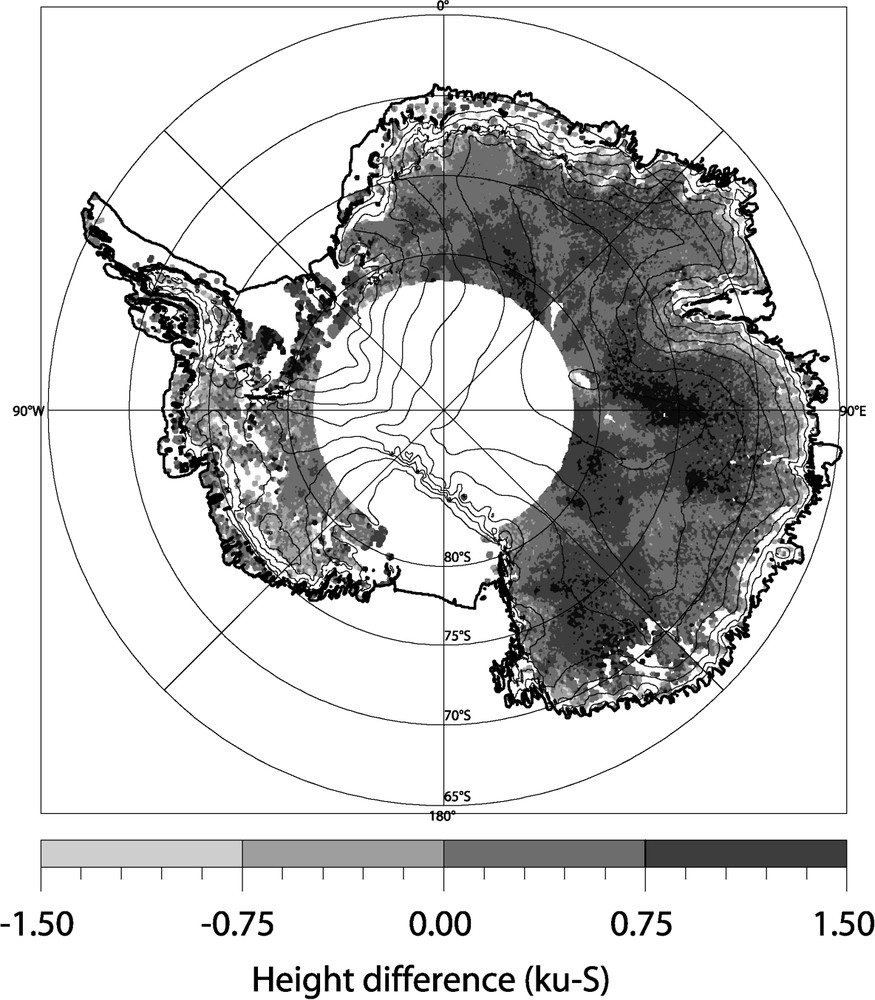
Difference of altimetric height as seen by both antenna of the dual-frequency altimeter of Envisat (one in Ku band at 13.6 GHz, the other in S band at 3.6 GHz) [25]. The difference is due to the difference between penetrations of both radar waves within the dry and cold snowpack. This gives an idea of how large the penetration error could be. Since the penetration error is due to the snowpack characteristics, it could change with time and induce an erroneous signal on an altimetry trend. This error is probably the dominant one in the interior of the Antarctic.
Différence d'appréhension de la topographie, vue par les deux fréquences de l'altimètre d'Envisat (l'une en bande ku ou 13,6 GHz, l'autre en bande S à 3,6 GHz) [25]. La différence est due à la différence de pénétration des ondes radar dans les deux fréquences. La figure illustre l'importance de cette erreur. Par ailleurs, l'erreur due à la pénétration n'est pas reproductible, puisqu'elle dépend des conditions du manteau neigeux (température, taille de grains de neige...) ; elle peut donc entraîner un biais sur l'estimation des tendances altimétriques.
La pause de coffee can ou de « boîte à café » à plusieurs dizaines de mètres de profondeur, dont on mesure la vitesse d'enfouissement, permet de mesurer directement l'équilibre de la surface par comparaison avec les taux d'accumulation locaux. Plusieurs de ces « boîtes à café » ont été installées entre dôme C, dôme Talos et Terra Nova Bay (voir Fig. 1). Dans le secteur étudié, la surface semble être en équilibre, mais l'incertitude est très forte, la forte variabilité spatiale des taux d'accumulation de neige étant le problème majeur qui se pose pour l'interprétation de ces observations.
Modélisation
La difficulté majeure pour la modélisation des calottes polaires, en dehors de la complexité des mécanismes atmosphériques et dynamiques et de la mauvaise connaissance de la majorité des paramètres, réside dans la diversité des temps de réaction (Fig. 2). Il faut, en effet, non seulement connaître les valeurs des forçages actuels, mais aussi des forçages passés, taux d'accumulation de neige, limites côtières, température... Un état donné simulé n'est représentatif et stationnaire qu'après une modélisation de plusieurs centaines de milliers d'années [44]. Il est d'usage de discerner le signal lié aux altérations climatiques actuelles (positif ?) du signal résiduel dynamique lié au réchauffement du début de l'Holocène (négatif ?). Les incertitudes pour le signal actuel viennent essentiellement de la discordance entre les modèles prévoyant un réchauffement austral et les données suggérant un refroidissement. Celles pour le signal résiduel viennent, pour une bonne part, de la manière de prendre en compte, dans les modèles, l'effet des grandes plates-formes de glace qui bordent le continent. Le consensus actuel veut que l'on assiste encore quelque temps à une très légère diminution du volume de glace consécutive aux effets résiduels, avant que l'augmentation des taux d'accumulation causée par un réchauffement potentiel de la région australe ne vienne inverser la tendance [21]. Les discordances entre modèles et scénarios climatiques adoptés n'affectent essentiellement que le moment où la tendance s'inverse.
Enfin, l'Antarctique est tellement inerte qu'il ne répond que lentement à des fluctuations climatiques rapides. L'effet cumulatif sur la calotte de la variabilité des taux d'accumulation de neige peut être comparé à une marche au hasard, c'est-à-dire à l'addition de petites perturbations aléatoires intégrées [31,34], où l'on peut s'éloigner fortement de la position initiale, sans raison physique déterministe. On montre qu'une variabilité annuelle de 25% de la valeur moyenne a une chance sur cinq de générer un signal supérieur à 10% des taux d'accumulation sur plusieurs décennies (Fig. 5), ce qui correspond à une évolution du niveau de la mer de 0,65 mm an−1.
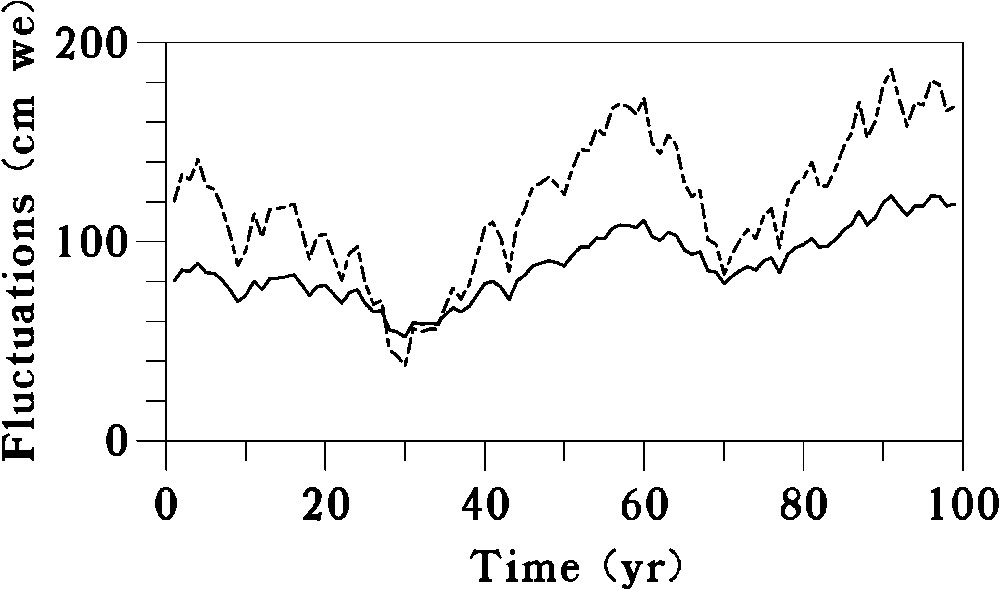
Fluctuations of ice mass (in bold) and of ice elevation (shown by dotted line) due to short-scale random fluctuations of 25% of the mean accumulation rate (i.e., 16.6 cm yr−1). The process is initialised for 1000 years before the time t=0 of the plot. Note that in one place a 1-m increase of the topography can occur over 30 yr, without any climatic significance.
Fluctuations locales en terme de masse (en gras, autrement dit avec une densification instantanée) et d'altitude (en pointillé, autrement dit avec un taux de densification moyen), causées par des fluctuations aléatoires de 25% du taux d'accumulation moyen en Antarctique (c'est-a-dire 16,6 cm an−1). Le processus stochastique a été initialisé durant 1000 ans avant l'expérience. Remarquer que l'on peut observer une élévation moyenne de 1 m en 30 ans par ce processus, sans aucun lien avec les conditions climatiques.
Synthèse et conclusion
En dépit des incertitudes fortes, les deux moyens d'observations du bilan de masse de l'Antarctique (comparaison des flux entrants et sortants ou mesure intégrée des effets sur la surface) montrent une relative stabilité de la partie est et une légère diminution de la partie ouest, correspondant à une élévation du niveau de la mer de 0,15 à 0,20 mm an−1. Cette perte de masse est partiellement due au retrait des lignes d'ancrage de la glace dans la zone de Pine Island (Fig. 1), causée par un réchauffement local. Cette perte pourrait être théoriquement compensée par une augmentation des taux d'accumulation de neige, entraînée par un réchauffement de l'atmosphère polaire austral, mais ceci n'est en aucun cas observé à l'heure actuelle.
1 Introduction
The mass balance of the Antarctic ice sheet is one of the sources of uncertainty about the sea-level rise and it is possible that this will be even truer in the future [29,55]. Moreover, calculations with global climate models (GCMs) indicate that warming in the polar areas will be enhanced by a factor of 2 or 3. One of the consequences of such an increase in polar warming could be a sea-level rise due to melting of the Greenland and Antarctic ice sheets.
This paper focuses on the mass balance of the Antarctic ice sheet (Fig. 1). With a surface area of 14 million km2 and an average ice thickness of 2200 m, it accounts for 90% of the terrestrial ice, which would raise the sea level by 70 m if it melted. Each year, about 2200 gigatonnes of snow fall on the Antarctic ice sheet and sink toward deeper layers, where it slowly changes into ice. The ice then flows toward the coast, where the same quantity of ice is rejected to the ocean much later (Fig. 2). This annual balance corresponds to 6.5 mm of sea level, so that a slight imbalance may have an impact on sea-level change.
The behaviour of the West Antarctic ice sheet (WAIS), which would lead to a sea-level rise of 6 m if it melted, is more critical than that of the eastern part because its bedrock is largely below the sea level and because, as demonstrated in recent works, its geothermal flux is high, inducing warmer basal temperature than in the eastern part [26,45]. This western part is thus suspected to be unstable and likely to suffer a large collapse, especially during warm periods. Finally, geological data indicate that the WAIS extended far away from its current position during the last interglacial period, unlike the eastern part, which appears to be very stable.
The mass balance problem can be easily understood with a simplified mass conservation equation:
| (1) |
This equation states that imbalance between input mass (input ice flow and accumulation) and output (output flow) is counterbalanced by the change in height. The actual mass balance can thus be estimated either by measuring the difference between output and input (the component method, see Section 2) or by surveying the ice volume (left hand of Eq. (1), the integrated method, see Section 3). Temporal changes of the input and output mass can also be modelled in order to predict future changes (see Section 4).
Both snow accumulation and ice flow processes are strongly temperature dependent, but with different time lags. Warming of air temperature is supposed to rapidly increase snow precipitation. On the contrary, changes in air temperature slowly modify the ice temperature and hence also the rate of deformation when the temperature change reaches a depth at which significant deformation occurs. This last process takes a very long time, more than several thousand years. (See Fig. 2 for the different time scales on which ice sheet mechanisms work.)
It is very difficult to estimate mass balance for the Antarctica ice sheet, for many reasons:
- – this huge continent is the coldest, highest, driest and windiest continent on Earth. In situ measurements are still very sparse, and satellite remote sensing techniques are affected by the lack of validation, the penetration of the radar wave within the snowpack and the high variability of the surface roughness so that a lot of geophysical parameters are still difficult to evaluate. For instance, as will be seen in Section 2.1, one of the most pertinent climate parameters, the accumulation rate, has a very large uncertainty, leading to an equivalent error in the global sea-level change of more than ;
- – the physical processes are complex and not well known. Physical processes linking climate and snow precipitation are still being investigated. The parameters of the rheological law linking ice velocity to the basal shear stress, surface slope and ice temperature get a very large set of values [1,19], which means that there is great discrepancy in velocity estimations. Also, much of the ice is transported from the interior to the coast via fast-flowing ice streams. These streams dynamics are strongly controlled not only by poorly-known bed conditions, but also by basal temperature, water content, tills, etc.;
- – the boundary conditions are not fully known. Most of the ice is evacuated through floating ice shelves, which means that the transition from grounded to floating ice, the grounding line, which determines the exact boundary of the ice sheet, is frequently not well located. Furthermore, the bedrock topography and ice thickness that are two of the relevant parameters for modelling ice dynamics are not known over huge sectors of a few tens of thousands of square kilometres [27];
- – due to the large thermal inertia of ice, the present state of the ice sheet is a result of its past history over several climatic cycles. Consequently knowledge of past climate forcing is an important constraint;
- – as for any component of the global climate, the Antarctic climate has large space and time variability. For instance, the annual precipitation variability is estimated at about 25% of the mean value. The large relaxation time of the ice sheet induces a low-frequency response in this random fluctuation [30,40];
- – finally, the snow densification process is also a limitation. The relation between ice volume change, as monitored by altimetry or topography surveys, and ice mass change which is directly related to sea-level change and monitored by gravity mission, requires knowledge of the snow density profile [37].
No claim is made here to an exhaustive review of Antarctica mass balance studies, but an attempt has been made to consider different tools and results. The article is thus structured around a discussion of these different tools, namely: (1) the comparison between mass input and mass output, or the ‘component survey’, (2) the integrated measurement of the volume either through satellite remote sensing or from in situ measurements, (3) the ice sheet dynamics and heuristic or stochastic modelling based on climate forcing.
2 The component survey
The component (or flux) approach to mass balance consists of algebraically summing mass input, i.e. net mass balance at the surface and the mass output of solid ice from the ice sheet.
2.1 Input measurements
Snow accumulation or surface mass balance on the Antarctic Plateau is the sum of precipitation, sublimation/deposition and wind-blown snow. Its estimation by compilation and interpolation work [16,54] has indicated that surface mass balance estimates tend to converge towards a common value, suggesting a residual error of less than 10% for the Antarctic ice sheet (equivalent to a sea-level rise of ). However, ITASE research has indicated a high variability of surface mass balance and also that single core, stake or snow pit measurements are not representative of geographical/environmental characteristics of the site. The new data collected in the ITASE framework or associated with projects for deep drilling surveys (Dome C, DML, Siple Dome, US West Antarctica, Law Dome, Dome Fuji, etc.) show systematic biases with respect to previous compilations of up to 65% [8,28]. Most of the data for East Antarctica used for the snow surface compilation [16,54] were collected during the 1960s, using snow pit stratigraphy, which generally overestimates accumulation rates [31]. In addition, there are large gaps in data coverage, due to the very sparse distribution of in situ measurements (1860 accumulation data points for about 12 million square kilometres, i.e. one data point every 6500 km2), particularly in East Antarctica.
Moreover, satellite image analysis enables the discovery of unexpected surface features as shown in Fig. 3 (megadune, wide glazed surface, etc.) due to a feedback system between the cryosphere and the atmosphere [6,9]. Field observations have shown that the interaction of surface wind with subtle variations of surface slope along the wind direction has a considerable impact on the spatial distribution of snow on short and long spatial scales [10]. Wind-driven ablation greatly affects the surface mass balance. Our idea of a flat ice sheet surface and homogeneous snow accumulation variability over the Antarctic ice sheet has radically altered. Most snow variability has previously been linked to the redistribution process (erosion/deposition) leading to dune/sastrugi formation, but detailed field surveys have pointed out that the redistribution process is a local effect that has a strong impact on the annual scale variability of accumulation (i.e. noise). The high variability of surface mass balance is due to ablation processes driven by katabatic winds (wind scouring, surface and blowing sublimation); only megadunes and some occasional transversal dunes show depositional features [8].
The re-analysis of ECMWF (ERA) surface mass balance for the interior of the continent also yields significantly lower values than that provided by surface mass balance compilation [49]. Systematic negative ‘biases’ between Atmospheric General Circulation Models and surface mass balance compilations have been observed in regions devoid of field observations or characterised by strong winds [15].
2.2 Output measurements
Remote sensing surveys by satellite and/or airborne are ideal tools [13,41] for evaluating the ice discharge. This is done by measuring ice thickness at the grounding line (with airborne Radio-Echo sounding, or freeboard of floating ice from satellite altimetry) and by measuring ice velocities (with InSAR or/and tracking methods). These observations of the Antarctic Ice Sheet in the last decade have identified recent unexpected changes, which are much more dynamic than had previously been thought. Some glaciers are found to accelerate strongly, for instance the Pine Island Glacier on the West Antarctic ice sheet is accelerating by 45 m yr−1 [43], while others have been found to decelerate or even stop.
Rignot and Thomas [43] reviewed the mass budget of 33 Antarctic glaciers, using remote sensing measurements to determine the ice discharge at the grounding line with the most recent snow accumulation compilations [16,54]. The authors pointed out that the West Antarctic Ice Sheet is thinning in the west with a loss of 72 km3 yr in the Admundsen Sea sector and that it is thickening in the north with a slight gain of 33 km3 yr−1 in the Ross Sea sector. The main reason for the positive balance of this region is the stoppage of ice stream C about 150 years ago and the continued slowing-down of the Whillians ice stream [43]. Nevertheless, the West Antarctic ice sheet is probably thinning on the whole . On the contrary, the mass imbalance of the East Antarctic Ice Sheet, estimated with 20 surveyed glaciers, is likely to be small , and even its sign cannot be determined yet.
2.3 Limitation of the method
Mass balance results are subject to large uncertainties both on the distribution of accumulation rate over the basin and on the basin delimitation itself. Indeed, recent results [7,8,50] have pointed out that the ice divide has migrated or has been encroached upon by other basin systems, also on a relatively short timescale of a few hundred years. The current coastal ice flux does thus not correspond to the basin delimitation, as estimated with the current topography. Fricker et al. [14] also pointed out that mass balance results are subject to large uncertainties in the distribution of accumulation over the basin, such that current estimates of Antarctic accumulation rates are unsuitable for this type of study; this is the most significant gap in current mass balance estimates. New data have shown that there is currently no proven method of interpolating across the huge gaps remaining between existing snow accumulation data. Acquisitions of more and more accurate field determinations of the Antarctic surface mass balance are crucial.
Accurate knowledge of the surface mass balance is thus the most significant gap in current estimates of mass balance.
3 Volume survey
A volume or elevation change survey (i.e. to determine values for the left term of Eq. (1)) is a direct way of estimating mass balance. This can be either done on the global scale with satellite altimetry or locally with submergence velocity estimation. Both methods are great tools for surveying elevation change, but the first one requires ground-based measurements to check the satellite data and study the process involved in elevation change, while the second one only provides a local measurement where markers have been installed.
3.1 Satellite altimetry
Since the launch of ERS-1 in 1992, the Antarctica ice sheet can be surveyed with a radar altimeter to the north of 81.5°S, which is the satellite inclination. However, a lot of corrections have to be applied to take into account the tracker lag, the orbit restitution, the atmospheric delay, the slope-induced error or the solid tide (see for instance [35]). Moreover, due to the penetration of the waveform within the snow pack, temporal changes in surface scattering strongly affect the radar height measurements [24]. This error is very critical because the snow pack and the surface characteristics may change from the meteorological scale to the long-term scale. However, the surface topography of Antarctica, one of the most relevant parameters for investigating ice sheet dynamics and mass balance, can be mapped with an accuracy of less than a metre in the central part of the continent, north of 82°S [39].
The ERS data were used to survey Antarctica from 1992 to 1996 [57]. The 5-year rate of elevation change was mapped with a resolution of . In order to decrease the noise, estimated to be 4 cm yr−1 over a cell, the data were averaged over a correlation length of 200 km. The imbalance of West Antarctica was found to be , whereas the imbalance of the East part was found to be . A more recent study with 11 years of ERS data suggests now a positive imbalance for the eastern part of [5]. This signal is correlated with an increase of precipitation and corresponds to of decrease of 0.12 mm yr−1 of the sea level. Nevertheless, neither changes in snowpack characteristics nor snow densification are taken into account in this study, so that this estimation is an upper limit of the mass change.
An attempt was made to compare the observations of the Seasat altimeter, obtained in 1978 with those of ERS-1, launched in 1992 over the common survey area in the Wilkes Land north of 72°S, in order to take advantage of the long time between the two missions [34]. Unfortunately no cross-calibration has ever been performed between the two altimeters, so that only the relative difference between the two topographical surveys can be determined. The geographical changes in height during the 14 years separating the missions have been mapped via an inverse technique that allows us to take the whole altimetric error budget into account. Also the error due to changes in snow characteristics over time was corrected for after the inversion. In a belt between 70°S and 72°S and between 150°E and 80°E, a precision of better than 40 cm, e.g. 2.8 cm yr−1 for the trend was found for the surface elevation change. The mapped change in height between the two missions suggests a rise of the western part of the sector (150 E–140 E) and of the high-altitude region, corresponding respectively to a positive imbalance of 20% and 25%.
The major altimetry error is probably due to the surface slope. This error is not reproducible from one track to the other because the across-track orbit fluctuations induce large noise. This error strongly increases with the surface slope, which means that it is not possible to survey the coastal areas that are probably more sensitive to climate change. The second error, dominant in the central part, is due to the variation of the snowpack characteristics over all temporal time scales. The recent launch of the Envisat satellite now makes it possible, for the first time ever, to survey the ice caps with a dual-frequency altimeter in order to better understand the penetration error. The difference in height as seen by the two altimeters working with different radar frequencies could be as much as 2 m (see Fig. 4 and [25]) suggesting a relation between penetration and frequency. The survey of change in this difference may allow detecting modification in penetration. Finally, snow densification may have created a bias between mass change (what we need) and volume change (what we are looking for with altimetry). Densification rate may change with environmental conditions so that another error may happen.
3.2 Coffee can
Surface elevation change can also be measured in situ by land surveys using the submergence velocity system. The simultaneous knowledge of the vertical velocity and of the accumulation rate then gives the change in elevation directly. This system is a field approach, using repeated geodetic surveys (GPS–DORIS), to determine the local rate of ice elevation change [17,20]. The submergence velocity system is based on GPS surveys of markers anchored some tens of metres deep in the firn. The markers have been frozen to the borehole base using water and are connected to the surface by steel cables that cannot stretch. These vertical velocity measurements are then compared with the rate of snow accumulation, determined from the different sources (stake farm, fin core or snow radar profiling).
Submergence velocity systems were installed during a transect Terra Nova Bay–Dome C (seven sites) and in Wilkes Land (D66-Talos Dome, eight sites) in 1998–1999 and during the 2001–02 ITASE traverse. A first repetition of these measurements along the Terra Nova Bay-Dome C transect was performed and elaborated using the GIPSY-OASIS II software [56]. In this study, the horizontal position accuracy is about , and the vertical accuracy is around (with uncertainties at 95% confidence level). In an analysis by Vittuari et al [56], Tambora's marker (1816) was used based on nssSO4 core stratigraphy. Different authors [17,18,20] have pointed out that errors in submergence ice velocity measurements are dominated by temporal variability in snow accumulation rates. On the contrary, Frezzotti et al. [12] pointed out that the spatial variability of snow accumulation at the kilometre scale, derived from snow-radar and cores, is one order of magnitude higher than its temporal variability (20–30%) at the century scale. At the submerged pole sites, snow accumulation rates derived with different time periods (four years, stake farm; 40 years, tritium marker level; 180 years, Tambora marker) differ significantly due to the horizontal ice displacement and to the high spatial variability of snow accumulation correlated with a wind-driven sublimation process.
The calculated rates of thickness change were all quite small and the formal uncertainties were close to the estimated thickness changes. Analysis of submergence velocity using different methods and time scales yielded different results. The sites with high snow accumulation variability () showed thinning, whereas the sites with accumulation variability showed thickening or balance value. Analysis of submergence velocity in areas with high spatial variability of snow accumulation is a complicated task. This is because processes induced by spatial variability of snow accumulation that occur mainly in porous snow (depth hoar) under wind crust such as densification, and metamorphic processes (highly recrystallised firn, permeability, grain size, snow porosity), may also be reasons why elevation change is misinterpreted. It is important to consider aeolian processes when selecting optimum sites for the submergence velocity system, because slope variations of even a few metres per kilometre have a significant impact on winds and on the snow accumulation process. As a consequence, a lack of information on local conditions can lead to an erroneous definition of climatic conditions based on the interpretation of snow accumulation and submergence velocity alone. According to our observations, the sites with high standard deviation of spatial variability in snow accumulation are not useful sources of information on temporal variations in snow accumulation and also submergence velocity, because it is very difficult or impossible to interpret the data when the snow originated under different snow accumulation conditions. However, the result seems to indicate steady-state conditions in this part of East Antarctica, but due to the very slow submergence velocity (a few cm yr−1) and the low accumulation rate (from 100 to 30 kg m2 yr−1), the uncertainty is high.
4 Ice sheet modelling
Few authors have attempted to model mass balance of the ice sheet according to different climate warming scenarios, in order to predict their future. A review of such models can be found in [53]. The model of Huybrechts and de Wolde [21] is one of the most recent and complete ones. Their dynamic and ablation models were used by the Intergovernmental Panel on Climate Change (IPCC) to derive the sea-level projections described in the third assessment report [3]. These models generate ice sheet geometry for a given set of changes in sea level, surface temperature and net surface balance (precipitation minus ablation).
4.1 Modelling the net surface mass balance
As already mentioned, short-term mass balance changes are due to the net surface mass balance. Until now, the usual assumption has been that precipitation changes are related to the actual precipitation amount weighted by change in temperature, according to the saturation vapour pressure law of Clausius–Clapeyron.
The difficulty and the uncertainty inherent in this method are due to the estimation of the current temperature change, of the current average accumulation rate and to the parameterization of the relation between temperature and precipitation changes. The relation is derived from ice core data by comparing past temperature variations with past accumulation variations [4]. Precipitation increase is thus estimated at between 4 and 10% per degree of warming.
Note that the fraction of precipitation on the grounded ice that is returned to the atmosphere through sublimation in Antarctica is not negligible [7] and that the sublimation process is not explicitly included in numerical weather prediction and general circulation models [15]. Moreover, changes in precipitation can also be induced by changes in atmospheric circulation as has been suspected by [23]. In any case, it is clear that the relation between precipitation and climate is not trivial and probably depends on the considered time-scale, climatic period and ice sheet geometry. For all these reasons, the uncertainties on the rate of precipitation increase due to climate warming could be very great [53].
Ablation is deduced from the relation between positive degree days and melt rate or from an energy balance model. However, there are never many positive degree days in Antarctic and these occur only near the coast [48]. Even for the middle warming scenario of Huybrechts and de Wolde [21], the mean ablation in 200 years will be still less than 5% of the total loss.
Finally, both precipitation and melting are parameterized in terms of temperature which is derived from a coupled climate and ocean model. For instance, the forcing used in the Huybrechts and de Wolde model [21] yields a mean increase of the Antarctica surface temperature of between 2 °C (low scenario) to 8 °C (high scenario) within the next 150 years. Depending on different scenarios, the ice sheet thickening corresponds to a decrease in sea level over the next 200 years of between 10 and 30 cm, corresponding to a sea-level change of between −0.5 to . However, in the high scenario case, due to a high melting rate in the marginal zone and warm ice shelves, the trend would be reversed in 500 years.
The last IPCC report used the Huybrechts and de Wolde model to project the evolution of sea level with another climatic scenario. This new estimation gave a greater increase in precipitation.
However these results are questionable. First, an increase in snow precipitation coupled with an increase in temperature and/or wind could increase the surface mass balance in the inner part of East Antarctica alone, while inducing a decrease in surface mass balance in the windy areas that account for 90% of the Antarctic surface [11]. Second, Van der Veen [53] has shown that the observations on which these models are based are not relevant enough and that the models do not capture all relevant physical processes. In particular it is difficult to take into account changes in atmospheric circulation patterns, which play an important role. Third, an analysis of 20 years of microwave emissivity of the Antarctic surface has shown that the cumulative melting surface has decreased by 1.8% yr−1, with a confidence of 1% yr−1, resulting from a mean (at least) summer cooling of the continent [48]. This result is in contradiction with the climate forcing scenario used.
4.2 Background trend: dynamic modelling
The processes controlling the shape of the Antarctic ice sheet are the grounding line migration, the internal structure and the thermo-mechanical coupling between velocity and temperature. The three components of the ice sheet: the grounded ice, the ice streams and the ice shelves, are taken into account. Due to the large inertia of ice, especially of ice internal temperature, modelling of the current background evolution of the ice sheet has to be initialized during several glacial cycles. Inadequate knowledge of past conditions is one of the major limiting factors on confidence in the actual state, with others being the assumption about the dynamical processes, rheological law or ice stream parameterization. Over these timescales, grounding line migration due to sea level change mostly controls ice geometry, while snow accumulation controls ice volume. Due to the long integration time and to the grid size (40 km), the ice streams are not treated individually. Because slower flowing ice contributes slowly to sea-level change, this grid coarsening induces models to respond more slowly than actual ice sheets [2]. The temperature is parameterized with the help of the surface height and the accumulation rate with the help of the temperature. Past accumulation is deduced as previously explained. These models are usually tested from available geological and glaciological field data; see [44].
In Ritz's model [44], the Vostok area is still increasing due to the enhanced accumulation rate, as the warming of the ice has not yet reversed the trend. In the Huybrechts and de Wolde model [21], the retreat of the WAIS takes place between 9000 and 4000 years before the present day. For both models, the Antarctic ice sheet is still reacting to the Holocene warming, which leads to an actual equivalent increase of sea level of 0.4 mm yr−1, mostly due to the retreat of the WAIS groundling line. For the next millennium, the predicted sea level rise is 20 cm, corresponding to a mean rate of 0.2 mm yr−1.
Note that at the century–millennium timescale, the dynamic response of the ice shelves may also play a role. Taking into account the ice shelves' behaviour mostly acts on the timescale where the growth of Antarctica, following a warming is reversed. A warmer ice shelf will deform more rapidly, leading to upslope thinning and acceleration. However, it is very difficult to model ice shelf dynamics and the greatest limitation is temperature parameterization. Another constraint is basal melting above the ice shelf. New observations have revealed that the basal melting rate experienced by large outlet glaciers near their grounding line is one order of magnitude greater than had previously been assumed [13,41]. The ice shelf melting rate is positively correlated with thermal forcing, increasing by 1 m yr−1 for each 0.1 °C rise in ocean temperature. When deep water has direct access to the grounding line, glaciers and ice shelves are vulnerable to ongoing increases in ocean temperature. For instance, acceleration of Admundsen Coast glaciers increased mass flux to ice shelves, while ice shelves have thinned. This suggests that basal melting increases probably due to a penetration of the warm Circumpolar Deep Water [2]. Ocean temperature directly seaward of Antarctic's continental shelf break has risen by ∼0.2 °C over recent decades, which is sufficient to increase basal melting by ∼2 m yr−1 [42].
Finally, it should be noted that some authors argue that grounding line instability has been an important factor in the collapse of palaeo-ice sheets and may play such a role in the future [17]. Titus and Narayanan [47] estimated at 5% the chance of this instability, making a substantial contribution to sea level of up to 16 cm in the next century.
4.3 Stochastic modelling
Throughout this paper, we have seen how large and problematic the snow-accumulation fluctuations are. Another induced effect is that the long relaxation time of the ice sheet induces a low-frequency response to these random fluctuations of snow accumulation. Short-term changes in the volume of ice sheets as analyzed, for instance by radar altimetry or short-term change in the mass of the ice sheets as measured by gravity satellite may not be related to long-term climatic change. Since the time scale of the response is great in comparison to the average human lifetime, the effect of these random fluctuations on sea-level change may be important even if they are not linked to climatic change [30,40,51].
Indeed, the mass conservation equation can be re-written as:
| (2) |
Moreover, when the densification process linking volume and mass is taken into account, the induced trend in elevation, as measured by satellite altimetry, is enhanced [37]. One now has a 30% chance of having an artificial absolute trend greater than 20% of the accumulation rate. The scatter of the induced trends is such that the sign of the ice mass trend may be opposite to the sign of the measured volume trend (see Fig. 5). The impact of this climatic noise increases when the frequency of the variability decreases.
The conclusion is that we need to know the ‘recent-past’ variability in order to eliminate the residual error due to snow densification and accumulation rate fluctuations, above all in areas of high densification, namely in the coastal areas. At least 10 years of data are needed to reduce the induced error by a factor of 6. Longer series do not significantly reduce the error for the surface trend estimation.
Note that this combined effect of fluctuations in snow densification and random snow accumulation rate only affects the interpretation of surface elevation trend. Measurements taken using the coffee-can technique are not affected by the snow densification process.
Other random fluctuations, such as outlet boundary conditions can also affect the shape of the Antarctic ice sheet [36,52]. Indeed, these outlet flow boundary perturbations are transmitted toward the inland ice sheet. The induced inland undulation wavelength and transmission increase with the temporal wavelength of the perturbation, so that short time scale perturbations are strongly filtered [36]. However, because outlet perturbations are poorly quantified, it is difficult to predict their impact on sea-level change. However, an analysis of anomalies in ice sheet topography suggests that in some places, the actual response to a past outlet perturbation can be suspected, which means that the interpretation of local elevation change may be erroneous [36].
5 Synthesis and conclusion
Observations of the Antarctica ice sheet suggest that the East Antarctica ice sheet is nowadays more or less in balance, while the West Antarctica ice sheet exhibits some changes likely to be related to climate change and is in negative balance. For the East Antarctica ice sheet, the component survey method indicates a slight positive imbalance of ( eq. s.l.), while survey by altimetry gives an upper limit of twice this value and the coffee-can technique indicates steady-state conditions in the surveyed sector of East Antarctica. The model finds a slight negative imbalance for this part due to the actual response of the warming of the Holocene, as the signature of this signal was indeed observed with altimetry analysis. For the western part, the component survey suggests a negative imbalance of ( eq. s.l.) and the altimetry survey a negative imbalance of ( eq. s.l.), which may be explained by the retreat of ice shelves. These observations, namely a slight increase of the east part and a significant decrease of the western part, have been recently confirmed by the analysis of 2.5 yr of Grace [32].
Modelling of the Antarctica ice sheet [21] suggests that the current response of the Antarctica ice sheet is dominated by the background trend due to the retreat of the grounding line, leading to a sea-level rise of 0.4 mm yr−1 over the short-time scale (100 yr). This component is again found to be dominant during the following centuries, depending on the climate scenario. Later, the precipitation increase will counterbalance this residual signal, leading to a thickening of the ice sheet and thus a decrease in sea level. Taking into account the low and middle scenarios leads to a decrease in sea level over the next millennium, while the high scenario inverses the trend in 500 years due to the grounding-line retreat. It should be noted that the time when the trend is reversed not only depends on the climatic scenario, but also on the ice shelves modelling, basal melting rate beneath ice shelves being the most critical factor to be estimated.
However, these determinist models do not take into account the stochastic fluctuations of the forcing given the inertia of the ice sheet. For instance, taking random fluctuations of snow accumulation rate into account alone yields a probability of a present-day induced sea level rise of between 0.5 and 1 mm yr−1 over a 30-year time scale at [40].
Both observations and modelling are still not reliable enough. Let us point out some limitations and problems that should be carefully considered.
The more critical factor is probably the surface mass balance, which varies from location to location due to interaction between precipitation and wind driven by slope along wind direction [9,11]. One of the major unexpected discoveries regarding cryosphere/atmosphere interaction was indeed made in the remotest part of the East Antarctic Ice Sheet with the discovery of the megadunes [6,9]. Major gaps in our knowledge of the temporal and spatial variability processes and of the exact relation between climate change and precipitation change prevent us from producing a reliable estimate of current surface mass balance and from predicting its future trend.
From the point of view of ice sheet dynamics, observations of the Antarctic Ice Sheet over the last decade have identified recent unexpected changes, which are much more dynamic than previously thought. Thus our idea of a slowly evolving Antarctic ice sheet is radically changing [22]. However, the models lack some of the physical processes that may explain these unexpected changes. For instance, a limitation on predicting the future with respect to actual measurements and knowledge lies in the effect of ice-shelf retreat on upslope glaciers. It may be argued either that ice-shelf retreat has little effect on glaciers or that the breaking up of the ice-shelf will accelerate. Considerable improvements are also needed, in particular for characterizing fast moving outlet glaciers. The first results with ICEsat on the glaciers of the Ross embayment suggest that we can hope a very good precision with a very fine space resolution [46]. Lastly, due to numerical limitations, small-scale features such as fast glaciers are poorly taken into account, so that ice sheet modelling underestimates rates of changes [2].
The ICEsat satellite, launched in 2003, will enable measuring of ice sheet mass balance, but on a small temporal scale, which is insufficient for inferring long-term trends. Grace, launched in 2002, will give access to change in ice mass. By combining this data with the ERS and Envisat series, uncertainty with respect to ice sheet mass balance will be reduced.
In the future, the launch of several satellites dedicated to the study of the ice sheet may further our understanding of the physical processes acting on ice sheet and of its actual state. Unhappily due to a launch failure, the Cryosat satellite, devoted for the survey of polar regions, is postponed. Carisma mission, a P-band radar designed to sound the ice sheet, will provide us with exact ice thickness and volume, and will detect internal ice layering, which will be of great help for ice-sheet modelling.


