1 Introduction
The field of molecular magnetism [1] continues to depend largely on the use of chelating–bridging polydentate ligands that possess the coordination abilities to direct the formation of discrete high-nuclearity complexes and to assemble precursor building blocks into higher dimensionality systems. Indeed, discrete complexes with a combination of sufficient high-spin ground state (ST) and uni-axial anisotropy (D) have been one of the main areas in this field of research in the past two decades as they may exhibit Single-Molecule Magnet (SMM) properties i.e. slow dynamics of their magnetization [2]. On the other hand, the assembly of magnetic building blocks into extended bi- (2D) or tri-dimensional (3D) coordination networks has been used to obtain classical magnets, in certain cases of high critical temperatures [3]. More recently, one-dimensional (1D) systems have been shown to potentially present slow relaxation of the magnetization [4–9]. In these materials, called single-chain magnets (SCMs) [5], the slow relaxation of magnetization depends on the magnetic correlations (J) along the chain, although a high-spin ground state (ST) and uni-axial anisotropy (D) of each spin unit composing the chain remain essential ingredients to obtain this property [6]. More generally, these compounds are 1D assemblies of spins (metal ions, organic radicals or metal–ion complexes) that can be coupled ferro- [5,7,8] or antiferromagnetically [4]. The latter case corresponds to systems with a non-compensation of spins along the chain (ferrimagnetic or canted-antiferromagnetic arrangements) [4]. In all these cases i.e. SMMs, classical magnets or SCMs, the hexacyanometalate [M(CN)6]n− building block, which acts as a cyanide donor ligand to coordinate another [M′(L)]m+ acceptor complex has been widely used to form a bimetallic assembly, resulting in the synthesis of high TC molecule-based magnets with long-range magnetic ordering [10], SMMs [11], photomagnetic molecular or molecule-based materials [12], etc. On the other hand, Mn(III) Schiff base complexes, [Mn(L)]+ (where L = tetradentate ligand), featuring a high-spin ground state and strong uni-axial anisotropy induced by the Jahn–Teller distortion of Mn(III) ion in an octahedral ligand field, have shown great versatility to obtain SMM [13] and SCM [5] systems. Therefore the combination of hexacyanometalate and Mn(III) Schiff base complex building blocks seems a promising strategy that has already produced a great number of bimetallic complexes with polynuclear [14], 1D [5] and 2D [15] structures with remarkable magnetic behaviors. In our efforts towards the design of new SCMs based on hexacyanometalate, we have isolated a new 2D bimetallic system, [{Mn(saltmen)}4Cr(CN)6]ClO4 (1), obtained by the assembly of [Mn(saltmen)]+ and [Cr(CN)6]3− building blocks (saltmen = N,N′-(1,1,2,2-tetramethylethylene)bis(salicylideneiminato) dianion). We report here its synthesis and crystal structure, together with magnetic studies indicating the presence of a long-range magnetic order at low temperature that will be compared with known related 2D systems.
2 Experimental section
2.1 Materials
All chemicals and solvents used in the synthesis were of reagent grade. The precursor, [Mn2(saltmen)2(H2O)2](ClO4)2, was prepared according to the literature procedure [16]. Caution: perchlorate salts of metal complexes with organic ligands are potentially explosive. Only a small amount of the material should be prepared and it should be handled with extreme care.
2.2 Physical measurements
FTIR spectra were recorded in the range of 400–4000 cm−1 on a Nicolet 750 Magna-IR spectrometer using KBr pellets. Magnetic susceptibility measurements were obtained with the use of a Quantum Design SQUID magnetometer (MPMS-XL). dc measurements were conducted from 1.8 to 300 K and from −70 kOe to 70 kOe. The measurements were performed on a polycrystalline sample. Experimental data were corrected for the sample holder and for the diamagnetic contribution of the sample. Crystallography: X-ray crystallographic data were collected on a Nonius Kappa CCD diffractometer with a graphite-monochromated Mo Kα radiation (λ = 0.71073 Å) at 150 K. A suitable crystal was affixed to the end of a glass fiber using silicone grease and transferred to the goniostat. DENZO-SMN [17] was used for data integration and SCALEPACK [17] corrected data for Lorentz-polarisation effects. The structure was solved by direct methods and refined by a full-matrix least-squares method on F2 using the SHELXTL crystallographic software package [18]. Crystal data of 1 are summarized in (Table 1).
Crystal data of [{Mn(saltmen)}4Cr(CN)6]ClO4
| Empirical formula | C172H176Cl2Cr2Mn8N28O24 |
| Formula weight | 3633.83 |
| Temperature (K) | 150(2) |
| Wavelength | 0.71073 Å |
| Crystal size | 0.22 × 0.20 × 0.07 mm3 |
| Crystal system, space group | Tetragonal, I4/m |
| Unit cell dimensions | a = 18.706(2) Å, α = 90.00° |
| b = 18.706(2) Å, β = 90.00° | |
| c = 28.268(5) Å, γ = 90.00° | |
| Volume | 9891(2) Å3 |
| Z | 2 |
| Absorption coefficient | 0.689 mm−1 |
| F(000) | 3756 |
| Max. and min. transmission | 0.95 and 0.86 |
| Data/restraints/parameters | 3084/34/286 |
| Final R indices [I > 2σ(I)] | R1 = 0.0872, wR2 = 0.2363 |
| R indices (all data) | R1 = 0.1295, wR2 = 0.2725 |
| Goodness-of-fit on F2 | 1.084 |
| Largest diff. peak and hole | 0.783 and −0.455 e Å−3 |
2.3 Synthesis of [{Mn(saltmen)}4Cr(CN)6]ClO4
The synthetic process was carried out in the dark to avoid decomposition of K3[Cr(CN)6]. [Mn2(saltmen)2(H2O)2](ClO4)2 (0.2 mmol, 0.1908 g) in methanol was mixed with K3[Cr(CN)6] (0.2 mmol, 0.0650 g) in H2O. A precipitate formed after about one week. This solid was then dissolved in warm H2O/methanol mixture and the resulting black solution filtered. The filtrate was left undisturbed to produce brown plate-like crystals suitable for X-ray diffraction within a few days. These crystals were collected by filtration, washed with 1:1 (v/v) water/methanol, and dried in air at room temperature. Yield: 0.128 g (35% based on Cr). IR (KBr, cm−1): 3425.3 (br, m), 2981.9 (w), 2141.4 (m), 1602.3 (vs), 1542.2 (vs), 1468.8 (m), 1443.9 (s), 1394.4 (s), 1302.6 (s), 1210.9 (w), 1144.6 (s), 1126.2 (m), 1095.7 (br, s), 958.6 (w), 907.0 (w), 848.0 (w), 798.7 (w), 950.8 (s), 628.0 (s), 534.8 (m), 456.6 (m), 429.4 (w).
3 Results and discussion
3.1 Synthesis and structure of 1
While one-dimensional architectures were targeted in this work, the reactions between hexacyanochromate precursor and [Mn2(saltmen)2(H2O)2](ClO4)2 in various ratios reproductively led to brown crystals of [{Mn(saltmen)}4Cr(CN)6](ClO4) (1) that exhibited an extended 2D network. This result illustrates that a simple stoichiometry-based strategy to control the architecture of a given system is not in most of cases sufficient and requires the use of designed building blocks with coordination sites selectively blocked by polydentate chelating ligands [12a]. Complex 1 consists of pentanuclear [{Mn(saltmen)}4Cr(CN)6]+ unit and a perchlorate counter ion (Fig. 1). Selected bond distances and angles for 1 are listed in Table 2.
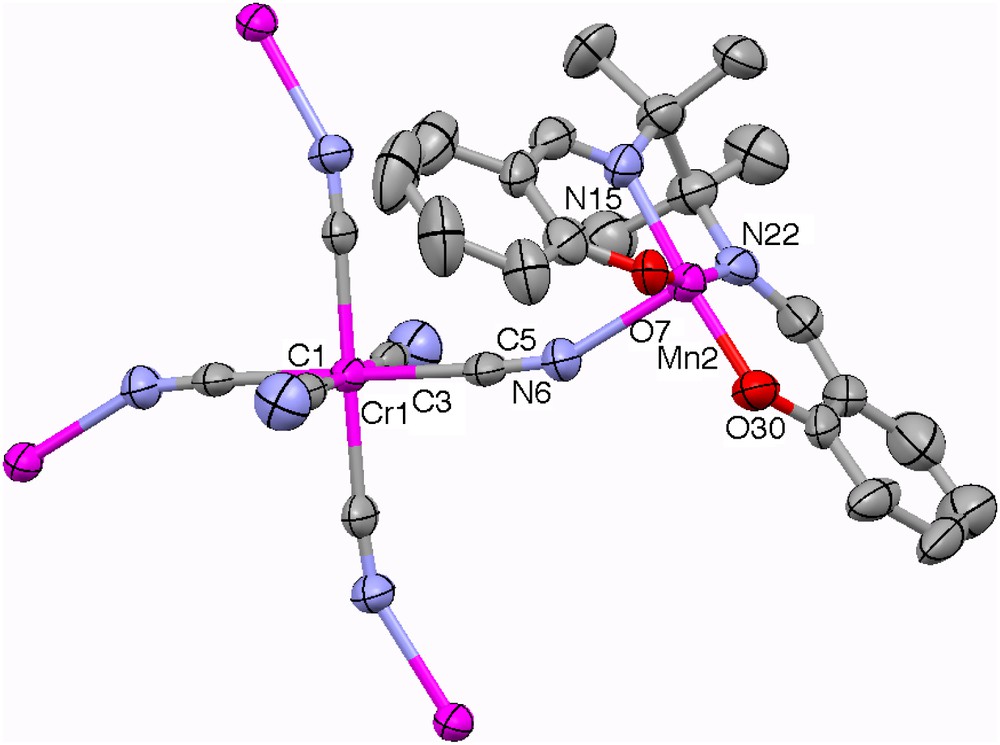
ORTEP view of the pentanuclear unit [{Mn(saltmen)}4Cr(CN)6]+ in 1. For clarity, only one of the four symmetry-equivalent saltmen ligands is depicted, and the perchlorate anion and H atoms are omitted. Ellipsoids are drawn at 50% probability. Colour code: Cr and Mn, purple; N, blue; C, grey; O, red. For interpretation of the references to colour in this figure legend, the reader is referred to the web version of this article.
Selected bond lengths [Å ] and angles [°] in 1
| Cr1–C3 | 1.99(3) | C1–Cr1–C5 | 91.4(3) |
| Cr1–C1 | 2.06(2) | N2–C1–Cr1 | 180 |
| Cr1–C5 | 2.068(9) | N4–C3–Cr1 | 180 |
| Mn2–N22 | 1.954(8) | C5–N6–Mn2 | 156.2(6) |
| Mn2–O7 | 1.876(6) | O7–Mn2–N6 | 92.3(2) |
| Mn2–O30 | 1.882(5) | O30–Mn2–N6 | 93.4(2) |
| Mn2–N15 | 1.980(7) | N22–Mn2–N6 | 98.3(3) |
| Mn2–N6 | 2.205(7) | N15–Mn2–N6 | 89.5(2) |
| C3–Cr1–C1 | 180 | N6–C5–Cr1 | 174.7(7) |
| C3–Cr1–C5 | 88.6(3) |
The CrIII ion in the pentanuclear unit is coordinated via four cyanido bridges to four symmetry-related MnIII ions with the C5–N6–Mn2 bond angle of 156.2(6)°. This geometric parameter is very similar to the one found in the three analogue compounds: [{Mn(saltmen)}4Cr(CN)5(NO)]ClO4·3H2O [19], [{Mn(saltmen)}4{Fe(CN)6}]ClO4·H2O [15a] and [{Mn(saltmen)}4Fe(CN)5(1 − CH3im)]n[ClO4]2n·9nH2O [20] where the corresponding angles are 154.6(8)°, 156.1(10)° and 157.3(7)°, respectively. Each pentanuclear motif is then connected to four other adjacent units via the out-of-plane bi-phenoxo bridges forming the well-known dimeric [Mn(saltmen)]22+ cation [9,16] to give the 2D network structure (Fig. 2). The MnIII ion has a distorted octahedral geometry with equatorial plane occupied by O2N2 donor atoms from tetradentate Schiff base ligand (saltmen), and axial positions occupied by one N atom from cyanide bridge and one phenolate oxygen atom from a [Mn(saltmen)]+ cation of an adjacent pentanuclear unit. Due to the Jahn–Teller distortion of the MnIII ion, its two axial bond lengths, 2.205(7) Å (Mn–Ncyanido) and 2.607 Å (Mn–Ophenoxy), are relatively longer than those of its equatorial bond lengths (between 1.876 Å and 1.890 Å), but comparable to those of [{Mn(saltmen)}4Cr(CN)5(NO)]ClO4·3H2O (2.166(9) Å and 2.629(11) Å), [{Mn(saltmen)}4{Fe(CN)6}]ClO4·H2O (2.19(1) Å and 2.847(9) Å) and [{Mn(saltmen)}4Fe(CN)5(1 − CH3im)](ClO4)2·9H2O (2.197(8) Å and 2.844(8) Å) [15a,19,20]. The Mn⋯Cr distance in the [Mn4Cr] unit is 5.280 Å, while the Mn⋯Mn distance in the Mn dimer is 3.480 Å. As shown in Fig. 3, which presents a view of the stacking of layers in 1, the 2D sheets are well separated by the ClO4− anions.
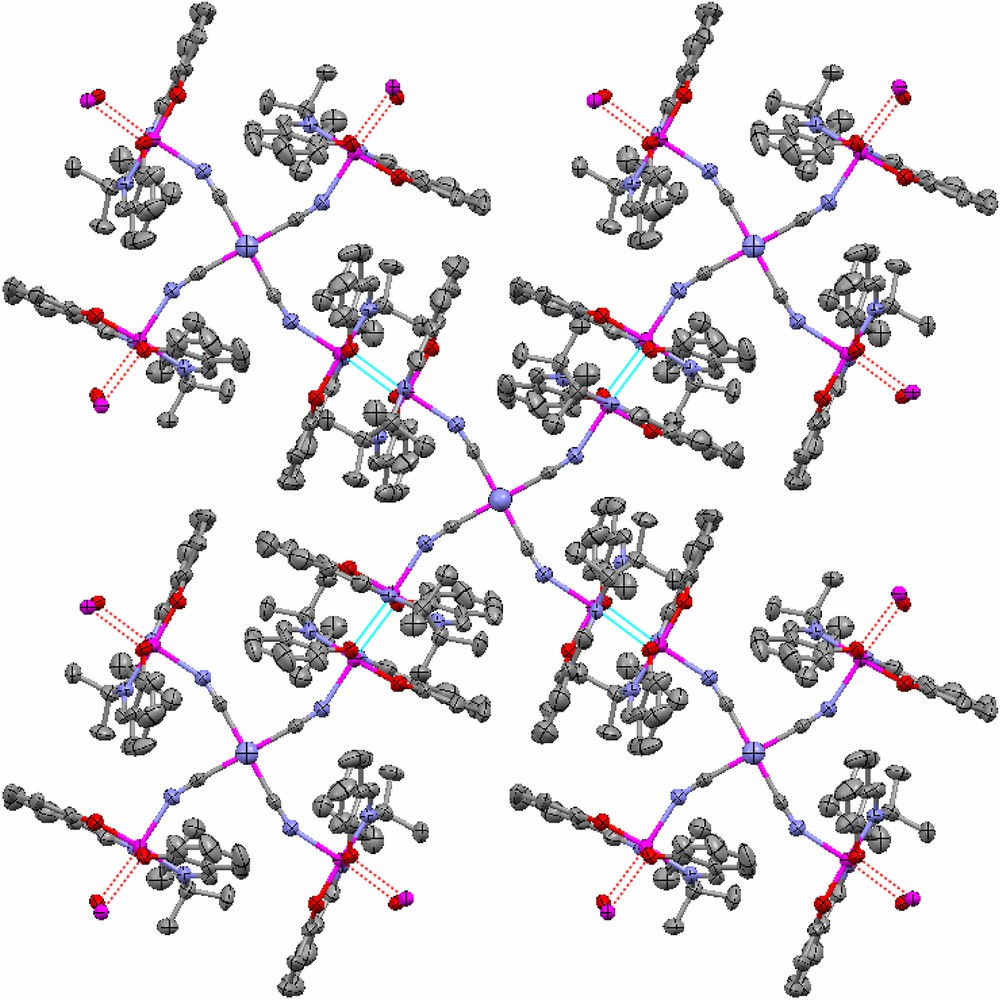
A view along the crystallographic c axis showing the 2D network structure in 1 constructed by out-of-plane double phenoxo bridges (see the dotted blue and red lines) between [Mn(saltmen)]+ ions. Perchlorate anions and H atoms are omitted for clarity. Colour code: Cr and Mn, purple; N, blue; C, grey; O, red. For interpretation of the references to colour in this figure legend, the reader is referred to the web version of this article.
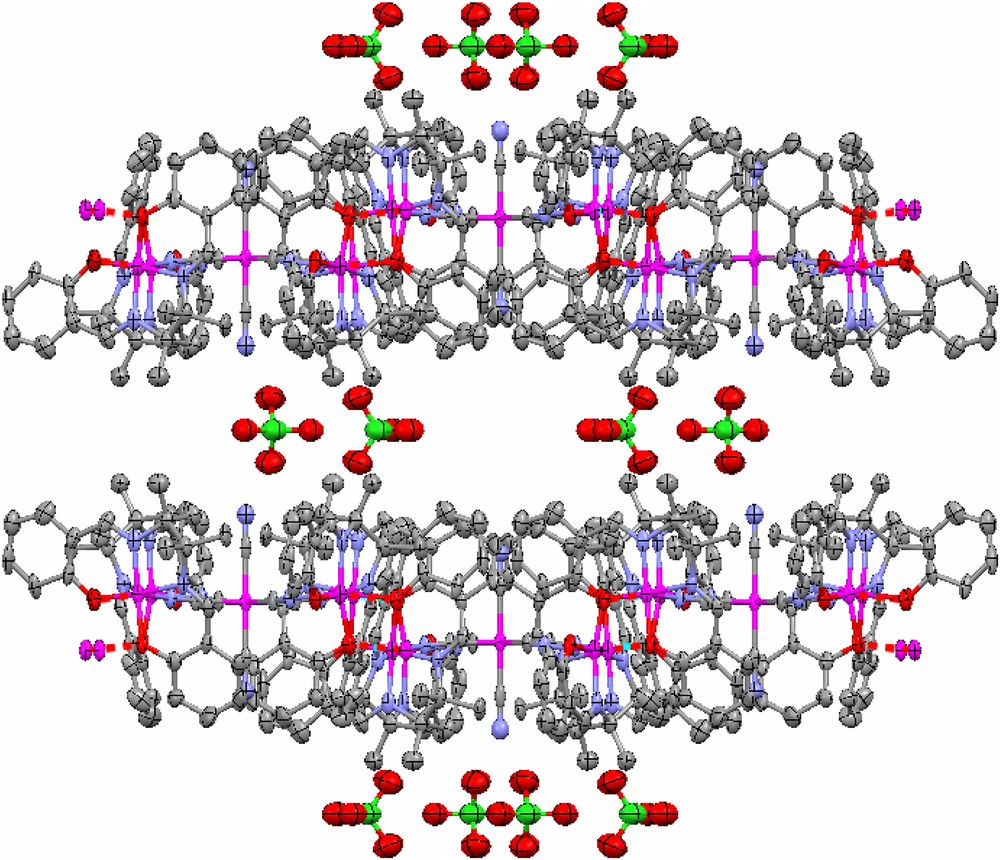
view along the crystallographic a axis showing the interlayer stacking by van der Waals contact. H atoms are omitted for clarity. Colour code: Cr and Mn, purple; N, blue; C, grey; O, red; Cl, green. For interpretation of the references to colour in this figure legend, the reader is referred to the web version of this article.
3.2 Magnetic properties of 1
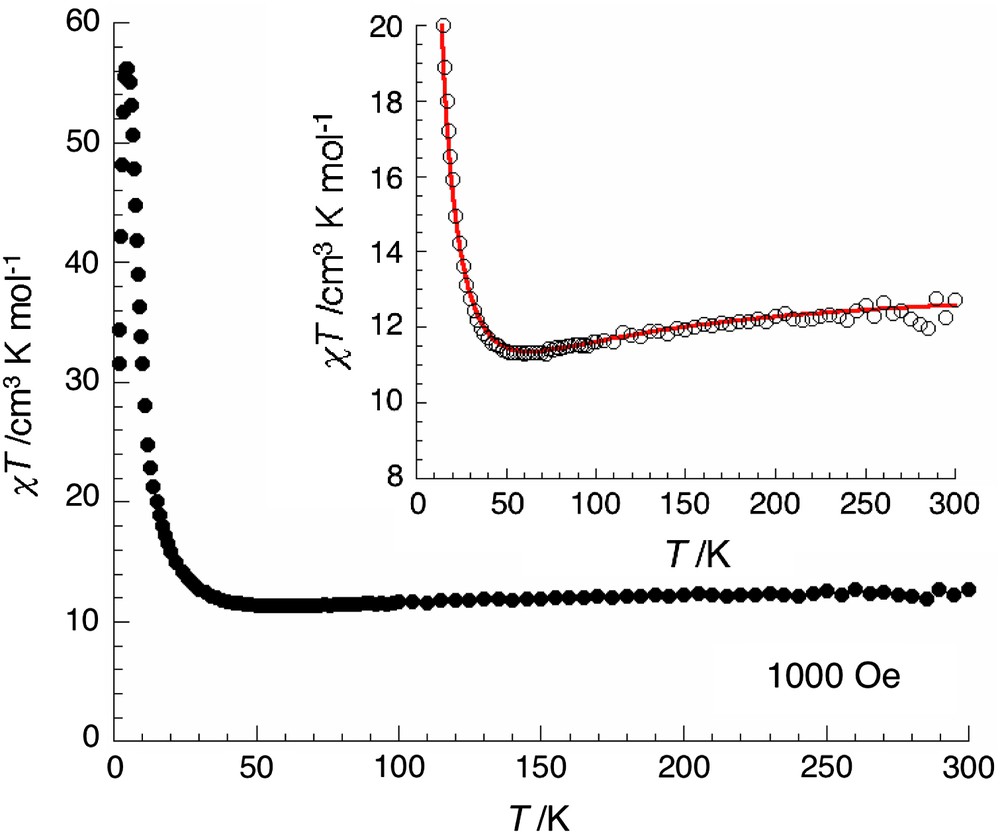
The magnetic susceptibility of 1 was measured in the temperature range of 1.8–300 K under an applied magnetic field of 1000 Oe. The plot of χT vs T is shown in Fig. 4, where χ is the magnetic susceptibility (M/H) per Mn4Cr unit. At room temperature, the χT product is 12.8 cm3 K/mol. As expected when antiferromagnetic interactions are present (vide infra), this value is slightly lower than the spin-only value (13.875 cm3 K/mol with an average g value of 2) for four Mn(III) (S = 2) and one Cr(III) (S = 3/2) magnetically isolated centers. When the temperature is lowered, χT decreases gradually to reach a minimum value of ca. 11.1 cm3 K/mol around 60 K, and then increases smoothly until 30 K before rapidly reaching a maximum value of 56.4 cm3 K/mol at 5 K. Finally at lower temperatures, the χT product abruptly decreases to ca. 31.6 cm3 K/mol at 1.8 K suggesting the presence of additional weaker antiferromagnetic interactions or magnetic anisotropy. Above 5 K, the thermal magnetic behavior indicates the presence of dominating antiferromagnetic interactions (J) as expected between MnIII and CrIII through cyanido bridges [14b,21]. Therefore the magnetic centers in the [{Mn(saltmen)}4Cr(CN)6]+ unit are ferrimagnetically organized. Moreover, in the two-dimensional network, these pentanuclear motifs are expected to be weakly ferromagnetically coupled (J′) through the out-plane double phenoxo bridges between the two adjacent MnIII sites [5,6,9,16]. Considering this topology of the magnetic interactions and also that antiferromagnetic interactions are dominating (|J| ≫ |J′|), an estimation of the exchange couplings has been done, simulating the thermal dependence of the magnetic susceptibility from the following isotropic Heisenberg Hamiltonian:
At low temperature, this coupling scheme leads to an ST = 13/2 spin ground state for the [Mn4Cr] unit confirmed by the M vs H plot, at 1.8 K, shown in Fig. 5, which is almost saturated at 7 T reaching 12.6 μB. Two additional comments should be made on Fig. 5: (i) no inflection point on the M vs H plots or maximum in the dM/dH vs H plots is observed suggesting that the coupling between planes is negligible or ferromagnetic in nature; and (ii) the increase of the magnetization with the applied magnetic field is slow as expected in the presence of magnetic anisotropy. Indeed, this last remark is well in agreement with the presence of Mn(III) metal ions that usually possess magnetic anisotropy.
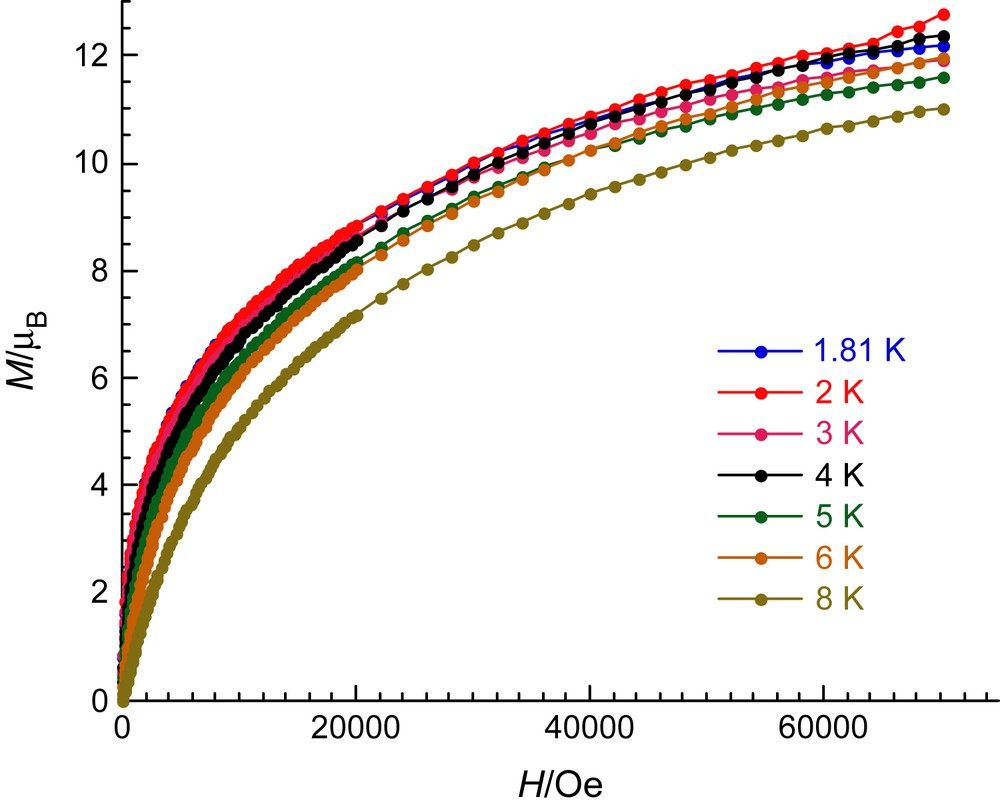
Field dependence of magnetization in the field range of 0–70 000 Oe and between 1.8 and 8 K.
Interestingly, the magnetization exhibits at 1.8 K a hysteresis loop with a coercive field of about 20 Oe (Fig. 6). This behavior was still observed up to 3 K as confirmed by the field-cooled (FC) and the zero-field-cooled (ZFC) susceptibility vs T data obtained at 50 Oe that separate around 3 K (see inset of Fig. 6).
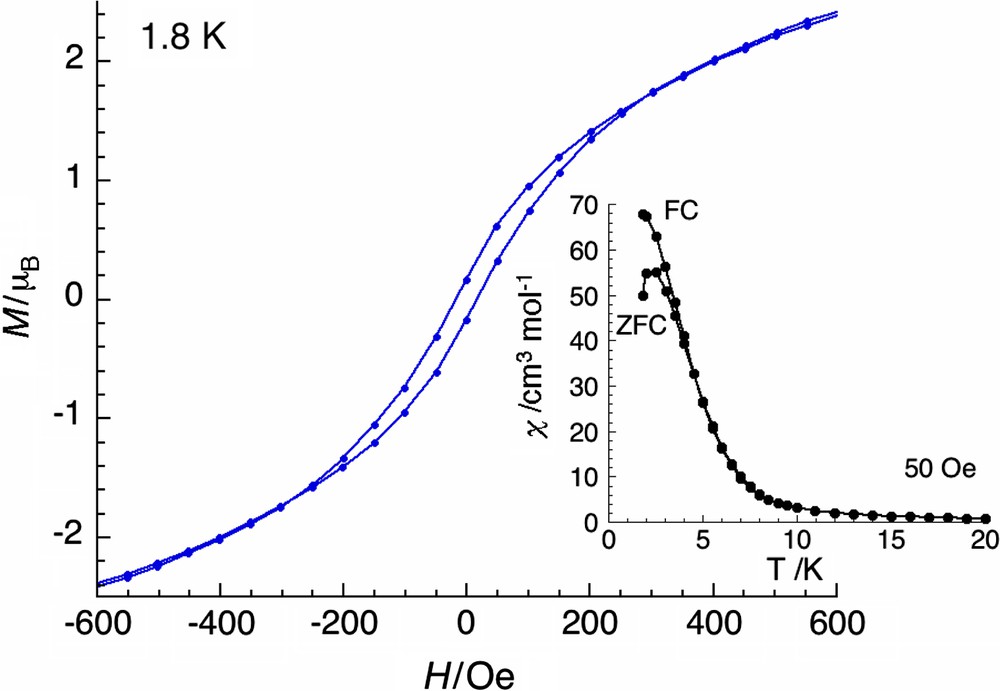
Field dependence of the magnetization at low fields at 1.8 K highlighting the hysteretic behavior with a sweep-rate used in a traditional SQUID magnetometer (≈100 Oe/min). Inset: field-cooled (FC) magnetization and the zero-field-cooled (ZFC) magnetization vs T curves under a weak magnetic field of 50 Oe.
This behavior suggests the presence of a magnetic phase transition that would be a ferrimagnetic long-range order based on the spin topology described above. In order to further investigate this magnetic behavior, ac susceptibility measurements between 1.8 and 10 K have been performed in zero dc field and 3 Oe ac field oscillating at frequencies of 1–1000 Hz. As shown in Fig. 7, the out-of-phase signals (χ″) become non-zero around 5 K independently of the ac frequency used, suggesting the occurrence of a ferrimagnetic order at TC = 5 K. Nevertheless, both components of the ac susceptibility exhibit a slight frequency dependence on both the temperature of their maximum and the height of the peak. The position of the χ′ and the χ″ peaks increases by ∼0.4 K when the frequency is increased from 10 to 1000 Hz. This behavior is not expected to occur for three-dimensional “perfectly” ordered magnets. Indeed, a shift of the peak maximum of χ′ with frequency is usually indicative of a glassy magnetic phase or a superparamagnetic phase [24,25].
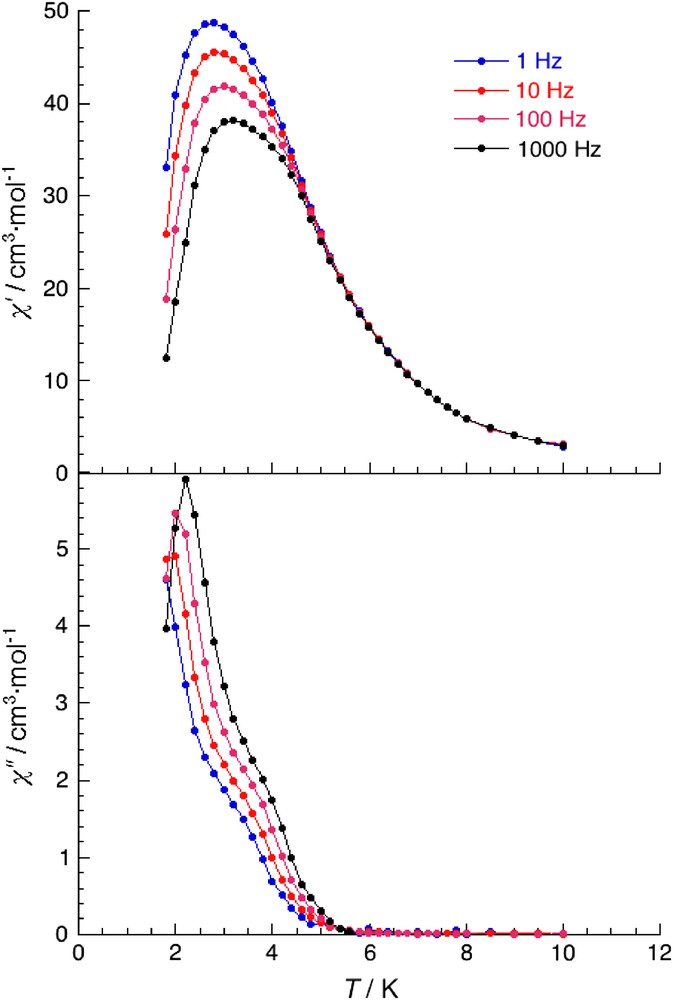
Temperature dependence of the real and imaginary components of the ac susceptibility in a zero applied static field with an oscillating field of 3 Oe at frequencies of 1, 10, 100 and 1000 Hz.
It is worth pointing out that we are avoiding the use of the term spin-glass because this term was coined for magnetically dilute systems prepared by random doping of a few atom percent of a magnetic compound into a non-magnetic, conducting host [25k]. In order to quantify the glassiness, the concept of the frequency shift γ was introduced by Mydosh who defined γ as the slope normalized at Tf(0) of Tf vs log(ν) curve where Tf is the “freezing” temperature located at the peak maximum of χ′ at each frequency, Tf(0) is the “freezing” temperature defined as the peak maximum of χ′ extrapolated at log(ν) = 0 and log(ν) is the logarithm of the ac frequency (ν) [24a]. In the present system, the frequency shift is estimated at 0.063 and according to Mydosh this value is consistent with glassy magnetic behavior and not with superparamagnetism for which much larger γ values (>0.1) are usually observed [24a,25a,d]. The ac susceptibility measurements reveal that this compound possesses a glassy magnetic behavior at low temperature and therefore does not undergo a “perfect” three-dimensional order. Glassiness [24] can result from two main origins (i) randomness (i.e. atom or bond disorder or defect in the crystal structure) and (ii) competing magnetic effects (i.e. interactions, anisotropy). In the present compound, both phenomena are indeed present and could be responsible for the observed glassy behavior: disorder of the ClO4− anions and competition between the magnetic anisotropy of the Mn(III) metal ions and the Mn(III)–Cr(III) antiferromagnetic interactions.
3.3 Magneto-structural considerations
The magneto-structural data of compound 1 can be inspected by comparison with the structurally similar previously reported MnFe systems, namely [{Mn(saltmen)}4{Fe(CN)6}]ClO4·H2O [15] and [{Mn(saltmen)}4Fe(CN)5(1 − CH3im)](ClO4)2·9H2O [20]. Indeed, in both cases a soft magnet is observed with, respectively, a TC of 4.5 and 4.8 K and coercive fields of 80 and 500 Oe, which reasonably compares with the values, 5 K and 20 Oe, found here for 1. Nevertheless in these MnFe systems, both Mn–Mn (through double saltmen phenoxo bridge) and Mn–Fe (through cyanido bridge) interactions were found to be ferromagnetic. On the other hand, the only known structurally similar MnCr system, namely [{Mn(saltmen)}4Cr(CN)5(NO)]ClO4·3H2O [19], also presents the magnetic properties of a soft magnet (TC = 6.4 K and HC = 10 Oe) and does show antiferromagnetic interaction in its Mn–NC–Cr pairs. Ferromagnetic interaction in the FeIII–CN–MnIII pairs is thought to occur because bending of the Mn–NC moiety that results in diminishing of the orbital overlap among the metallic cations. Nevertheless, a similar bending is observed in 1 and these three related compounds, the Mn–N–C angle being, respectively, 156.2°, 156.1°, 149.8° and 154.6°. In the two MnCr systems, 1 and [{Mn(saltmen)}4Cr(CN)5(NO)]ClO4·3H2O, the Cr–CN moiety is also bent while it is linear in the MnFe ones, but the deviation from linearity is too small (the Cr–C–N angle is, respectively, 174.7° and 175.2°) to explain the change in sign of the interaction. The observations on these four 2D Mn(III)–M(III) systems actually pertain to a general trend, since most cyanide-bridged Mn(III)–Fe(III) complexes reported in the literature exhibit ferromagnetic interactions while this is not so far the case for any Mn(III)–Cr(III) complex. Simple orbital considerations, based on the model of localized orbitals developed by Kahn,2 may explain these observations. Indeed, if one chooses the z local axis to be along the Mn and Fe or Cr–cyanido bond, then two antiferromagnetic paths corresponding to the dxz–dxz and dyz–dyz orbital overlaps occur. Nevertheless, the electronic configurations for the low spin Fe(III) that possesses only one unpaired electron on dxy, dxz or dyz orbitals, are degenerated and therefore equally populated with a statistical factor of 1/3. On the other hand in the case of Cr(III), a single electronic configuration is possible with one unpaired electron in both dxz and dyz orbitals. Therefore, with similar structural parameters, the overlaps among magnetic orbitals, giving rise to the antiferromagnetic contribution, should be three times stronger for the Mn(III)–NC–Cr(III) pair than for the Mn(III)–NC–Fe(III) pair. In the latter case bending of the Mn–NC moiety can reduce sufficiently these overlaps to result in an overall ferromagnetic interaction, while in the former case, the antiferromagnetic contribution to the exchange coupling seems to remain dominant, in agreement with experimental data of both compound 1 and [{Mn(saltmen)}4Cr(CN)5(NO)]ClO4·3H2O.3
4 Conclusions
An extended 2D layered Mn(III)–Cr(III) coordination complex has been obtained through reaction of [CrIII(CN)6]3− and [MnIII(saltmen)]+ building block sources. Its structure is based on a pentameric unit formed through four Cr(III)–CN–Mn(III) bridges, these units being connected into layers through double phenoxo Mn–O⋯Mn bridges. The corresponding antiferromagnetic and weak ferromagnetic exchange couplings result in a ferrimagnetic ordering at around 5 K, although some degree of glassiness has been observed. Magneto-structural comparison with similar ferromagnetically coupled Fe(III)Mn(III) systems indicates that the observed antiferromagnetic coupling in the cyanide-bridged Cr(III)Mn(III) systems is simply resulting from Cr(III) d3 electronic configuration resulting in a stronger overlap among magnetic orbitals.
Acknowledgements
This work was supported by the CNRS, the University of Bordeaux 1 and the Conseil Régional d'Aquitaine. R.C. and X.L. thank the Chinese Scholarship Council for the funding of the scientific stay of X.L. at the CRPP.
1 In order to take into account the inter-complex interaction, the following definition of the susceptibility has been used:
2 See Ref. [1], Chapter IX.
3 Although the authors in Ref. [19] assigned the oxidation state of Cr in [Cr(CN)5(NO)] as +1, the observed magnetic behavior point at a probable +3 oxidation state, as indeed considered by the authors in their conclusion and previously argued in M. Ardon, S. Cohen, Inorg. Chem. 32 (1993) 3241.


