1 Introduction
The industrial materials titanium based ilmenite-type perovskites with general formula MTiO3 (M = transition metal) have attracted great interest over the past decades because of their utilization in the field of pigments, photoactive catalysts, sensors, and so forth, due to the specially electronic and optical properties as well as the highly chemical stability, large surface areas and nontoxicity [1–4].
Nickel titanate (NiTiO3), being one member of this well-known binary oxides family, has a broad range of the above-mentioned properties and is applicable for industries, such as semiconductor rectifiers [5], electrodes of solid oxide fuel cell [6], metal-air barrier [7], hydrocarbonate catalyzers [8] and gas sensors [9]. NiTiO3 has been also investigated as a tribological coating to reduce friction and wear in high temperature applications without using liquid lubricants [10,11].
The electronic properties of ceramics are greatly affected by the characteristics of the powder, such as particle size, morphology, purity and chemical composition. Many efforts have been aimed to improve these properties by controlling their microstructure.
It is still a great challenge to search for a simple and cost-effective route to prepare nano-structured NiTiO3 with a high yield. The traditional solid-state method used in preparing NiTiO3 powder leads to poor homogeneity and high sintering temperatures. At the same time, many methods, such as sol-gel, the flux method, co-precipitations, solid-state reactions, electrospinning and the Pechini process, have been reported for the synthesis of crystalline NiTiO3 powders [12–15].
Phani and Santucci [16] prepared NiTiO3 thin films by sol-gel method using titanium isopropoxide and nickel acetate. Structural, morphological and elemental evolution were characterized by X-ray diffraction (XRD), tapping mode atomic force microscopy and X-ray photoelectron spectroscopy.
NiTiO3 powders were prepared by a simple co-precipitation technique using ammonium carbonate and stoichiometric amounts of Ni(NO3)2 and TiOCl2 [11]. The phase evolution was investigated by XRD patterns. Particle size and morphology was studied by transmission electron microscopy (TEM).
Nanocrystalline NiTiO3 powders were produced at low temperature of 500 °C by sol-gel route [14]. XRD patterns and Fourier transform infrared (FT-IR) spectroscopy revealed that the powders contained NiTiO3 besides impurities of NiO, as well as anatase and rutile-TiO2 depending on the annealing temperature and Ni:Ti molar ratio. Based on Brunauer-Emmett-Taylor (BET) analysis, the synthesized powders showed a mesoporous structure containing pores with needle and plate-like shapes.
NiTiO3 microtubes was constructed successfully via a simple solution-combusting method employing a mixture of ethanol and ethyleneglycol, nickel acetate, tetra-n-butyl titanate and oxygen gas [12]. The as-obtained product was characterized by XRD patterns, TEM, scanning electron microscopy (SEM), and energy dispersive X-ray spectrometry (EDS). The BET surface area of the product was 14.06 m2/g and the pore size distribution mainly located from 20 to 30 nm.
Pure NiTiO3 nanopowders were prepared by wet-chemistry method, using nickel stearate, tetra-n-butyl titanate and stearic acid [17]. The synthesis process was followed using thermal analysis techniques. FT-IR, XRD and TEM were used to characterize the crystallization process, the particle size and morphology of the calcined powders.
Therefore, in this paper, we communicate two simple processes for preparing nanocrystalline nickel titanate powder. These processes involve the formation of entire titanate through the thermal decomposition reaction of stoichiometric coprecipitated or impregnated mixture of nickel oxalate-dihydrate and titanium dioxide (anatase). The formation process and structural characterization of NiTiO3 will be investigated by DTA-TG, FT-IR, XRD, and TEM. Furthermore, the specific surface area and electrical properties will be also evaluated. In addition, a mechanism for the thermal decomposition process and the kinetic parameters will be achieved using non-isothermal TG curves.
2 Experimental details
2.1 Materials
Nickel nitrate hexahydrate; Ni(NO3)2·6H2O, basic nickel carbonate; NiCO3.2Ni(OH)2·4H2O, oxalic acid; H2C2O4 and titanium dioxide (anatase); TiO2 were employed as the starting reagents. All chemicals are of analytical grade (BDH) and used without any further purification.
2.2 Synthesis of NiTiO3 powders
Nickel titanate (NiTiO3) powder were synthesized through the thermal decomposition reaction of NiC2O4·2H2O–TiO2 precursor prepared through the impregnation technique or the coprecipitation route.
In the impregnation technique [18], pure NiC2O4·2H2O was prepared by direct precipitation, using oxalic acid added dropwise to a solution containing the calculated amounts of nickel nitrate. The obtained fine precipitate was filtered, washed with distilled water and dried. Few drops of bi-distilled water were then added to weighed mixture of both NiC2O4·2H2O and TiO2 (1:1 mole ratio) with vigorous stirring to assure complete homogeneity. The wetted mixture was then dried at 80 °C.
In the co-precipitation method [19], TiO2 was added to an aqueous solution containing a stoichiometric amount of NiCO3·2Ni(OH)2·4H2O under vigorous stirring. A stoichiometric amount of oxalic acid solution was then added dropwise into the suspension under constant stirring. During this process, the formed nickel oxalate will be precipitated on the surface of TiO2 particles by heterogeneous nucleation. The resulting solution was then evaporated until dryness.
Samples of the prepared precursors were then calcined at different temperatures to characterize the decomposition reaction and follow titanate formation. The calcination temperatures and calcination times were 400 °C for 30 min, 600, 800 or 1000 °C for 2 h.
2.3 Characterizations
The decomposition course of the precursors and titanate formation were followed using simultaneous differential thermal analysis-thermogravimetry techniques (DTA-TG). The measurements were carried out using a Perkin–Elmer, STA 6000 thermal analyzer up to 1000 °C at a heating rate of 5 °C/min in air atmosphere. For kinetic measurements, other heating rates of 1, 2 and 3 °C/min were used.
The structure of the powders is examined by X-ray diffraction technique (XRD) using a Bruker D8 high-resolution diffractometer with nickel filtered Cu Kα radiation.
The Fourier transform infrared (FT-IR) spectra of the samples were recorded in the range 4000–200 cm−1 on JASCO FT-IR 310 spectrometer using the KBr pellet method.
The transmission electron microscopy (TEMJ) image of the NiTiO3 nanopowders was obtained using an electron microscope (JEOL-2010) operating at 100 kV. The samples were prepared by ultrasonically dispersing the powder in ethanol and allowing a drop of this to dry on a carbon-coated copper grid.
The Brunauer, Emmett and Teller (BET) method has been used to determine the specific surface area of titanate by means of a nitrogen adsorption isotherm on a Micromeritics, ASAP 2010.
For the electrical measurements, titanate powder was pressed into a pellet under a pressure of 2 ton/cm2. The polished pellet with a silver paint was used for measuring temperature-dependent electrical conductivity, at different frequencies (100 Hz–5 MHz), using a Hioki LCR bridge model 3531.
3 Results and discussion
3.1 Thermal analysis studies
To investigate the different crystalline intermediates resulting from the thermal decomposition course of coprecipitated NiC2O4·2H2O–TiO2 precursor and find out the optimum calcining temperature for titanate formation, the corresponding DTA-TG thermogram was conducted and illustrated in Fig. 1.
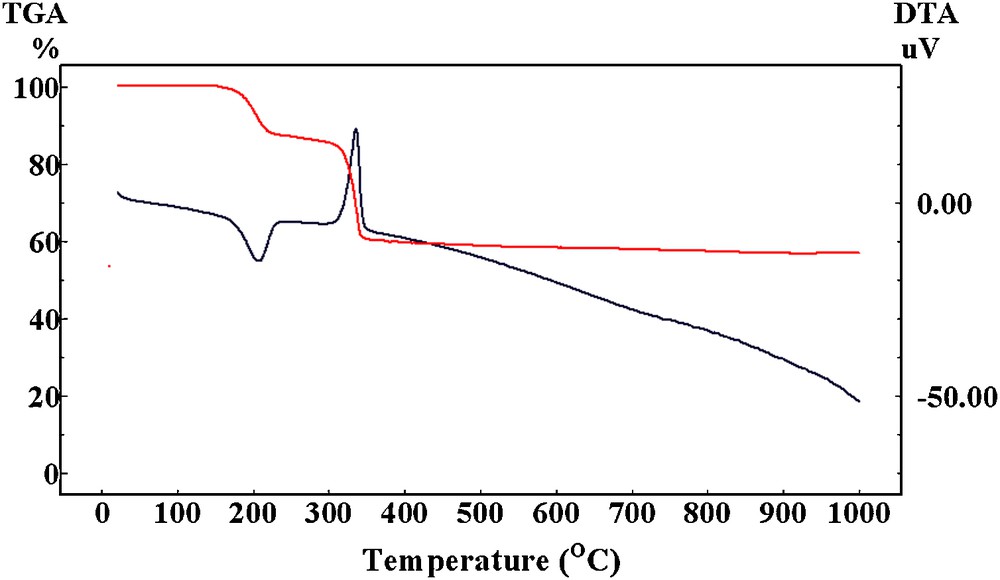
DTA-TG curves for the thermal decomposition of NiC2O4·2H2O–TiO2 precursor in air at heating rate of 5 °C/min.
From the figure, it is clear that the decomposition proceeds through two weight loss steps in the range 160–360 °C. The observed DTA peaks are closely corresponding to the obtained weight losses. The two steps, based on the decomposition range and weight loss calculations, can be assigned to the decomposition of the oxalate contents of the precursor.
The first step (Obs. Wt loss = 13.6%) is mostly due to dehydration with the loss of two water molecules (Calc. Wt. loss = 13.7%). The endothermic DTA peak characterizing this dehydration lies in the range 163–234 °C with peak temperature at 207 °C. This dehydration range suggests that the water molecules are coordinately bounded to the oxalate moiety [20]. The second TG step (Obs. Wt loss = 27.5%) between 300 and 360 °C seems to be associated with the decomposition of anhydrous nickel oxalate with the formation of NiO. The accompanying exothermic peak at 336 °C can be attributed to the oxidation of Ni metal and CO decomposition products to NiO and CO2, respectively [21,22].
No excessive weight loss or DTA change can be detected after 360 °C, suggesting the inability of titanate formation within this temperature range.
DTA-TG thermogram of the impregnated NiC2O4·2H2O–TiO2 precursor showed consistent behavior with that estimated for coprecipitated precursor.
3.2 X-ray diffraction patterns
XRD patterns taken for the as-prepared precursors as well as their calcined powders at different temperatures are shown in Figs. 2 and 3. From the figures, it is obvious that, the two as-prepared precursors as well as their calcined powders at 400, 600 and 800 °C showed identical XRD patterns.
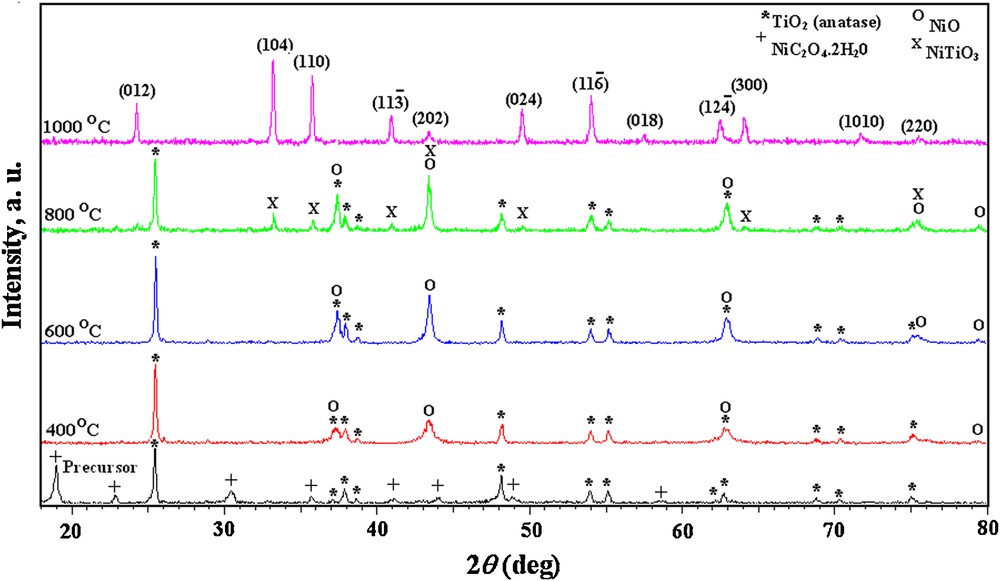
XRD patterns of impregnated NiC2O4·2H2O–TiO2 precursor calcined at different temperatures.
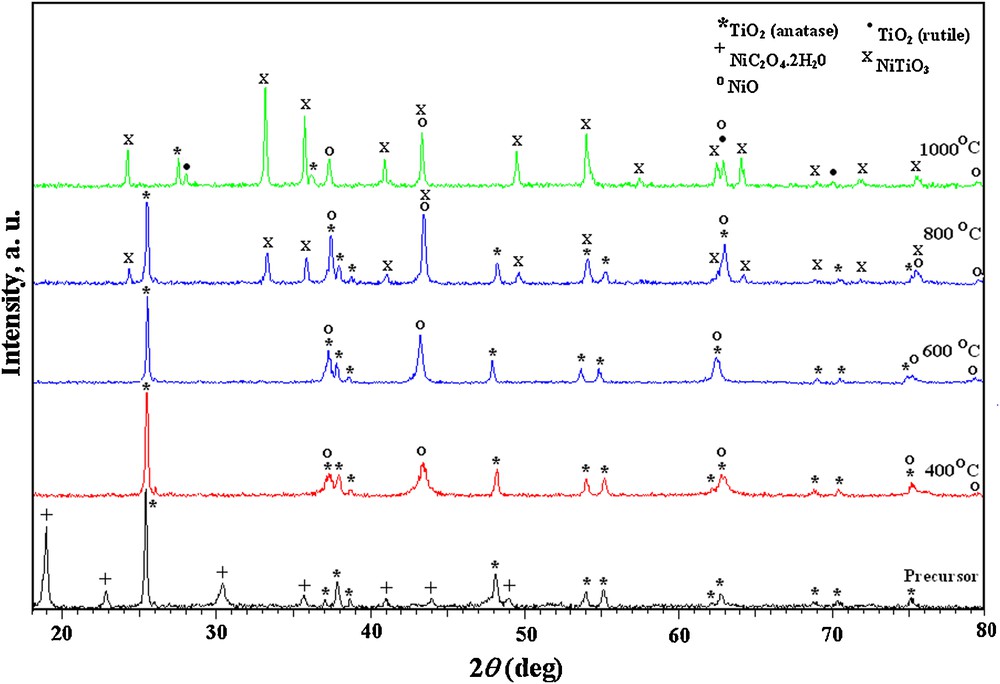
XRD patterns of coprecipitated NiC2O4·2H2O–TiO2 precursor calcined at different temperatures.
The as-prepared precursors exhibited XRD peaks, which can be assigned to the tetragonal TiO2 (anatase) and NiC2O4·2H2O (JCPDS file No.: 78-2486 and JCPDS file No.: 14-0742, respectively). XRD of the calcined precursors at 400 °C revealed, consistently with DTA-TG measurements, the appearance of the characteristic peaks of NiO (JCPDS file No.: 78-0423) produced from the decomposition of nickel oxalate content besides those of TiO2 (anatase). No obvious changes are observed by rising the calcination temperature to 600 °C while, at 800 °C, the characteristic XRD peaks of titanate are evidenced; NiTiO3 started to appear besides those assigned to NiO and anatase-TiO2.
The XRD pattern of the impregnated NiC2O4·2H2O–TiO2 precursor calcined at 1000 °C (Fig. 2) showed that all the peaks can be indexed for complete formation of NiTiO3 powders with a single-phase rhombohedral structure and space group of and six formula units per unit cell (JCPDS file No.: 76-0334 or 33-0960). The calculated lattice parameters (a = b = 5.0252 Å, c = 13.7761 Å) agree well with those reported [11,23]. The average crystalline size calculated from full width half maximum (FWHM) using the Scherrer formula [24] for the reflections in Fig. 2 is found to be 87 nm. The X-ray density calculated using estimated lattice parameters is 4.43 g/cm3.
On the other hand, XRD patterns of the calcined coprecipitated NiC2O4·2H2O–TiO2 precursor at 1000 °C (Fig. 3) exhibits some impurity peaks, which can be indexed to NiO, TiO2 (anatase) and TiO2 (rutile) (JCPDS file No.: 82-0514) phases, besides characteristic NiTiO3 peaks. This suggests, in accordance with the reported results in the literature [1,14], the incompletion of the titanate single-phase within this temperature range. The phase transformation of the higher total free energy anatase-TiO2, at such high temperature, results in the formation of more stable rutile structure [25].
3.3 Morphological study
Fig. 4a shows SEM micrograph of NiTiO3 powder obtained through the impregnation method. The micrograph reflects the agglomeration nature of the particles with porous morphology. The particles are agglomerated into clusters of varying sizes and shapes. The size of the particles can be identified using TEM technique. TEM image of the powder (Fig. 4b) exhibited hexagonal particles with uniform morphology in size and shape. The average size is around 61 nm, which is slightly smaller than that obtained through XRD patterns.
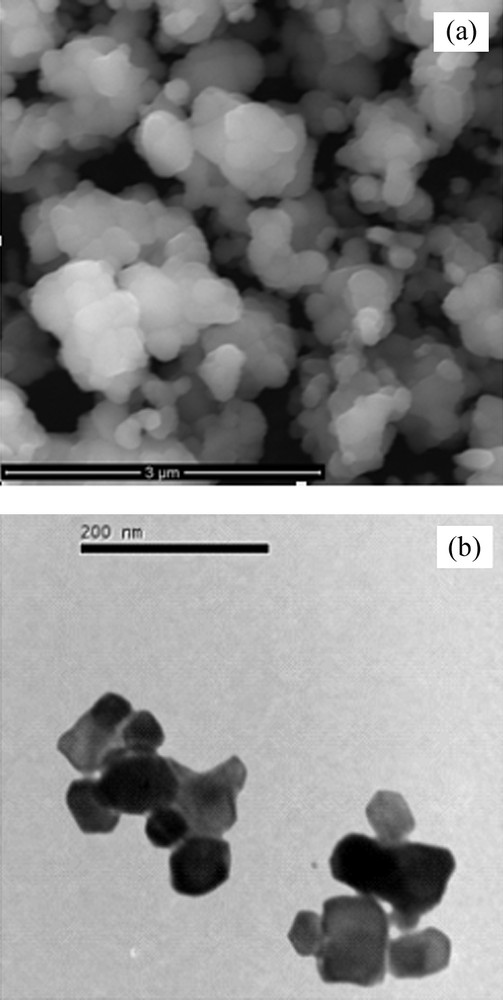
(a) Low magnification SEM image and (b) TEM image of NiTiO3 powder.
3.4 FT-IR spectra
Fig. 5 exhibited the FT-IR spectra of the as-prepared impregnated precursor along with its calcined samples at different temperatures. FT-IR spectrum for the as-prepared precursor shows a broad absorption band around 3740 cm−1 due to the stretching vibration of O–H group. The absorption band appearing at 1650 cm−1 can be assigned to the asymmetric vibrations of the carbonyl group, while the strong band at 1300 cm−1 is assigned to the symmetric mode. The bands at 773 and 512 cm−1 are attributed to the out-of-plane bending mode of water and OCO in-plane bending mode of oxalate, respectively [26]. The band at 352 cm−1 can be assigned to the vibration of metal ion, and the bands at 675 and 2929 cm−1 to the presence of TiO2 [27].
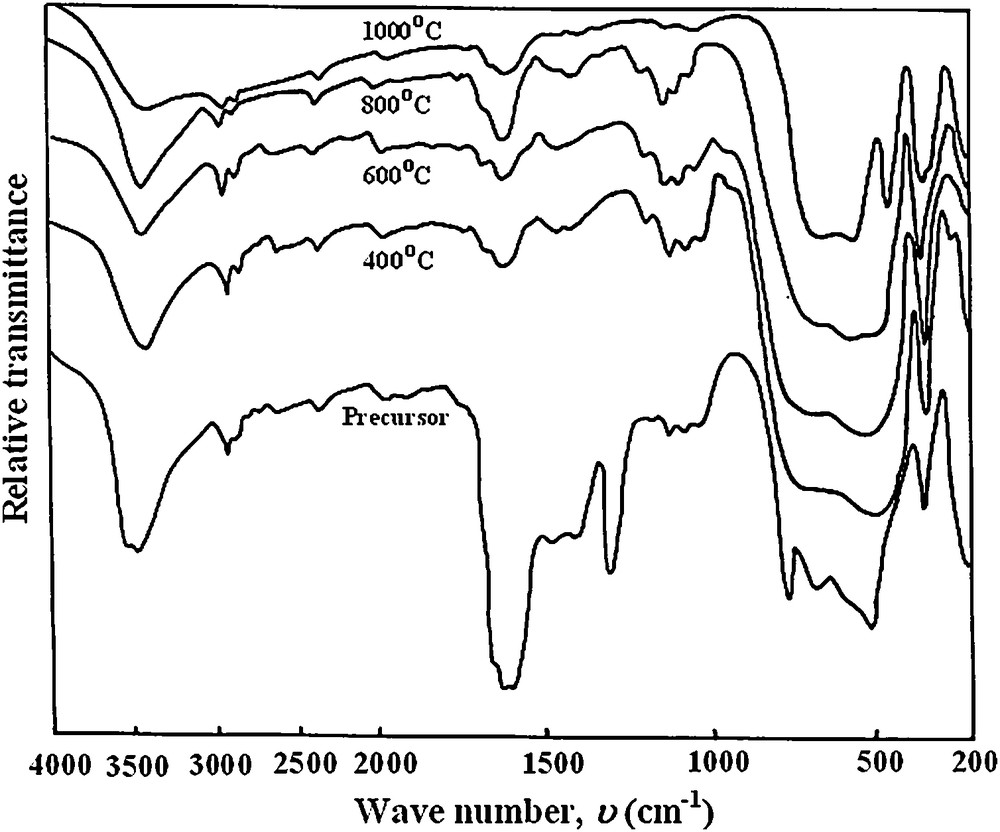
FT-IR spectra of NiC2O4·2H2O–TiO2 precursor calcined at different temperatures.
The spectrum of the calcined precursor at 400 °C showed a decrease in the intensities of the bands characteristic for the carbonyl group due to the decomposition of the oxalate content with the formation of metal oxides. Consequently, the new broad intense bands appearing at 681 and 525 cm−1 can be assigned to the metal-oxygen and TiO2 vibrations, respectively [19].
Similar spectra are observed for powders calcined at 600 and 800 °C. The appearance of a new band at 566 cm−1 in the spectrum of powder calcined at 800 °C indicating, in accordance with XRD patterns, the starting of titanate formation. At 1000 °C, the three characteristic bands observed at 651, 567 and 461 cm−1 can correspond to the formation of NiTiO3 powders in accordance with previously reported results [10,14,28].
3.5 BET measurement
Fig. 6 illustrates the N2 adsorption-desorption isotherms of impregnated precursor annealed at 1000 °C. The isotherm represents a type III isotherm, which describes adsorption on macroporous adsorbents with weak adsorbate-adsorbent interactions [29]. The specific surface area measured by the BET method (SBET) is 152 m2/g. This relatively high value compared to those reported in the literature [12,14,23] suggests the validity of the present synthesis route in obtaining nano-sized titanate powder, with very high surface area, having applications in catalysis, separation and purification processes.
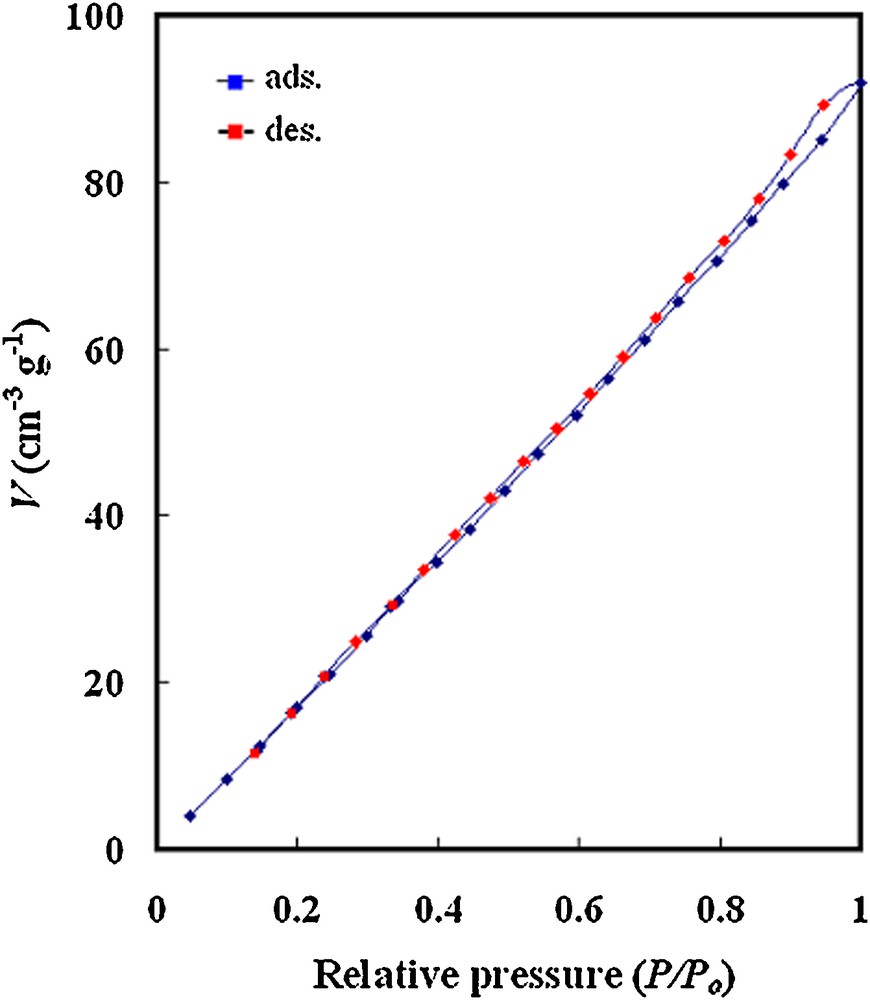
N2 adsorption/desorption isotherm obtained for NiTiO3.
3.6 AC conductivity
The plot of conductivity (ln σac) for NiTiO3 powder as a function of temperature and frequency is illustrated in Fig. 7. The figure indicates that the conductivity shows strong dependence on both temperature and frequency of the applied field. At low temperatures, the conductivity shows a metallic behavior in which the conductivity is nearly stable with increasing temperature. By increasing temperature, a pronounced peak at 605 K is observed after which an obvious increase in the conductivity values with increasing temperature is revealed. The appearance of this peak can be attributed to a ferroelectric phase transition [30,31]. Singh et al. [30] has reported a marked change in the conduction mechanism of NiTiO3 at 700 K during measuring electrical conductivity as a function of temperature. Bamzai et al. [31] indicated, through measuring the dependence of dielectric constant, dielectric loss and AC conductivity on applied frequency and temperature, the appearance of this peak at 773 K instead of 700 K.
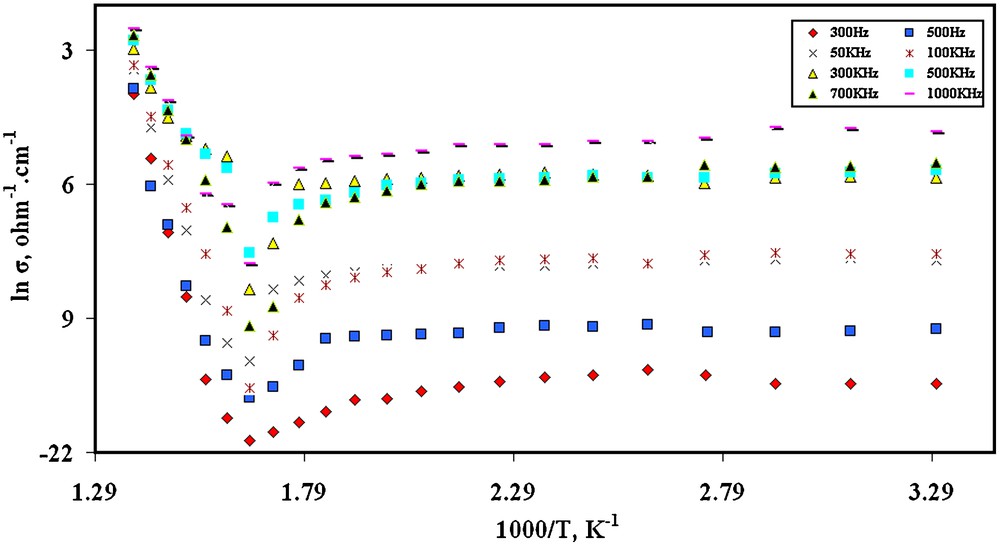
Relation between ln σac and the reciprocal of the absolute temperature as a function of applied frequency for NiTiO3.
The increase in the conductivity at high temperatures can be explained by considering the mobility of charge carriers responsible for hopping which increases also by increasing temperature. This situation suggests semiconducting behavior of NiTiO3. The activation energy per mole (Ea) required for this hopping process, calculated in the ferroelectric region from the slope of ln σac versus reciprocal of absolute temperature, is 0.93 eV. This higher value than 0.2 eV per molecule suggests that the conduction mechanism is due to hopping [30].
The obvious increasing of AC conductivity with increasing frequency is a characteristic feature of materials where hopping mechanism dominates [31].
3.7 Kinetic studies
To characterize the solid-state decompositions mechanism during thermal treatment of NiC2O4·2H2O–TiO2 precursor, aiming at the production of NiTiO3, the kinetic analysis under non-isothermal conditions of TG curves was carried out using different heating rates and assuming different solid-state reaction equations [20] The kinetic parameters: E, activation energy (kJ/mol) and A, frequency factor (min−1) were calculated in view of three integral methods: Diefallah's composite method [20,32,33], Coats–Redfern method [34] and Ozawa method [35]. The details of these methods were reported elsewhere [20,32].
The composite method involves a model-fitting kinetic approach, since it does not assume a particular reaction model, but it allows the choice of the kinetic mechanism which best fits the data and gives the highest correlation coefficient [32]. Fig. 8 correlates representative weight changes as a function of temperature obtained from non-isothermal measurements. Kinetic analysis according to the composite method assuming different models of heterogeneous solid-state reactions (Table 1) shows that the dehydration step is best described by phase boundary controlled reaction mechanism (R2 and R3), in which the reaction is controlled by the movement of an interface at constant velocity and nucleation occurs virtually instantaneously. On the other hand, the oxalate decomposition step follows Avrami–Erofeev random nucleation mechanism (A2, A3 and A4) characteristic for random nucleation growth. The other reaction models gave less satisfactory results.
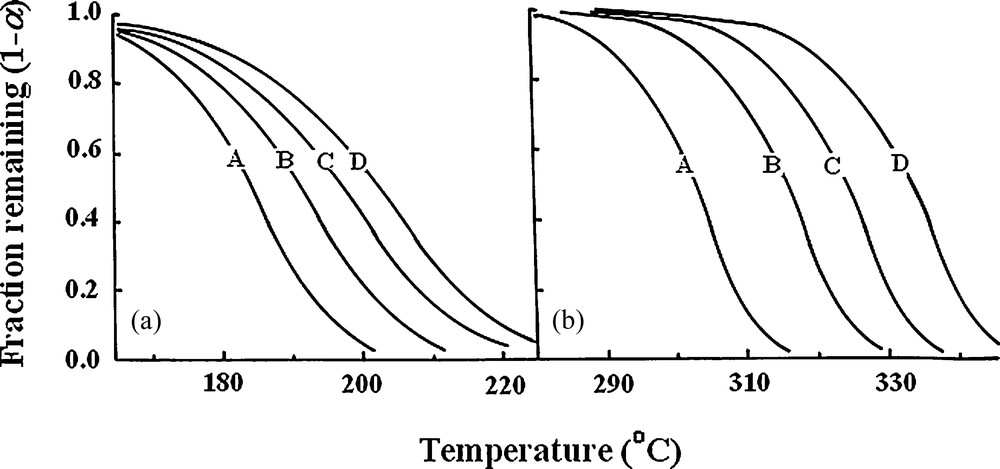
Non-isothermal measurements for the thermal decomposition in air of NiC2O4·2H2O–TiO2 precursor: (a) dehydration and (b) decomposition of oxalate content Heating rate: curve A, 1 °C/min; curve B, 2 °C/min; curve C, 3 °C/min and curve D, 5 °C/min.
Activation parameters for non-isothermal decomposition in air of the NiC2O4·2H2O–TiO2 precursor, calculated according to the composite method, assuming different kinetic models.
| Model | Dehydration (step I) | Decomposition (step II) | ||||
| E (kJ/mol) | log A (min–1) | r | E (kJ/mol) | log A (min–1) | r | |
| D1 | 200 ± 4 | 21.1 ± 0.5 | 0.981 | 382 ± 33 | 32.4 ± 3.1 | 0.817 |
| D2 | 217 ± 4 | 22.8 ± 0.5 | 0.982 | 403 ± 35 | 34.2 ± 3.3 | 0.813 |
| D3 | 238 ± 4 | 24.7 ± 0.5 | 0.982 | 431 ± 39 | 36.2 ± 3.6 | 0.807 |
| D4 | 224 ± 4 | 23.0 ± 0.5 | 0.982 | 413 ± 36 | 34.4 ± 3.4 | 0.811 |
| R2 | 123 ± 2 | 12.4 ± 0.2 | 0.987 | 246 ± 13 | 20.4 ± 1.2 | 0.914 |
| R3 | 128 ± 2 | 12.9 ± 0.2 | 0.990 | 253 ± 14 | 20.8 ± 1.3 | 0.910 |
| F1 | 140 ± 2 | 14.7 ± 0.2 | 0.993 | 268 ± 16 | 22.8 ± 1.5 | 0.899 |
| F2 | 91 ± 5 | 9.9 ± 0.6 | 0.877 | 172 ± 11 | 14.8 ± 1.0 | 0.891 |
| F3 | 164 ± 8 | 18.6 ± 0.9 | 0.908 | 270 ± 25 | 24.0 ± 2.4 | 0.795 |
| A2 | 79 ± 4 | 8.0 ± 0.4 | 0.917 | 171 ± 3 | 14.2 ± 0.3 | 0.989 |
| A3 | 59 ± 4 | 5.8 ± 0.5 | 0.811 | 139 ± 2 | 11.3 ± 0.2 | 0.991 |
| A4 | 49 ± 5 | 4.7 ± 0.6 | 0.723 | 123 ± 4 | 9.9 ± 0.4 | 0.963 |
| E1 | 90 ± 11 | 13.9 ± 1.3 | 0.642 | 80 ± 32 | 9.3 ± 3.0 | 0.290 |
The activation parameters were then also calculated using Coats–Redfern and Ozawa methods assuming R3 and A3 models for the dehydration and oxalate decomposition steps, respectively. The calculation results according to the three methods are summarized in Table 2. The results show a good agreement (within the experimental errors) between the values of the calculated activation parameters assuming different calculation methods.
Activation parameters calculated using different methods for the non-isothermal decomposition in air of NiC2O4·2H2O–TiO2 precursor.
| Method of analysis | Dehydration (R3 model) | Decomposition (A3 model) | ||
| E (kJ/mol) | log A (min–1) | E (kJ/mol) | log A (min–1) | |
| Composite | 128 ± 2 | 12.9 ± 0.2 | 139 ± 2 | 11.3 ± 0.2 |
| Coats–Redfern | 130 ± 19 | 13.1 ± 0.4 | 125 ± 10 | 11.1 ± 0.3 |
| Ozawa | 153 ± 23 | 13.4 ± 0.5 | 147 ± 4 | 11.5 ± 0.3 |
It is well known that the activation energy for the losing of crystal water lie in the range 60–80 kJ/mol, while the value for the coordinately bounded one are within the range 130–160 kJ/mol [20]. The obtained activation energy for the oxalate dehydration (Table 2) suggests that the water molecules are coordinately linked water. This result agrees well with the dehydration temperature obtained from DTA-TG experiments.
The obtained activation energy for the oxalate decomposition reaction (Table 2) appears to be lower than that reported in the literature [22,36]. This can be attributed to the presence of titanium dioxide during the thermal decomposition of oxalate, which can act as a spacer causing fewer points of contact between particles of nickel oxalate and increasing the interfaces, which facilitates the decomposition and consequently, decreases the activation energy.
4 Conclusions
In summary, single-phase NiTiO3 nanopowders was successfully synthesized through simple impregnation technique using the NiC2O4·2H2O–TiO2 precursor. The thermal decomposition course of precursor was followed by DTA-TG measurements. Calcined samples of precursor at different temperatures were investigated using XRD patterns and FT-IR spectra to evaluate the formation of NiTiO3 phase. Particle size and morphology of NiTiO3 nanopowders studied by TEM image revealed the presence of hexagonal nano-particles with a size of 61 nm. BET measurements estimated a very large surface area of 152 m2/g suggesting its use as a catalyst. AC conductivity measurements as a function of temperature revealed a semiconducting behavior with the predominance of a hopping conduction mechanism. In addition, the obtained ferroelectric phase transition at 605 K suggests ferroelectric properties at such high temperature range. Kinetic analysis of the non-isothermal TG curves showed that the presence of TiO2 reduces the activation energy for the oxalate decomposition.
Acknowledgement
This paper was funded by the Deanship of Scientific Research (DSR), King Abdulaziz University, Jeddah, under grant No. [518-130-1432]). The authors therefore acknowledge with thanks DSR technical and financial support.


