Plan
Comptes Rendus
no. 12

Rare-earth-doped glasses for fiber amplifiers in broadband telecommunication
Setsuhisa Tanabe1 
1 Faculty of Integrated Studies, Kyoto University, Kyoto 606-8501, Japan
Comptes Rendus. Chimie, Volume 5 (2002) no. 12, pp. 815-824.

Résumés

Rare-earth-doped optical amplifiers have a great potential for broadband Wavelength-Division-Multiplexed (WDM) telecommunication by tailoring host glass compositions. In order to design the emission spectra of doped rare-earth ions, it is important to understand the relationship between the local ligand field and various optical properties of specific 4f-levels, such as the radiative transition probability, the nonradiative decay probability, which dominate the spectral line width and quantum efficiency of amplification transitions. For the Er3+:1.55 μm transition, the role of the Judd–Ofelt Ω6 parameters is presented, which is correlated to the Er–ligand bond covalency in glasses. The Tm3+: 1.46-μm transition shows quantum efficiency over 90% high enough for the S-band application, in heavy metal oxide glasses with moderate phonon energy and wider spectra than fluorides. A way to improve population inversion by selective energy transfer with codoped lanthanide ions is presented. Finally, the energy level structures and resultant spectral properties of Pr3+, Nd3+ and Dy3+ ions, 1.3-μm-active ions, are compared. The hypersensitivity of Dy3+ transitions appears especially in chalcogenide glasses, where the nonradiative loss due to multiphonon decay is also minimized. In conclusion, glass materials have opportunities to vary the radiative cross section, quantum efficiency, and gain flatness, which are important for novel amplifiers in the future DWDM system.

Les télécommunications à large bande basées sur le multiplexage en division de longueur d’onde (WDM) font appel à des amplificateurs optiques dopés avec des terres rares. Le spectre d’émission de ces terres rares peut être ajusté en modifiant la composition du verre. Il faut pour cela connaître la relation entre le champ local et les caractéristiques optiques des niveaux concernés, en particulier les probabilités de transition radiative et de désexcitation non radiative, qui déterminent la largeur spectrale et le rendement quantique des transitions. Le rôle du paramètre de Judd–Ofeld Ω6 dans la transition de l’erbium à 1,55 μm est corrélé à la covalence de la liaison Er–ligande dans le verre. Les transitions du Tm3+ pour la bande S et celle des ions Pr3+, Nd3+ et Dy3+ à 1,3 μm sont également abordées et discutées. Dans les matériaux vitreux, on peut ainsi faire varier la section efficace radiative, le rendement quantique et la constance du gain, ce qui peut se révéler important pour les nouveaux amplificateurs dans les futurs systèmes DWDM.

Métadonnées
Reçu le :
Accepté le :
Publié le :
DOI : 10.1016/S1631-0748(02)01449-2
Keywords: rare earths, glass, optical amplifier, telecommunication, Wavelength-Division Multiplexing, optical fiber
Mots-clés : terres rares, verre, amplificateur optique, télécommunication, Wavelength-Division Multiplexing (WDM), fibre optique

Setsuhisa Tanabe 1

1 Faculty of Integrated Studies, Kyoto University, Kyoto 606-8501, Japan 
@article{CRCHIM_2002__5_12_815_0,
     author = {Setsuhisa Tanabe},
     title = {Rare-earth-doped glasses for fiber amplifiers in broadband telecommunication},
     journal = {Comptes Rendus. Chimie},
     pages = {815--824},
     publisher = {Elsevier},
     volume = {5},
     number = {12},
     year = {2002},
     doi = {10.1016/S1631-0748(02)01449-2},
     language = {en},
}
TY  - JOUR
AU  - Setsuhisa Tanabe
TI  - Rare-earth-doped glasses for fiber amplifiers in broadband telecommunication
JO  - Comptes Rendus. Chimie
PY  - 2002
SP  - 815
EP  - 824
VL  - 5
IS  - 12
PB  - Elsevier
DO  - 10.1016/S1631-0748(02)01449-2
LA  - en
ID  - CRCHIM_2002__5_12_815_0
ER  - 
%0 Journal Article
%A Setsuhisa Tanabe
%T Rare-earth-doped glasses for fiber amplifiers in broadband telecommunication
%J Comptes Rendus. Chimie
%D 2002
%P 815-824
%V 5
%N 12
%I Elsevier
%R 10.1016/S1631-0748(02)01449-2
%G en
%F CRCHIM_2002__5_12_815_0
Setsuhisa Tanabe. Rare-earth-doped glasses for fiber amplifiers in broadband telecommunication. Comptes Rendus. Chimie, Volume 5 (2002) no. 12, pp. 815-824. doi : 10.1016/S1631-0748(02)01449-2. https://comptes-rendus.academie-sciences.fr/chimie/articles/10.1016/S1631-0748(02)01449-2/

Version originale du texte intégral

Le texte intégral ci-dessous peut contenir quelques erreurs de conversion par rapport à la version officielle de l'article publié.

1 Introduction

The growing demand and future potentials of an advanced information society stimulate research for devices composing a network system with excellent flexibility and larger information capacities at much faster rates. Installation of broadband Wavelength-Division-Multiplexing (WDM) optical network system is indispensable and novel amplifier materials, which would overcome the performance of the present silica-based erbium-doped fiber amplifier (EDFA), can be a key to enable more number of channels. Among several possible choices of devices, rare-earth-doped amplifiers have implied high-power conversion efficiency than Raman and scalability of gain spectra by tailoring the host-glass composition. In telecommunication systems, the invention of the EDFA 〚1〛 can be likened to that of the transistors in electronics in terms of its technological impact. The technology to amplify the light signal directly without the conversion of light/electricity/light has been achieved by stimulated emission of 4f optical transition in rare-earth-doped fibers 〚2〛, which realizes ideal amplification with high gain and low noise. The technological development of optical telecommunication is based on the growth of technologies of fiber fabrication and those of laser diodes (LD). In fact, the invention of efficient III-V LD has also enabled efficient pumping of Er3+ with its three-level system 〚3–5〛. In addition, there exists the history of technological transition from passive fibers to active fibers 〚2〛, in which we can find a quite interesting relationship between active ions and the host glasses. Although the fiber amplifiers are already playing crucial roles in the optical networks both at 1.55 μm and at 1.3 μm bands, there exist further requirements to fully utilize the window of optical fibers with superior performance. The requirements are wide and flat gain spectrum around 1.53∼1.65 μm (C+L band) in a novel EDFA and around 1.45∼1.51 μm (S-band) in Tm3+ (TDFA) for the WDM systems 〚6〛, greater gain per pump-power at 1.31 μm in Pr3+- 〚7, 8〛 or possibly Dy3+-doped glasses 〚9–11〛.

According to the report of the Japanese Photonic Network Research Committee, the DWDM system will require 3000 channels in the year 2010 〚12〛. To enable 3000-channel WDM, we need more various amplifiers as well as a transmission fiber with a window from 1 to 1.65 μm. Fig. 1 shows a typical loss spectrum of the present silica fiber and emission bands of several rare-earth ions.

Fig. 1

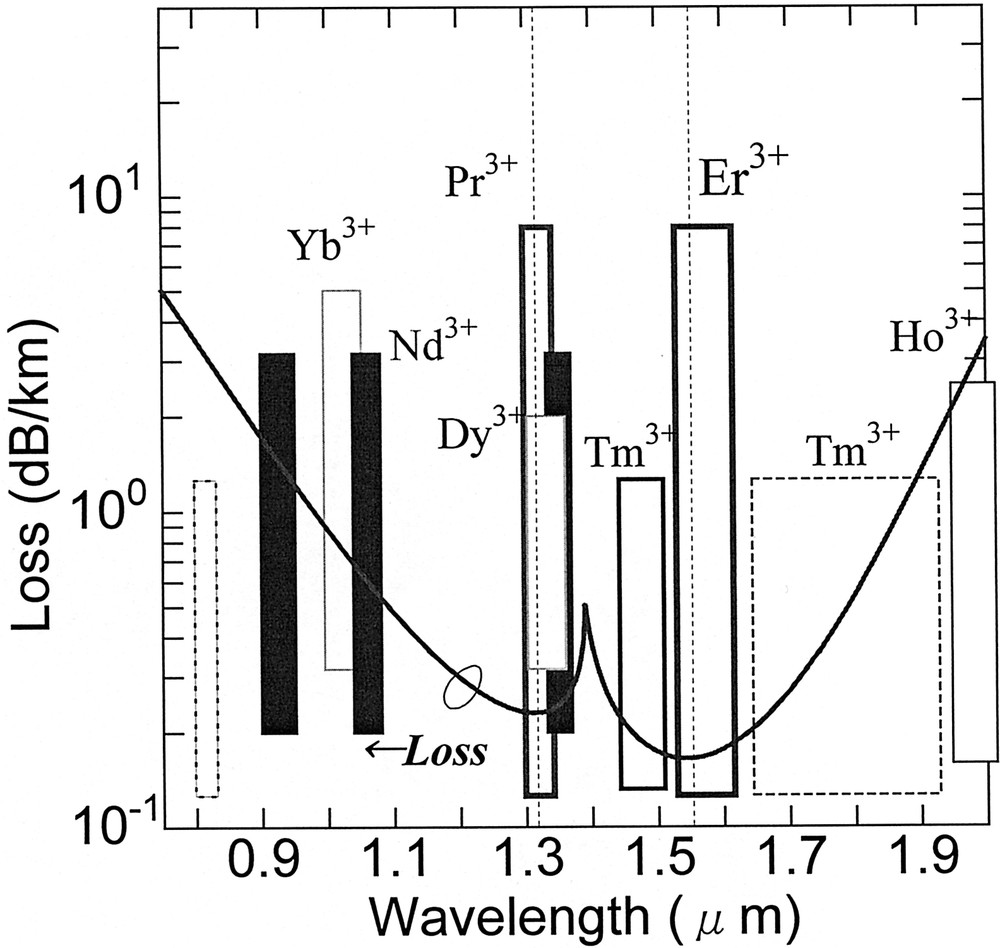
Loss characteristics of silica fiber and emission bands of some rare-earth ions.

Not only in fiber forms, planar waveguide forms will also play a role in photonic devices, since integration of optical circuits improves functions with fewer components 〚13〛. Because a shorter device length is usually expected for these waveguides, it might suffer from the problem of concentration quenching and new solutions of host materials would be required, due to the need of greater doping concentrations of rare-earth ions. In devices of either form, it is necessary to understand the 4f transitions of the ions, the structure of their sites in glass to design amplifier materials with better properties. This paper reviews some studies on the spectroscopic properties, radiative and nonradiative processes, and site structure of rare-earth ions in glasses at each wavelength.

2 Research scheme for rare-earth laser glasses

An optical amplifier works by the principle of light amplification by stimulated emission of radiation, nothing different from that of lasers. It requires a pump to create a population inversion in the amplifier medium. When an incoming signal photon stimulates an excited electron, the electron relaxes back into a lower energy state and emits a second signal photon with the same phase as the incoming photon. This process of stimulated emission amplifiers the signal. The success of the first generation silica-based EDFA is that the energy level of Er3+ ions can cause amplification to emit a photon with precisely the wavelength at which the network operates, with high efficiency, even in silica hosts having high phonon energy and poor RE solubility. The erbium was really a lucky boy to be the first, with an ideal energy level structure workable in silica and several pumping LDs available. Anyway, it is not a surprising idea to apply a research scheme for various rare-earth laser glasses to the design of novel amplifiers. To quantitatively comprehend optical phenomena of rare-earth ions in glasses, it is important to evaluate radiative and nonradiative decay process of related 4f levels. The Judd-Ofelt theory is usually adopted to obtain the radiative transition probabilities including emission by utilizing the data of absorption cross-sections of several f–f electric-dipole lines, especially for four-level emissions. The physical and chemical implement of three Ωt parameters (t = 2,4,6) are becoming clearer by combining the information of the local ligand field of doped ions by other spectroscopic techniques such as the 151Eu-Mössbauer effect 〚14〛. In some cases, the isomer shift and quadrupole splitting give us more discrete information about the rare-earth environment in glass; bond covalency and symmetry 〚15〛. The nonradiative decay rate can be evaluated experimentally by combining the lifetime measurement, which include contributions of multiphonon decay, energy transfer such as cross relaxation, cooperative upconversion, etc. In order to distinguish each contribution, systematic studies on concentration dependence of the rates are necessary. The phonon sideband spectra of Eu3+ ion can be supplementary to inclusive understanding of local structure in glass hosts, where the stretching vibrational mode of the network former bond often plays an important role 〚16〛 in the decay as well as in the phonon-assisted energy transfer 〚17〛. The above-mentioned research scheme is shown in Fig. 2. In many cases, the site selectivity of doped RE ions cannot be predicted in multicomponent glasses. However, we can feed back the several characteristics obtained for selected glasses toward a better material design based on logical direction.

Fig. 2

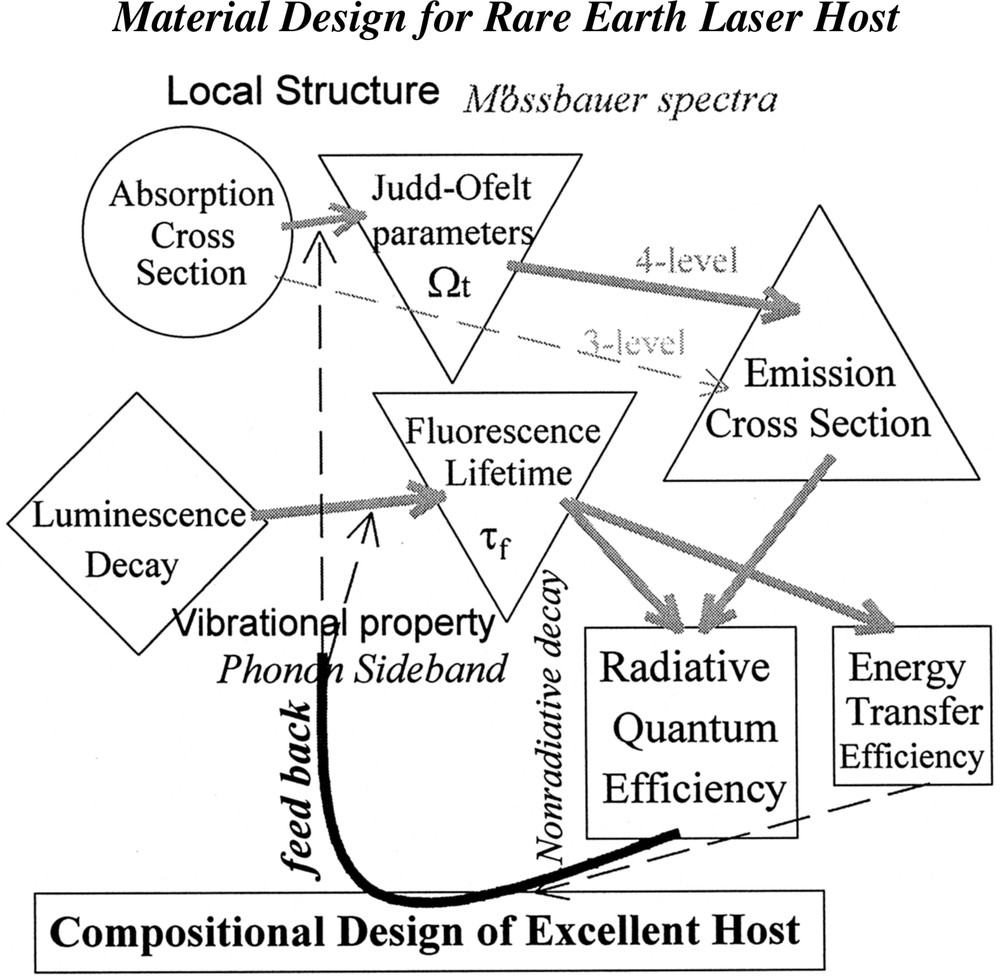
Research scheme for efficient laser materials.

3 The 1.55-μm band in Er3+-doped glasses for broadband amplifiers

There is now a worldwide consensus that the WDM networking has superior functions and applications for future telecommunication systems 〚18〛 to the single channel TDM only at 1.55 μm. It offers not only large-scale information capacity, but also flexibility and transparency by utilizing the properties of light. Among various properties of EDFA, such as low-noise, broad bandwidth (not broad enough now!!) and efficient gain, the ability to amplify more than one wavelength at a time has replaced the regenerator for each channel. Although the excellent performance of the present silica-based EDFA can be used for a WDM system, but with fewer channels, there exists requirement for a larger number of channels, which could be possible by using an EDFA with a wider gain spectrum 〚6〛. From a practical standpoint, the flatness of the gain is also critically important because the light intensity for different channels would be varied by the multi-step amplifications if the gain of the amplifier were not independent of wavelengths. Therefore, the design of a host for Er3+ for wide and flat spectra of the 4I13/2–4I15/2 transition at around 1.5∼1.6 μm is a target at present.

For the transitions between the states with the difference in the total angular momentum by ΔJ = 1, there exists the contribution of the magnetic-dipole transition 〚19〛 in the 4I13/2–4I15/2. The spontaneous emission probability of this transition is given by:

AJ'J=64 π4e23 h2 J'+1 λ3 n𝑛2+229×Sed+n3×Smd
where h is the Planck constant, m and e are the mass and charge of the electron, c is the velocity of light, n is the refractive index at the mean wavelength, λ, Sed and Smd are the line strengths of the electric-dipole and magnetic-dipole transitions, respectively. The Smd is characteristic of the transition and thus a constant. Thus the second term is not varied with ligand field and dominated only by the refractive index, which has usually about 30% contribution in silicate glasses. That is part of the reason why the Er3+ ions in silicate glasses have a narrow 1.55 m spectrum 〚20〛. In order to get flat emission spectra, it can be effective to increase the relative contribution of the electric-dipole transition, the spectra of which can be varied due to the variations of width of the Stark splitting (J + 1/2), inhomogeneous broadening in amorphous structures and also to sensitivity to the local fields 〚21–23〛. While the line strength of the magnetic-dipole transition is independent of ligand fields, whereas that of the electric-dipole transition is a function of them 〚20, 21〛. According to the Judd–Ofelt theory 〚24, 25〛, the line strength of the electric dipole components of the 1.55-μm transition is given by 〚26〛:

Sed 4I13/2; 4I15/2=∑t=2,4,6 Ωt< 4I13/2∥Ut∥ 4I15/2> 2=0.019 Ω2+0.118 Ω4+1.462 Ω6
where the three coefficients of Ωt are the reduced matrix elements of the unit tensor operators, U(t), calculated in the intermediate-coupling approximation, and Ωt (t = 2,4,6), are the intensity parameters. Since the <SLJ |U(t)| S′L′J′> of other transitions are also a constant characteristic of each transition, three intensity parameters Ωt (t = 2,4,6) can be obtained from more than three measured absorption cross-sections by using the method of least squares fitting 〚27〛. What varies depending on the host composition and structures is the electric-dipole transitions, where the Ω6 parameter is dominant. Excellent spectra with larger cross-section are observed in tellurite 〚6〛 and bismuth-based oxide glasses 〚28〛 (Fig. 3), than aluminosilicates 〚20〛. The higher bond-ionicity is confirmed for the glasses with a large Ω6 of Er3+ by 151Eu Mössbauer spectroscopy, which can be explained by the largest contribution of the overlap integral of 4f and 5d orbitals 〚14, 21〛. Also there may be another possible reason. The relative ratio of the field correction factors (n2 + 2)2/9 n2 takes a minimum at n = 1.414 and increases monotonically with increasing n; i.e., under constant Sed and Smd, the relative contribution of ED transition should increase with increasing n of the host glass, which is not practically the case, because these values are not independent. However, it is empirically true that many glass hosts with a large n show broad Er3+ emission spectra. There are few exceptions from the tendency that the Er3+ ions in those glasses take a large Ω6 value, since the electron polarizability and bond covalency are strongly correlated.

Fig. 3

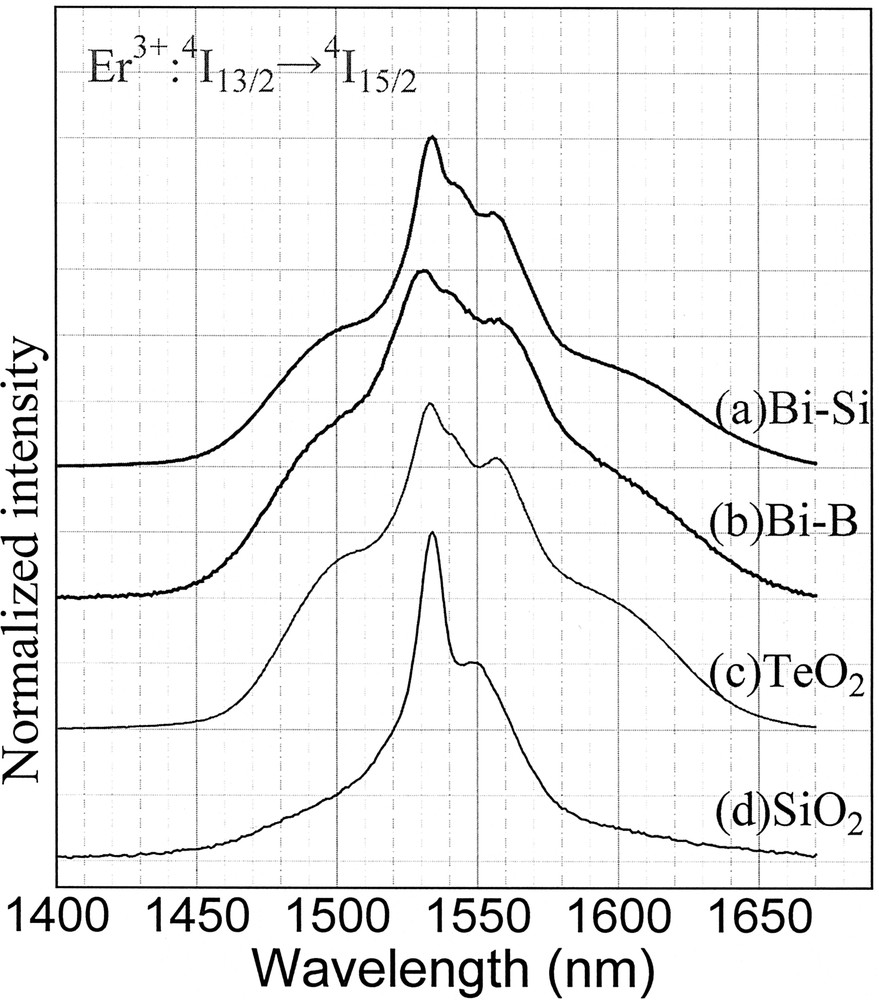
Fluorescence spectra of Er3+-doped glasses; (a) Bi-silicate, (b) Bi-borate, (c) Tellurite and (d) Al-silica.

4 Tm-doped glasses for S-band amplifier

4.1 Background of S-band requirement

Due to the rapid increase of information traffic, great research effort has been paid to development of telecommunication devices for the WDM network system 〚29〛. Because the silica-based transmission fiber has a wide and low-loss window from 1.4 to 1.65 μm, there is an emergent demand for optical amplifiers, which can be used around 1.4 and 1.6 μm, in addition to the present silica-based EDFA. Tellurite-based EDFA was reported to have 80-nm-wide gain up to 1.6 μm (L-band), which also shows various excellent material properties 〚30, 31〛. For the 1.45–1.49-μm band (S+-band), the fluoride-based Tm-doped fiber (TDF) 〚32〛 can be used as an amplifier, although it still presents difficulties compared with the use of EDFA. One of the reasons for inferior performance of TDFA is a longer lifetime of the terminal 3F4 level than that of the initial 3H4 level 〚33〛. The performance of the TDFA is improved by use of an upconversion pumping scheme with a 1.06-μm laser, which produces a population inversion. Codoping of other lanthanide ion, such as Ho3+, was also found to improve the population inversion by means of the energy transfer from the 3F4 level 〚34〛. In addition, a larger branching ratio, β of the 3H4→3H6 band at 0.80 μm than that of the 1.46 μm make it difficult to realize amplification, because the fiber can easily lase at 0.80 μm, resulting in the gain saturation 〚35〛. According to the Judd–Ofelt calculation, β of 0.80 μm is nearly 90%, which is 11 times larger than that of 1.46 μm emission in most glasses 〚36, 37〛. Therefore, the suppression of the 0.80 μm amplified spontaneous emission (ASE) is desirable to avoid lasing at unexpected wavelength for improving the amplifier performance. In either case, in spite of difficulty as practical materials, nonoxide-fiber hosts with lower phonon energy have been used, because the 3H4 level is more easily quenched in high-phonon-energy environment, due to its small energy gap. However, the energy gap of the 3H4 level is not so small as that of the Pr:1G4 for 1.3-μm amplifiers and thus good performance can be expected in some oxide hosts with low phonon energy and better fiberizability.

Fig. 4 shows the relation between the multiphonon decay rate, Wp of the Tm:3H4, Pr:1G4 levels and the inverse phonon energy of various glass hosts. We can see the lower Wp of the Tm:3H4 in tellurite than that of the Pr:1G4 in ZBLAN, even with higher phonon energy.

Fig. 4

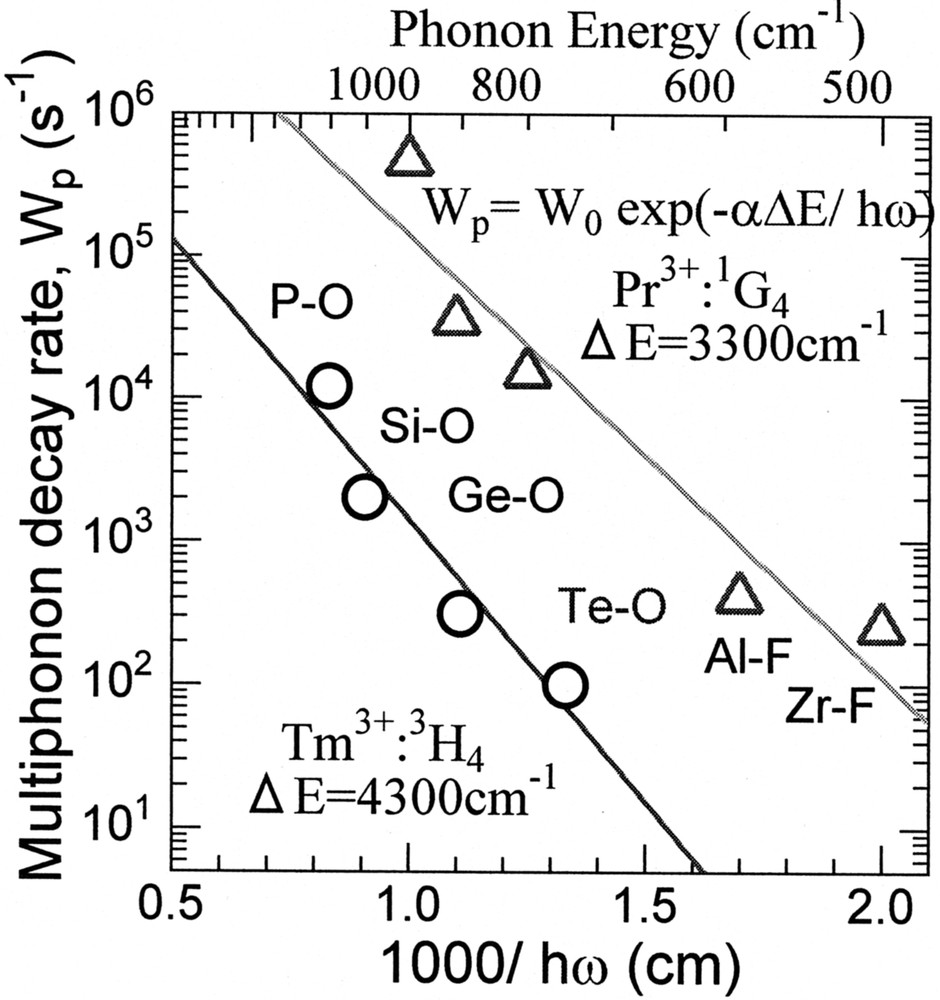
Relationship between multiphonon decay rates of Tm3+:3H4, Pr3+:1G4 levels and inverse phonon energy.

In this chapter, the tellurite glass was chosen as a host because it has relatively low phonon energy, excellent properties for fiber fabrications 〚30, 38〛, and thus can be considered as a candidate material for TDFA.

4.2 Spectroscopy of singly doped glass

4.2.1 Fluorescence spectra

Fig. 5 shows the Tm3+ energy level and a fluorescence spectrum in the tellurite glass, excited at 790 nm. The emission bands at 0.80 μm, 1.46 μm, and 1.80 μm are due to the 3H4→3H6, 3H4→3F4, and 3F4→3H6 transitions, respectively. The wavelength region longer than 1.3 μm is multiplied by 10 times. The area integration was carried out after converting the wavelength into wavenumber scale, which is directly proportional to the energy. The relative intensity ratio of 0.80 to 1.46 μm was about 11, almost unchanged with glass compositions and Tm-concentration. On the other hand, that of 1.46 to 1.80 μm was largely changed with these factors, which is due to the effect of the nonradiative relaxations.

Fig. 5

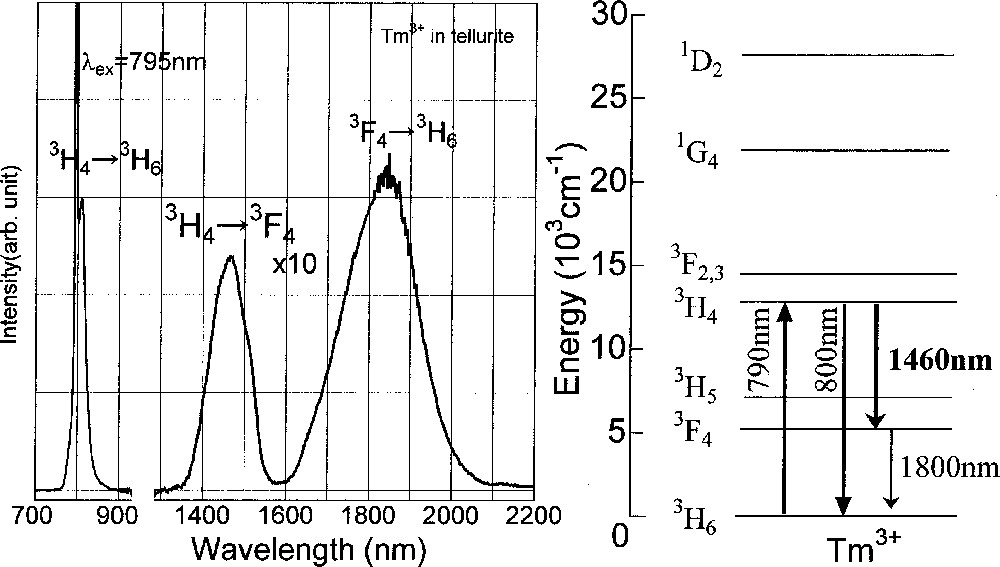
Fluorescence spectra of Tm3+-doped tellurite glass. Energy level of Tm3+ ion is also shown for assignment.

4.2.2 Judd–Ofelt analysis and quantum efficiency in tellurite

The obtained Judd–Ofelt parameters of Tm3+ in the present glass were: Ω2 = 4.69 pm2, Ω4 = 1.83 pm2, Ω6 = 1.14 pm2. Table 1 shows spontaneous emission probabilities, A and β  from the 3H4 level of Tm3+ ions in the tellurite glass. The β of 0.80-μm emission is 11 times larger than that of 1.4-μm emission, which is almost similar to the case in fluoride and other oxide glasses. The calculated τR was 366 μs, while the measured lifetime was 350 μs. This indicates that the quantum efficiency of the 3H4 level is 96% in tellurite glass, which is comparable to that in ZrF4-based fluoride glasses (∼100%).

Table 1

Spontaneous emission probability and branching ratios of Tm3+:3H4 level in the tellurite glass.

TransitionAed (s–1)Amd (s–1)Calculated branch ratio β(%)Ratio of measured emission band
3H4→3H62426–88.8∼11
3H4→3F4229–8.41
3H4→3H554232.8–
Atotal = 2732 s–1, τrad = A–1 = 366 s

4.2.3 Concentration dependence of emission

Fig. 6 shows the Tm2O3-concentration dependence of ratios of the τf(3F4)/τf(3H4) and the integrated intensity of (1.80 μm)/(1.46 μm) of fluorescence spectra. Both ratios increase drastically with increasing the Tm2O3 content. These phenomena are well understood by the so-called ‘Two-for-One Process’ 〚31〛, which is a result of the cross relaxation between two Tm3+ ions; 〚3H4,3H6〛 → 〚3F4,3F4〛 and unfavorable for population inversion between the 3H4 and 3F4 levels. Therefore, a low concentration is desirable to keep a high quantum efficiency of the 3H4 level for 1.4-μm application.

Fig. 6

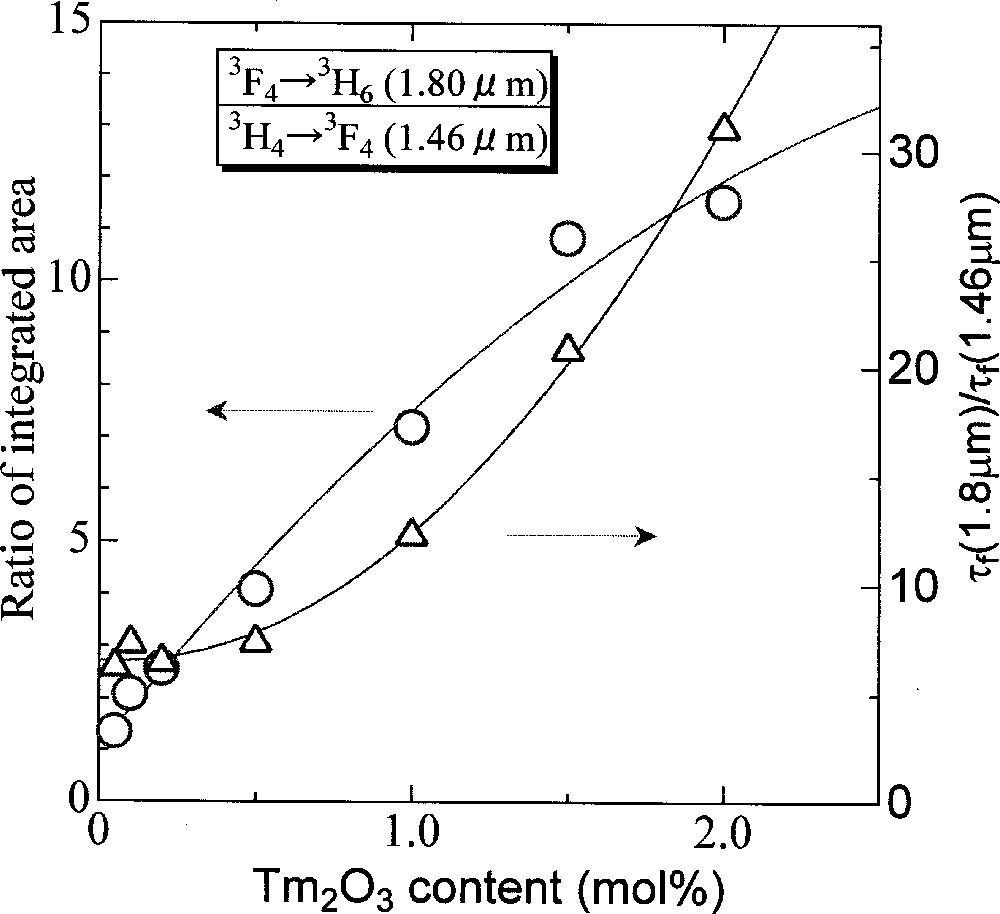
Ratio of integrated area and lifetime of 1.4 μm/1.8 μm in 72 TeO2–20 ZnO–5 Na2O–(2.9 – x) Y2O3–x Tm2O3 glasses.

4.2.4 Temperature dependence

Fig. 7 shows temperature variation of the fluorescence spectra. The peak intensity of the 1.46-μm band increases with lowering temperature, while the line shape of the 1.8-μm band becomes sharp. It can be seen that the mean wavelength of both bands shift to the longer side. The temperature dependence of integrated intensities of both bands is plotted in Fig. 8. The integrated area of the 1.46-μm band is almost unchanged at lower temperature and drops with temperature above 150 K. On the other hand, that of the 1.8-μm band increases slightly and decreases above 250 K. The tendency of the 1.46-μm emission can be explained by considering the temperature dependence of the nonradiative decay from the 3H4 level, which has smaller energy gap to the next lower level. The increasing tendency of the 1.8-μm band is ascribed to the improved population from the upper level by nonradiative processes. Therefore, the lower the temperature is, the better the population inversion becomes. Another advantage of the lower temperature can be the much-improved intensity at 1.50–1.52 μm, which is hardly obtained by conventional EDFA, though not impossible by TDFA 〚39〛.

Fig. 7

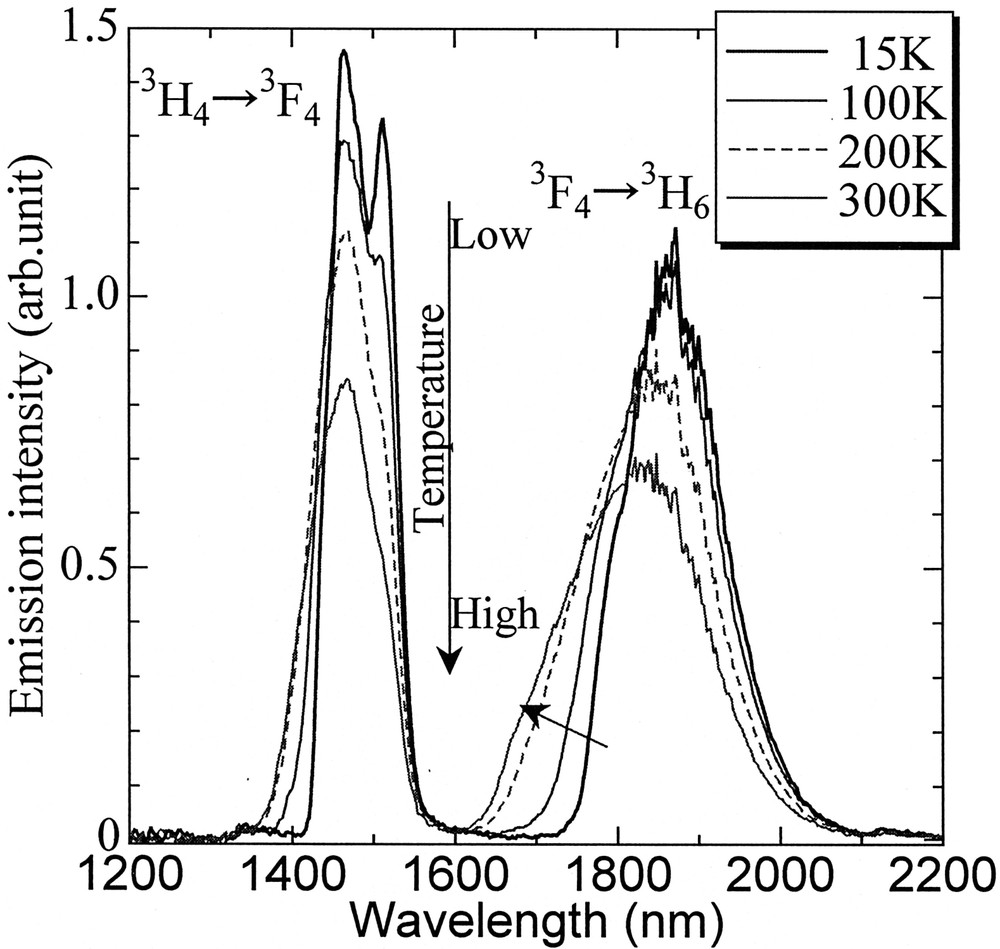
Temperature dependence of fluorescence spectra of 72 TeO2–20 ZnO–5 Na2O–2.9 Y2O3–0.1 Tm2O3 glass.

Fig. 8

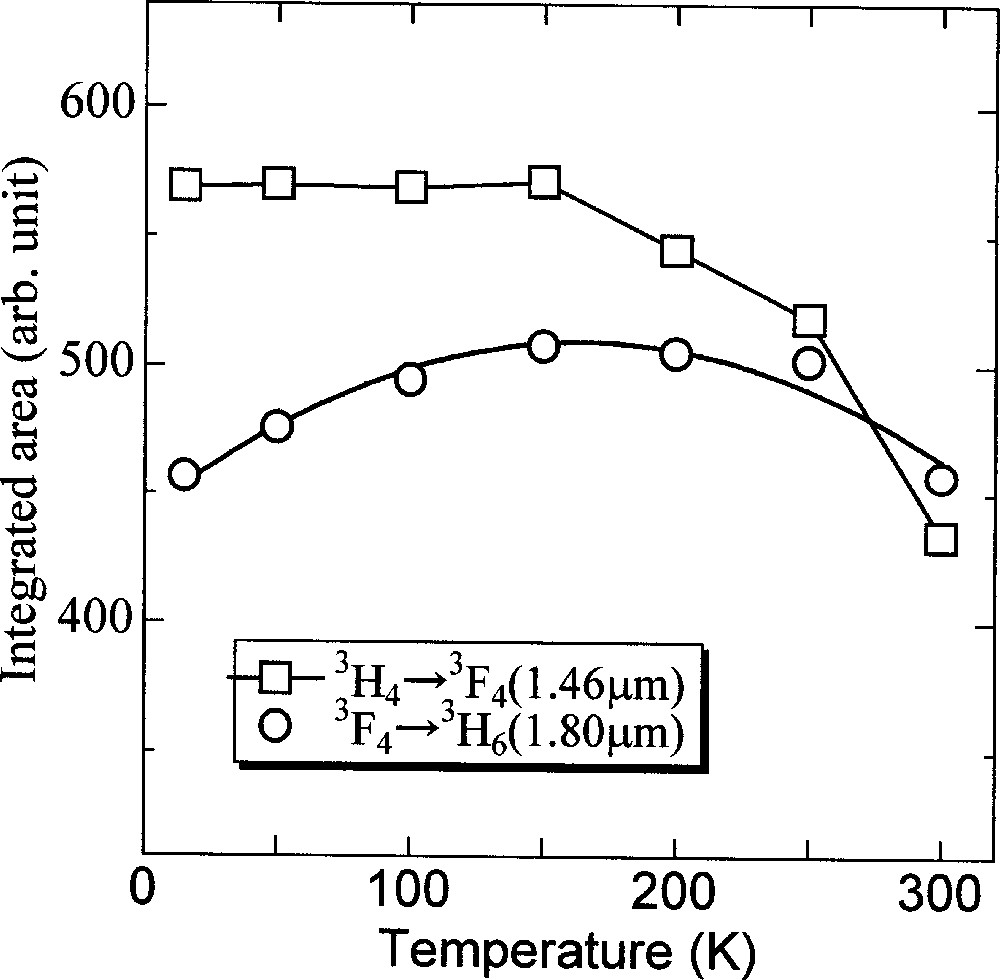
Temperature dependence of the integrated area of the 1.4-μm and 1.8-μm band in the 0.1 Tm2O3 glass.

4.3 Effect of codoping of Ho, Tb, Eu

One of the possibilities to improve the population inversion can be a selective quenching of the terminal level by codoping of other lanthanide 〚34, 40〛, because nothing is better than to pump directly the 3H4 with 0.79-μm LD of AlGaAs rather than upconversion scheme 〚35, 41〛. The role expected for a codopant is to quench the 3F4 level selectively without quenching the 3H4 level. From this viewpoint, the Eu3+, Tb3+ and Ho3+ can be a candidate among 13 4f-active lanthanide ions. The energy level diagrams of these ions are shown in Fig. 9. The Ln-concentration dependence of the lifetimes of the Tm3+:3H4 and 3F4 levels is plotted in Fig. 10. Among three codopants, the Eu3+ ion quenches both levels most significantly and the Ho3+ ion shows the best selectivity; i.e., the least effect on the 3H4 lifetime with a large quenching effect on the 3F4. The variation of the fluorescence spectra of Tm–Ho codoped tellurite glasses are shown in Fig. 11. The spectra are normalized by the intensity of 1.46-μm band, because it showed the least change. We see a drastic decrease of the 1.8-μm band and a rapid increase of the Ho3+:5I7 → 5I8 emission intensity at 2 μm. This result is an evidence of the Tm:3F4 → Ho:5I7 energy transfer.

Fig. 9

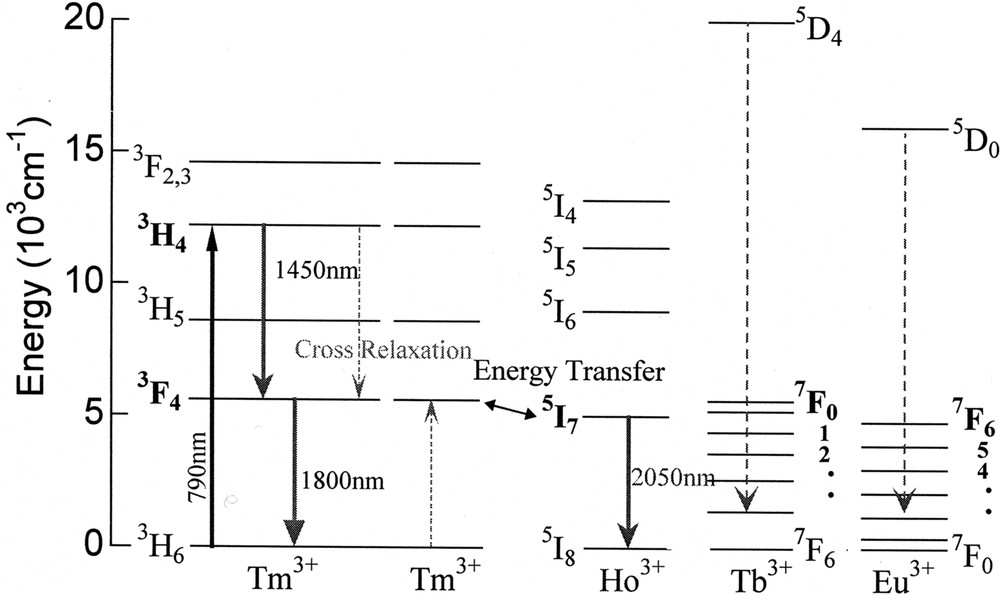
Energy level of Tm3+, Ho3+, Tb3+ and Eu3+ ion.

Fig. 10

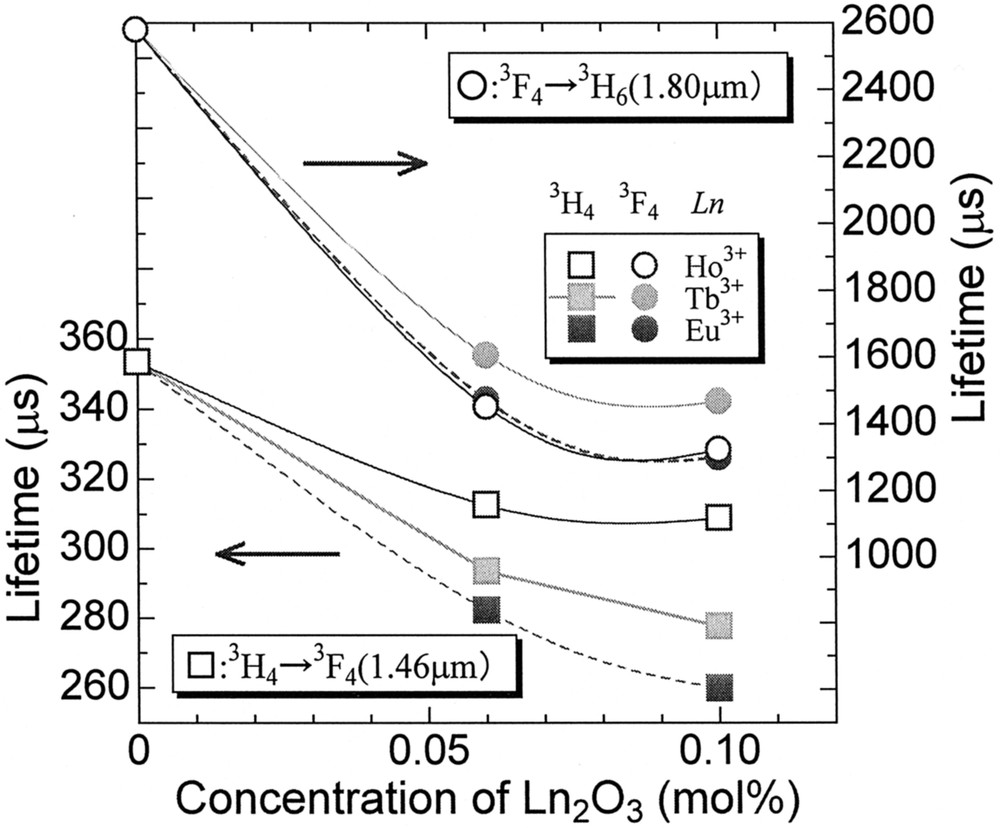
Effect of codopant on the lifetime of Tm3+ levels.

Fig. 11

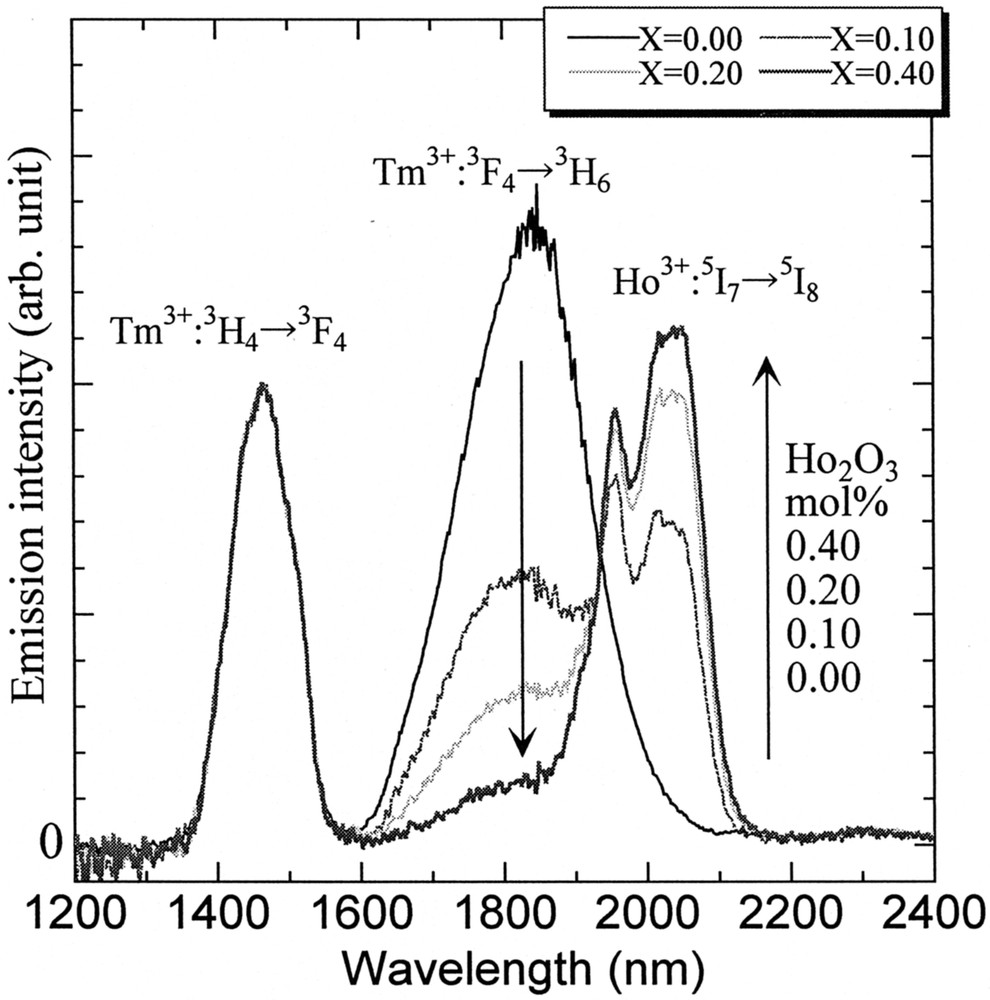
Fluorescence spectra of 72 TeO2–20 ZnO–5 Na2O–(2.9 – x) Y2O3–x Ho2O3–0.1 Tm2O3 glasses.

5 Active ions at 1.3 μm

5.1 Comparison of candidates

The world land-based optical networks installed are composed of 1.3 μm zero-dispersion silica fiber. However, no practical amplifiers have sufficient performance at 1.3-μm like EDFAs at 1.55-μm band. Fig. 12 shows the energy levels of Pr3+, Nd3+, and Dy3+ ions, which are active ions at 1.3 μm. Based on the huge amount of data on laser glasses for 1.06-μm applications 〚42〛, the Nd3+ ion was extensively studied as a candidate at first in many glasses. However, the excited state absorption from the 4F3/2 and the resulting gain shift to 1.35 μm were found to be a problem as well as a small branching ratio compared with that of 1.06 μm, which is a cause of intense amplified spontaneous emission (ASE) 〚43〛. The Pr3+ ion is probably most often studied in fluoride 〚7〛 and chalcogenide glasses 〚8〛 and practical devices have already been produced 〚44〛. The branching ratio from the 1G4 level is usually more than 60% in most glasses 〚45〛. The nonradiative loss due to the small energy gap limits the choice of host to nonoxide glasses. In addition, the small absorption cross-section of the only pumpable level (1G4) at 1.02 μm must be overcome by high-power pumping laser. Nonradiative population from the upper 1D2 level cannot be expected due to its larger energy gap. Since it is difficult to get high-quality lasers at 1.02 μm with semiconductor lasers, an 0.8 μm-LD- pumped Nd:YLiF4 solid-state laser (λ = 1047 nm) is often used as a pumping source 〚45〛. Among various glasses, the Ga–Na–S system has the highest quantum efficiency of 62% and the largest gain per pumping power at 1.34 μm 〚8〛. However, the gain at 1.31 μm is still low partly due to the peak shift to longer wavelength in sulfides 〚8〛.

Fig. 12

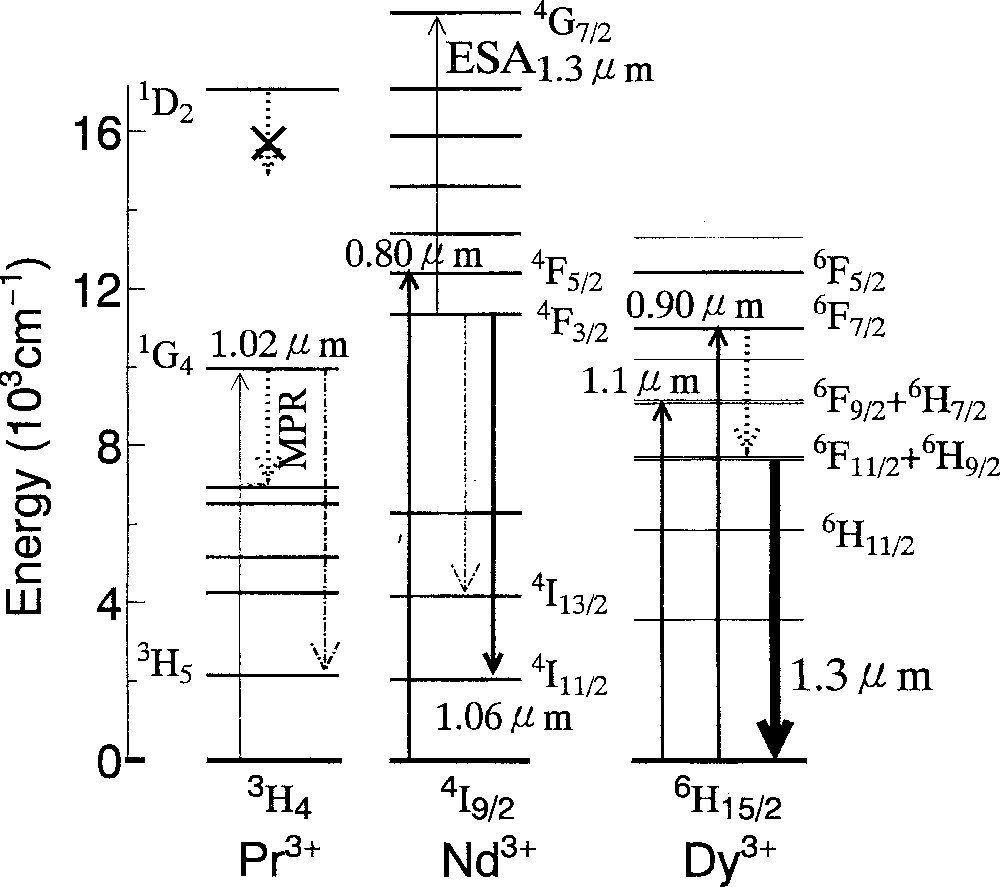
Energy level of Pr3+, Nd3+ and Dy3+ ion.

Compared with these two ions, the Dy3+ has unique properties, though it is also affected by multiphonon loss. The Dy3+ ion can be pumped by 0.8 μm, 0.9 μm, 1.1 μm or 1.28 μm with large cross sections, for which the high-power LD’s can be used. Table 2 shows the reduced matrix elements of 1.3-μm emission transitions for Pr3+, Nd3+ and Dy3+ ions 〚11〛. While the transition probabilities in Pr3+ and Nd3+ ions depend most largely on Ω6, that in Dy3+ is dominated by the Ω2 parameter, which is most sensitive to the local symmetry and thus to the glass composition. The transition, the cross section of which is very sensitive to the local structure, is called ‘hypersensitive transition’ 〚46〛. The 615-nm emission of the Eu3+ ion, used for red-phosphor, is a typical example, where the <|U(2)|>2 is the largest among the three <|U(t)|>2 〚47〛.

Table 2

Reduced matrix elements of 1.3-μm emission transitions for Pr3+, Nd3+ and Dy3+ ions 〚11〛.

Ln3+4fNaJbJ′<U(2)>2<U(4)>2<U(6)>2λ (μm)
Pr3+21G43H50.03070.07150.33441.32
Nd3+34F3/24I13/2000.21171.35
Dy3+96H9/2 + 6F11/26H15/20.93940.84650.40781.32

5.2 Spectroscopy of dysprosium in glasses

5.2.1 Judd–Ofelt parameters and optical transitions

Absorption spectra of Dy3+ in the fluoride and tellurite glasses are shown in Fig. 13, where the assignment of transitions is also indicated 〚11〛. We can see that the absorption coefficients of transition peaks in this near-infrared region are not so distinct from each other for the fluoride glass. On the other hand, in the tellurite glass the intensity of 6F11/2+6H9/2 band is the largest among all transitions. The Ωt parameters obtained for the glasses are shown in Fig. 14. The Ω2 value is the largest in the sulfide and tellurite glasses and decreases in the order Ga2S3 > TeO2 > ZrF4 > InF3, whereas Ω6 increases in this order. The change in the absorption cross-section of the 6F11/2+6H9/2 band due to glass compositions can be ascribed to the set of the reduced matrix elements 〚11〛. The <6F11/2|U(2)|6H15/2>2 is the largest among three <|U(t)|>2 (t = 2, 4, 6), thus the SJJ′ of this band is dependent on the Ω2 of Dy3+ ion in the host. The <|U(2)|>2 of other transitions are small or zero and the SJJ′ of them is dependent mainly on Ω6. It is known that  Ω2 is affected by the local symmetry of ligand field and bond covalency 〚15〛. The electronegativity of the anions is as follows; S < O < F 〚48〛. Therefore, the chemical bond covalency between the Dy3+ and the ligand varies with compositions: Ga2S3 > TeO2 > ZrF4 > InF3. Therefore, the opposite tendency of Ω2 and  Ω6 against glass compositions can be understood in terms of the differences in the bond covalency in the glasses 〚37〛.

Fig. 13

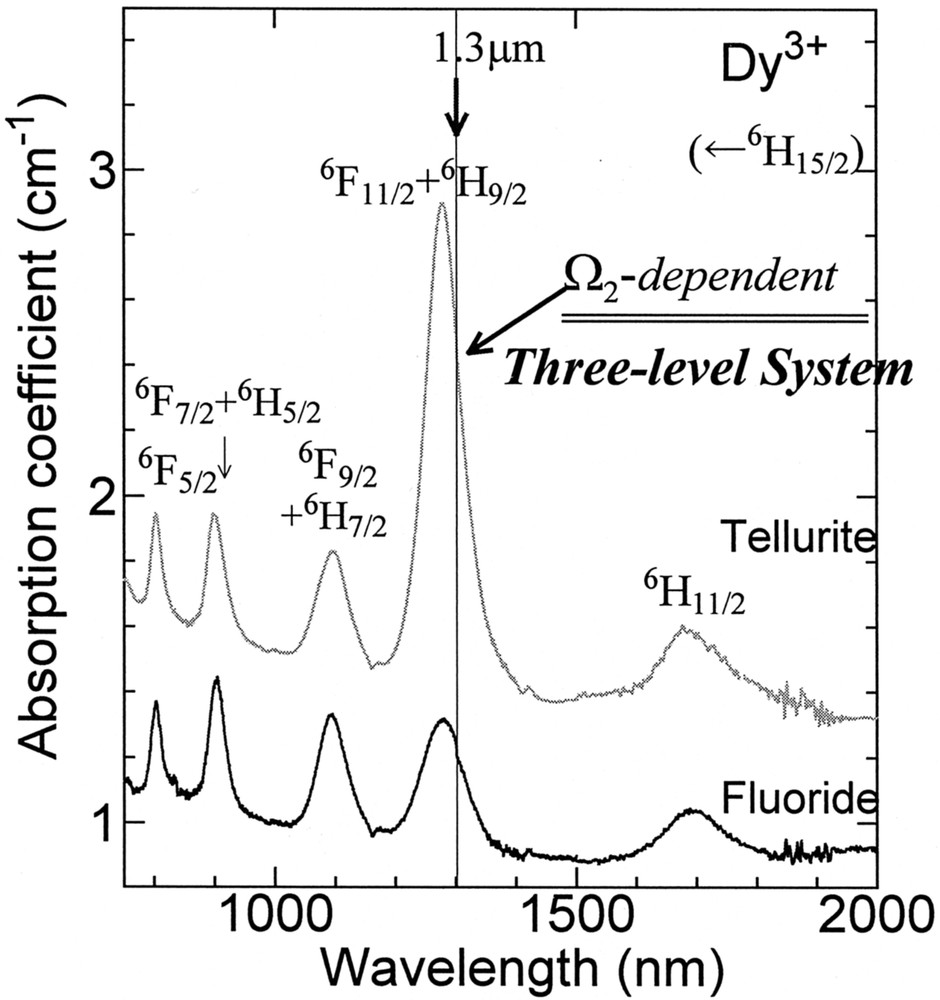
Absorption spectra of Dy3+-doped glasses.

Fig. 14

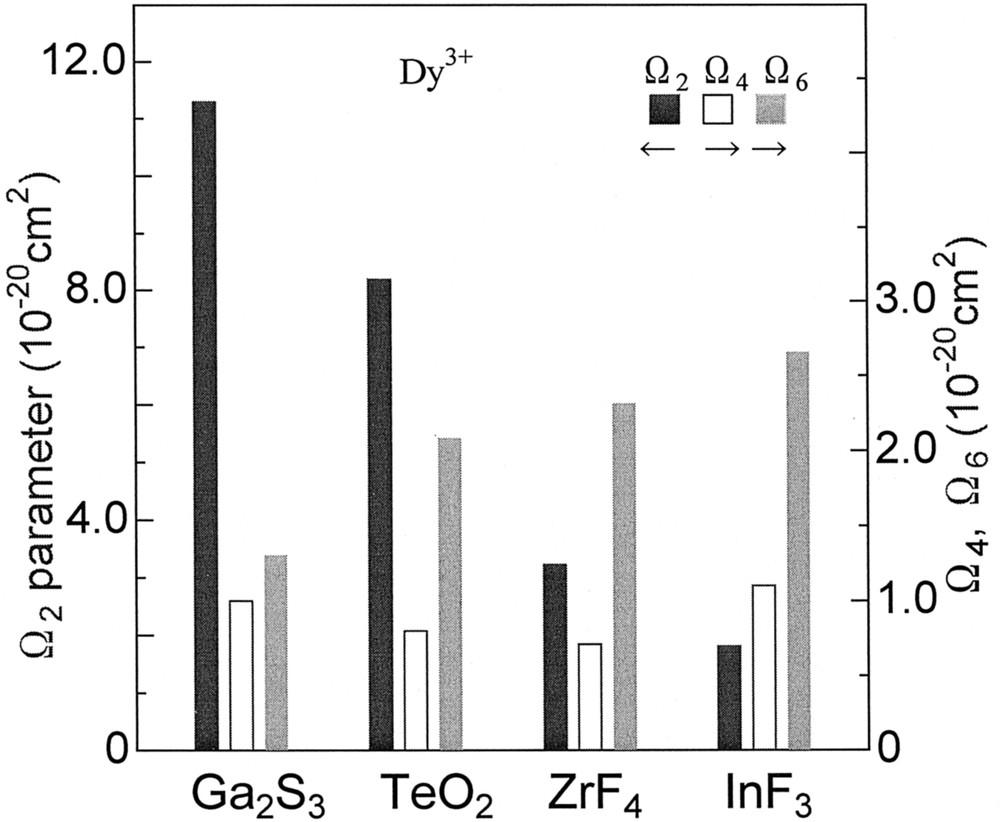
Ωt parameters of Dy3+ ions in glasses.

5.2.2 Can dysprosium be a candidate?

Because of the large branching ratio and cross section of 1.3 μm, an efficient amplifier can be expected if the nonradiative loss were reduced. Table 3 shows the lifetime and quantum efficiency of Dy3+ in several glasses. A selenide glass host can give 90% quantum efficiency 〚49〛, which is mainly due to the phonon energy being much smaller than even sulfides. This efficiency seems high enough for practical devices. Moreover, smaller electron-phonon coupling strength in chalcogenides 〚50〛 seems more promising for smaller multiphonon loss at levels with a small energy gap than in a host with comparable phonon energy, such as chlorides.

Table 3

Lifetime and quantum efficiency of the Dy3+ level for 1.3-μm emission in several glass systems.

HostLifetime (μs)Quantum efficiency
Pb–Bi–Ga–O51.5% 〚53〛
Ge–Ga–S3817% 〚12〛
Ga–La–S5919% 〚13〛
Ge–As–Se30090% 〚49〛

One more problem of Dy3+ is bottlenecking at lower levels with longer lifetimes resulting in depression of pumping efficiency and large emission intensities at longer-wavelength side 〚51〛, because the energy gap of the lower levels is larger than that of the 1.3-μm level. If the quantum efficiency of the 1.3-μm level is large enough, the effect of bottlenecking at lower levels would be negligible, since the excited initial state relaxes directly to the ground state due to the branching ratio of more than 90% 〚52〛. There seems a great potential in Dy3+-doped chalcogenides. Further progress in the fabrication of low-loss single-mode fiber is expected.

6 Conclusions

The broad and flat spectral features of Er3+:1.55 μm transition can be obtained in novel glass compositions with a large Ω6 parameter. The Er3+ spectral change is accompanied by compositional evolution of Er–O bond ionicity and local structure.

The Tm3+:1.46 μm transition shows high quantum efficiency in heavy metal oxide glasses due to the larger energy gap of the 3H4 level than the Pr3+:1G4. Selective quenching of the terminal level is possible in a Ho-codoped system.

New materials are expected for the optical amplifiers in the future WDM network system, to control the 4f transitions of RE ions, which show a variety of spectroscopy in glasses.

Bibliographie

[〚1〛] R.J. Mears; L. Reekie; I.M. Jauncey; D.N. Payne Tech. Digest OFC/IOOC'87, Reno, Nevada, 1987, p. 167

[〚2〛] C.J. Koestner; E. Snitzer Appl. Opt., 3 (1964), p. 1182

[〚3〛] S.B. Poole; D.N. Payne; M.E. Fermann Electron. Lett., 21 (1985), p. 737

[〚4〛] E. Snitzer; H. Po; F. Hakimi; R. Tumminelli; B.C. McCollum Tech. Digest OFCˈ88, 1988, p. 447

[〚5〛] E. Snitzer; R. Woodcock Appl. Phys. Lett., 6 (1965), p. 45

[〚6〛] A. Mori; Y. Ohishi; M. Yamada; H. Ono; Y. Nishida; K. Oikawa; S. Sudo OFCˈ97, 1997, PD1

[〚7〛] Y. Ohishi; T. Kanamori; T. Kitagawa; S. Takahashi; E. Snitzer; G.H. Sigel Jr Opt. Lett., 16 (1991), p. 1747

[〚8〛] E. Ishikawa; H. Tawarayama; K. Ito; H. Aoki; H. Yanagita; H. Toratani Tech. Rep. IECIE, 1996 (OPE96-111)

[〚9〛] K. Wei; D.P. Machewirth; J. Wenzel; E. Snitzer; G.H. Sigel Opt. Lett., 19 (1994), p. 904

[〚10〛] D.W. Hewak; B.N. Samson; J.A. Mederios Neto; R.I. Laming; D.N. Payne Electron. Lett., 30 (1994), p. 968

[〚11〛] S. Tanabe; T. Hanada; M. Watanabe; T. Hayashi; N. Soga J. Am. Ceram. Soc., 78 (1995), p. 2917

[〚12〛] http://www.joho.soumu.go.jp/pressrelease/japanese/tsusin/000615j502.html

[〚13〛] J. Ballato; R. Riman; E. Snitzer J. Non-Cryst. Solids, 213–214 (1997), p. 126

[〚14〛] S. Tanabe; K. Hirao; N. Soga J. Non-Cryst. Solids, 113 (1989), p. 178

[〚15〛] S. Tanabe; K. Hirao; N. Soga J. Non-Cryst. Solids, 142 (1992), p. 148

[〚16〛] S. Tanabe; S. Todoroki New Glass, 7 (1992), p. 189

[〚17〛] S. Tanabe; K. Suzuki; N. Soga; T. Hanada J. Lumin., 65 (1995), p. 247

[〚18〛] C.A. Bracket; J. Lightwave Technol., 14 (1996), p. 936

[〚19〛] W.T. Carnal; P.R. Fields; K. Rajnak J. Chem. Phys., 49 (1968), p. 4424

[〚20〛] S. Tanabe; T. Hanada J. Non-Cryst. Solids, 196 (1996), p. 101

[〚21〛] S. Tanabe; T. Ohyagi; S. Todoroki; T. Hanada; N. Soga J. Appl. Phys., 73 (1993), p. 8451

[〚22〛] S. Tanabe; T. Hanada; T. Ohyagi; N. Soga Phys. Rev. B, 48 (1993), p. 10591

[〚23〛] S. Tanabe; S. Yoshii; K. Hirao; N. Soga Phys. Rev. B, 45 (1992), p. 4620

[〚24〛] B.R. Judd Phys. Rev., 127 (1962) no. 3, p. 750

[〚25〛] G.S. Ofelt J. Chem. Phys., 37 (1962) no. 3, p. 511

[〚26〛] M.J. Weber Phys. Rev., 157 (1967), p. 262

[〚27〛] S. Tanabe; T. Ohyagi; N. Soga; T. Hanada Phys. Rev. B, 46 (1992), p. 3305

[〚28〛] N. Sugimoto; S. Tanabe; S. Ito; T. Hanada Proc. 10th Meeting Glasses for Photonics, Tokyo, January 1999, p. 32

[〚29〛] H. Taga Tech. Digest 10th Optical Amplifiers and their Applications, OSA, Washington, DC, June 1999, WC1, 1999, p. 22

[〚30〛] A. Mori; Y. Ohishi; S. Sudo Electron. Lett., 33 (1997) no. 10, p. 863

[〚31〛] J.S. Wang; E.M. Vogel; E. Snitzer Opt. Mater., 3 (1994), p. 187

[〚32〛] T. Sakamoto; A. Aozasa; T. Konamori; K. Hoshino; M. Yamada; M. Shimizu Tech. Digest 10th Optical Amplifiers and their Applications, OSA, Washington DC, June, 1999, WD2-1, p. 50

[〚33〛] R.M. Percival; D. Szebesta; J.R. Williams Electron. Lett., 30 (1994) no. 13, p. 1057

[〚34〛] T. Sakamoto; M. Shimizu; T. Kanamori; Y. Terunuma; Y. Ohishi; M. Yamada; S. Sudo IEEE Photonics Tech. Lett., 7 (1995) no. 9, p. 983

[〚35〛] T. Komukai; T. Yamamoto; T. Sugawa; Y. Miyajima IEEE J. Quantum Electron., 31 (1995) no. 11, p. 1880

[〚36〛] S. Tanabe; K. Suzuki; N. Soga; T. Hanada J. Opt. Soc. Am. B, 11 (1994) no. 5, p. 933

[〚37〛] S. Tanabe; K. Tamai; K. Hirao; N. Soga Phys. Rev. B, 53 (1996) no. 13, p. 8358

[〚38〛] S. Tanabe; T. Kouda; T. Hanada Opt. Mater., 12 (1999), p. 35

[〚39〛] T. Kasamatsu; Y. Yano; H. Sekita Opt. Lett., 24 (1999) no. 23, p. 1684

[〚40〛] P.M. Percival; D. Szebesta; S.T. Davey Electron. Lett., 29 (1993) no. 12, p. 1054

[〚41〛] S. Tanabe; K. Tamai; K. Hirao; N. Soga Phys. Rev. B, 47 (1993) no. 5, p. 2507

[〚42〛] S.E. Stokowsky; R.A. Saroyan; M.J. Weber Nd-doped laser glass spectroscopic and physical properties, Lawrence Libermore National Laboratories, University of California, CA, 1981

[〚43〛] S.G. Grubb; W.L. Barnes; E.R. Taylor; D.N. Payne Electron. Lett., 26 (1990), p. 121

[〚44〛] T. Whitley; R. Wyatt; D. Szebesta; S. Davey; J.R. Williams Tech. Digests OAAˈ92, Washington, DC, 1992, PD4

[〚45〛] Y. Ohishi; J. Temmyo Bull. Ceram. Soc. Jpn, 28 (1993), p. 110

[〚46〛] C.K. Jørgensen; B.R. Judd Mol. Phys., 8 (1964), p. 281

[〚47〛] M.J. Weber Optical Properties of Ions in Crystals (H.M. Crosswhite; H.M. Moos, eds.), Wiley-Interscience, New York, USA, 1966, p. 467

[〚48〛] L. Pauling The Nature of Chemical Bond, Cornel University Press, Ithaca, New York, 1960, p. 93

[〚49〛] L.B. Shaw; B.J. Cole; J.S. Sanghera; I.D. Aggarwal; D.T. Schaafsma OFC'98 Tech. Digest, WG8, 1998, p. 141

[〚50〛] S. Tanabe Science of Rare Earths (G. Adachi, ed.), Kagaku-Dojin Kyoto, 1999, p. 780 (in Japanese)

[〚51〛] B.N. Samson; T. Schweitzer; D.W. Hewak; R.I. Laming Opt. Lett., 22 (1997), p. 703

[〚52〛] J. Heo; Y.B. Shin; H.-S. Kim, Tech. Digest OFC'99, San Diego, CA, USA, February 1999 (#WG7, p. 120)

[〚53〛] Y.-G. Choi; J. Heo J. Non-Cryst. Solids, 217 (1997), p. 199

Cité par

  • Riccardo Ballarini; Stefano Taccheo Numerical Investigation of Emission Properties and Pump Noise Transfer Functions of an Yb3+:Er3+:Tm3+:Ho3+ Co-Doped Glass, Journal of Lightwave Technology, Volume 43 (2025) no. 6, p. 2764 | DOI:10.1109/jlt.2024.3509995
  • Nguyen Thi Quynh Lien; Le Xuan Hung; Nguyen Thi Phuong Thao; Tran Thi Hong; Trinh Ngoc Dat; Nguyen Ngoc Trac; Phan Van Do; Ho Van Tuyen Reduction process and energy transfer from Eu3+/Sm3+ co-doped Sr2MgSi2O7 phosphors by heat treatment in 100 | DOI:10.1016/j.jpcs.2025.112591
  • Florian Calzavara; V.A.G. Rivera; Alexandre Fargues; Jean-Paul Salvetat; Marc Dussauze; Younès Messaddeq; Théo Guérineau; Véronique Jubera; Thierry Cardinal; Évelyne Fargin Dehydration processing of Er3+-doped barium gallo-germanate glasses with ultra-low hydroxyl absorption for near and mid-infrared applications, Optical Materials, Volume 165 (2025), p. 117096 | DOI:10.1016/j.optmat.2025.117096
  • Najla Khaled Almulhem; Hayat. H. Almulhim; M. A. Farag; Aly Saeed Efficient red-NIR laser of Er3+/Yb3+, Er3+/Nd3+, and Er3+/Ce3+ co-doped in oxyfluorophosphate glass, Scientific Reports, Volume 15 (2025) no. 1 | DOI:10.1038/s41598-025-89190-y
  • Ke Zhang; Quan Dong; Jingfei Chen; Tianxia Wei; Dazhao Wang; Hongwei Li; Dianhao Hou; Jianfeng Yan; Xueliang Li; Xu Feng; Shifeng Zhou Cluster Control for Construction of Bismuth‐Doped Glass Fiber with Broadband Optical Response, Advanced Physics Research, Volume 3 (2024) no. 4 | DOI:10.1002/apxr.202300141
  • Beibei Xu; Chaoyuan Jin; Jae‐Seong Park; Huiyun Liu; Xing Lin; Junjie Cui; Daoyuan Chen; Jianrong Qiu Emerging near‐infrared luminescent materials for next‐generation broadband optical communications, InfoMat, Volume 6 (2024) no. 8 | DOI:10.1002/inf2.12550
  • Wenwen Fan; Junfeng Xu; Zhirui Yao; Na Li; Xuyang Li The temperature variation of different cooling methods for the preparation of chalcogenide glasses, Infrared Physics Technology, Volume 136 (2024), p. 105083 | DOI:10.1016/j.infrared.2023.105083
  • I. Fuks-Janczarek; R. Miedzinski; Luciana R.P. Kassab Optical characterization and thermal analysis of rare earth-doped PbO-GeO2-Ga2O3 glasses embedded with gold nanoparticles, Journal of Alloys and Compounds, Volume 1002 (2024), p. 175221 | DOI:10.1016/j.jallcom.2024.175221
  • Weiwei Chen; Xiongjian Huang; Quan Dong; Puxian Xiong; Dandan Yang; Jianrong Qiu; Zhongmin Yang; Guoping Dong Tunable ultra-broadband multi-band NIR emission in Bi-doped aluminogermanate glasses and fibers via controllable Al2O3 content for broadband amplifiers, Journal of Materials Chemistry C, Volume 12 (2024) no. 2, p. 459 | DOI:10.1039/d3tc03315e
  • Quan Dong; Xu Feng; Jianrong Qiu; Shifeng Zhou Main Group Elements Activated Near‐Infrared Photonic Materials, Laser Photonics Reviews, Volume 18 (2024) no. 6 | DOI:10.1002/lpor.202301053
  • Karolina Kowalska; Marta Kuwik; Tomasz Goryczka; Joanna Pisarska; Wojciech A. Pisarski The impact of pair Er3+/Yb3+ on titanate-germanate glasses: Physicochemical and near-infrared luminescence investigations, Materials Science and Engineering: B, Volume 301 (2024), p. 117117 | DOI:10.1016/j.mseb.2023.117117
  • Shiwen Song; Min Zhang Broadband Near-Infrared Emission from Bi/Cr Co-Doped Aluminosilicate Glasses, Micromachines, Volume 15 (2024) no. 9, p. 1093 | DOI:10.3390/mi15091093
  • Hosam M. Gomaa; H. A. Saudi; H. Y. Zahran; I. S. Yahia RETRACTED ARTICLE: Differences between CeO2-doped sodium borate glass and Cu2O3-doped sodium borate glass in terms of structural defects concentration and plasmon frequency, Optical and Quantum Electronics, Volume 56 (2024) no. 2 | DOI:10.1007/s11082-023-05819-0
  • Yixin Wang; Quan Xie; Chun Jiang Modulation of the emission spectrum of rare-earth ions using inverse-designed photonic crystals cavities, Optics Express, Volume 32 (2024) no. 3, p. 4346 | DOI:10.1364/oe.509912
  • B. V. Padlyak; I. I. Kindrat; V. T. Adamiv; A. Drzewiecki; B. Cieniek; I. Stefaniuk Structural and spectroscopic studies of lithium tetraborate glass co-doped with Sm and Cu, Physical Chemistry Chemical Physics, Volume 26 (2024) no. 33, p. 22006 | DOI:10.1039/d4cp01633e
  • E. M. Ghardi; A. Scrimshire; R. Smith; P. A. Bingham; S. C. Middleburgh; W. E. Lee; M. J. D. Rushton First-principles study of lithium aluminosilicate glass scintillators, Physical Chemistry Chemical Physics, Volume 26 (2024) no. 7, p. 6138 | DOI:10.1039/d3cp05576k
  • Takahiro Ohkubo; Shunta Sasaki; Atsunobu Masuno; Eiji Tsuchida Ab Initio Molecular Dynamics Study of Trivalent Rare Earth Rich Borate Glasses: Structural Insights and Formation Mechanisms, The Journal of Physical Chemistry B, Volume 128 (2024) no. 47, p. 11800 | DOI:10.1021/acs.jpcb.4c05039
  • Veeramani Rajendran; Wen-Tse Huang; Kuan-Chun Chen; Da-Hua Wei; Ho Chang; Ru-Shi Liu Shortwave Infrared Luminescence of Tetravalent Chromium and Divalent Nickel: Phosphor Design Principles and Applications, ACS Applied Optical Materials, Volume 1 (2023) no. 6, p. 1063 | DOI:10.1021/acsaom.2c00182
  • Veeramani Rajendran; Kuan-Chun Chen; Wen-Tse Huang; Natalia Majewska; Tadeusz Leśniewski; Maciej Grzegorczyk; Sebastian Mahlik; Grzegorz Leniec; Sławomir Maksymilian Kaczmarek; Wei Kong Pang; Vanessa K. Peterson; Kuang-Mao Lu; Ho Chang; Ru-Shi Liu Pentavalent Manganese Luminescence: Designing Narrow-Band Near-Infrared Light-Emitting Diodes as Next-Generation Compact Light Sources, ACS Energy Letters, Volume 8 (2023) no. 1, p. 289 | DOI:10.1021/acsenergylett.2c02403
  • Ke Zhang; Jingfei Chen; Quan Dong; Tianxia Wei; Dazhao Wang; Xueliang Li; Xu Feng; Zhixue He; Jianrong Qiu; Shifeng Zhou Broadband Optical Amplification in Bi‐Doped Multicomponent Glass Fiber, Advanced Materials Technologies, Volume 8 (2023) no. 12 | DOI:10.1002/admt.202202042
  • Asmahani Awang; S. K. Ghoshal; Alireza Samavati Spectroscopic Studies of Rare-Earth-Doped Glasses for LED Applications, Advanced Materials for Solid State Lighting, Volume 25 (2023), p. 225 | DOI:10.1007/978-981-99-4145-2_9
  • Jakub Markiewicz; Tomasz Ragin; Magdalena Leśniak; Karolina Sadowska; Jacek Żmojda; Piotr Miluski; Wojciech A. Pisarski; Joanna Pisarska; Patryk Szymczak; Bartosz Handke; Jan Dorosz; Marcin Kochanowicz; Dominik Dorosz Spectroscopic properties of the silicate-gallo-germanate glasses and glass-ceramic optical fiber co-doped with Ni2+/Er3+, Ceramics International, Volume 49 (2023) no. 24, p. 41222 | DOI:10.1016/j.ceramint.2023.02.195
  • Devarajulu Gelija; B. Kiran Kumar; P. Reddi Babu; Yuwaraj K. Kshetri; Tae-Ho Kim; B. Deva Prasad Raju; Moon-Deock Kim Study of Eu3+–Gd3+ co-doped Ba–Bi–B glasses for red-laser applications: Physical, structural, photoluminescence and time-resolved spectral characteristics, Ceramics International, Volume 49 (2023) no. 4, p. 5872 | DOI:10.1016/j.ceramint.2022.10.208
  • Bilel Charfi; Mohamed Zekri; Andreas Herrmann; Kamel Damak; Ramzi Maâlej Atomic scale network structure of a barium aluminosilicate glass doped with different concentrations of rare-earth ions explored by molecular dynamics simulations, Computational Materials Science, Volume 218 (2023), p. 111965 | DOI:10.1016/j.commatsci.2022.111965
  • Ho Van Tuyen; Tran Thi Hong; Le Van Thanh Son; Nguyen Thi Quynh Lien; Ramachari Doddoji; Huynh Bao Nguyen; Le Vu Truong Son Effects of Ce3+/Sm3+ co-doping as a luminescent modifier in borotellurite glasses for solid-state lighting, Journal of Materials Science: Materials in Electronics, Volume 34 (2023) no. 10 | DOI:10.1007/s10854-023-10265-5
  • Vishab Kesarwani; Vineet Kumar Rai Impact of AgNPs on the optical thermometry and stability of bismuth modified tellurium-tungstate upconverting glass, Journal of Non-Crystalline Solids, Volume 603 (2023), p. 122129 | DOI:10.1016/j.jnoncrysol.2022.122129
  • Santosh Kumar; K. Singh; Devender Kumar SiO2/B2O3 glass formers effect on transparency and mechanical properties of soda-lime borosilicate glasses for automobile applications, Journal of Non-Crystalline Solids, Volume 618 (2023), p. 122530 | DOI:10.1016/j.jnoncrysol.2023.122530
  • R. Ramaraghavulu; K. Pavani; P. C. Nagajyothi; Jaesool Shim Excitation‐dependent energy transfer and color tunability in Dy3+/Eu3+ co‐doped multi‐component borophosphate glasses, Journal of the American Ceramic Society, Volume 106 (2023) no. 6, p. 3509 | DOI:10.1111/jace.19028
  • Weiwei Chen; Yafei Wang; Jing Zhang; Baotian Qiu; Jianrong Qiu; Guoping Dong Ultra‐broadband and thermally stable NIR emission in Bi‐doped glasses and fibers enabled by a metal reduction strategy, Journal of the American Ceramic Society, Volume 106 (2023) no. 7, p. 4128 | DOI:10.1111/jace.19071
  • Vishab Kesarwani; Vineet Kumar Rai Effect of adding TiO2 as modifier on the optical thermometric ability of tellurium tungstate glass, Materials Research Bulletin, Volume 167 (2023), p. 112445 | DOI:10.1016/j.materresbull.2023.112445
  • Yu Wang; Siyi Wang; Arindam Halder; Jayanta Sahu (INVITED) Bi-doped optical fibers and fiber amplifiers, Optical Materials: X, Volume 17 (2023), p. 100219 | DOI:10.1016/j.omx.2022.100219
  • Adam Shearer; Brittney Hauke; Maziar Montazerian; John C. Mauro A critical review of infrared transparent oxide glasses, Optical Materials: X, Volume 20 (2023), p. 100258 | DOI:10.1016/j.omx.2023.100258
  • Gabriella Tessitore; Gabrielle A. Mandl; Steven L. Maurizio; Mannu Kaur; John A. Capobianco The role of lanthanide luminescence in advancing technology, RSC Advances, Volume 13 (2023) no. 26, p. 17787 | DOI:10.1039/d3ra00991b
  • R. Rajaramakrishna; N. Intachai; S. Kothan; J. Kaewkhao Tri-doped Ln3+ ions in barium zinc borate glasses: Luminescence behavior at room and cryogenic temperatures, Results in Optics, Volume 12 (2023), p. 100428 | DOI:10.1016/j.rio.2023.100428
  • Hüseyin Can Çamiçi; Théo Guérineau; V. A. G. Rivera; Rodrigo Ferreira Falci; Sophie LaRochelle; Younès Messaddeq The role of tungsten oxide in Er3+-doped bismuth-germanate glasses for optical amplification in L-band, Scientific Reports, Volume 13 (2023) no. 1 | DOI:10.1038/s41598-023-35995-8
  • Piotr Miluski; Krzysztof Markowski; Marcin Kochanowicz; Marek Łodziński; Jacek Żmojda; Wojciech A. Pisarski; Joanna Pisarska; Marta Kuwik; Magdalena Leśniak; Dominik Dorosz; Tomasz Ragiń; Valiantsin Askirka; Jan Dorosz Tm3+/Ho3+ profiled co-doped core area optical fiber for emission in the range of 1.6–2.1 µm, Scientific Reports, Volume 13 (2023) no. 1 | DOI:10.1038/s41598-023-41097-2
  • Patarawagee Yasaka; Kitipun Boonin; Jakrapong Kaewkhao; Rajanavaneethakrishna Rajaramakrishna; Suchart Kothan Effect of Erbium Oxide on Luminescence and Spectroscopy Properties of a Zinc Barium Boro‐Tellurite Glass System for Photonic Applications, physica status solidi (a), Volume 220 (2023) no. 10 | DOI:10.1002/pssa.202200438
  • G. Devarajulu; B. Kiran Kumar; P. Reddi Babu; M. Dhananjaya; Na-hyun Bak; Kedhareswara Sairam Pasupuleti; B. Deva Prasad Raju; Moon-Deock Kim Sensitization effect of Nd3+ ions on Yb3+/Nd3+ co-doped oxyfluoride glasses and study of their optical, fluorescence, and upconversion abilities for visible laser and NIR amplifier applications, Ceramics International, Volume 48 (2022) no. 17, p. 24550 | DOI:10.1016/j.ceramint.2022.05.098
  • G. Lakshminarayana; A.N. Meza-Rocha; O. Soriano-Romero; U. Caldiño; A. Lira; Dong-Eun Lee; Jonghun Yoon; Taejoon Park Exploration of concentration-reliant optical and luminescence aspects of Sm3+: B2O3-Gd2O3-Li2O-Na2O-LiF glasses for color displays and visible lasers utilization, Journal of Alloys and Compounds, Volume 926 (2022), p. 166936 | DOI:10.1016/j.jallcom.2022.166936
  • Ihor I. Kindrat; Bohdan V. Padlyak; Radosław Lisiecki; Adam Drzewiecki; Volodymyr T. Adamiv Effect of silver co-doping on luminescence of the Pr3+-doped lithium tetraborate glass, Journal of Luminescence, Volume 241 (2022), p. 118468 | DOI:10.1016/j.jlumin.2021.118468
  • Nguyen Thi Quynh Lien; Nguyen Ngoc Trac; Phan Van Do; Ho Van Tuyen Judd-Ofelt analysis of Eu3+ and adjustable emission in Eu3+/Eu2+ co-doped sodium aluminosilicate glasses, Journal of Physics and Chemistry of Solids, Volume 164 (2022), p. 110637 | DOI:10.1016/j.jpcs.2022.110637
  • Vishab Kesarwani; Vineet Kumar Rai Fluorescence intensity ratio technique and its reliability, Methods and Applications in Fluorescence, Volume 10 (2022) no. 3, p. 034006 | DOI:10.1088/2050-6120/ac70ab
  • Vishab Kesarwani; Vineet Kumar Rai Optical thermometry and broad infrared luminescence in highly sensitized TBO glass, Optics Laser Technology, Volume 146 (2022), p. 107535 | DOI:10.1016/j.optlastec.2021.107535
  • Hosam M. Gomaa; H.A. Saudi; I.S. Yahia; M.A. Ibrahim; H.Y. Zahran Influence of exchanging CeO2 with Cu2O3 on structural matrix, shielding, and linear/nonlinear optical parameters of the cerium-sodium borate glass, Optik, Volume 249 (2022), p. 168267 | DOI:10.1016/j.ijleo.2021.168267
  • Pritha Patra; Kalyandurg Annapurna Transparent tellurite glass-ceramics for photonics applications: A comprehensive review on crystalline phases and crystallization mechanisms, Progress in Materials Science, Volume 125 (2022), p. 100890 | DOI:10.1016/j.pmatsci.2021.100890
  • Xu Feng; Liting Lin; Rui Duan; Jianrong Qiu; Shifeng Zhou Transition metal ion activated near-infrared luminescent materials, Progress in Materials Science, Volume 129 (2022), p. 100973 | DOI:10.1016/j.pmatsci.2022.100973
  • Atsushi Takeo; Shuhei Ichikawa; Shogo Maeda; Dolf Timmerman; Jun Tatebayashi; Yasufumi Fujiwara Droop-free amplified red emission from Eu ions in GaN, Japanese Journal of Applied Physics, Volume 60 (2021) no. 12, p. 120905 | DOI:10.35848/1347-4065/ac3b88
  • Hosam M. Gomaa; H. A. Saudi; I. S. Yahia; M. A. Ibrahim; H. Y. Zahran Impact of graphite impurities on the structure and optical properties of the sodium borate oxide glass, Journal of Materials Science: Materials in Electronics, Volume 32 (2021) no. 23, p. 27553 | DOI:10.1007/s10854-021-07130-8
  • Dalip Singh; Surinder Singh; Tejbir Singh; Preet Kaur Samarium and gadolinium-co-doped lead borate glasses for luminescent applications, Journal of Materials Science: Materials in Electronics, Volume 32 (2021) no. 6, p. 6900 | DOI:10.1007/s10854-021-05396-6
  • A. L. Fanai; S. Rai Effect of TiO2 and Al2O3 on energy transfer and upconversion in Ho–Yb co-doped sol–gel silica glass, Journal of Sol-Gel Science and Technology, Volume 97 (2021) no. 2, p. 452 | DOI:10.1007/s10971-020-05424-7
  • Weiwei Chen; Jiangkun Cao; Mingying Peng; Yafei Wang; Puxian Xiong Enhancement of ultrabroadband Bi NIR emission via fluorination for all wavelength amplification of optical communication, Journal of the American Ceramic Society, Volume 104 (2021) no. 3, p. 1309 | DOI:10.1111/jace.17507
  • Karolina Kowalska; Marta Kuwik; Justyna Polak; Joanna Pisarska; Wojciech A. Pisarski Transition Metals (Cr3+) and Lanthanides (Eu3+) in Inorganic Glasses with Extremely Different Glass-Formers B2O3 and GeO2, Materials, Volume 14 (2021) no. 23, p. 7156 | DOI:10.3390/ma14237156
  • Magdalena Lesniak; Marcin Kochanowicz; Agata Baranowska; Piotr Golonko; Marta Kuwik; Jacek Zmojda; Piotr Miluski; Jan Dorosz; Wojciech Andrzej Pisarski; Joanna Pisarska; Dominik Dorosz Structure and Luminescence Properties of Transparent Germanate Glass-Ceramics Co-Doped with Ni2+/Er3+ for Near-Infrared Optical Fiber Application, Nanomaterials, Volume 11 (2021) no. 8, p. 2115 | DOI:10.3390/nano11082115
  • M. Kumar; Y.C. Ratnakaram Role of TeO2 coordination with the BaF2 and Bi2O3 on structural and emission properties in Nd3+ doped fluoro phosphate glasses for NIR 1.058 μm laser emission, Optical Materials, Volume 112 (2021), p. 110738 | DOI:10.1016/j.optmat.2020.110738
  • Fahimeh Ahmadi; Rosli Hussin; Sib Krishna Ghoshal Physical and structural properties of dysprosium ion doped phosphate glasses, Optik, Volume 227 (2021), p. 166000 | DOI:10.1016/j.ijleo.2020.166000
  • Nur Najahatul Huda Saris; Azura Hamzah; Sumiaty Ambran; Osamu Mikami; Takaaki Ishigure; Toshimi Fukui Optical Amplification in Multiple Cores of Europium Aluminium Composite Incorporated Polymer-Based Optical Waveguide Amplifier by Using Mosquito Method, Advances in Electronics Engineering, Volume 619 (2020), p. 25 | DOI:10.1007/978-981-15-1289-6_3
  • A. S. Abouhaswa; M. S. Al-Buriahi; M. Chalermpon; Y. S. Rammah Influence of ZrO2 on gamma shielding properties of lead borate glasses, Applied Physics A, Volume 126 (2020) no. 1 | DOI:10.1007/s00339-019-3264-7
  • Jibo Yu; Xin Wang; Wenhao Li; Meng Zhang; Jiquan Zhang; Ke Tian; Yanqiu Du; Sile Nic Chormaic; Pengfei Wang An Experimental and Theoretical Investigation of a 2 μm Wavelength Low-Threshold Microsphere Laser, Journal of Lightwave Technology, Volume 38 (2020) no. 7, p. 1880 | DOI:10.1109/jlt.2019.2958349
  • Rajinder Kaur; Atul Khanna Photoluminescence and thermal properties of trivalent ion-doped lanthanum tellurite anti-glass and glass composite samples, Journal of Luminescence, Volume 225 (2020), p. 117375 | DOI:10.1016/j.jlumin.2020.117375
  • Roghayeh Imani; Guillermo Huerta Cuellar Introductory Chapter: Optical Fibers, Optical Fiber Applications (2020) | DOI:10.5772/intechopen.91397
  • C. Parthasaradhi Reddy; R. Ramaraghavulu; T. Kalpana; J. Gajendiran Energy transfer induced enhancement in NIR luminescence characteristics of Yb3+/Er3+ co-doped sodium zinc bismuth fluorophosphate glasses, Optical Materials, Volume 100 (2020), p. 109616 | DOI:10.1016/j.optmat.2019.109616
  • Ho Van Tuyen; Masayuki Nogami; Le Xuan Hung Reduction of Sm3+ and Eu3+ ions-co-doped Al2O3–SiO2 glasses and photoluminescence properties, Optical Materials, Volume 100 (2020), p. 109639 | DOI:10.1016/j.optmat.2019.109639
  • J. Yu; J. Zhang; R. Wang; A. Li; M. Zhang; S. Wang; P. Wang; J. M. Ward; S. Nic Chormaic A tellurite glass optical microbubble resonator, Optics Express, Volume 28 (2020) no. 22, p. 32858 | DOI:10.1364/oe.406256
  • Sulaiman Wadi Harun; Nurfarhanah Zulkipli; Ahmad Razif Muhammad; Anas Abdul Latiff Q‐Switching Pulses Generation Using Topology Insulators as Saturable Absorber, Advanced Topological Insulators (2019), p. 207 | DOI:10.1002/9781119407317.ch6
  • Guangning Hou; Chaomin Zhang; Wenbin Fu; Guishun Li; Jinan Xia; Yunxia Ping Improvement of mechanical strength in Y3+/La3+ co-doped silicate glasses for display screen, Ceramics International, Volume 45 (2019) no. 9, p. 11850 | DOI:10.1016/j.ceramint.2019.03.066
  • Yingying Ning; Mengliang Zhu; Jun-Long Zhang Near-infrared (NIR) lanthanide molecular probes for bioimaging and biosensing, Coordination Chemistry Reviews, Volume 399 (2019), p. 213028 | DOI:10.1016/j.ccr.2019.213028
  • Alexander Veber; Zhuorui Lu; Manuel Vermillac; Franck Pigeonneau; Wilfried Blanc; Laeticia Petit Nano-Structured Optical Fibers Made of Glass-Ceramics, and Phase Separated and Metallic Particle-Containing Glasses, Fibers, Volume 7 (2019) no. 12, p. 105 | DOI:10.3390/fib7120105
  • K. Soler-Carracedo; A. Ruiz; I.R. Martín; F. Lahoz Luminescence whispering gallery modes in Ho3+ doped microresonator glasses for temperature sensing, Journal of Alloys and Compounds, Volume 777 (2019), p. 198 | DOI:10.1016/j.jallcom.2018.10.297
  • E. Kolobkova; A. Alkhlef; B.M. Dinh; A.S. Yasukevich; O.P. Dernovich; N.V. Kuleshov; N. Nikonorov Spectral properties of Nd3+ ions in the new fluoride glasses with small additives of the phosphates, Journal of Luminescence, Volume 206 (2019), p. 523 | DOI:10.1016/j.jlumin.2018.10.082
  • I.I. Kindrat; B.V. Padlyak; B. Kukliński; A. Drzewiecki; V.T. Adamiv Effect of silver co-doping on enhancement of the Sm3+ luminescence in lithium tetraborate glass, Journal of Luminescence, Volume 213 (2019), p. 290 | DOI:10.1016/j.jlumin.2019.05.045
  • Masayuki Nogami; Xuan Hung Le; Xuan Quang Vu Novel silicate glasses in the acceleration of hydrogen diffusion for reducing dopant metal ions, Journal of Non-Crystalline Solids, Volume 503-504 (2019), p. 260 | DOI:10.1016/j.jnoncrysol.2018.10.003
  • Nupur Gupta; Hirdesh; Rajinder Kaur; Atul Khanna; Satbir Singh; Bipin Kumar Gupta Spatially resolved X-ray fluorescence, Raman and photoluminescence spectroscopy of Eu3+/Er3+ doped tellurite glasses and anti-glasses, Journal of Non-Crystalline Solids, Volume 513 (2019), p. 24 | DOI:10.1016/j.jnoncrysol.2019.01.039
  • Yasemin Pepe; Murat Erdem; Alphan Sennaroglu; Gonul Eryurek Enhanced gain bandwidth of Tm3+ and Er3+ doped tellurite glasses for broadband optical amplifier, Journal of Non-Crystalline Solids, Volume 522 (2019), p. 119501 | DOI:10.1016/j.jnoncrysol.2019.119501
  • E. Kolobkova; A. Yasukevich; N. Kuleshov; N. Nikonorov; A. Babkina Concentration dependence of spectroscopic properties and energy transfer analysis of the fluorophosphate glasses with small phosphates content doped with Nd3+ ions, Journal of Non-Crystalline Solids, Volume 526 (2019), p. 119703 | DOI:10.1016/j.jnoncrysol.2019.119703
  • P. Mpourazanis; G. Stogiannidis; S. Tsigoias; G.N. Papatheodorou; A.G. Kalampounias Ionic to covalent glass network transition: Effects on elastic and vibrational properties according to ultrasonic echography and Raman spectroscopy, Journal of Physics and Chemistry of Solids, Volume 125 (2019), p. 43 | DOI:10.1016/j.jpcs.2018.10.010
  • Łukasz Marek; Marcin Sobczyk; Władysław Wrzeszcz Relaxation dynamics of excited states of Tm3+ ions in TeO2-ZnO-Na2O-Y2O3 glasses, Journal of Rare Earths, Volume 37 (2019) no. 11, p. 1188 | DOI:10.1016/j.jre.2019.03.011
  • Jacek Zmojda; Marcin Kochanowicz; Piotr Miluski; Dominik Dorosz The Luminescent Properties of Photonic Glasses and Optical Fibers, Molecular Spectroscopy—Experiment and Theory, Volume 26 (2019), p. 427 | DOI:10.1007/978-3-030-01355-4_14
  • N. K. Thipparapu; Y. Wang; S. Wang; A. A. Umnikov; P. Barua; J. K. Sahu Bi-doped fiber amplifiers and lasers [Invited], Optical Materials Express, Volume 9 (2019) no. 6, p. 2446 | DOI:10.1364/ome.9.002446
  • Shengzhi Sun; Bin Qian; Zhenyan Wang; Xiaofeng Liu; Jianrong Qiu Near-infrared emitting colloidal solution of nanocrystals for multi-band optical amplification, Optical Materials Express, Volume 9 (2019) no. 6, p. 2523 | DOI:10.1364/ome.9.002523
  • E. Kolobkova; A. Alkhlef; A. Yasukevich; A. Babkina Spectroscopic and lasing properties of Er3+/Yb3+-doped fluorophosphate glass with small additives of phosphates, Optical Materials Express, Volume 9 (2019) no. 9, p. 3666 | DOI:10.1364/ome.9.003666
  • S.O. Kasap; K. Koughia; Jai Singh; Harry E. Ruda; Asim K. Ray Fundamental Optical Properties of MaterialsII, Optical Properties of Materials and Their Applications (2019), p. 37 | DOI:10.1002/9781119506003.ch2
  • K. Lemański; M. Babij; P.J. Dereń Upconversion emission of the GaN nanocrystals doped with rare earth ions, Solid State Sciences, Volume 94 (2019), p. 127 | DOI:10.1016/j.solidstatesciences.2019.06.005
  • Juejun Hu; Lan Yang Glass in Integrated Photonics, Springer Handbook of Glass (2019), p. 1441 | DOI:10.1007/978-3-319-93728-1_42
  • Wenyan Zheng; Xiyang Wang; Wei Mao; Xvsheng Qiao; Shiqing Xu; Junjie Zhang The Nitrogen-Hole-Center Electron Transfer Imparts Reduction Ability to Eu Ion in AlN-Containing Phosphate Glasses, The Journal of Physical Chemistry C, Volume 123 (2019) no. 45, p. 27794 | DOI:10.1021/acs.jpcc.9b08747
  • Ayoub Ladaci; Sylvain Girard; Luciano Mescia; Thierry Robin; Benoit Cadier; Arnaud Laurent; Mathieu Boutillier; Baidy Sane; Emmanuel Marin; Youcef Ouerdane; Aziz Boukenter Validity of the McCumber Theory at High Temperatures in Erbium and Ytterbium-Doped Aluminosilicate Fibers, IEEE Journal of Quantum Electronics, Volume 54 (2018) no. 4, p. 1 | DOI:10.1109/jqe.2018.2836674
  • Elisabeth Kreidt; Christian Kruck; Michael Seitz Nonradiative Deactivation of Lanthanoid Luminescence by Multiphonon Relaxation in Molecular Complexes, Including Actinides, Volume 53 (2018), p. 35 | DOI:10.1016/bs.hpcre.2018.04.001
  • M.S.A. Mohd Saidi; S.K. Ghoshal; R. Arifin; M.K. Roslan; R. Muhammad; W.N.W. Shamsuri; M. Abdullah; M.S. Shaharin Spectroscopic properties of Dy3+ doped tellurite glass with Ag/TiO2 nanoparticles inclusion: Judd−Ofelt analysis, Journal of Alloys and Compounds, Volume 754 (2018), p. 171 | DOI:10.1016/j.jallcom.2018.04.280
  • K. Linganna; G.L. Agawane; Jung-Hwan In; June Park; Ju H. Choi Spectroscopic properties of Er3+/Yb3+ co-doped fluorophosphate glasses for NIR luminescence and optical temperature sensor applications, Journal of Industrial and Engineering Chemistry, Volume 67 (2018), p. 236 | DOI:10.1016/j.jiec.2018.06.034
  • I.I. Kindrat; B.V. Padlyak; B. Kukliński; A. Drzewiecki; V.T. Adamiv Enhancement of the Eu3+ luminescence in Li2B4O7 glasses co-doped with Eu and Ag, Journal of Luminescence, Volume 204 (2018), p. 122 | DOI:10.1016/j.jlumin.2018.07.051
  • Xiaoman Li; Mingying Peng; Jiangkun Cao; Zhongmin Yang; Shanhui Xu Distribution and stabilization of bismuth NIR centers in Bi-doped aluminosilicate laser glasses by managing glass network structure, Journal of Materials Chemistry C, Volume 6 (2018) no. 29, p. 7814 | DOI:10.1039/c8tc01042k
  • V.K. Goncharuk; V.Ya. Kavun; A.B. Slobodyuk; V.E. Silant'ev; A.Yu. Mamaev; A.G. Mirochnik; I.G. Maslennikova Crystallization and luminescence properties of Eu 3+ -doped ZrF 4 –BaF 2 –NaPO 3 glass and glass ceramics, Journal of Non-Crystalline Solids, Volume 480 (2018), p. 61 | DOI:10.1016/j.jnoncrysol.2017.10.018
  • M.S.A. Mohd Saidi; S.K. Ghoshal; K. Hamzah; R. Arifin; M.F. Omar; M.K. Roslan; E.S. Sazali Visible light emission from Dy3+ doped tellurite glass: Role of silver and titania nanoparticles co-embedment, Journal of Non-Crystalline Solids, Volume 502 (2018), p. 198 | DOI:10.1016/j.jnoncrysol.2018.09.012
  • Xiaoman Li; Jiangkun Cao; Liping Wang; Mingying Peng Predictable tendency of Bi NIR emission in Bi‐doped magnesium aluminosilicate laser glasses, Journal of the American Ceramic Society, Volume 101 (2018) no. 3, p. 1159 | DOI:10.1111/jace.15277
  • Xiaoman Li; Jiangkun Cao; Mingying Peng The origin of the heterogeneous distribution of bismuth in aluminosilicate laser glasses, Journal of the American Ceramic Society, Volume 101 (2018) no. 7, p. 2921 | DOI:10.1111/jace.15469
  • Gandham Lakshminarayana; I.V. Kityk; M.A. Mahdi; K.J. Plucinski Er/Pr-codoped borotellurite glasses as efficient laser operated nonlinear optical materials, Materials Letters, Volume 214 (2018), p. 23 | DOI:10.1016/j.matlet.2017.11.100
  • I.I. Kindrat; B.V. Padlyak Luminescence properties and quantum efficiency of the Eu-doped borate glasses, Optical Materials, Volume 77 (2018), p. 93 | DOI:10.1016/j.optmat.2018.01.019
  • Masayuki Nogami; Vu Xuan Quang; Shinobu Ohki; Kenzo Deguchi; Tadashi Shimizu Reduction Mechanisms of Cu2+-Doped Na2O–Al2O3–SiO2 Glasses during Heating in H2 Gas, The Journal of Physical Chemistry B, Volume 122 (2018) no. 3, p. 1315 | DOI:10.1021/acs.jpcb.7b10913
  • Savidh Khan; G. Kaur; K. Singh Effect of ZrO2 on dielectric, optical and structural properties of yttrium calcium borosilicate glasses, Ceramics International, Volume 43 (2017) no. 1, p. 722 | DOI:10.1016/j.ceramint.2016.09.219
  • Lini Li; Ge Li; Tengyu Zhang; Changgui Lin; Guoxiang Wang; Shixun Dai; Qiuhua Nie; Qing Jiao Preparation and properties of Ge–Ga–La–S–AgI chalcogenide glass, Ceramics International, Volume 43 (2017) no. 5, p. 4508 | DOI:10.1016/j.ceramint.2016.12.102
  • L. Shamshad; G. Rooh; K. Kirdsiri; N. Srisittipokakun; B. Damdee; H.J. Kim; J. Kaewkhao Photoluminescence and white light generation behavior of lithium gadolinium silicoborate glasses, Journal of Alloys and Compounds, Volume 695 (2017), p. 2347 | DOI:10.1016/j.jallcom.2016.11.105
  • Melis Gökçe; Ufuk Şentürk; Deniz K. Uslu; Gözde Burgaz; Yüksel Şahin; Aytaç Gürhan Gökçe Investigation of europium concentration dependence on the luminescent properties of borogermanate glasses, Journal of Luminescence, Volume 192 (2017), p. 263 | DOI:10.1016/j.jlumin.2017.06.041
  • W. C. Wang; Q. H. Le; Q. Y. Zhang; L. Wondraczek Fluoride-sulfophosphate glasses as hosts for broadband optical amplification through transition metal activators, Journal of Materials Chemistry C, Volume 5 (2017) no. 31, p. 7969 | DOI:10.1039/c7tc01853c
  • G. Lakshminarayana; S. O. Baki; A. Lira; M. I. Sayyed; I. V. Kityk; M. K. Halimah; M. A. Mahdi X-ray photoelectron spectroscopy (XPS) and radiation shielding parameters investigations for zinc molybdenum borotellurite glasses containing different network modifiers, Journal of Materials Science, Volume 52 (2017) no. 12, p. 7394 | DOI:10.1007/s10853-017-0974-0
  • Quyen Huyen Le; Theresia Palenta; Omar Benzine; Kristin Griebenow; Rene Limbach; Efstratios I. Kamitsos; Lothar Wondraczek Formation, structure and properties of fluoro-sulfo-phosphate poly-anionic glasses, Journal of Non-Crystalline Solids, Volume 477 (2017), p. 58 | DOI:10.1016/j.jnoncrysol.2017.09.043
  • Masayuki Nogami; Vu Xuan Quang; Takamasa Nonaka; Tadashi Shimizu; Shinobu Ohki; Kenzo Deguchi Diffusion and reaction of H 2 gas for reducing Eu 3+ ions in glasses, Journal of Physics and Chemistry of Solids, Volume 105 (2017), p. 54 | DOI:10.1016/j.jpcs.2017.02.007
  • Kaushal Jha; Mula Jayasimhadri Structural and emission properties of Eu3+‐doped alkaline earth zinc‐phosphate glasses for white LED applications, Journal of the American Ceramic Society, Volume 100 (2017) no. 4, p. 1402 | DOI:10.1111/jace.14668
  • Xili Liao; Xiaobo Jiang; Qiuhong Yang; Longfei Wang; Danping Chen Spectral Properties of Er3+/Tm3+ Co-Doped ZBLAN Glasses and Fibers, Materials, Volume 10 (2017) no. 5, p. 486 | DOI:10.3390/ma10050486
  • Kawa M. Kaky; G. Lakshminarayana; S.O. Baki; A. Lira; U. Caldiño; A.N. Meza-Rocha; C. Falcony; I.V. Kityk; Y.H. Taufiq-Yap; M.K. Halimah; M.A. Mahdi Structural and optical studies of Er 3+ -doped alkali/alkaline oxide containing zinc boro-aluminosilicate glasses for 1.5 μm optical amplifier applications, Optical Materials, Volume 69 (2017), p. 401 | DOI:10.1016/j.optmat.2017.04.006
  • S. B. Meshkova; P. G. Doga; A. A. Kucher IR Luminescence of Nd3+, Sm3+, and Yb3+ β-Diketonates in Different Aggregate States, Russian Journal of Coordination Chemistry, Volume 43 (2017) no. 10, p. 657 | DOI:10.1134/s1070328417090056
  • Kummara Venkata Krishnaiah; Jose Marques-Hueso; Raman Kashyap Broadband Emission in Tellurite Glasses, Technological Advances in Tellurite Glasses, Volume 254 (2017), p. 155 | DOI:10.1007/978-3-319-53038-3_8
  • J. Zmojda; M. Kochanowicz; P. Miluski; A. Lukowiak; W. A. Pisarski; J. Pisarska; M. Marciniak; M. Ferrari; G. Righini; M. Sitarz; D. Dorosz, 2016 18th International Conference on Transparent Optical Networks (ICTON) (2016), p. 1 | DOI:10.1109/icton.2016.7550679
  • Z. T. Chen; E. H. Song; M. Wu; B. Zhou; Q. Y. Zhang Exchange coupled Mn-Mn pair: An approach for super-broadband 1380 nm emission in α-MnS, Applied Physics Letters, Volume 109 (2016) no. 19 | DOI:10.1063/1.4967452
  • G. Lakshminarayana; Kawa M. Kaky; S.O. Baki; Song Ye; A. Lira; I.V. Kityk; M.A. Mahdi Concentration dependent structural, thermal, and optical features of Pr 3+ -doped multicomponent tellurite glasses, Journal of Alloys and Compounds, Volume 686 (2016), p. 769 | DOI:10.1016/j.jallcom.2016.06.069
  • Damian Szymański; Marcin Sobczyk Optical and structural investigation of dysprosium doped-Y2Te4O11, Journal of Luminescence, Volume 173 (2016), p. 11 | DOI:10.1016/j.jlumin.2015.12.023
  • Lidia Żur; Joanna Janek; Marta Sołtys; Tomasz Goryczka; Joanna Pisarska; Wojciech A. Pisarski Structural and optical investigations of rare earth doped lead-free germanate glasses modified by MO and MF2 (M = Ca, Sr, Ba), Journal of Non-Crystalline Solids, Volume 431 (2016), p. 145 | DOI:10.1016/j.jnoncrysol.2015.03.008
  • Wilfried Blanc; Bernard Dussardier Formation and applications of nanoparticles in silica optical fibers, Journal of Optics, Volume 45 (2016) no. 3, p. 247 | DOI:10.1007/s12596-015-0281-6
  • Masayuki Nogami; Akihiko Koiwai; Takamasa Nonaka; J. Ballato Control of Oxidation State of Eu Ions in Na2O–Al2O3–SiO2 Glasses, Journal of the American Ceramic Society, Volume 99 (2016) no. 4, p. 1248 | DOI:10.1111/jace.14111
  • F. Zaman; J. Kaewkhao; N. Srisittipokakun; N. Wantana; H.J. Kim; G. Rooh Investigation of luminescence and laser transition of Dy3+ in Li2O-Gd2O3-Bi2O3-B2O3 glasses, Optical Materials, Volume 55 (2016), p. 136 | DOI:10.1016/j.optmat.2016.03.024
  • Olfa Maalej; Julien Merigeon; Brigitte Boulard; Mihaela Girtan Visible to near-infrared down-shifting in Tm 3+ doped fluoride glasses for solar cells efficiency enhancement, Optical Materials, Volume 60 (2016), p. 235 | DOI:10.1016/j.optmat.2016.07.047
  • Lidia Zur; Joanna Janek; Ewa Pietrasik; Marta Sołtys; Joanna Pisarska; Wojciech A. Pisarski Influence of MO/MF2 modifiers (M = Ca, Sr, Ba) on spectroscopic properties of Eu3+ ions in germanate and borate glasses, Optical Materials, Volume 61 (2016), p. 59 | DOI:10.1016/j.optmat.2016.05.045
  • Darayas N. Patel; Sergey S. Sarkisov; Abdalla M. Darwish; John Ballato Optical gain in capillary light guides filled with NaYF_4: Yb^3+, Er^3+ nanocolloids, Optics Express, Volume 24 (2016) no. 18, p. 21147 | DOI:10.1364/oe.24.021147
  • S. B. Meshkova; P. G. Doga; A. V. Kiriyak; A. A. Kucher Changes in Luminescence Characteristics of Terbium Hydroxybenzoates in Condensed Matter, Russian Journal of Inorganic Chemistry, Volume 61 (2016) no. 1, p. 73 | DOI:10.1134/s0036023616010162
  • S. B. Meshkova; P. G. Doga; V. P. Antonovich Effect of Condensed Media on the Luminescent Characteristics of Lanthanide Complexes, Russian Journal of Physical Chemistry A, Volume 90 (2016) no. 4, p. 870 | DOI:10.1134/s003602441604021x
  • Setsuhisa Tanabe Glass and Rare‐Earth Elements: A Personal Perspective, International Journal of Applied Glass Science, Volume 6 (2015) no. 4, p. 305 | DOI:10.1111/ijag.12142
  • A. de Pablos-Martín; J. Méndez-Ramos; J. del-Castillo; A. Durán; V.D. Rodríguez; M.J. Pascual Crystallization and up-conversion luminescence properties of Er3+/Yb3+-doped NaYF4-based nano-glass-ceramics, Journal of the European Ceramic Society, Volume 35 (2015) no. 6, p. 1831 | DOI:10.1016/j.jeurceramsoc.2014.12.034
  • S. B. Meshkova; A. V. Kiriyak; P. G. Doga; V. P. Dotsenko; A. A. Kucher The influence of viscous media on the nonradiative deactivation of the luminescence of Eu3+ and Tb3+ complexes with acyl-2-aminobenzoic acids, Optics and Spectroscopy, Volume 119 (2015) no. 1, p. 70 | DOI:10.1134/s0030400x15070176
  • D. Dorosz; J. Zmojda; M. Kochanowicz; P. Miluski; P. Jelen; M. Sitarz Structural and optical study on antimony-silicate glasses doped with thulium ions, Spectrochimica Acta Part A: Molecular and Biomolecular Spectroscopy, Volume 134 (2015), p. 608 | DOI:10.1016/j.saa.2014.06.070
  • Masayuki Nogami Reduction Mechanism for Eu Ions in Al2O3-Containing Glasses by Heat Treatment in H2 Gas, The Journal of Physical Chemistry B, Volume 119 (2015) no. 4, p. 1778 | DOI:10.1021/jp511513n
  • J. Pisarska; M. Sołtys; L. Żur; W. A. Pisarski; C. K. Jayasankar Excitation and luminescence of rare earth-doped lead phosphate glasses, Applied Physics B, Volume 116 (2014) no. 4, p. 837 | DOI:10.1007/s00340-014-5770-9
  • Yixi Zhuang; Jumpei Ueda; Setsuhisa Tanabe Multi-color persistent luminescence in transparent glass ceramics containing spinel nano-crystals with Mn2+ ions, Applied Physics Letters, Volume 105 (2014) no. 19 | DOI:10.1063/1.4901749
  • Hao Tang; Batric Pesic Electrochemistry of ErCl3 and morphology of erbium electrodeposits produced on Mo substrate in early stages of electrocrystallization from LiCl–KCl molten salts, Electrochimica Acta, Volume 133 (2014), p. 224 | DOI:10.1016/j.electacta.2014.04.014
  • M. Kochanowicz; D. Dorosz; J. Zmojda; J. Dorosz; P. Miluski Influence of temperature on upconversion luminescence in tellurite glass co-doped with Yb3+/Er3+ and Yb3+/Tm3+, Journal of Luminescence, Volume 151 (2014), p. 155 | DOI:10.1016/j.jlumin.2014.02.012
  • Yixi Zhuang; Setsuhisa Tanabe; Jianrong Qiu; J. Ballato Wavelength Tailorability of Broadband Near‐Infrared Luminescence in Cr4+‐Activated Transparent Glass‐Ceramics, Journal of the American Ceramic Society, Volume 97 (2014) no. 11, p. 3519 | DOI:10.1111/jace.13128
  • Zhi-Yuan Yan; Bing Yan Novel organic–inorganic hybrid soft xerogels with lanthanide complexes through an ionic liquid linkage, New J. Chem., Volume 38 (2014) no. 6, p. 2604 | DOI:10.1039/c3nj01639k
  • Dominik Dorosz; Jacek Zmojda; Marcin Kochanowicz Broadband near infrared emission in antimony-germanate glass co-doped with erbium and thulium ions, Optical Engineering, Volume 53 (2014) no. 7, p. 071807 | DOI:10.1117/1.oe.53.7.071807
  • M. Yamada; H. Ono; K. Ohta; S. Aozasa; T. Tanaka; K. Senda; Y. Maeda; O. Koyama; J. Ono, Optical Fiber Communication Conference (2014), p. Tu2D.3 | DOI:10.1364/ofc.2014.tu2d.3
  • V.A.G. Rivera; Y. Ledemi; M. El-Amraoui; Y. Messaddeq; E. Marega Control of the radiative properties via photon-plasmon interaction in Er^3+-Tm^3+-codoped tellurite glasses in the near infrared region, Optics Express, Volume 22 (2014) no. 17, p. 21122 | DOI:10.1364/oe.22.021122
  • Fakhra Nawaz; Md. Rahim Sahar; S.K. Ghoshal; Asmahani Awang; Ishaq Ahmed Concentration dependent structural and spectroscopic properties of Sm3+/Yb3+ co-doped sodium tellurite glass, Physica B: Condensed Matter, Volume 433 (2014), p. 89 | DOI:10.1016/j.physb.2013.09.021
  • S. Selvi; G. Venkataiah; S. Arunkumar; G. Muralidharan; K. Marimuthu Structural and luminescence studies on Dy3+ doped lead boro–telluro-phosphate glasses, Physica B: Condensed Matter, Volume 454 (2014), p. 72 | DOI:10.1016/j.physb.2014.07.018
  • Huan Zhan; Aidong Zhang; Jianli He; Zhiguang Zhou; Jinhai Si; Aoxiang Lin 123  μm emission of Er/Pr-doped water-free fluorotellurite glasses, Applied Optics, Volume 52 (2013) no. 28, p. 7002 | DOI:10.1364/ao.52.007002
  • Fabrice Pointillart; Boris Le Guennic; Thomas Cauchy; Stéphane Golhen; Olivier Cador; Olivier Maury; Lahcène Ouahab A Series of Tetrathiafulvalene-Based Lanthanide Complexes Displaying Either Single Molecule Magnet or Luminescence—Direct Magnetic and Photo-Physical Correlations in the Ytterbium Analogue, Inorganic Chemistry, Volume 52 (2013) no. 10, p. 5978 | DOI:10.1021/ic400253m
  • Fabrice Pointillart; Boris Le Guennic; Olivier Maury; Stéphane Golhen; Olivier Cador; Lahcène Ouahab Lanthanide Dinuclear Complexes Involving Tetrathiafulvalene-3-pyridine-N-oxide Ligand: Semiconductor Radical Salt, Magnetic, and Photophysical Studies, Inorganic Chemistry, Volume 52 (2013) no. 3, p. 1398 | DOI:10.1021/ic302095h
  • D. Dorosz; J. Zmojda; M. Kochanowicz Investigation on broadband near-infrared emission in Yb3+/Ho3+ co-doped antimony–silicate glass and optical fiber, Optical Materials, Volume 35 (2013) no. 12, p. 2577 | DOI:10.1016/j.optmat.2013.07.022
  • Felipe Thomaz Aquino; Jefferson Luis Ferrari; Sidney José Lima Ribeiro; Alban Ferrier; Philippe Goldner; Rogéria Rocha Gonçalves Broadband NIR emission in novel sol–gel Er3+-doped SiO2–Nb2O5 glass ceramic planar waveguides for photonic applications, Optical Materials, Volume 35 (2013) no. 3, p. 387 | DOI:10.1016/j.optmat.2012.09.029
  • Bryan van Saders; Lara Al-Baroudi; Mei Chee Tan; Richard E. Riman Rare-earth doped particles with tunable infrared emissions for biomedical imaging, Optical Materials Express, Volume 3 (2013) no. 5, p. 566 | DOI:10.1364/ome.3.000566
  • Xiang Peng; Kyungbum Kim; Michael Mielke; Stephen Jennings; Gordon Masor; Dave Stohl; Arturo Chavez-Pirson; Dan T. Nguyen; Dan Rhonehouse; Jie Zong; Dmitriy Churin; N. Peyghambarian High efficiency, monolithic fiber chirped pulse amplification system for high energy femtosecond pulse generation, Optics Express, Volume 21 (2013) no. 21, p. 25440 | DOI:10.1364/oe.21.025440
  • Rongfei Wang; Zhengwen Yang; Dacheng Zhou; Zhiguo Song; Zhaoyi Yin; Yuanyuan Xu; Kan Zhu; Chen Li; Jianbei Qiu, 2012 Symposium on Photonics and Optoelectronics (2012), p. 1 | DOI:10.1109/sopo.2012.6270532
  • D. Dorosz; J. Żmojda; M. Kochanowicz; P. Miluski; J. Dorosz Tm3+/Ho3+-Doped ASE Fibre Source for Mid-Infrared Sensor Applications, Acta Physica Polonica A, Volume 122 (2012) no. 5, p. 927 | DOI:10.12693/aphyspola.122.927
  • Tao Zheng; Jie-Ming Qin; Da-Yong Jiang; Jing-Wen Lü; Sheng-Chun Xiao Spectroscopic properties in Er3+/Yb3+Co-doped fluorophosphate glass, Chinese Physics B, Volume 21 (2012) no. 4, p. 043302 | DOI:10.1088/1674-1056/21/4/043302
  • G.P. Kothiyal; Arvind Ananthanarayanan; G.K. Dey Glass and Glass-Ceramics, Functional Materials (2012), p. 323 | DOI:10.1016/b978-0-12-385142-0.00009-x
  • Scott R. Daly; Do Young Kim; Gregory S. Girolami Lanthanide N,N-Dimethylaminodiboranates as a New Class of Highly Volatile Chemical Vapor Deposition Precursors, Inorganic Chemistry, Volume 51 (2012) no. 13, p. 7050 | DOI:10.1021/ic201852j
  • S. B. Meshkova; A. V. Kiriyak; A. N. Gusev; G. A. Nischimenko; V. F. Shul’gin IR luminescence of mixed-ligand complexes of Nd(III), Yb(III), and Er(III) with dibenzoylmethane and 1,2,4-triazoles, Journal of Applied Spectroscopy, Volume 79 (2012) no. 5, p. 708 | DOI:10.1007/s10812-012-9660-9
  • K. Selvaraju; K. Marimuthu Structural and spectroscopic studies on concentration dependent Er3+ doped boro-tellurite glasses, Journal of Luminescence, Volume 132 (2012) no. 5, p. 1171 | DOI:10.1016/j.jlumin.2011.12.056
  • Bo Zhou; Lili Tao; Yuen H. Tsang; Wei Jin; Edwin Yue-Bun Pun Superbroadband near-IR photoluminescence from Pr^3+-doped fluorotellurite glasses, Optics Express, Volume 20 (2012) no. 4, p. 3803 | DOI:10.1364/oe.20.003803
  • V. F. Shul’gin; S. V. Abkhairova; O. V. Konnik; S. B. Meshkova; Z. M. Topilova; M. A. Kiskin; I. L. Eremenko Synthesis, structure, and luminescent properties of lanthanide coordination compounds with 3-methyl-4-formyl-1-phenylpyrazol-5-one, Russian Journal of Inorganic Chemistry, Volume 57 (2012) no. 3, p. 420 | DOI:10.1134/s0036023612030291
  • M. Frumar; B. Frumarova; T. Wagner Amorphous and Glassy Semiconducting Chalcogenides, Comprehensive Semiconductor Science and Technology (2011), p. 206 | DOI:10.1016/b978-0-44-453153-7.00122-x
  • H. Maestre; Torregrosa; Fernandez-Pousa; Pereda; J. Capmany Widely Tuneable Dual-Wavelength Operation of a Highly-Doped Erbium Fiber Laser Based on Diffraction Gratings, IEEE Journal of Quantum Electronics (2011) | DOI:10.1109/jqe.2011.2162612
  • Huihua Xiong; Gao Tang; Lan Luo; Wei Chen Tm3+-Doped Chalcohalide Glass for Optical Amplifiers at 1.22 and 1.47 µm, Japanese Journal of Applied Physics, Volume 50 (2011) no. 10R, p. 102602 | DOI:10.7567/jjap.50.102602
  • Dacheng Zhou; Rongfei Wang; Zhengwen Yang; Zhiguo Song; Zhaoyi Yin; Jianbei Qiu Spectroscopic properties of Tm3+ doped TeO2-R2O-La2O3 glasses for 1.47μm optical amplifiers, Journal of Non-Crystalline Solids, Volume 357 (2011) no. 11-13, p. 2409 | DOI:10.1016/j.jnoncrysol.2010.12.027
  • Bo Zhou; Hai Lin; Baojie Chen; Edwin Yue-Bun Pun Superbroadband near-infrared emission in Tm-Bi codoped sodium-germanium-gallate glasses, Optics Express, Volume 19 (2011) no. 7, p. 6514 | DOI:10.1364/oe.19.006514
  • S. B. Meshkova; Z. M. Topilova; N. N. Devyatykh; A. N. Gusev; V. F. Shul’gin IR luminescence of neodymium(III) and ytterbium(III) ions in complexes with N-alkyl-substituted 2-aminobenzoic acids, Russian Journal of Inorganic Chemistry, Volume 56 (2011) no. 2, p. 262 | DOI:10.1134/s0036023611020203
  • Huiyan Fan; Guojun Gao; Guonian Wang; Junjiang Hu; Lili Hu Tm3+ doped Bi2O3–GeO2–Na2O glasses for 1.8μm fluorescence, Optical Materials, Volume 32 (2010) no. 5, p. 627 | DOI:10.1016/j.optmat.2009.12.012
  • J.L. Ferrari; K.O. Lima; L.J.Q. Maia; R.R. Gonçalves Sol-gel preparation of near-infrared broadband emitting Er3+-doped SiO2-Ta2O5 nanocomposite films, Thin Solid Films, Volume 519 (2010) no. 4, p. 1319 | DOI:10.1016/j.tsf.2010.09.035
  • S.W. Yung; H.J. Lin; Y.Y. Lin; R.K. Brow; Y.S. Lai; J.S. Horng; T. Zhang Concentration effect of Yb3+ on the thermal and optical properties of Er3+/Yb3+-codoped ZnF2–Al2O3–P2O5 glasses, Materials Chemistry and Physics, Volume 117 (2009) no. 1, p. 29 | DOI:10.1016/j.matchemphys.2008.11.060
  • Ch. Basavapoornima; C.K. Jayasankar; P.P. Chandrachoodan Luminescence and laser transition studies of Dy3+:K–Mg–Al fluorophosphate glasses, Physica B: Condensed Matter, Volume 404 (2009) no. 2, p. 235 | DOI:10.1016/j.physb.2008.10.045
  • Y.Y. Zhang; B.J. Chen; E.Y.B. Pun; H. Lin Optical radiative parameters and emission anticipation of in two kinds of bismuth-containing oxide glasses with lower phonon energies, Physica B: Condensed Matter, Volume 404 (2009) no. 8-11, p. 1132 | DOI:10.1016/j.physb.2008.11.075
  • Xingqiang Lü; Weiyu Bi; Wenli Chai; Jirong Song; Jianxin Meng; Wai-Yeung Wong; Wai-Kwok Wong; Xiaoping Yang; Richard A. Jones Multinuclear NIR luminescent 1,4-BDC bridged Schiff-base complexes of Nd(III), Polyhedron, Volume 28 (2009) no. 1, p. 27 | DOI:10.1016/j.poly.2008.09.014
  • Keiji Tanaka; Koichi Shimakawa Chalcogenide glasses in Japan: A review on photoinduced phenomena, physica status solidi (b), Volume 246 (2009) no. 8, p. 1744 | DOI:10.1002/pssb.200982002
  • Gao Tang; Jiqian Zhu; Yumei Zhu; Chaoyin Bai The study on properties of Eu3+-doped fluorogallate glasses, Journal of Alloys and Compounds, Volume 453 (2008) no. 1-2, p. 487 | DOI:10.1016/j.jallcom.2006.11.170
  • H. Lin; X.Y. Wang; C.M. Li; H.X. Yang; E.Y.B. Pun; S. Tanabe Near-infrared emissions and quantum efficiencies in Tm3+-doped heavy metal gallate glasses for S- and U-band amplifiers and 1.8μm infrared laser, Journal of Luminescence, Volume 128 (2008) no. 1, p. 74 | DOI:10.1016/j.jlumin.2007.05.015
  • R. Paschotta Erbium-doped Laser Gain Media - an encyclopedia article, RP Photonics Encyclopedia (2008) | DOI:10.61835/6ml
  • Steve Comby; Jean-Claude G. Bünzli Chapter 235 Lanthanide Near-Infrared Luminescence in Molecular Probes and Devices, Volume 37 (2007), p. 217 | DOI:10.1016/s0168-1273(07)37035-9
  • Tanya K. Ronson; Harry Adams; Lindsay P. Harding; Simon J. A. Pope; Daniel Sykes; Stephen Faulkner; Michael D. Ward Polynuclear lanthanide complexes of a series of bridging ligands containing two tridentate N,N′,O-donor units: structures and luminescence properties, Dalton Trans. (2007) no. 10, p. 1006 | DOI:10.1039/b618258e
  • Theodore Lazarides; Mohammed A. H. Alamiry; Harry Adams; Simon J. A. Pope; Stephen Faulkner; Julia A. Weinstein; Michael D. Ward Anthracene as a sensitiser for near-infrared luminescence in complexes of Nd(iii), Er(iii) and Yb(iii): an unexpected sensitisation mechanism based on electron transfer, Dalton Transactions (2007) no. 15, p. 1484 | DOI:10.1039/b700714k
  • Debao Zhang; Setsuhisa Tanabe Study on Upconversion Characteristics of Silica-Based Erbium-Doped Fibers Using Integrating Sphere, Japanese Journal of Applied Physics, Volume 46 (2007) no. 10R, p. 6676 | DOI:10.1143/jjap.46.6676
  • Hai Lin; Xueying Wang; Lin Lin; Changmin Li; Dianlai Yang; Setsuhisa Tanabe Near-infrared emission character of Tm3+-doped heavy metal tellurite glasses for optical amplifiers and 1.8 µm infrared laser, Journal of Physics D: Applied Physics, Volume 40 (2007) no. 12, p. 3567 | DOI:10.1088/0022-3727/40/12/004
  • M Tsvirko; S Meshkova; G Kiriiak; V Gorodnyuk Detection of dysprosium (III) in the presence of terbium (III) by using the time-resolved luminescence, Journal of Physics: Conference Series, Volume 79 (2007), p. 012007 | DOI:10.1088/1742-6596/79/1/012007
  • Tao Li; Qin Yuan Zhang; Zhou Ming Feng; Yue Hui Liu; Zai De Deng; Zhong Hong Jiang Effect of Alkaline-Earth Metal Composition on Spectroscopic Properties of Er3+ in Fluorophosphate Glasses, Key Engineering Materials, Volume 280-283 (2007), p. 957 | DOI:10.4028/www.scientific.net/kem.280-283.957
  • Theodore Lazarides; Graham M. Davies; Harry Adams; Cristiana Sabatini; Francesco Barigelletti; Andrea Barbieri; Simon J. A. Pope; Stephen Faulkner; Michael D. Ward Ligand-field excited states of hexacyanochromate and hexacyanocobaltate as sensitisers for near-infrared luminescence from Nd(iii) and Yb(iii) in cyanide-bridged d–f assemblies, Photochemical Photobiological Sciences, Volume 6 (2007) no. 11, p. 1152 | DOI:10.1039/b708683k
  • Setsuhisa Tanabe Novel Oxide Glass and Glass Ceramic Materials for Optical Amplifier, Advances in Photonic Materials and Devices, Volume 163 (2006), p. 1 | DOI:10.1002/9781118407233.ch1
  • Tanya K. Ronson; Theodore Lazarides; Harry Adams; Simon J. A. Pope; Daniel Sykes; Stephen Faulkner; Simon J. Coles; Michael B. Hursthouse; William Clegg; Ross W. Harrington; Michael D. Ward Luminescent PtII(bipyridyl)(diacetylide) Chromophores with Pendant Binding Sites as Energy Donors for Sensitised Near‐Infrared Emission from Lanthanides: Structures and Photophysics of PtII/LnIII Assemblies, Chemistry – A European Journal, Volume 12 (2006) no. 36, p. 9299 | DOI:10.1002/chem.200600698
  • Juan-Manuel Herrera; Simon J. A. Pope; Harry Adams; Stephen Faulkner; Michael D. Ward Structural and Photophysical Properties of Coordination Networks Combining [Ru(Bpym)(CN)4]2- or [Ru(CN)42(μ-bpym)]4- Anions (bpym = 2,2‘-Bipyrimidine) with Lanthanide(III) Cations:  Sensitized Near-Infrared Luminescence from Yb(III), Nd(III), and Er(III) Following Ru-to-Lanthanide Energy Transfer, Inorganic Chemistry, Volume 45 (2006) no. 10, p. 3895 | DOI:10.1021/ic0521574
  • Setsuhisa Tanabe Optical properties and local structure of rare-earth-doped amplifier for broadband telecommunication, Journal of Alloys and Compounds, Volume 408-412 (2006), p. 675 | DOI:10.1016/j.jallcom.2005.01.106
  • A. Sennaroglu; I. Kabalci; A. Kurt; U. Demirbas; G. Ozen Spectroscopic properties of Tm3+:TeO2–PbF2 glasses, Journal of Luminescence, Volume 116 (2006) no. 1-2, p. 79 | DOI:10.1016/j.jlumin.2005.03.006
  • Hiromichi Takebe; Takashi Harada; Makoto Kuwabara Effect of B2O3 addition on the thermal properties and density of barium phosphate glasses, Journal of Non-Crystalline Solids, Volume 352 (2006) no. 6-7, p. 709 | DOI:10.1016/j.jnoncrysol.2005.11.066
  • Ren Jing; Yang Guang; Zeng Huidan; Zhang Xianghua; Yang Yunxia; Chen Guorong Properties of Dy3+‐Doped Ge–As–Ga–Se Chalcogenide Glasses, Journal of the American Ceramic Society, Volume 89 (2006) no. 8, p. 2486 | DOI:10.1111/j.1551-2916.2006.01070.x
  • Isabella-Ioana Oprea; Hartmut Hesse; Klaus Betzler Luminescence of erbium-doped bismuth–borate glasses, Optical Materials, Volume 28 (2006) no. 10, p. 1136 | DOI:10.1016/j.optmat.2005.07.004
  • V. Nazabal; P. Němec; J. Jedelský; C. Duverger; J. Le Person; J.L. Adam; M. Frumar Dysprosium doped amorphous chalcogenide films prepared by pulsed laser deposition, Optical Materials, Volume 29 (2006) no. 2-3, p. 273 | DOI:10.1016/j.optmat.2005.08.034
  • Hai Lin; Ke Liu; Lin Lin; Yanyan Hou; Dianlai Yang; Tiecheng Ma; Edwin Yun Bun Pun; Qingda An; Jiayou Yu; Setsuhisa Tanabe Optical parameters and upconversion fluorescence in Tm3+/Yb3+-doped alkali-barium-bismuth-tellurite glasses, Spectrochimica Acta Part A: Molecular and Biomolecular Spectroscopy, Volume 65 (2006) no. 3-4, p. 702 | DOI:10.1016/j.saa.2005.12.034
  • Akira Saitoh; Shuhei Murata; Satoru Matsuishi; Masanori Oto; Taisuke Miura; Masahiro Hirano; Hideo Hosono Elucidation of Phosphorus Co-doping Effect on Photoluminescence in Ce3+-activated SiO2 Glasses: Determination of Solvation Shell Structure by Pulsed EPR, Chemistry Letters, Volume 34 (2005) no. 8, p. 1116 | DOI:10.1246/cl.2005.1116
  • Nail M. Shavaleev; Gianluca Accorsi; Dalia Virgili; Zöe R. Bell; Theodore Lazarides; Giuseppe Calogero; Nicola Armaroli; Michael D. Ward Syntheses and Crystal Structures of Dinuclear Complexes Containing d-Block and f-Block Luminophores. Sensitization of NIR Luminescence from Yb(III), Nd(III), and Er(III) Centers by Energy Transfer from Re(I)− and Pt(II)−Bipyrimidine Metal Centers, Inorganic Chemistry, Volume 44 (2005) no. 1, p. 61 | DOI:10.1021/ic048875s
  • Graham M. Davies; Simon J. A. Pope; Harry Adams; Stephen Faulkner; Michael D. Ward Structural and Photophysical Properties of Coordination Networks Combining [Ru(bipy)(CN)4]2- Anions and Lanthanide(III) Cations:  Rates of Photoinduced Ru-to-Lanthanide Energy Transfer and Sensitized Near-Infrared Luminescence, Inorganic Chemistry, Volume 44 (2005) no. 13, p. 4656 | DOI:10.1021/ic050512k
  • Luciano A. Bueno; Anderson S.L. Gomes; Y. Messaddeq; Celso V. Santilli; Jeanette Dexpert-Ghys; S.J.L. Ribeiro Tm3+ and Tm3+–Ho3+ doped fluorogermanate glasses for S-band amplifiers, Journal of Non-Crystalline Solids, Volume 351 (2005) no. 21-23, p. 1743 | DOI:10.1016/j.jnoncrysol.2005.04.007
  • Jennifer Gordon; John Ballato; Dennis W. Smith Jr.; Jianyong Jin Optical properties of perfluorocyclobutyl polymers III Spectroscopic characterization of rare-earth-doped perfluorocyclobutyl polymers, Journal of the Optical Society of America B, Volume 22 (2005) no. 8, p. 1654 | DOI:10.1364/josab.22.001654
  • L.R. Moorthy; M. Jayasimhadri; A. Radhapathy; R.V.S.S.N. Ravikumar Lasing properties of Pr3+-doped tellurofluorophosphate glasses, Materials Chemistry and Physics, Volume 93 (2005) no. 2-3, p. 455 | DOI:10.1016/j.matchemphys.2005.03.035
  • Graham M. Davies; Harry Adams; Simon J. A. Pope; Stephen Faulkner; Michael D. Ward Photophysical properties of Pr(iii) and Er(iii) complexes of poly(pyrazolyl)borates, Photochemical Photobiological Sciences, Volume 4 (2005) no. 10, p. 829 | DOI:10.1039/b508382f
  • G. A. Kumar; Richard Riman; Elias Snitzer; John Ballato Solution synthesis and spectroscopic characterization of high Er3+ content LaF3 for broadband 1.5 μm amplification, Journal of Applied Physics, Volume 95 (2004) no. 1, p. 40 | DOI:10.1063/1.1629772
  • S.J.L. Ribeiro; C.C. Araújo; L.A. Bueno; R.R. Gonçalves; Y. Messaddeq Sol–gel Eu3+/Tm3+ doped transparent glass–ceramic waveguides, Journal of Non-Crystalline Solids, Volume 348 (2004), p. 180 | DOI:10.1016/j.jnoncrysol.2004.08.164
  • Determination of optical properties of Pr3+-doped selenide glasses of Ge-Sb-Se system using spectroscopic ellipsometry, Korean Journal of Optics and Photonics, Volume 14 (2003) no. 6, p. 594 | DOI:10.3807/kjop.2003.14.6.594

Cité par 199 documents. Sources : Crossref

Commentaires - Politique

Ces articles pourraient vous intéresser

Compositional effects and spectroscopy of rare earths (Er3+, Tm3+, and Nd3+) in tellurite glasses

Shaoxiong Shen; Animesh Jha; Edward Zhang; ...

C. R. Chim (2002)

Rare-earth-doped transparent glass ceramics

M. Clara Gonçalves; Luís F. Santos; Rui M. Almeida

C. R. Chim (2002)

1.3-μm-emission properties and local structure of Dy3+ in chalcohalide glasses

Jong Heo

C. R. Chim (2002)